Difference between revisions of "Aufgaben:Exercise 2.07Z: Reed-Solomon Code (15, 5, 11) to Base 16"
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Definition_and_Properties_of_Reed-Solomon_Codes}} |
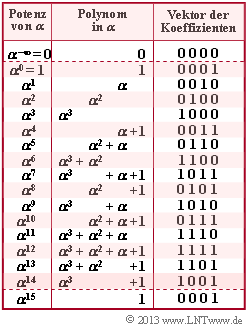
| − | [[File: P_ID2534__KC_Z_2_7.png|right|frame|$\rm GF(2^4)$ in | + | [[File: P_ID2534__KC_Z_2_7.png|right|frame|$\rm GF(2^4)$ in power, polynomial and coefficient notation]] |
| − | + | The task at hand is similar to the one in [[Aufgaben:Exercise_2.07:_Reed-Solomon_Code_(7,_3,_5)_to_Base_8|"Exercise 2.7"]]. However, we now refer here to the Galois field $\rm GF(2^4)$, whose elements are given opposite both in powers and polynomial representations and by the coefficient vector. Further, in $\rm GF(2^4)$: | |
:$$\alpha^{16} = \alpha^{1}\hspace{0.05cm},\hspace{0.2cm} \alpha^{17} = \alpha^{2}\hspace{0.05cm},\hspace{0.2cm} | :$$\alpha^{16} = \alpha^{1}\hspace{0.05cm},\hspace{0.2cm} \alpha^{17} = \alpha^{2}\hspace{0.05cm},\hspace{0.2cm} | ||
\alpha^{18} = \alpha^{3}\hspace{0.05cm},\hspace{0.05cm}\text{...} $$ | \alpha^{18} = \alpha^{3}\hspace{0.05cm},\hspace{0.05cm}\text{...} $$ | ||
| − | + | To encode the information block of length $k = 5$, | |
:$$\underline{u} = (u_0,\ u_1,\ u_2,\ u_3,\ u_4)\hspace{0.05cm},$$ | :$$\underline{u} = (u_0,\ u_1,\ u_2,\ u_3,\ u_4)\hspace{0.05cm},$$ | ||
| − | + | we form the polynomial | |
:$$u(x) = u_0 + u_1 \cdot x + u_2 \cdot x^2 + u_3 \cdot x^3 + u_4 \cdot x^4 $$ | :$$u(x) = u_0 + u_1 \cdot x + u_2 \cdot x^2 + u_3 \cdot x^3 + u_4 \cdot x^4 $$ | ||
| − | + | with $u_0, \hspace{0.05cm}\text{...} \hspace{0.1cm} , u_4 ∈ \rm GF(2^4)$. | |
| − | + | The $n = 15$ codewords are then obtained by substituting into $u(x)$ the elements of $\rm GF(2^4) \ \backslash \ \{0\}$ : | |
:$$c_0 = u(\alpha^{0})\hspace{0.05cm},\hspace{0.2cm} c_1 = u(\alpha^{1})\hspace{0.05cm}, | :$$c_0 = u(\alpha^{0})\hspace{0.05cm},\hspace{0.2cm} c_1 = u(\alpha^{1})\hspace{0.05cm}, | ||
\hspace{0.2cm} c_2 = u(\alpha^{2})\hspace{0.05cm}, | \hspace{0.2cm} c_2 = u(\alpha^{2})\hspace{0.05cm}, | ||
| Line 26: | Line 26: | ||
| − | + | Hints: | |
| − | * | + | * This exercise belongs to the chapter [[Channel_Coding/Definition_and_Properties_of_Reed-Solomon_Codes| "Definition and Properties of Reed-Solomon Codes"]]. |
| Line 33: | Line 33: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {How many symbol errors can be corrected? |
|type="{}"} | |type="{}"} | ||
$t \ = \ $ { 5 } | $t \ = \ $ { 5 } | ||
| − | { | + | {What is the polynomial $u(x)$ for $\underline{u} = (\alpha^3, \, 0, \, 0, \, 1, \, \alpha^{10})$? |
|type="()"} | |type="()"} | ||
- $u(x) = \alpha^3 + x + \alpha^{10} \cdot x^2$, | - $u(x) = \alpha^3 + x + \alpha^{10} \cdot x^2$, | ||
| Line 45: | Line 45: | ||
- $u(x) = 1 + x + x^2 + x^3 + x^4$. | - $u(x) = 1 + x + x^2 + x^3 + x^4$. | ||
| − | { | + | {What is the symbol $c_0$ of the associated codeword $\underline{c}$? |
|type="()"} | |type="()"} | ||
- $c_0 = 1$, | - $c_0 = 1$, | ||
| Line 52: | Line 52: | ||
- $c_0 = \alpha^{14}$. | - $c_0 = \alpha^{14}$. | ||
| − | { | + | {What is the symbol $c_1$ of the associated codeword $\underline{c}$? |
|type="()"} | |type="()"} | ||
- $c_1 = 1$, | - $c_1 = 1$, | ||
| Line 59: | Line 59: | ||
+ $c_1 = \alpha^{14}$. | + $c_1 = \alpha^{14}$. | ||
| − | { | + | {What is the symbol $c_{13}$ of the associated codeword $\underline{c}$? |
|type="()"} | |type="()"} | ||
- $c_{13} = 1$, | - $c_{13} = 1$, | ||
| Line 66: | Line 66: | ||
- $c_{13} = \alpha^{14}$. | - $c_{13} = \alpha^{14}$. | ||
| − | { | + | {What is the last symbol of the associated codeword $\underline{c}$? |
|type="()"} | |type="()"} | ||
- $c_{15} = 1$, | - $c_{15} = 1$, | ||
| Line 73: | Line 73: | ||
- $c_{14} = \alpha^{14}$. | - $c_{14} = \alpha^{14}$. | ||
| − | { | + | {Which statements are true? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The code symbol "$0$" is not possible for $\rm RSC \, (15, \, 5, \, 11)_{16}$ . |
| − | - | + | - A code symbol "$0$" results only for $\underline{u} = (0, \, 0, \, 0, \, 0)$. |
| − | + | + | + Also for $\underline{u} ≠ (0, \, 0, \, 0, \, 0)$ there can be code symbols "$0$". |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' From $n = 15$ and $k = 5$ follows: |
:$$d_{\rm min} = n - k +1 = 15 - 5 + 1 = 11 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | :$$d_{\rm min} = n - k +1 = 15 - 5 + 1 = 11 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ||
t = \frac{d_{\rm min}-1}{2}\hspace{0.15cm}\underline {=5}\hspace{0.05cm}.$$ | t = \frac{d_{\rm min}-1}{2}\hspace{0.15cm}\underline {=5}\hspace{0.05cm}.$$ | ||
| Line 88: | Line 88: | ||
| − | '''(2)''' | + | '''(2)''' In general, for the sought polynomial $u(x)$ with $k = 5$: |
:$$u(x) = \sum_{i = 0}^{k-1} u_i \cdot x^{i}= u_0 + u_1 \cdot x + u_2 \cdot x^2 + u_3 \cdot x^3 + | :$$u(x) = \sum_{i = 0}^{k-1} u_i \cdot x^{i}= u_0 + u_1 \cdot x + u_2 \cdot x^2 + u_3 \cdot x^3 + | ||
u_4 \cdot x^4 \hspace{0.05cm}.$$ | u_4 \cdot x^4 \hspace{0.05cm}.$$ | ||
| − | + | For $u_0 = \alpha^3, \ u_1 = u_2 = 0, \ u_3 = 1$ and $u_4 = \alpha^{10}$ the <u>proposed solution 2</u> turns out to be correct. | |
| − | '''(3)''' | + | '''(3)''' It holds $c_0 = u(\alpha^0) = u(1)$: |
:$$c_0 = \alpha^{3} + 1 \cdot 1^3 + \alpha^{10} \cdot 1^{4} = (1000) + (0001) + (0111) = (1110)= \alpha^{11} | :$$c_0 = \alpha^{3} + 1 \cdot 1^3 + \alpha^{10} \cdot 1^{4} = (1000) + (0001) + (0111) = (1110)= \alpha^{11} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The correct solution is therefore <u>proposed solution 3</u>. | |
| − | '''(4)''' | + | '''(4)''' From $c_1 = u(\alpha)$ one obtains the <u>proposed solution 4</u>. |
:$$c_1 = u(\alpha^{1}) =\alpha^{3} +1 \cdot \alpha^{3} + \alpha^{10} \cdot \alpha^{4} = \alpha^{14} | :$$c_1 = u(\alpha^{1}) =\alpha^{3} +1 \cdot \alpha^{3} + \alpha^{10} \cdot \alpha^{4} = \alpha^{14} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| Line 110: | Line 110: | ||
| − | '''(5)''' | + | '''(5)''' For the penultimate symbol $c_{13} = u(\alpha^{13})$ holds: |
:$$c_{13} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u(\alpha^{13}) =\alpha^{3} + 1 \cdot \alpha^{13 \hspace{0.05cm}\cdot \hspace{0.05cm}3} + \alpha^{10} \cdot \alpha^{13 \hspace{0.05cm}\cdot \hspace{0.05cm}4} = \alpha^{3} + \alpha^{39}+ \alpha^{62} =\alpha^{3} + \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}2} \cdot \alpha^{9}+ \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}4} \cdot \alpha^{2} = | :$$c_{13} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u(\alpha^{13}) =\alpha^{3} + 1 \cdot \alpha^{13 \hspace{0.05cm}\cdot \hspace{0.05cm}3} + \alpha^{10} \cdot \alpha^{13 \hspace{0.05cm}\cdot \hspace{0.05cm}4} = \alpha^{3} + \alpha^{39}+ \alpha^{62} =\alpha^{3} + \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}2} \cdot \alpha^{9}+ \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}4} \cdot \alpha^{2} = | ||
\alpha^{3} + \alpha^{9} + \alpha^{2}$$ | \alpha^{3} + \alpha^{9} + \alpha^{2}$$ | ||
| Line 116: | Line 116: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The correct solution is therefore <u>proposed solution 2</u>. | |
| − | '''(6)''' | + | '''(6)''' The last code symbol is $c_{14} = u(\alpha^{14})$: |
:$$c_{14} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u(\alpha^{14}) =\alpha^{3} + 1 \cdot \alpha^{14 \hspace{0.05cm}\cdot \hspace{0.05cm}3} + \alpha^{10} \cdot \alpha^{14 \hspace{0.05cm}\cdot \hspace{0.05cm}4} = \alpha^{3} + \alpha^{42}+ \alpha^{66} =\alpha^{3} + \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}2} \cdot \alpha^{12}+ \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}4} \cdot \alpha^{6} = | :$$c_{14} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u(\alpha^{14}) =\alpha^{3} + 1 \cdot \alpha^{14 \hspace{0.05cm}\cdot \hspace{0.05cm}3} + \alpha^{10} \cdot \alpha^{14 \hspace{0.05cm}\cdot \hspace{0.05cm}4} = \alpha^{3} + \alpha^{42}+ \alpha^{66} =\alpha^{3} + \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}2} \cdot \alpha^{12}+ \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}4} \cdot \alpha^{6} = | ||
\alpha^{3} + \alpha^{12} + \alpha^{6} =$$ | \alpha^{3} + \alpha^{12} + \alpha^{6} =$$ | ||
| Line 126: | Line 126: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The correct solution is therefore <u>proposed solution 3</u>. | |
| − | '''(7)''' | + | '''(7)''' The code symbol "$0$" occurs just as often as all other symbols "$\alpha^i$" ⇒ <u>proposed solution 3</u>. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 18:16, 2 September 2022
The task at hand is similar to the one in "Exercise 2.7". However, we now refer here to the Galois field $\rm GF(2^4)$, whose elements are given opposite both in powers and polynomial representations and by the coefficient vector. Further, in $\rm GF(2^4)$:
- $$\alpha^{16} = \alpha^{1}\hspace{0.05cm},\hspace{0.2cm} \alpha^{17} = \alpha^{2}\hspace{0.05cm},\hspace{0.2cm} \alpha^{18} = \alpha^{3}\hspace{0.05cm},\hspace{0.05cm}\text{...} $$
To encode the information block of length $k = 5$,
- $$\underline{u} = (u_0,\ u_1,\ u_2,\ u_3,\ u_4)\hspace{0.05cm},$$
we form the polynomial
- $$u(x) = u_0 + u_1 \cdot x + u_2 \cdot x^2 + u_3 \cdot x^3 + u_4 \cdot x^4 $$
with $u_0, \hspace{0.05cm}\text{...} \hspace{0.1cm} , u_4 ∈ \rm GF(2^4)$.
The $n = 15$ codewords are then obtained by substituting into $u(x)$ the elements of $\rm GF(2^4) \ \backslash \ \{0\}$ :
- $$c_0 = u(\alpha^{0})\hspace{0.05cm},\hspace{0.2cm} c_1 = u(\alpha^{1})\hspace{0.05cm}, \hspace{0.2cm} c_2 = u(\alpha^{2})\hspace{0.05cm}, \hspace{0.15cm} ... \hspace{0.15cm},\hspace{0.20cm} c_{14} = u(\alpha^{14})\hspace{0.05cm}.$$
Hints:
- This exercise belongs to the chapter "Definition and Properties of Reed-Solomon Codes".
Questions
Solution
- $$d_{\rm min} = n - k +1 = 15 - 5 + 1 = 11 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} t = \frac{d_{\rm min}-1}{2}\hspace{0.15cm}\underline {=5}\hspace{0.05cm}.$$
(2) In general, for the sought polynomial $u(x)$ with $k = 5$:
- $$u(x) = \sum_{i = 0}^{k-1} u_i \cdot x^{i}= u_0 + u_1 \cdot x + u_2 \cdot x^2 + u_3 \cdot x^3 + u_4 \cdot x^4 \hspace{0.05cm}.$$
For $u_0 = \alpha^3, \ u_1 = u_2 = 0, \ u_3 = 1$ and $u_4 = \alpha^{10}$ the proposed solution 2 turns out to be correct.
(3) It holds $c_0 = u(\alpha^0) = u(1)$:
- $$c_0 = \alpha^{3} + 1 \cdot 1^3 + \alpha^{10} \cdot 1^{4} = (1000) + (0001) + (0111) = (1110)= \alpha^{11} \hspace{0.05cm}.$$
The correct solution is therefore proposed solution 3.
(4) From $c_1 = u(\alpha)$ one obtains the proposed solution 4.
- $$c_1 = u(\alpha^{1}) =\alpha^{3} +1 \cdot \alpha^{3} + \alpha^{10} \cdot \alpha^{4} = \alpha^{14} \hspace{0.05cm}.$$
(5) For the penultimate symbol $c_{13} = u(\alpha^{13})$ holds:
- $$c_{13} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u(\alpha^{13}) =\alpha^{3} + 1 \cdot \alpha^{13 \hspace{0.05cm}\cdot \hspace{0.05cm}3} + \alpha^{10} \cdot \alpha^{13 \hspace{0.05cm}\cdot \hspace{0.05cm}4} = \alpha^{3} + \alpha^{39}+ \alpha^{62} =\alpha^{3} + \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}2} \cdot \alpha^{9}+ \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}4} \cdot \alpha^{2} = \alpha^{3} + \alpha^{9} + \alpha^{2}$$
- $$\Rightarrow \hspace{0.3cm}c_{13} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} (1000) + (1010) + (0100) = (0110) = \alpha^{5} \hspace{0.05cm}.$$
The correct solution is therefore proposed solution 2.
(6) The last code symbol is $c_{14} = u(\alpha^{14})$:
- $$c_{14} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u(\alpha^{14}) =\alpha^{3} + 1 \cdot \alpha^{14 \hspace{0.05cm}\cdot \hspace{0.05cm}3} + \alpha^{10} \cdot \alpha^{14 \hspace{0.05cm}\cdot \hspace{0.05cm}4} = \alpha^{3} + \alpha^{42}+ \alpha^{66} =\alpha^{3} + \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}2} \cdot \alpha^{12}+ \alpha^{15 \hspace{0.05cm}\cdot \hspace{0.05cm}4} \cdot \alpha^{6} = \alpha^{3} + \alpha^{12} + \alpha^{6} =$$
- $$\Rightarrow \hspace{0.3cm}c_{14} \hspace{-0.15cm} \ = \ \hspace{-0.15cm}(1000) + (1111) + (1100) = (1011) = \alpha^{7} \hspace{0.05cm}.$$
The correct solution is therefore proposed solution 3.
(7) The code symbol "$0$" occurs just as often as all other symbols "$\alpha^i$" ⇒ proposed solution 3.