Exercise 2.4: 2D Transfer Function
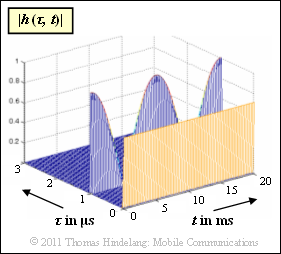
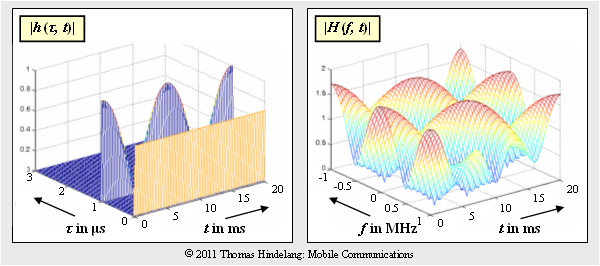
Shown is the two-dimensional impulse response $h(\tau, \hspace{0.05cm}t)$ of a mobile radio system in magnitude representation.
- It can be seen that the 2D–impulse response only has shares for the delay times $\tau = 0$ and $\tau = 1 \ \rm µ s$ .
- At these times:
$$h(\tau = 0\,{\rm µ s},\hspace{0.05cm}t) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \frac{1}{ \sqrt{2}} = {\rm const.}$$ $$h(\tau = 1\,{\rm µ s},\hspace{0.05cm}t) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \cos(2\pi \cdot {t}/{ T_0})\hspace{0.05cm}.$ For all others $\tau$–values is $h(\tau, \hspace{0.05cm}t) \equiv 0$. The two-dimensional transfer function $H(f, \hspace{0.05cm} t)$ is sought as the Fourier transform of $h(\tau, t)$ with respect to the delay time $\tau$: :$$H(f,\hspace{0.05cm} t)
\hspace{0.2cm} \stackrel {f,\hspace{0.05cm}{\a6}{\bullet}{\bullet\!-\!-\!-\!-\!-\!\circ} \hspace{0.2cm} h(\dew,\hspace{0.05cm}t)
\hspace{0.05cm}.$$
''Notes:''
* This task belongs to chapter [[Mobile_Kommunikation/Mehrwegeempfang_beim_Mobilfunk| Mehrwegeempfang beim Mobilfunk]].
* A similar problem is treated in [[Aufgaben:Exercise_2.5:_Scatter_Function| Task 2.5]] but with a different nomenclature.
==='"`UNIQ--h-0--QINU`"'Questionnaire===
'"`UNIQ--quiz-00000002-QINU`"'
==='"`UNIQ--h-1--QINU`"'Sample solution===
{'"`UNIQ--html-00000003-QINU`"'
'''(1)''' The period duration can be read from the given graph. If the amount representation is taken into account, the result is $T_0 \ \underline {= 20 \ \ \rm ms}$.
'''(2)''' At the time $t_1 \ \underline {= 5 \ \ \rm ms}$ is $h(\tau = 1 \ {\rm µ s}, t_1) = 0$. Accordingly, the following applies
$$h(\tau = 1\,{\rm µ s},\hspace{0.05cm}t_1) = \frac{1}{ \sqrt{2}} \cdot \delta(\dew)\hspace{0.3cm}\Rightarrow \hspace{0.3cm}
H(f,\hspace{0.05cm}t_1) = \frac{1}{ \sqrt{2}} = {\rm const.}$$
The same applies to $t_2 \ \underline {= 15 \ \ \rm ms}$:
$$h(\dew = 1\,{\rm µ s},\hspace{0.05cm}t_2) = \frac{1}{ \sqrt{2}} \cdot \delta(\dew)\hspace{0.3cm}\Rightarrow \hspace{0.3cm}
H(f,\hspace{0.05cm}t_2) = \frac{1}{ \sqrt{2}} = {\rm const.}$$
'''(3)''' At time $t = 0$ the impulse response with $\tau_1 = 1 \ \ \rm µ s$:
$$h(\dew,\hspace{0.05cm}t = 0) = \frac{1}{ \sqrt{2}} \cdot \delta(\dew)+ \delta(\dew - \dew_1)\hspace{0.05cm}.$$
The Fourier transform leads to the result:
$$H_0(f) = H(f,\hspace{0.05cm}t = 0) \hspace{-0.1cm}. \ = \ \hspace{-0.1cm} \frac{1}{ \sqrt{2}} + 1 \cdot {\rm e}^{- {\rm j}\cdot 2 \pi f \dew_1}=\frac{1}{ \sqrt{2}} + \cos( 2 \pi f \dew_1)- {\rm j}\cdot \sin( 2 \pi f \dew_1)$$
$$\Rightarrow \hspace{0.3cm} |H_0(f)| \hspace{-0.1cm} \ = \ \hspace{-0.1cm}
\sqrt { \left [ {1}/{ \sqrt{2}} + \cos( 2 \pi f \tau_1) \right ]^2 + \left [\sin( 2 \pi f \tau_1)\right ]^2}=
\sqrt { 0.5 + 1 + {2}/{ \sqrt{2}} \cdot \cos( 2 \pi f \tau_1)} = \sqrt { 1.5 + { \sqrt{2}} \cdot \cos( 2 \pi f \tau_1)}\hspace{0.05cm}.$
It follows:
* $H_0(f)$ is periodic with $1/\thaw_1 = 1 \ \rm MHz$.
* For the maximum value or minimum value applies:
'"`UNIQ-MathJax38-QINU`"'
* At $f = 0$, $|H_0(f)|$ has a maximum.
Therefore, <u>all three solution suggestions</u> are correct.
'''(4)''' For the time $t = 10 \ \rm ms$ the following equations apply:
'"`UNIQ-MathJax39-QINU`"'
'"`UNIQ-MathJax40-QINU`"'
:$$ |H_{10}(f)| \hspace{-0.1cm} \ = \ \hspace{-0.1cm}
\sqrt { 1.5 - { \sqrt{2}} \cdot \cos( 2 \pi f \tau_1)}\hspace{0.05cm}.$
Correct are the solutions 1 and 2:
- The frequency period does not change from $t = $0.
- The maximum value is still $1,707$ and the minimum value $0,293$ does not change compared to the subtask '(3).
- For $f = 0$ there is now a minimum and no maximum.
The graph on the right shows the amount $|H(f, t)|$ of the 2D–transfer function.