Difference between revisions of "Aufgaben:Exercise 2.5Z: Multi-Path Scenario"
(Die Seite wurde neu angelegt: „ {{quiz-Header|Buchseite=Mobile Kommunikation/Das GWSSUS–Kanalmodell}} [[File:P_ID2169__Mob_Z_2_5.png|right|frame|Mobilfunk–Szenario mit drei Pfaden]…“) |
m (Text replacement - "Category:Exercises for Mobile Communications" to "Category:Mobile Communications: Exercises") |
||
| (24 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Mobile_Communications/The_GWSSUS_Channel_Model}} |
| − | [[File: | + | [[File:EN_Mob_A_2_5Z.png|right|frame|Mobile radio scenario with three paths]] |
| − | In [[Aufgaben: | + | In [[Aufgaben:Exercise_2.5:_Scatter_Function| Exercise 2.5]], a delay–Doppler function (or scatter function) was given. From this, you should calculate and interpret the other system functions. The given scatter function $s(\tau_0, f_{\rm D})$ was |
:$$s(\tau_0, f_{\rm D}) =\frac{1}{\sqrt{2}} \cdot \delta (\tau_0) \cdot \delta (f_{\rm D} - 100\,{\rm Hz}) \ - \ $$ | :$$s(\tau_0, f_{\rm D}) =\frac{1}{\sqrt{2}} \cdot \delta (\tau_0) \cdot \delta (f_{\rm D} - 100\,{\rm Hz}) \ - \ $$ | ||
| − | :$$\hspace{1.5cm} \ - \ \hspace{-0.2cm} \frac{1}{2} \cdot \delta (\tau_0 \hspace{-0.05cm}- \hspace{-0.05cm}1\,{\rm \mu s}) \cdot \delta (f_{\rm D} \hspace{-0.05cm}- \hspace{-0.05cm}50\,{\rm Hz}) \ - | + | :$$\hspace{1.5cm} \ - \ \hspace{-0.2cm} \frac{1}{2} \cdot \delta (\tau_0 \hspace{-0.05cm}- \hspace{-0.05cm}1\,{\rm \mu s}) \cdot \delta (f_{\rm D} \hspace{-0.05cm}- \hspace{-0.05cm}50\,{\rm Hz}) \ - \frac{1}{2} \cdot \delta (\tau_0 \hspace{-0.05cm}- \hspace{-0.05cm}1\,{\rm \mu s}) \cdot \delta (f_{\rm D}\hspace{-0.05cm} + \hspace{-0.05cm}50\,{\rm Hz}) |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | '' | + | ''Note:'' In our learning tutorial, $s(\tau_0, \hspace{0.05cm} f_{\rm D})$ is also identified with $\eta_{\rm VD}(\tau_0, \hspace{0.05cm}f_{\rm D})$. |
| − | + | Here we have replaced the delay variable $\tau$ with $\tau_0$ . The new variable $\tau_0$ describes the difference between the delay of a path and the delay $\tau_1$ of the main path. The main path is thus identified in the above equation by $\tau_0 = 0$. | |
| − | + | Now, we try to find a mobile radio scenario in which this scatter function would actually occur. The basic structure is sketched above as a top view, and the following hold: | |
| − | * | + | * A single frequency is transmitted: $f_{\rm S} = 2 \ \rm GHz$. |
| − | * | + | * The mobile receiver $\rm (E)$ is represented here by a yellow dot. It is not known whether the vehicle is stationary, moving towards the transmitter $\rm (S)$ or moving away. |
| − | * | + | * The signal reaches the receiver via a main path (red) and two secondary paths (blue and green). Reflections from the obstacles cause phase shifts of $\pi$. |
| − | * ${\rm S}_2$ | + | * ${\rm S}_2$ and ${\rm S}_3$ are to be understood here as fictitious transmitters from whose position the angles of incidence $\alpha_2$ and $\alpha_3$ of the secondary paths can be determined. |
| − | * | + | * Let the signal frequency be $f_{\rm S}$, the angle of incidence $\alpha$, the velocity $v$ and the velocity of light $c = 3 \cdot 10^8 \ \rm m/s$. Then, the Doppler frequency is |
:$$f_{\rm D}= {v}/{c} \cdot f_{\rm S} \cdot \cos(\alpha) | :$$f_{\rm D}= {v}/{c} \cdot f_{\rm S} \cdot \cos(\alpha) | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | * The damping factors $k_1$, $k_2$ and $k_3$ are inversely proportional to the path lengths $d_1$, $d_2$ and $d_3$. This corresponds to the path loss exponent $\gamma = 2$. |
| − | * | + | *This means: The signal power decreases quadratically with distance $d$ and accordingly the signal amplitude decreases linearly with $d$. |
| Line 30: | Line 30: | ||
| − | '' | + | ''Notes:'' |
| − | * | + | * This task belongs to chapter [[Mobile_Communications/The_GWSSUS_Channel_Model| The GWSSUS Channel Model]]. |
| − | * | + | *We also refer to the pages [[Mobile_Communications/Distance_Dependent_Attenuation_and_Shading#Common_path_loss_model| Common path-loss model]] and [[Mobile_Communications/Statistical_Bindings_within_the_Rayleigh_Process#Doppler_frequency_and_its_distribution| Doppler frequency and its distribution]]. |
| − | === | + | ===Questionnaire=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {At first, consider only the Dirac function at $\tau = 0$ and $f_{\rm D} = 100 \ \rm Hz$. Which statements apply to the receiver? |
|type="()"} | |type="()"} | ||
| − | - | + | - The receiver is standing. |
| − | + | + | + The receiver moves directly towards the transmitter. |
| − | - | + | - The receiver moves away in the opposite direction to the transmitter. |
| − | { | + | {What is the vehicle speed? |
|type="{}"} | |type="{}"} | ||
| − | $v \ = \ ${ 54 3% } $\ \rm km/h$ | + | $v \ = \ ${ 54 3% } $\ \ \rm km/h$ |
| − | { | + | {Which statements apply to the Dirac at $\tau_0 = 1 \ \ \rm µ s$ and $f_{\rm D} = +50 \ \ \rm Hz$? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + This Dirac comes from the blue path. |
| − | - | + | - This Dirac comes from the green path. |
| − | - | + | - The angle is $30^\circ$. |
| − | + | + | + The angle is $60^\circ$. |
| − | { | + | {What statements apply to the green path? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + We have $\tau_0 = 1 \ \rm µ s$ and $f_{\rm D} = -50 \ \rm Hz$. |
| − | - | + | - The angle $\alpha_3$ (see graph) is $60^\circ$. |
| − | + | + | + The angle $\alpha_3$ is $240^\circ$. |
| − | { | + | {Which of the following relations hold between the two side paths? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + $d_3 = d_2$. |
| − | + | + | + $k_3 = k_2$. |
| − | + | + | + $\tau_3 = \tau_2$. |
| − | { | + | {What is the difference $\Delta d = d_2 - d_1$ in time? |
|type="{}"} | |type="{}"} | ||
| − | $\Delta d \ = \ ${ 300 3% } $\ \rm m$ | + | $\Delta d \ = \ ${ 300 3% } $\ \ \rm m$ |
| − | { | + | {What is the relationship between $d_2$ and $d_1$? |
|type="{}"} | |type="{}"} | ||
| − | $d_2/d_1 \ = \ ${ 1 | + | $d_2/d_1 \ = \ ${ 1,414 3% } |
| − | { | + | {Find the distances $d_1$ and $d_2$ . |
|type="{}"} | |type="{}"} | ||
$d_1 \ = \ ${ 724 3% } $\ \rm m$ | $d_1 \ = \ ${ 724 3% } $\ \rm m$ | ||
| Line 82: | Line 82: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The Doppler frequency is positive for $\tau_0$. This means that the receiver is moving towards the transmitter ⇒ <u>solution 2</u> is correct. |
| − | '''(2)''' | + | '''(2)''' The equation for the Doppler frequency is |
:$$f_{\rm D}= \frac{v}{c} \cdot f_{\rm S} \cdot \cos(\alpha) | :$$f_{\rm D}= \frac{v}{c} \cdot f_{\rm S} \cdot \cos(\alpha) | ||
| − | \hspace{0.05cm} | + | \hspace{0.05cm}.$$ |
| + | *If the angle of incidence is $\alpha=0$, then the Doppler frequency is | ||
| + | :$$f_{\rm D}=\frac{v}{c}\cdot f_{\rm S}.$$ | ||
| − | * | + | *In this case the speed of the receiver is |
:$$v = \frac{f_{\rm D}}{f_{\rm S}} \cdot c = \frac{10^2\,{\rm Hz}}{2 \cdot 10^9\,{\rm Hz}} \cdot 3 \cdot 10^8\,{\rm m/s} = 15\,{\rm m/s} | :$$v = \frac{f_{\rm D}}{f_{\rm S}} \cdot c = \frac{10^2\,{\rm Hz}}{2 \cdot 10^9\,{\rm Hz}} \cdot 3 \cdot 10^8\,{\rm m/s} = 15\,{\rm m/s} | ||
\hspace{0.1cm} \underline {= 54 \,{\rm km/h}} | \hspace{0.1cm} \underline {= 54 \,{\rm km/h}} | ||
| Line 97: | Line 99: | ||
| − | '''(3)''' | + | '''(3)''' <u>Solutions 1 and 4</u> are correct: |
| − | * | + | *The Doppler frequency $f_{\rm D} = 50 \ \rm Hz$ comes from the blue path, because the receiver moves towards the virtual transmitter ${\rm S}_2$ (i.e., towards the reflection point), although not directly. |
| − | * | + | *In other words: The movement of the receiver <b>reduces</b> the blue path's length. |
| + | *The angle $\alpha_2$ between the direction of movement and the connecting line ${\rm S_2 – E}$ is $60^\circ$: | ||
:$$\cos(\alpha_2) = \frac{f_{\rm D}}{f_{\rm S}} \cdot \frac{c}{v} = \frac{50 \,{\rm Hz}\cdot 3 \cdot 10^8\,{\rm m/s}}{2 \cdot 10^9\,{\rm Hz}\cdot 15\,{\rm m/s}} = 0.5 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \alpha_2 | :$$\cos(\alpha_2) = \frac{f_{\rm D}}{f_{\rm S}} \cdot \frac{c}{v} = \frac{50 \,{\rm Hz}\cdot 3 \cdot 10^8\,{\rm m/s}}{2 \cdot 10^9\,{\rm Hz}\cdot 15\,{\rm m/s}} = 0.5 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \alpha_2 | ||
\hspace{0.1cm} \underline {= 60^{\circ} } | \hspace{0.1cm} \underline {= 60^{\circ} } | ||
| Line 105: | Line 108: | ||
| − | '''(4)''' | + | '''(4)''' <u>Statements 1 and 3</u> are correct: From $f_{\rm D} = \, –50 \ \rm Hz$ follows $\alpha_3 = \alpha_2 ± \pi$ ⇒ $\alpha_3 \ \underline {= 240^\circ}$. |
| − | |||
| − | '''(5)''' <u> | + | '''(5)''' <u>All statements are correct</u>: |
| − | * | + | *The two Dirac functions at $± 50 \ \ \rm Hz$ have the same delay. We have $\tau_3 = \tau_2 = \tau_1 + \tau_0$. |
| − | * | + | *From the equality of the delays, however, it follows also $d_3 = d_2$. |
| + | *As both paths have the same length, their damping factors are equal, too. | ||
| − | '''(6)''' | + | '''(6)''' The delay difference is $\tau_0 = 1 \ \rm µ s$, as shown in the equation for $s(\tau_0, f_{\rm D})$. This gives the difference in length: |
| − | + | :$$\Delta d = \tau_0 \cdot c = 10^{–6} {\rm s} \cdot 3 \cdot 10^8 \ \rm m/s \ \ \underline {= 300 \ \ \rm m}.$$ | |
| − | :$$\Delta d = \tau_0 \cdot c = 10^{–6} {\rm s} \cdot 3 \cdot 10^8 \ \rm m/s \ \underline {= 300 \ \rm m}.$$ | ||
| + | '''(7)''' The path loss exponent was assumed to be $\gamma = 2$ for this task. | ||
| + | *Then $k_1 = K/d_1$ and $k_2 = K/d_2$. The constant $K$ is only an auxiliary variable that does not need to be considered further. | ||
| − | + | *The minus sign takes into account the $180^\circ$ phase rotation on the secondary paths. | |
| − | * | + | *From the weights of the Dirac functions one can read $k_1 = \sqrt{0.5}$ and $k_2 = -0.5$. Therefore: |
| − | |||
| − | |||
:$$\frac{d_2}{d_1} = \frac{k_1}{-k_2} = \frac{1/\sqrt{2}}{0.5} = \sqrt{2} | :$$\frac{d_2}{d_1} = \frac{k_1}{-k_2} = \frac{1/\sqrt{2}}{0.5} = \sqrt{2} | ||
\hspace{0.15cm} \underline {= 1.414} | \hspace{0.15cm} \underline {= 1.414} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | |||
| − | |||
| − | |||
| − | '''(8)''' | + | '''(8)''' From $d_2/d_1 = 2^{-0.5}$ and $\Delta d = d_2 \, - d_1 = 300 \ \rm m$ finally follows: |
:$$\sqrt{2} \cdot d_1 - d_1 = 300\,{\rm m} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | :$$\sqrt{2} \cdot d_1 - d_1 = 300\,{\rm m} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ||
d_1 = \frac{300\,{\rm m}}{\sqrt{2} - 1} \hspace{0.15cm} \underline {= 724\,{\rm m}} | d_1 = \frac{300\,{\rm m}}{\sqrt{2} - 1} \hspace{0.15cm} \underline {= 724\,{\rm m}} | ||
| Line 143: | Line 142: | ||
| − | + | [[Category:Mobile Communications: Exercises|^2.3 The GWSSUS Channel Model^]] | |
| − | [[Category: | ||
Latest revision as of 14:38, 23 March 2021
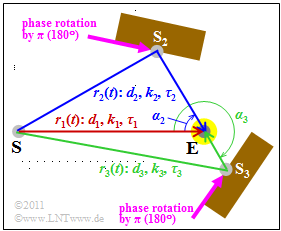
In Exercise 2.5, a delay–Doppler function (or scatter function) was given. From this, you should calculate and interpret the other system functions. The given scatter function $s(\tau_0, f_{\rm D})$ was
- $$s(\tau_0, f_{\rm D}) =\frac{1}{\sqrt{2}} \cdot \delta (\tau_0) \cdot \delta (f_{\rm D} - 100\,{\rm Hz}) \ - \ $$
- $$\hspace{1.5cm} \ - \ \hspace{-0.2cm} \frac{1}{2} \cdot \delta (\tau_0 \hspace{-0.05cm}- \hspace{-0.05cm}1\,{\rm \mu s}) \cdot \delta (f_{\rm D} \hspace{-0.05cm}- \hspace{-0.05cm}50\,{\rm Hz}) \ - \frac{1}{2} \cdot \delta (\tau_0 \hspace{-0.05cm}- \hspace{-0.05cm}1\,{\rm \mu s}) \cdot \delta (f_{\rm D}\hspace{-0.05cm} + \hspace{-0.05cm}50\,{\rm Hz}) \hspace{0.05cm}.$$
Note: In our learning tutorial, $s(\tau_0, \hspace{0.05cm} f_{\rm D})$ is also identified with $\eta_{\rm VD}(\tau_0, \hspace{0.05cm}f_{\rm D})$.
Here we have replaced the delay variable $\tau$ with $\tau_0$ . The new variable $\tau_0$ describes the difference between the delay of a path and the delay $\tau_1$ of the main path. The main path is thus identified in the above equation by $\tau_0 = 0$.
Now, we try to find a mobile radio scenario in which this scatter function would actually occur. The basic structure is sketched above as a top view, and the following hold:
- A single frequency is transmitted: $f_{\rm S} = 2 \ \rm GHz$.
- The mobile receiver $\rm (E)$ is represented here by a yellow dot. It is not known whether the vehicle is stationary, moving towards the transmitter $\rm (S)$ or moving away.
- The signal reaches the receiver via a main path (red) and two secondary paths (blue and green). Reflections from the obstacles cause phase shifts of $\pi$.
- ${\rm S}_2$ and ${\rm S}_3$ are to be understood here as fictitious transmitters from whose position the angles of incidence $\alpha_2$ and $\alpha_3$ of the secondary paths can be determined.
- Let the signal frequency be $f_{\rm S}$, the angle of incidence $\alpha$, the velocity $v$ and the velocity of light $c = 3 \cdot 10^8 \ \rm m/s$. Then, the Doppler frequency is
- $$f_{\rm D}= {v}/{c} \cdot f_{\rm S} \cdot \cos(\alpha) \hspace{0.05cm}.$$
- The damping factors $k_1$, $k_2$ and $k_3$ are inversely proportional to the path lengths $d_1$, $d_2$ and $d_3$. This corresponds to the path loss exponent $\gamma = 2$.
- This means: The signal power decreases quadratically with distance $d$ and accordingly the signal amplitude decreases linearly with $d$.
Notes:
- This task belongs to chapter The GWSSUS Channel Model.
- We also refer to the pages Common path-loss model and Doppler frequency and its distribution.
Questionnaire
Solution
(2) The equation for the Doppler frequency is
- $$f_{\rm D}= \frac{v}{c} \cdot f_{\rm S} \cdot \cos(\alpha) \hspace{0.05cm}.$$
- If the angle of incidence is $\alpha=0$, then the Doppler frequency is
- $$f_{\rm D}=\frac{v}{c}\cdot f_{\rm S}.$$
- In this case the speed of the receiver is
- $$v = \frac{f_{\rm D}}{f_{\rm S}} \cdot c = \frac{10^2\,{\rm Hz}}{2 \cdot 10^9\,{\rm Hz}} \cdot 3 \cdot 10^8\,{\rm m/s} = 15\,{\rm m/s} \hspace{0.1cm} \underline {= 54 \,{\rm km/h}} \hspace{0.05cm}.$$
(3) Solutions 1 and 4 are correct:
- The Doppler frequency $f_{\rm D} = 50 \ \rm Hz$ comes from the blue path, because the receiver moves towards the virtual transmitter ${\rm S}_2$ (i.e., towards the reflection point), although not directly.
- In other words: The movement of the receiver reduces the blue path's length.
- The angle $\alpha_2$ between the direction of movement and the connecting line ${\rm S_2 – E}$ is $60^\circ$:
- $$\cos(\alpha_2) = \frac{f_{\rm D}}{f_{\rm S}} \cdot \frac{c}{v} = \frac{50 \,{\rm Hz}\cdot 3 \cdot 10^8\,{\rm m/s}}{2 \cdot 10^9\,{\rm Hz}\cdot 15\,{\rm m/s}} = 0.5 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \alpha_2 \hspace{0.1cm} \underline {= 60^{\circ} } \hspace{0.05cm}.$$
(4) Statements 1 and 3 are correct: From $f_{\rm D} = \, –50 \ \rm Hz$ follows $\alpha_3 = \alpha_2 ± \pi$ ⇒ $\alpha_3 \ \underline {= 240^\circ}$.
(5) All statements are correct:
- The two Dirac functions at $± 50 \ \ \rm Hz$ have the same delay. We have $\tau_3 = \tau_2 = \tau_1 + \tau_0$.
- From the equality of the delays, however, it follows also $d_3 = d_2$.
- As both paths have the same length, their damping factors are equal, too.
(6) The delay difference is $\tau_0 = 1 \ \rm µ s$, as shown in the equation for $s(\tau_0, f_{\rm D})$. This gives the difference in length:
- $$\Delta d = \tau_0 \cdot c = 10^{–6} {\rm s} \cdot 3 \cdot 10^8 \ \rm m/s \ \ \underline {= 300 \ \ \rm m}.$$
(7) The path loss exponent was assumed to be $\gamma = 2$ for this task.
- Then $k_1 = K/d_1$ and $k_2 = K/d_2$. The constant $K$ is only an auxiliary variable that does not need to be considered further.
- The minus sign takes into account the $180^\circ$ phase rotation on the secondary paths.
- From the weights of the Dirac functions one can read $k_1 = \sqrt{0.5}$ and $k_2 = -0.5$. Therefore:
- $$\frac{d_2}{d_1} = \frac{k_1}{-k_2} = \frac{1/\sqrt{2}}{0.5} = \sqrt{2} \hspace{0.15cm} \underline {= 1.414} \hspace{0.05cm}.$$
(8) From $d_2/d_1 = 2^{-0.5}$ and $\Delta d = d_2 \, - d_1 = 300 \ \rm m$ finally follows:
- $$\sqrt{2} \cdot d_1 - d_1 = 300\,{\rm m} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} d_1 = \frac{300\,{\rm m}}{\sqrt{2} - 1} \hspace{0.15cm} \underline {= 724\,{\rm m}} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} d_2 = \sqrt{2} \cdot d_1 \hspace{0.15cm} \underline {= 1024\,{\rm m}} \hspace{0.05cm}. $$