Difference between revisions of "Aufgaben:Exercise 2.6: About the Huffman Coding"
| Line 110: | Line 110: | ||
===Solution=== | ===Solution=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Before the Huffman algorithm, the symbols must be sorted according to their probabilities of occurrence. |
| − | * | + | *Since the two least likely symbols are already combined in the first step, the probabilities of occurrence decrease from top to bottom <br>(from left to right in the diagram for this sample solution). |
| − | * | + | *By comparison with the data sheet, one obtains: |
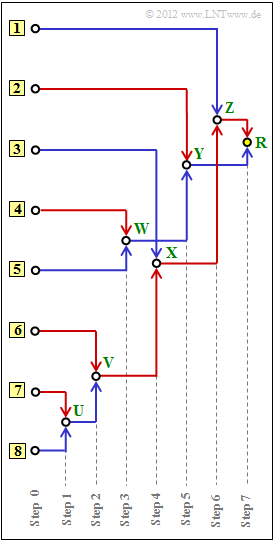
| − | :Symbol $\rm A$: <u>Eingang 7</u>, Symbol $\rm B$: <u>Eingang 6</u>, Symbol $\rm C$: <u>Eingang 3</u>, Symbol $\rm D$: <u> | + | :Symbol $\rm A$: <u>Eingang 7</u>, Symbol $\rm B$: <u>Eingang 6</u>, Symbol $\rm C$: <u>Eingang 3</u>, Symbol $\rm D$: <u>Input 1</u>. |
| − | [[File:EN_Inf_Z_2_6b.png|right|right|frame| | + | [[File:EN_Inf_Z_2_6b.png|right|right|frame|Tree diagram adapted to the exercise]] |
P_ID2452__Inf_A_2_6a.png | P_ID2452__Inf_A_2_6a.png | ||
| − | '''(2)''' | + | '''(2)''' The node $\rm R$ is the tree (<i>root</i>). This is always assigned $\underline{R=1}$ , regardless of the occurrence probabilities. |
| − | + | The following applies to the other values: | |
| − | : | + | :Step 1: $p_{\rm U} = p_{\rm A} + p_{\rm H} = 0.04 + 0.03 \hspace{0.15cm}\underline{ =0.07}$, |
| − | : | + | :Step 2: $p_{\rm V} = p_{\rm U} + p_{\rm B} = 0.07 + 0.08 \hspace{0.15cm}\underline{ =0.15}$, |
| − | : | + | :Step 3: $p_{\rm W} = p_{\rm F} + p_{\rm G} = 0.12 + 0.10 \hspace{0.15cm}\underline{ =0.22}$, |
| − | : | + | :Step 4: $p_{\rm X} = p_{\rm V} + p_{\rm C} = 0.15 + 0.14 \hspace{0.15cm}\underline{ =0.29}$, |
| − | : | + | :Step 5: $p_{\rm Y} = p_{\rm W} + p_{\rm E} = 0.22 + 0.24 \hspace{0.15cm}\underline{ =0.46}$, |
:Schritt 6: $p_{\rm Z} = p_{\rm X} + p_{\rm D} = 0.29 + 0.25 \hspace{0.15cm}\underline{ =0.54}$. | :Schritt 6: $p_{\rm Z} = p_{\rm X} + p_{\rm D} = 0.29 + 0.25 \hspace{0.15cm}\underline{ =0.54}$. | ||
<br clear=all> | <br clear=all> | ||
| − | '''(3)''' | + | '''(3)''' The Huffman code for symbol $\rm A$ is obtained by tracing the path from the $\rm root$ (yellow dot) to symbol $\rm A$ and assigning a <b>1</b> to each red connecting line and a <b>0</b> to each blue one.. |
| − | * Symbol $\rm A$: | + | * Symbol $\rm A$: red–red–red–blue–red→ <b><u>11101</u></b>, |
| − | * Symbol $\rm B$: | + | * Symbol $\rm B$: red–red–red–red → <b><u>1111</u></b>, |
| − | * Symbol $\rm C$: | + | * Symbol $\rm C$: red–red–blue → <b><u>110</u></b>, |
| − | * Symbol $\rm D$: | + | * Symbol $\rm D$: red–blue– → <b><u>10</u></b>, |
| − | * Symbol $\rm E$: | + | * Symbol $\rm E$: blue–red → <b>01</b>, |
| − | * Symbol $\rm F$: | + | * Symbol $\rm F$: blue–blue–red → <b>001</b>, |
| − | * Symbol $\rm G$: | + | * Symbol $\rm G$: blue–blue–blue → <b>000</b>, |
| − | * Symbol $\rm H$: | + | * Symbol $\rm H$: red–red–red–blue–blue → <b>11100</b>. |
| − | '''(4)''' | + | '''(4)''' The coding under point '''(3)''' has shown that |
| − | * | + | * the symbols $\rm D$ and $\rm E$ with two bits, |
| − | * | + | * the symbols $\rm C$, $\rm F$ and $\rm G$ with three bits, |
| − | * | + | * the symbols $\rm B$ with four bits, and |
| − | * | + | * the symbols $\rm A$ and $\rm H$ are each represented with five bits |
| − | + | are represented. Thus, for the mean codeword length (in "bit/source symbol&dquo;): | |
:$$L_{\rm M} \hspace{0.2cm} = \hspace{0.2cm} (p_{\rm D} + p_{\rm E}) \cdot 2 + (p_{\rm C} + p_{\rm F} + p_{\rm G}) \cdot 3 + p_{\rm B} \cdot 4 +(p_{\rm A} + p_{\rm H}) \cdot 5 = 0.49 \cdot 2 + 0.36 \cdot 3 +0.08 \cdot 4 +0.07 \cdot 5 | :$$L_{\rm M} \hspace{0.2cm} = \hspace{0.2cm} (p_{\rm D} + p_{\rm E}) \cdot 2 + (p_{\rm C} + p_{\rm F} + p_{\rm G}) \cdot 3 + p_{\rm B} \cdot 4 +(p_{\rm A} + p_{\rm H}) \cdot 5 = 0.49 \cdot 2 + 0.36 \cdot 3 +0.08 \cdot 4 +0.07 \cdot 5 | ||
\hspace{0.15cm}\underline{= 2.73}\hspace{0.05cm}.$$ | \hspace{0.15cm}\underline{= 2.73}\hspace{0.05cm}.$$ | ||
| Line 173: | Line 173: | ||
| − | '''(5)''' | + | '''(5)''' Only <u>answer 1</u> is correct: |
| − | * | + | *The mean codeword length $L_{\rm M}$ cannot be smaller than the source entropy $H$. This eliminates answers 2 and 3. |
| − | * | + | *From the given probabilities of occurrence, the source entropy can actually be calculated to $H = 2.71$ bit/source symbol. |
| − | * | + | *It can be seen that this Huffman coding almost reaches the given limitnbsp; (source coding theorem) $L_{\rm M, \ min } \ge H = 2.71$ bit/source symbol. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 22:39, 2 August 2021
We consider here a source symbol sequence $\langle q_\nu \rangle$ with symbol range $M = 8$:
- $$q_{\nu} = \{ \hspace{0.05cm}q_{\mu} \} = \{ \boldsymbol{\rm A} \hspace{0.05cm},\hspace{0.05cm} \boldsymbol{\rm B}\hspace{0.05cm},\hspace{0.05cm} \boldsymbol{\rm C}\hspace{0.05cm},\hspace{0.05cm} \boldsymbol{\rm D}\hspace{0.05cm},\hspace{0.05cm} \boldsymbol{\rm E}\hspace{0.05cm},\hspace{0.05cm} \boldsymbol{\rm F}\hspace{0.05cm},\hspace{0.05cm} \boldsymbol{\rm G}\hspace{0.05cm},\hspace{0.05cm} \boldsymbol{\rm H}\hspace{0.05cm} \}\hspace{0.05cm}.$$
If the symbols are equally probable, i.e. $p_{\rm A} = p_{\rm B} =$ ... $ = p_{\rm H} = 1/M$ then source coding makes no sense. Already with the dual code $\rm A$ → 000, $\rm B$ → 001, ... , $\rm H$ → 111, the mean codeword length $L_{\rm M}$ is its lower bound $H$ according to the source coding theorem $(H$ denotes the source entropy$ here)$:
- $$L_{\rm M,\hspace{0.08cm}min} = H = 3 \hspace{0.15cm}{\rm bit/source symbol} \hspace{0.05cm}.$$
However, let the symbol probabilities in this task be given as follows:
- $$p_{\rm A} \hspace{-0.05cm}= \hspace{-0.05cm} 0.04 \hspace{0.05cm},\hspace{0.1cm}p_{\rm B} \hspace{-0.05cm}= \hspace{-0.05cm} 0.08 \hspace{0.05cm},\hspace{0.1cm}p_{\rm C} \hspace{-0.05cm}= \hspace{-0.05cm} 0.14 \hspace{0.05cm},\hspace{0.1cm} p_{\rm D} \hspace{-0.05cm}= \hspace{-0.05cm} 0.25 \hspace{0.05cm},\hspace{0.1cm} p_{\rm E} \hspace{-0.05cm}= \hspace{-0.05cm} 0.24 \hspace{0.05cm},\hspace{0.1cm}p_{\rm F} \hspace{-0.05cm}= \hspace{-0.05cm} 0.12 \hspace{0.05cm},\hspace{0.1cm}p_{\rm G} \hspace{-0.05cm}= \hspace{-0.05cm} 0.10 \hspace{0.05cm},\hspace{0.1cm} p_{\rm H} \hspace{-0.05cm}= \hspace{-0.05cm} 0.03 \hspace{0.05cm}.$$
- So here we have a redundant message source that can be compressed by Huffman coding.
- This algorithm was published by David Albert Huffman – shortly after Shannon's groundbreaking work on information theory – and allows the construction of optimal prefix-free codes.
The algorithm is given here without derivation and proof, whereby we restrict ourselves to binary codes ⇒ the code symbol sequence consists only of zeros and ones:
- (1) Order the symbols according to decreasing probabilities of occurrence.
- (2) Combine the two least likely symbols into a new symbol.
- (3) Repeat steps (1) and (2), until only two (combined) symbols remain.
- (4) Binary code the more probable set of symbols with 1 , the other set with 0.
- (5) Step by step (from bottom to top) add 1 or 0 to the split partial codes.
This algorithm is often illustrated by a tree diagram. The diagram above shows this for the case at hand.
You have the following tasks:
- (a) Assign the symbols $\rm A$, ... , $\rm H$ to the inputs labelled [1], ... , [8] .
- (b) Determination of the sum probabilities $U$, ... , $Z$ and $R$ (root).
- (c) Assignment of the symbols $\rm A$, ... , $\rm H$ to the corresponding Huffman binary sequences. A red connection corresponds to a 1, a blue connection to a 0.
You will notice that the mean codeword length is
- $$L_{\rm M} = \sum_{\mu = 1}^{M}\hspace{0.05cm} p_{\mu} \cdot L_{\mu} $$
for Huffman coding is only slightly larger than the source entropy $H$. IIn this equation, the following values apply to the present case:
- $M = 8$, $p_1 = p_{\rm A}$, ... , $p_8 = p_{\rm H}$.
- The respective number of bits of the code symbols for $\rm A$, ... , $\rm H$ is denoted by $L_1$, ... , $L_8$ .
Hints:
- The exercise belongs to the chapter Entropy Coding according to Huffman.
- In particular, reference is made to the pages
- To check your results, please refer to the interaction module Shannon–Fano– and Huffman–coding.
Questions
Solution
- Since the two least likely symbols are already combined in the first step, the probabilities of occurrence decrease from top to bottom
(from left to right in the diagram for this sample solution). - By comparison with the data sheet, one obtains:
- Symbol $\rm A$: Eingang 7, Symbol $\rm B$: Eingang 6, Symbol $\rm C$: Eingang 3, Symbol $\rm D$: Input 1.
P_ID2452__Inf_A_2_6a.png
(2) The node $\rm R$ is the tree (root). This is always assigned $\underline{R=1}$ , regardless of the occurrence probabilities.
The following applies to the other values:
- Step 1: $p_{\rm U} = p_{\rm A} + p_{\rm H} = 0.04 + 0.03 \hspace{0.15cm}\underline{ =0.07}$,
- Step 2: $p_{\rm V} = p_{\rm U} + p_{\rm B} = 0.07 + 0.08 \hspace{0.15cm}\underline{ =0.15}$,
- Step 3: $p_{\rm W} = p_{\rm F} + p_{\rm G} = 0.12 + 0.10 \hspace{0.15cm}\underline{ =0.22}$,
- Step 4: $p_{\rm X} = p_{\rm V} + p_{\rm C} = 0.15 + 0.14 \hspace{0.15cm}\underline{ =0.29}$,
- Step 5: $p_{\rm Y} = p_{\rm W} + p_{\rm E} = 0.22 + 0.24 \hspace{0.15cm}\underline{ =0.46}$,
- Schritt 6: $p_{\rm Z} = p_{\rm X} + p_{\rm D} = 0.29 + 0.25 \hspace{0.15cm}\underline{ =0.54}$.
(3) The Huffman code for symbol $\rm A$ is obtained by tracing the path from the $\rm root$ (yellow dot) to symbol $\rm A$ and assigning a 1 to each red connecting line and a 0 to each blue one..
- Symbol $\rm A$: red–red–red–blue–red→ 11101,
- Symbol $\rm B$: red–red–red–red → 1111,
- Symbol $\rm C$: red–red–blue → 110,
- Symbol $\rm D$: red–blue– → 10,
- Symbol $\rm E$: blue–red → 01,
- Symbol $\rm F$: blue–blue–red → 001,
- Symbol $\rm G$: blue–blue–blue → 000,
- Symbol $\rm H$: red–red–red–blue–blue → 11100.
(4) The coding under point (3) has shown that
- the symbols $\rm D$ and $\rm E$ with two bits,
- the symbols $\rm C$, $\rm F$ and $\rm G$ with three bits,
- the symbols $\rm B$ with four bits, and
- the symbols $\rm A$ and $\rm H$ are each represented with five bits
are represented. Thus, for the mean codeword length (in "bit/source symbol&dquo;):
- $$L_{\rm M} \hspace{0.2cm} = \hspace{0.2cm} (p_{\rm D} + p_{\rm E}) \cdot 2 + (p_{\rm C} + p_{\rm F} + p_{\rm G}) \cdot 3 + p_{\rm B} \cdot 4 +(p_{\rm A} + p_{\rm H}) \cdot 5 = 0.49 \cdot 2 + 0.36 \cdot 3 +0.08 \cdot 4 +0.07 \cdot 5 \hspace{0.15cm}\underline{= 2.73}\hspace{0.05cm}.$$
(5) Only answer 1 is correct:
- The mean codeword length $L_{\rm M}$ cannot be smaller than the source entropy $H$. This eliminates answers 2 and 3.
- From the given probabilities of occurrence, the source entropy can actually be calculated to $H = 2.71$ bit/source symbol.
- It can be seen that this Huffman coding almost reaches the given limitnbsp; (source coding theorem) $L_{\rm M, \ min } \ge H = 2.71$ bit/source symbol.