Difference between revisions of "Aufgaben:Exercise 2.6: GF(P power m). Which P, which m?"
m (Text replacement - "”" to """) |
|||
| (9 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Extension_Field}} |
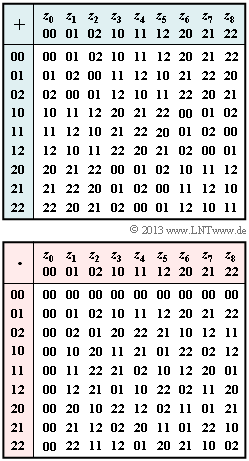
| − | [[File:P_ID2510__KC_A_2_6_neu.png|right|frame| | + | [[File:P_ID2510__KC_A_2_6_neu.png|right|frame|Underlying tables for <br>addition and multiplication]] |
| − | + | A Galois field ${\rm GF}(q)$ with $q = P^m$ elements defined by the adjacent tables is to be analyzed | |
| − | * | + | *for addition $($marked with "$+$"$)$, and |
| − | * | + | |
| + | *for multiplication $($marked with "$\hspace{0.05cm}\cdot\hspace{0.05cm}$)". | ||
| − | + | This Galois field ${\rm GF}(q) = \{\hspace{0.1cm}z_0,\hspace{0.1cm} z_1,\hspace{0.05cm} \text{...} , \hspace{0.1cm}z_{q-1}\}$ satisfies all the requirements for a finite field listed in the chapter [[Channel_Coding/Some_Basics_of_Algebra|"Some Basics of Algebra"]] . Thus, commutative, associative and distributive laws are also satisfied. | |
| − | + | Furthermore there is | |
| − | * | + | * a neutral element with respect to addition ⇒ $N_{\rm A}$: |
:$$\exists \hspace{0.15cm} z_j \in {\rm GF}(q)\text{:} | :$$\exists \hspace{0.15cm} z_j \in {\rm GF}(q)\text{:} | ||
| − | \hspace{0.25cm}z_i + z_j = z_i \hspace{0.3cm} \Rightarrow \hspace{0.3cm} z_j = N_{\rm A} \hspace{0.25cm}{\rm ( | + | \hspace{0.25cm}z_i + z_j = z_i \hspace{0.3cm} \Rightarrow \hspace{0.3cm} z_j = N_{\rm A} \hspace{0.25cm}{\rm (zero\hspace{0.15cm}element)} |
\hspace{0.05cm},$$ | \hspace{0.05cm},$$ | ||
| − | * | + | * a neutral element with respect to multiplication ⇒ $N_{\rm M}$: |
:$$\exists \hspace{0.15cm} z_j \in {\rm GF}(q)\text{:} | :$$\exists \hspace{0.15cm} z_j \in {\rm GF}(q)\text{:} | ||
| − | \hspace{0.25cm}z_i \cdot z_j = z_i \hspace{0.3cm} \Rightarrow \hspace{0.3cm} z_j = N_{\rm M} \hspace{0.25cm}{\rm ( | + | \hspace{0.25cm}z_i \cdot z_j = z_i \hspace{0.3cm} \Rightarrow \hspace{0.3cm} z_j = N_{\rm M} \hspace{0.25cm}{\rm (identity\hspace{0.15cm}element)} |
\hspace{0.05cm},$$ | \hspace{0.05cm},$$ | ||
| − | * | + | * for all elements $z_i$ an additive inverse ⇒ ${\rm Inv_A}(z_i)$: |
:$$\forall \hspace{0.15cm} z_i \in {\rm GF}(q)\hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_A}(z_i) \in {\rm GF}(q)\text{:}$$ | :$$\forall \hspace{0.15cm} z_i \in {\rm GF}(q)\hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_A}(z_i) \in {\rm GF}(q)\text{:}$$ | ||
| − | ::$$z_i + {\rm Inv_A}(z_i) = N_{\rm A} = {\rm "0"}\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm | + | ::$$z_i + {\rm Inv_A}(z_i) = N_{\rm A} = {\rm "\hspace{-0.15cm}0\hspace{-0.1cm}"}\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm short}\text{:}\hspace{0.15cm} |
{\rm Inv_A}(z_i) = - z_i \hspace{0.05cm}, $$ | {\rm Inv_A}(z_i) = - z_i \hspace{0.05cm}, $$ | ||
| − | * | + | * for all elements $z_i$ except the zero element a multiplicative inverse ⇒ ${\rm Inv_M}(z_i)$: |
:$$\forall \hspace{0.15cm} z_i \in {\rm GF}(q),\hspace{0.15cm} z_i \ne N_{\rm A} \hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_M}(z_i) \in {\rm GF}(q)\text{:}$$ | :$$\forall \hspace{0.15cm} z_i \in {\rm GF}(q),\hspace{0.15cm} z_i \ne N_{\rm A} \hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_M}(z_i) \in {\rm GF}(q)\text{:}$$ | ||
| − | ::$$z_i \cdot {\rm Inv_M}(z_i) = N_{\rm M} = {\rm "1"} | + | ::$$z_i \cdot {\rm Inv_M}(z_i) = N_{\rm M} = {\rm "\hspace{-0.15cm}1\hspace{-0.1cm}"} |
| − | \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm | + | \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm short}\text{:}\hspace{0.15cm} |
{\rm Inv_M}(z_i) = z_i^{-1} | {\rm Inv_M}(z_i) = z_i^{-1} | ||
\hspace{0.05cm}. $$ | \hspace{0.05cm}. $$ | ||
| Line 31: | Line 32: | ||
| + | Hints: | ||
| + | * This exercise belongs to the chapter [[Channel_Coding/Extension_Field| "Extension Field"]]. | ||
| + | |||
| + | * In the tables, the elements $z_0, \hspace{0.05cm} \text{...} \hspace{0.1cm} , \ z_8$ are called "coefficient vectors". | ||
| − | + | * For example, "$2 \hspace{0.03cm}1$" stands for "$2 \cdot \alpha + 1$". | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | * | ||
| − | |||
| Line 44: | Line 43: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Specify the parameters of the Galois field considered here. |
|type="{}"} | |type="{}"} | ||
$P \ = \ ${ 3 } | $P \ = \ ${ 3 } | ||
| Line 52: | Line 51: | ||
$q \ = \ ${ 9 } | $q \ = \ ${ 9 } | ||
| − | { | + | {What is the neutral element of addition? |
|type="()"} | |type="()"} | ||
| − | + | + | + The neutral element of addition is $N_{\rm A} = \,$ "$0\hspace{0.03cm}0$", |
| − | - | + | - The neutral element of addition is $N_{\rm A} = \,$ "$0\hspace{0.03cm}1$". |
| − | { | + | {What is the neutral element of multiplication? |
|type="()"} | |type="()"} | ||
| − | - | + | - The neutral element of multiplication is $N_{\rm M} = \,$ "$0\hspace{0.03cm}0$", |
| − | + | + | + The neutral element of multiplication is $N_{\rm M} = \,$ "$0\hspace{0.03cm}1$". |
| − | { | + | {What statements are true regarding additive inverses? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + It holds ${\rm Inv_A} ($"$0\hspace{0.03cm}2$") $\, = \, $ "$0\hspace{0.03cm}1$", |
| − | + | + | + It holds ${\rm Inv_A} ($"$1\hspace{0.03cm}1$") $\, = \, $ "$2\hspace{0.03cm}2$", |
| − | - | + | - It holds ${\rm Inv_A} ($"$2\hspace{0.03cm}2$") $\, = \, $ "$0\hspace{0.03cm}0$". |
| − | { | + | {Which of the following statements are true about the multiplication? |
|type="()"} | |type="()"} | ||
| − | - | + | - The multiplication is defined here modulo $p(\alpha) = \alpha^2 + 2$. |
| − | + | + | + The multiplication is defined here modulo $p(\alpha) = \alpha^2 + 2\alpha + 2$. |
| − | { | + | {What statements are true regarding multiplicative inverses? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - There is a multiplicative inverse for all elements $z_i ∈ {\rm GF}(P^m)$ . |
| − | + | + | + It holds ${\rm Inv_M} ($"$1\hspace{0.03cm}2$") $\, = \, $"$1\hspace{0.03cm}0$". |
| − | - | + | - It holds ${\rm Inv_M} ($"$2\hspace{0.03cm}1$") $\, = \, $ "$1\hspace{0.03cm}2$". |
| − | { | + | {Does $($"$2\hspace{0.03cm}0$" $\, + \,$ "$1\hspace{0.03cm}2$"$)$ $\, \cdot\, $ "$1\hspace{0.03cm}2$" $\, = \, $"$2\hspace{0.03cm}0$" $\, \cdot\, $ "$1\hspace{0.03cm}2$" $\, + \, $"$1\hspace{0.03cm}2$" $\, \cdot\, $ "$1\hspace{0.03cm}2$" hold? |
|type="()"} | |type="()"} | ||
| − | + | + | + Yes. |
| − | - | + | - No. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Each element consists of two ternaries ⇒ $\underline{P = 3}, \ \underline{m = 2}$. There are $q = P^m = 3^8 = \underline{9 \rm \hspace{0.2cm}elements}$. |
| − | '''(2)''' | + | '''(2)''' Correct is the <u>proposed solution 1</u>: |
| − | * | + | *The neutral element of the addition $(N_{\rm A})$ satisfies for all $z_i ∈ {\rm GF}(P^m)$ the condition $z_i + N_{\rm A} = z_i$. |
| − | * | + | *From the addition table it can be read that "$0\hspace{0.03cm}0$" satisfies this condition. |
| − | '''(3)''' | + | '''(3)''' Correct is the <u>proposed solution 2</u>: |
| − | * | + | *The neutral element of the multiplication $(N_{\rm M})$ must always satisfy the condition $z_i \cdot N_{\rm M} = z_i$. |
| − | * | + | |
| − | * In | + | *From the multiplication table, $N_{\rm M} = \, "\hspace{-0.15cm}0\hspace{0.03cm}1\hspace{-0.1cm}"$. |
| + | |||
| + | *In polynomial notation, this corresponds to $k_1 = 0$ and $k_0 = 1$: | ||
:$$k_1 \cdot \alpha + k_0 = 1 \hspace{0.05cm}.$$ | :$$k_1 \cdot \alpha + k_0 = 1 \hspace{0.05cm}.$$ | ||
| − | '''(4)''' | + | '''(4)''' With the polynomial representation, the following calculations result: |
| − | :$${\rm Inv_A}("\hspace{-0.05cm}0\hspace{0.03cm}2") \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(2) = (-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = 1 \hspace{0.25cm}\Rightarrow \hspace{0.25cm}{\rm | + | :$${\rm Inv_A}("\hspace{-0.05cm}0\hspace{0.03cm}2") \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(2) = (-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = 1 \hspace{0.25cm}\Rightarrow \hspace{0.25cm}{\rm vector}\hspace{0.15cm}"\hspace{-0.1cm}0\hspace{0.03cm}1\hspace{-0.1cm}"\hspace{0.05cm},$$ |
:$${\rm Inv_A}("\hspace{-0.05cm}1\hspace{0.03cm}1")\hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(\alpha + 1) = \big[(-\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + | :$${\rm Inv_A}("\hspace{-0.05cm}1\hspace{0.03cm}1")\hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(\alpha + 1) = \big[(-\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + | ||
| − | \big[(-1) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] =2\alpha + 2 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm | + | \big[(-1) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] =2\alpha + 2 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}\hspace{-0.05cm}2\hspace{0.03cm}2\hspace{-0.1cm}"\hspace{0.05cm},$$ |
:$${\rm Inv_A}("\hspace{-0.05cm}2\hspace{0.03cm}2")\hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(2\alpha + 2) = \big[(-2\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + | :$${\rm Inv_A}("\hspace{-0.05cm}2\hspace{0.03cm}2")\hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(2\alpha + 2) = \big[(-2\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + | ||
| − | \big[(-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] =\alpha + 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm | + | \big[(-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] =\alpha + 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}\hspace{-0.05cm}1\hspace{0.03cm}1\hspace{-0.1cm}"\hspace{0.05cm}.$$ |
| − | + | Consequently, only the <u>first two proposed solutions</u> are correct. | |
| + | |||
| + | However, the exercise can also be solved without calculation using the addition table alone: | ||
| + | *For example, you can find the inverse of "$2\hspace{0.03cm}2$" by looking for the column with the entry "$0\hspace{0.03cm}0$" in the last row. | ||
| + | |||
| + | *You find the column labeled "$1\hspace{0.03cm}1$" and thus ${\rm Inv_A}("\hspace{-0.15cm}2\hspace{0.03cm}2\hspace{-0.15cm}") = \, "\hspace{-0.15cm}1\hspace{0.03cm}1\hspace{-0.15cm}"$. | ||
| − | |||
| − | |||
| − | |||
| + | '''(5)''' Multiplying $\alpha$ $($vector "$1\hspace{0.03cm}0$"$)$ by itself gives $\alpha^2$. | ||
| + | * If the first proposed solution were valid, the condition $\alpha^2 + 2 = 0$ and thus $\alpha^2 = (-2) \, {\rm mod} \, 3 = 1$, thus yielding the vector "$0\hspace{0.03cm}1$". | ||
| − | + | * Assuming the second proposed solution, it follows from the condition $\alpha^2 + 2\alpha + 2 = 0$ in polynomial notation: | |
| − | + | :$$\alpha^2 = \big [(-2\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + \big[(-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big ] = \alpha + 1 $$ | |
| − | + | :and thus the coefficient vector "$1\hspace{0.03cm}1$". | |
| − | :$$\alpha^2 = [(-2\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3] + [(-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3] = \alpha + 1 $$ | ||
| − | : | ||
| − | In | + | *In the multiplication table, in row 4, column 4, we find exactly the entry "$1\hspace{0.03cm}1$" ⇒ So, the correct answert is the <u>proposed solution 2</u>. |
| − | '''(6)''' | + | '''(6)''' The multiplicative inverse to "$1\hspace{0.03cm}2$" can be found in row 6 of the multiplication table as the column with the entry "$0\hspace{0.03cm}1$" |
| + | *So the <u>proposed solution 2</u> is correct in contrast to proposal 3. Namely, ${\rm Inv_M}("\hspace{-0.15cm}21\hspace{-0.15cm}") = \, "\hspace{-0.15cm}2\hspace{0.03cm}0\hspace{-0.1cm}"$ holds. | ||
| − | + | *We check these results considering $\alpha^2 + 2\alpha + 2 = 0$ by multiplications: | |
| − | :$$"1\hspace{0.03cm}2" \hspace{0.05cm}\cdot \hspace{0.05cm}"1\hspace{0.03cm}0" \hspace{0.15cm} \Rightarrow \hspace{0.15cm} (\alpha + 2) \cdot \alpha = \alpha^2 + 2\alpha = (-2\alpha-2) + 2\alpha = -2 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = 1 \hspace{0.15cm} \Rightarrow \hspace{0.15cm} {\rm | + | :$$"\hspace{-0.15cm}1\hspace{0.03cm}2\hspace{-0.1cm}" \hspace{0.05cm}\cdot \hspace{0.05cm}"\hspace{-0.15cm}1\hspace{0.03cm}0\hspace{-0.1cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm} (\alpha + 2) \cdot \alpha = \alpha^2 + 2\alpha = (-2\alpha-2) + 2\alpha = -2 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = 1 \hspace{0.15cm} \Rightarrow \hspace{0.15cm} {\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}0\hspace{0.03cm}1\hspace{-0.15cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm}{\rm multiplicative \hspace{0.15cm}inverse}\hspace{0.05cm}.$$ |
| − | :$$"2\hspace{0.03cm}1" \hspace{0.05cm}\cdot \hspace{0.05cm}" | + | :$$"\hspace{-0.15cm}2\hspace{0.03cm}1\hspace{-0.1cm}" \hspace{0.05cm}\cdot \hspace{0.05cm}"\hspace{-0.15cm}1\hspace{0.03cm}2\hspace{-0.1cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm} (2\alpha + 1) \cdot (\alpha + 2) = 2 \alpha^2 + \alpha + 4\alpha + 2 = 2 \alpha^2 + 5\alpha + 2 = 2 \cdot (-2\alpha - 2) + 5\alpha + 2 = (\alpha - 2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = \alpha +1 $$ |
| − | + | :$$\hspace{2.725cm} \Rightarrow \ {\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}1\hspace{0.03cm}1\hspace{-0.15cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm}{\rm no\hspace{0.15cm}multiplicative \hspace{0.15cm}inverse}\hspace{0.05cm}.$$ | |
| − | :$$\hspace{2.725cm} \Rightarrow \ {\rm | ||
| − | + | *The solution suggestion 1 is therefore not correct, because there is no multiplicative inverse for "$00$". | |
| − | '''(7)''' | + | '''(7)''' The two expressions agree ⇒ <u>YES</u>, as the following calculations show: |
| − | :$$("20" + "12") \ \cdot "12" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "02"\cdot "12" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "21"\hspace{0.05cm},$$ | + | :$$("\hspace{-0.15cm}20\hspace{-0.1cm}" + "\hspace{-0.15cm}12\hspace{-0.1cm}") \ \cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}02\hspace{-0.1cm}"\cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}21\hspace{-0.1cm}"\hspace{0.05cm},$$ |
| − | :$$"20" \cdot "12" + "12" \cdot "12" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "02" + "22" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "21"\hspace{0.05cm}.$$ | + | :$$"\hspace{-0.15cm}20\hspace{-0.1cm}" \cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" + "\hspace{-0.15cm}12\hspace{-0.1cm}" \cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}02\hspace{-0.1cm}" + "\hspace{-0.15cm}22\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}21\hspace{-0.1cm}"\hspace{0.05cm}.$$ |
| − | + | This means: The distributive law has been proved at least on a single example. | |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category:Channel Coding: Exercises|^2.2 | + | [[Category:Channel Coding: Exercises|^2.2 Extension Field^]] |
Latest revision as of 15:29, 4 October 2022
A Galois field ${\rm GF}(q)$ with $q = P^m$ elements defined by the adjacent tables is to be analyzed
- for addition $($marked with "$+$"$)$, and
- for multiplication $($marked with "$\hspace{0.05cm}\cdot\hspace{0.05cm}$)".
This Galois field ${\rm GF}(q) = \{\hspace{0.1cm}z_0,\hspace{0.1cm} z_1,\hspace{0.05cm} \text{...} , \hspace{0.1cm}z_{q-1}\}$ satisfies all the requirements for a finite field listed in the chapter "Some Basics of Algebra" . Thus, commutative, associative and distributive laws are also satisfied.
Furthermore there is
- a neutral element with respect to addition ⇒ $N_{\rm A}$:
- $$\exists \hspace{0.15cm} z_j \in {\rm GF}(q)\text{:} \hspace{0.25cm}z_i + z_j = z_i \hspace{0.3cm} \Rightarrow \hspace{0.3cm} z_j = N_{\rm A} \hspace{0.25cm}{\rm (zero\hspace{0.15cm}element)} \hspace{0.05cm},$$
- a neutral element with respect to multiplication ⇒ $N_{\rm M}$:
- $$\exists \hspace{0.15cm} z_j \in {\rm GF}(q)\text{:} \hspace{0.25cm}z_i \cdot z_j = z_i \hspace{0.3cm} \Rightarrow \hspace{0.3cm} z_j = N_{\rm M} \hspace{0.25cm}{\rm (identity\hspace{0.15cm}element)} \hspace{0.05cm},$$
- for all elements $z_i$ an additive inverse ⇒ ${\rm Inv_A}(z_i)$:
- $$\forall \hspace{0.15cm} z_i \in {\rm GF}(q)\hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_A}(z_i) \in {\rm GF}(q)\text{:}$$
- $$z_i + {\rm Inv_A}(z_i) = N_{\rm A} = {\rm "\hspace{-0.15cm}0\hspace{-0.1cm}"}\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm short}\text{:}\hspace{0.15cm} {\rm Inv_A}(z_i) = - z_i \hspace{0.05cm}, $$
- for all elements $z_i$ except the zero element a multiplicative inverse ⇒ ${\rm Inv_M}(z_i)$:
- $$\forall \hspace{0.15cm} z_i \in {\rm GF}(q),\hspace{0.15cm} z_i \ne N_{\rm A} \hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_M}(z_i) \in {\rm GF}(q)\text{:}$$
- $$z_i \cdot {\rm Inv_M}(z_i) = N_{\rm M} = {\rm "\hspace{-0.15cm}1\hspace{-0.1cm}"} \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm short}\text{:}\hspace{0.15cm} {\rm Inv_M}(z_i) = z_i^{-1} \hspace{0.05cm}. $$
Hints:
- This exercise belongs to the chapter "Extension Field".
- In the tables, the elements $z_0, \hspace{0.05cm} \text{...} \hspace{0.1cm} , \ z_8$ are called "coefficient vectors".
- For example, "$2 \hspace{0.03cm}1$" stands for "$2 \cdot \alpha + 1$".
Questions
Solution
(2) Correct is the proposed solution 1:
- The neutral element of the addition $(N_{\rm A})$ satisfies for all $z_i ∈ {\rm GF}(P^m)$ the condition $z_i + N_{\rm A} = z_i$.
- From the addition table it can be read that "$0\hspace{0.03cm}0$" satisfies this condition.
(3) Correct is the proposed solution 2:
- The neutral element of the multiplication $(N_{\rm M})$ must always satisfy the condition $z_i \cdot N_{\rm M} = z_i$.
- From the multiplication table, $N_{\rm M} = \, "\hspace{-0.15cm}0\hspace{0.03cm}1\hspace{-0.1cm}"$.
- In polynomial notation, this corresponds to $k_1 = 0$ and $k_0 = 1$:
- $$k_1 \cdot \alpha + k_0 = 1 \hspace{0.05cm}.$$
(4) With the polynomial representation, the following calculations result:
- $${\rm Inv_A}("\hspace{-0.05cm}0\hspace{0.03cm}2") \hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(2) = (-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = 1 \hspace{0.25cm}\Rightarrow \hspace{0.25cm}{\rm vector}\hspace{0.15cm}"\hspace{-0.1cm}0\hspace{0.03cm}1\hspace{-0.1cm}"\hspace{0.05cm},$$
- $${\rm Inv_A}("\hspace{-0.05cm}1\hspace{0.03cm}1")\hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(\alpha + 1) = \big[(-\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + \big[(-1) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] =2\alpha + 2 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}\hspace{-0.05cm}2\hspace{0.03cm}2\hspace{-0.1cm}"\hspace{0.05cm},$$
- $${\rm Inv_A}("\hspace{-0.05cm}2\hspace{0.03cm}2")\hspace{-0.15cm} \ = \ \hspace{-0.15cm} {\rm Inv_A}(2\alpha + 2) = \big[(-2\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + \big[(-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] =\alpha + 1 \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}\hspace{-0.05cm}1\hspace{0.03cm}1\hspace{-0.1cm}"\hspace{0.05cm}.$$
Consequently, only the first two proposed solutions are correct.
However, the exercise can also be solved without calculation using the addition table alone:
- For example, you can find the inverse of "$2\hspace{0.03cm}2$" by looking for the column with the entry "$0\hspace{0.03cm}0$" in the last row.
- You find the column labeled "$1\hspace{0.03cm}1$" and thus ${\rm Inv_A}("\hspace{-0.15cm}2\hspace{0.03cm}2\hspace{-0.15cm}") = \, "\hspace{-0.15cm}1\hspace{0.03cm}1\hspace{-0.15cm}"$.
(5) Multiplying $\alpha$ $($vector "$1\hspace{0.03cm}0$"$)$ by itself gives $\alpha^2$.
- If the first proposed solution were valid, the condition $\alpha^2 + 2 = 0$ and thus $\alpha^2 = (-2) \, {\rm mod} \, 3 = 1$, thus yielding the vector "$0\hspace{0.03cm}1$".
- Assuming the second proposed solution, it follows from the condition $\alpha^2 + 2\alpha + 2 = 0$ in polynomial notation:
- $$\alpha^2 = \big [(-2\alpha) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big] + \big[(-2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3\big ] = \alpha + 1 $$
- and thus the coefficient vector "$1\hspace{0.03cm}1$".
- In the multiplication table, in row 4, column 4, we find exactly the entry "$1\hspace{0.03cm}1$" ⇒ So, the correct answert is the proposed solution 2.
(6) The multiplicative inverse to "$1\hspace{0.03cm}2$" can be found in row 6 of the multiplication table as the column with the entry "$0\hspace{0.03cm}1$"
- So the proposed solution 2 is correct in contrast to proposal 3. Namely, ${\rm Inv_M}("\hspace{-0.15cm}21\hspace{-0.15cm}") = \, "\hspace{-0.15cm}2\hspace{0.03cm}0\hspace{-0.1cm}"$ holds.
- We check these results considering $\alpha^2 + 2\alpha + 2 = 0$ by multiplications:
- $$"\hspace{-0.15cm}1\hspace{0.03cm}2\hspace{-0.1cm}" \hspace{0.05cm}\cdot \hspace{0.05cm}"\hspace{-0.15cm}1\hspace{0.03cm}0\hspace{-0.1cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm} (\alpha + 2) \cdot \alpha = \alpha^2 + 2\alpha = (-2\alpha-2) + 2\alpha = -2 \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = 1 \hspace{0.15cm} \Rightarrow \hspace{0.15cm} {\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}0\hspace{0.03cm}1\hspace{-0.15cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm}{\rm multiplicative \hspace{0.15cm}inverse}\hspace{0.05cm}.$$
- $$"\hspace{-0.15cm}2\hspace{0.03cm}1\hspace{-0.1cm}" \hspace{0.05cm}\cdot \hspace{0.05cm}"\hspace{-0.15cm}1\hspace{0.03cm}2\hspace{-0.1cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm} (2\alpha + 1) \cdot (\alpha + 2) = 2 \alpha^2 + \alpha + 4\alpha + 2 = 2 \alpha^2 + 5\alpha + 2 = 2 \cdot (-2\alpha - 2) + 5\alpha + 2 = (\alpha - 2) \hspace{0.1cm}{\rm mod} \hspace{0.1cm} 3 = \alpha +1 $$
- $$\hspace{2.725cm} \Rightarrow \ {\rm vector}\hspace{0.15cm}"\hspace{-0.15cm}1\hspace{0.03cm}1\hspace{-0.15cm}" \hspace{0.15cm} \Rightarrow \hspace{0.15cm}{\rm no\hspace{0.15cm}multiplicative \hspace{0.15cm}inverse}\hspace{0.05cm}.$$
- The solution suggestion 1 is therefore not correct, because there is no multiplicative inverse for "$00$".
(7) The two expressions agree ⇒ YES, as the following calculations show:
- $$("\hspace{-0.15cm}20\hspace{-0.1cm}" + "\hspace{-0.15cm}12\hspace{-0.1cm}") \ \cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}02\hspace{-0.1cm}"\cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}21\hspace{-0.1cm}"\hspace{0.05cm},$$
- $$"\hspace{-0.15cm}20\hspace{-0.1cm}" \cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" + "\hspace{-0.15cm}12\hspace{-0.1cm}" \cdot "\hspace{-0.15cm}12\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}02\hspace{-0.1cm}" + "\hspace{-0.15cm}22\hspace{-0.1cm}" \hspace{-0.15cm} \ = \ \hspace{-0.15cm} "\hspace{-0.15cm}21\hspace{-0.1cm}"\hspace{0.05cm}.$$
This means: The distributive law has been proved at least on a single example.