Difference between revisions of "Aufgaben:Exercise 3.2: Eye Pattern according to Gaussian Low-Pass"
m (Text replacement - "Category:Aufgaben zu Digitalsignalübertragung" to "Category:Digital Signal Transmission: Exercises") |
|||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Error_Probability_with_Intersymbol_Interference |
}} | }} | ||
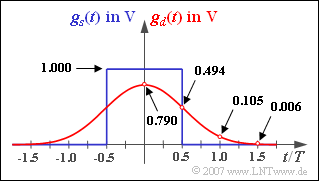
| − | [[File:P_ID1381__Dig_A_3_2.png|right|frame| | + | [[File:P_ID1381__Dig_A_3_2.png|right|frame|Transmission and basic transmitter pulse]] |
| − | + | Let a binary bipolar redundancy-free baseband system with bit rate $R_{\rm B} = 100\,{\rm Mbit/s}$ and the following properties be given: | |
| − | * | + | * Let the transmitted pulses be rectangular, and the possible amplitude values be $± 1\,{\rm V}$. |
| − | * | + | * The AWGN noise power density $($on the resistor $1 \, \Omega)$ is $10^{\rm -9} \, {\rm V}^2/{\rm Hz}$. |
| − | * | + | * A Gaussian low-pass filter with cutoff frequency $f_{\rm G} = 50 \, {\rm MHz}$ is used as the receiver filter. The frequency response is: |
:$$H_{\rm G}(f) = {\rm e}^{- \pi \hspace{0.05cm}\cdot \hspace{0.05cm}{f}^2/({2f_{\rm G}})^2} | :$$H_{\rm G}(f) = {\rm e}^{- \pi \hspace{0.05cm}\cdot \hspace{0.05cm}{f}^2/({2f_{\rm G}})^2} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | * The basic transmitter pulse $g_d(t) = g_s(t) * h_{\rm G}(t)$ is shown in the graph (red curve). Some prominent pulse values are indicated. |
| − | * | + | * The detection noise power can be calculated using the following equation: |
:$$\sigma_d^2 = {N_0}/{2} \cdot \int_{-\infty}^{+\infty} | :$$\sigma_d^2 = {N_0}/{2} \cdot \int_{-\infty}^{+\infty} | ||
|H_{\rm G}(f)|^2 \,{\rm d} f \hspace{0.05cm}.$$ | |H_{\rm G}(f)|^2 \,{\rm d} f \hspace{0.05cm}.$$ | ||
| − | + | For example, the eye diagram can be used to determine the error probability. | |
| − | * | + | * The mean symbol error probability $p_{\rm S}$ is obtained from this after averaging over all possible detection useful samples. |
| − | * | + | * The worst-case error probability serves as an upper bound for $p_{\rm S}$. |
:$$p_{\rm U} = {\rm Q} \left( \frac{\ddot{o}(T_{\rm D})/2}{ \sigma_d} | :$$p_{\rm U} = {\rm Q} \left( \frac{\ddot{o}(T_{\rm D})/2}{ \sigma_d} | ||
| − | \right) \hspace{0.3cm}{\rm | + | \right) \hspace{0.3cm}{\rm with}\hspace{0.3cm}\frac{\ddot{o}(T_{\rm D})}{ 2}= g_d(t=0) - |g_d(t=T)|- |g_d(t=-T)|-\hspace{0.01cm}\text{ ...}$$ |
| − | + | Here, $\ddot{o}(T_{\rm D})$ denotes the vertical eye opening. Let the detection time $T_{\rm D} = 0$ be optimally chosen. | |
| Line 27: | Line 27: | ||
| − | '' | + | ''Notes:'' |
| − | * | + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Error_Probability_with_Intersymbol_Interference|Error Probability with Intersymbol Interference]]. |
| − | * | + | * Use the interaction module [[Applets:Komplementäre_Gaußsche_Fehlerfunktionen|Complementary Gaussian Error Functions]] for the numerical evaluation of the Q-function. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the symbol duration? |
|type="{}"} | |type="{}"} | ||
$T \ = \ $ { 10 3% } $\ {\rm ns}$ | $T \ = \ $ { 10 3% } $\ {\rm ns}$ | ||
| − | { | + | {What is the rms value of the detection noise signal? |
|type="{}"} | |type="{}"} | ||
$\sigma_d\ = \ $ { 0.188 3% } $\ {\rm V}$ | $\sigma_d\ = \ $ { 0.188 3% } $\ {\rm V}$ | ||
| − | { | + | {What are the basic transmitter pulse values $g_{\rm \nu} = g_d(\nu \cdot T)$, in particular |
|type="{}"} | |type="{}"} | ||
$g_0\ = \ $ { 0.79 3% } $\ {\rm V}$ | $g_0\ = \ $ { 0.79 3% } $\ {\rm V}$ | ||
| Line 51: | Line 51: | ||
$g_2\ = \ $ { 0 3% } $\ {\rm V}$ | $g_2\ = \ $ { 0 3% } $\ {\rm V}$ | ||
| − | { | + | {Calculate the eye opening and the worst-case error probability. |
|type="{}"} | |type="{}"} | ||
$\ddot{o}(T_{\rm D})\ = \ $ { 1.16 3% } $\ {\rm V}$ | $\ddot{o}(T_{\rm D})\ = \ $ { 1.16 3% } $\ {\rm V}$ | ||
$p_{\rm U}\ = \ $ { 1 3% } $\ \cdot 10^{\rm -3}$ | $p_{\rm U}\ = \ $ { 1 3% } $\ \cdot 10^{\rm -3}$ | ||
| − | { | + | {Calculate the average error probability $p_{\rm S}$ by averaging over the possible useful samples. |
|type="{}"} | |type="{}"} | ||
$p_{\rm S}\ = \ $ { 0.256 3% } $\ \cdot 10^{\rm -3}$ | $p_{\rm S}\ = \ $ { 0.256 3% } $\ \cdot 10^{\rm -3}$ | ||
| − | { | + | {What would be the minimum increase in transmitted pulse amplitude $s_0$ required to satisfy the condition $p_{\rm S} \ ≤ 10^{\rm -10}$? |
|type="{}"} | |type="{}"} | ||
$s_0\ = \ ${ 1.993 3% } $\ {\rm V}$ | $s_0\ = \ ${ 1.993 3% } $\ {\rm V}$ | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The symbol duration is the reciprocal of the bit rate: |
:$$T = \frac{1}{10^8\,{\rm bit/s}} = 10^{-8}\,{\rm s}\hspace{0.15cm}\underline { = 10\,{\rm ns}} | :$$T = \frac{1}{10^8\,{\rm bit/s}} = 10^{-8}\,{\rm s}\hspace{0.15cm}\underline { = 10\,{\rm ns}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | '''(2)''' | + | '''(2)''' Integration according to the given equation leads to: |
:$$\sigma_d^2 = \frac{N_0}{2} \cdot \int_{-\infty}^{+\infty} | :$$\sigma_d^2 = \frac{N_0}{2} \cdot \int_{-\infty}^{+\infty} | ||
|H_{\rm G}(f)|^2 \,{\rm d} f = \frac{N_0 \cdot f_{\rm | |H_{\rm G}(f)|^2 \,{\rm d} f = \frac{N_0 \cdot f_{\rm | ||
| Line 81: | Line 81: | ||
| − | '''(3)''' | + | '''(3)''' These values can be obtained from the graph: |
:$$g_0 = g_d(0)\hspace{0.15cm}\underline { = 0.790\,{\rm V}}, \hspace{0.2cm}g_1 = g_d(10\,{\rm | :$$g_0 = g_d(0)\hspace{0.15cm}\underline { = 0.790\,{\rm V}}, \hspace{0.2cm}g_1 = g_d(10\,{\rm | ||
ns}) \hspace{0.15cm}\underline {= 0.105\,{\rm V}}= g_{-1}, \hspace{0.2cm}g_2 = g_{-2} \hspace{0.15cm}\underline { \approx | ns}) \hspace{0.15cm}\underline {= 0.105\,{\rm V}}= g_{-1}, \hspace{0.2cm}g_2 = g_{-2} \hspace{0.15cm}\underline { \approx | ||
| Line 87: | Line 87: | ||
| − | '''(4)''' | + | '''(4)''' Using the basic pulse values calculated in '''(3)''', we obtain for the vertical eye opening: |
:$$\ddot{o}(T_{\rm D}) = 2 \cdot (g_0 - g_1 - g_{-1}) = 2 \cdot | :$$\ddot{o}(T_{\rm D}) = 2 \cdot (g_0 - g_1 - g_{-1}) = 2 \cdot | ||
(0.790\,{\rm V} - 2\cdot 0.105\,{\rm V}) \hspace{0.15cm}\underline {= 1.16\,{\rm | (0.790\,{\rm V} - 2\cdot 0.105\,{\rm V}) \hspace{0.15cm}\underline {= 1.16\,{\rm | ||
V}}\hspace{0.05cm}.$$ | V}}\hspace{0.05cm}.$$ | ||
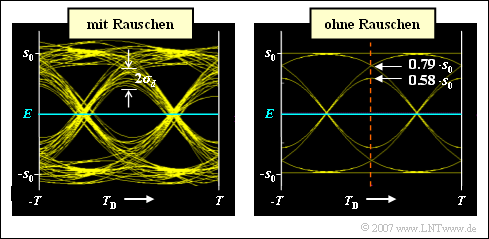
| − | [[File:P_ID1382__Dig_A_3_2d.png|right|frame| | + | [[File:P_ID1382__Dig_A_3_2d.png|right|frame|Eye diagram with and without noise]] |
| − | + | Thus, together with the noise rms value, we obtain for the worst-case error probability: | |
:$$p_{\rm U} = {\rm Q} \left( \frac{1.16\,{\rm | :$$p_{\rm U} = {\rm Q} \left( \frac{1.16\,{\rm | ||
V}/2}{ 0.188\,{\rm V}} | V}/2}{ 0.188\,{\rm V}} | ||
| Line 98: | Line 98: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The graph on the right shows the eye diagram without noise. One can see from this the vertical eye opening in the center of the symbol: | |
:$$\ddot{o}(T_{\rm D} = 0) = 2 \cdot 0.58 \cdot s_0.$$ | :$$\ddot{o}(T_{\rm D} = 0) = 2 \cdot 0.58 \cdot s_0.$$ | ||
<br clear=all> | <br clear=all> | ||
| − | '''(5)''' | + | '''(5)''' From the above eye diagram, one can see that the signal component at the detection time $T_{\rm D} = 0$ can assume six different values. In the upper half of the eye, these are: |
:$$1.)\hspace{0.2cm} g_0 + g_1 + g_{-1} = 0.790\,{\rm V} + 2\cdot 0.105\,{\rm | :$$1.)\hspace{0.2cm} g_0 + g_1 + g_{-1} = 0.790\,{\rm V} + 2\cdot 0.105\,{\rm | ||
V}= 1\,{\rm V} = s_0\hspace{0.3cm}\Rightarrow \hspace{0.3cm} | V}= 1\,{\rm V} = s_0\hspace{0.3cm}\Rightarrow \hspace{0.3cm} | ||
| Line 117: | Line 117: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | Averaging over these values with appropriate weighting $(p_2$ occurs twice as often as $p_1$ and $p_3$ auf$)$ gives: | |
:$$p_{\rm S} \ = \ {1}/{4} \cdot (p_{\rm 1} + 2 \cdot p_{\rm 2} + p_{\rm 3}) | :$$p_{\rm S} \ = \ {1}/{4} \cdot (p_{\rm 1} + 2 \cdot p_{\rm 2} + p_{\rm 3}) | ||
= {1}/{4} \cdot (5 \cdot 10^{-8} + 2 \cdot 1.3 \cdot 10^{-5} + 10^{-3}) | = {1}/{4} \cdot (5 \cdot 10^{-8} + 2 \cdot 1.3 \cdot 10^{-5} + 10^{-3}) | ||
\hspace{0.15cm}\underline { \approx 0.256 \cdot 10^{-3}} | \hspace{0.15cm}\underline { \approx 0.256 \cdot 10^{-3}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | Since $p_1$ and $p_2$ are much smaller than $p_3 = p_{\rm U}$, the average error probability is (almost) a factor of $4$ smaller than $p_{\rm U}$. | |
| − | '''(6)''' | + | '''(6)''' To reduce the error probability, $s_0$ must be increased. Thus, the approximation $p_{\rm S} ≈ p_{\rm U}/4$ is even more accurate: |
:$$p_{\rm S} \le 10^{-10}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}p_{\rm U} = {\rm Q} \left( \frac{0.58 \cdot s_0}{ 0.188\,{\rm V}} | :$$p_{\rm S} \le 10^{-10}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}p_{\rm U} = {\rm Q} \left( \frac{0.58 \cdot s_0}{ 0.188\,{\rm V}} | ||
\right)\le 4 \cdot 10^{-10}\hspace{0.3cm} | \right)\le 4 \cdot 10^{-10}\hspace{0.3cm} | ||
Revision as of 13:37, 26 April 2022
Let a binary bipolar redundancy-free baseband system with bit rate $R_{\rm B} = 100\,{\rm Mbit/s}$ and the following properties be given:
- Let the transmitted pulses be rectangular, and the possible amplitude values be $± 1\,{\rm V}$.
- The AWGN noise power density $($on the resistor $1 \, \Omega)$ is $10^{\rm -9} \, {\rm V}^2/{\rm Hz}$.
- A Gaussian low-pass filter with cutoff frequency $f_{\rm G} = 50 \, {\rm MHz}$ is used as the receiver filter. The frequency response is:
- $$H_{\rm G}(f) = {\rm e}^{- \pi \hspace{0.05cm}\cdot \hspace{0.05cm}{f}^2/({2f_{\rm G}})^2} \hspace{0.05cm}.$$
- The basic transmitter pulse $g_d(t) = g_s(t) * h_{\rm G}(t)$ is shown in the graph (red curve). Some prominent pulse values are indicated.
- The detection noise power can be calculated using the following equation:
- $$\sigma_d^2 = {N_0}/{2} \cdot \int_{-\infty}^{+\infty} |H_{\rm G}(f)|^2 \,{\rm d} f \hspace{0.05cm}.$$
For example, the eye diagram can be used to determine the error probability.
- The mean symbol error probability $p_{\rm S}$ is obtained from this after averaging over all possible detection useful samples.
- The worst-case error probability serves as an upper bound for $p_{\rm S}$.
- $$p_{\rm U} = {\rm Q} \left( \frac{\ddot{o}(T_{\rm D})/2}{ \sigma_d} \right) \hspace{0.3cm}{\rm with}\hspace{0.3cm}\frac{\ddot{o}(T_{\rm D})}{ 2}= g_d(t=0) - |g_d(t=T)|- |g_d(t=-T)|-\hspace{0.01cm}\text{ ...}$$
Here, $\ddot{o}(T_{\rm D})$ denotes the vertical eye opening. Let the detection time $T_{\rm D} = 0$ be optimally chosen.
Notes:
- The exercise belongs to the chapter Error Probability with Intersymbol Interference.
- Use the interaction module Complementary Gaussian Error Functions for the numerical evaluation of the Q-function.
Questions
Solution
- $$T = \frac{1}{10^8\,{\rm bit/s}} = 10^{-8}\,{\rm s}\hspace{0.15cm}\underline { = 10\,{\rm ns}} \hspace{0.05cm}.$$
(2) Integration according to the given equation leads to:
- $$\sigma_d^2 = \frac{N_0}{2} \cdot \int_{-\infty}^{+\infty} |H_{\rm G}(f)|^2 \,{\rm d} f = \frac{N_0 \cdot f_{\rm G}}{\sqrt{2}}= \frac{10^{-9}\,{\rm V/Hz} \cdot 5 \cdot 10^{7}\,{\rm Hz} }{\sqrt{2}}\approx 0.035\,{\rm V^2}\hspace{0.3cm} \Rightarrow \hspace{0.3cm}\sigma_d \hspace{0.15cm}\underline { = 0.188\,{\rm V}}\hspace{0.05cm}.$$
(3) These values can be obtained from the graph:
- $$g_0 = g_d(0)\hspace{0.15cm}\underline { = 0.790\,{\rm V}}, \hspace{0.2cm}g_1 = g_d(10\,{\rm ns}) \hspace{0.15cm}\underline {= 0.105\,{\rm V}}= g_{-1}, \hspace{0.2cm}g_2 = g_{-2} \hspace{0.15cm}\underline { \approx 0} \hspace{0.05cm}.$$
(4) Using the basic pulse values calculated in (3), we obtain for the vertical eye opening:
- $$\ddot{o}(T_{\rm D}) = 2 \cdot (g_0 - g_1 - g_{-1}) = 2 \cdot (0.790\,{\rm V} - 2\cdot 0.105\,{\rm V}) \hspace{0.15cm}\underline {= 1.16\,{\rm V}}\hspace{0.05cm}.$$
Thus, together with the noise rms value, we obtain for the worst-case error probability:
- $$p_{\rm U} = {\rm Q} \left( \frac{1.16\,{\rm V}/2}{ 0.188\,{\rm V}} \right) \approx {\rm Q}(3.08)\hspace{0.15cm}\underline {\approx 10^{-3}} \hspace{0.05cm}.$$
The graph on the right shows the eye diagram without noise. One can see from this the vertical eye opening in the center of the symbol:
- $$\ddot{o}(T_{\rm D} = 0) = 2 \cdot 0.58 \cdot s_0.$$
(5) From the above eye diagram, one can see that the signal component at the detection time $T_{\rm D} = 0$ can assume six different values. In the upper half of the eye, these are:
- $$1.)\hspace{0.2cm} g_0 + g_1 + g_{-1} = 0.790\,{\rm V} + 2\cdot 0.105\,{\rm V}= 1\,{\rm V} = s_0\hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm 1} = {\rm Q} \left( \frac{1\,{\rm V}}{ 0.188\,{\rm V}} \right) \approx 5 \cdot 10^{-8} \hspace{0.05cm},$$
- $$2.)\hspace{0.2cm} g_0 = 0.790\,{\rm V} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm 2} = {\rm Q} \left( \frac{0.790\,{\rm V}}{ 0.188\,{\rm V}} \right) \approx 1.3 \cdot 10^{-5} \hspace{0.05cm},$$
- $$3.)\hspace{0.2cm} g_0 - g_1 - g_{-1} = 0.580\,{\rm V} = \ddot{o}(T_{\rm D})/2\hspace{0.3cm}\Rightarrow \hspace{0.3cm}p_{\rm 3} = p_{\rm U} \approx 10^{-3} \hspace{0.05cm}.$$
Averaging over these values with appropriate weighting $(p_2$ occurs twice as often as $p_1$ and $p_3$ auf$)$ gives:
- $$p_{\rm S} \ = \ {1}/{4} \cdot (p_{\rm 1} + 2 \cdot p_{\rm 2} + p_{\rm 3}) = {1}/{4} \cdot (5 \cdot 10^{-8} + 2 \cdot 1.3 \cdot 10^{-5} + 10^{-3}) \hspace{0.15cm}\underline { \approx 0.256 \cdot 10^{-3}} \hspace{0.05cm}.$$
Since $p_1$ and $p_2$ are much smaller than $p_3 = p_{\rm U}$, the average error probability is (almost) a factor of $4$ smaller than $p_{\rm U}$.
(6) To reduce the error probability, $s_0$ must be increased. Thus, the approximation $p_{\rm S} ≈ p_{\rm U}/4$ is even more accurate:
- $$p_{\rm S} \le 10^{-10}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}p_{\rm U} = {\rm Q} \left( \frac{0.58 \cdot s_0}{ 0.188\,{\rm V}} \right)\le 4 \cdot 10^{-10}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} \frac{0.58 \cdot s_0}{ 0.188\,{\rm V}} \ge 6.15 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}s_0 \ge 1.993\,{\rm V} \hspace{0.15cm}\underline { \approx 2\,{\rm V}} \hspace{0.05cm}.$$