Difference between revisions of "Aufgaben:Exercise 3.3Z: Characteristics Determination"
m (Text replacement - "Category:Aufgaben zu Modulationsverfahren" to "Category:Modulation Methods: Exercises") |
m |
||
| (10 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Phase_Modulation_(PM) |
}} | }} | ||
| − | [[File:P_ID1085__Mod_Z_3_3.png|right|frame| | + | [[File:P_ID1085__Mod_Z_3_3.png|right|frame|Spectrum of the analytical signal]] |
| − | + | Let us consider the phase modulation of the harmonic oscillation | |
:$$q(t) = A_{\rm N} \cdot \cos(2 \pi \cdot f_{\rm N} \cdot t + \phi_{\rm N}) \hspace{0.05cm},$$ | :$$q(t) = A_{\rm N} \cdot \cos(2 \pi \cdot f_{\rm N} \cdot t + \phi_{\rm N}) \hspace{0.05cm},$$ | ||
| − | + | which, given a normalized carrier amplitude $(A_{\rm T} = 1)$ , leads to the following transmitted signal: | |
:$$ s(t) = \cos \hspace{-0.1cm}\big[\omega_{\rm T} \cdot t + K_{\rm PM} \cdot q(t) \big]\hspace{0.05cm}.$$ | :$$ s(t) = \cos \hspace{-0.1cm}\big[\omega_{\rm T} \cdot t + K_{\rm PM} \cdot q(t) \big]\hspace{0.05cm}.$$ | ||
| − | + | The spectrum of the corresponding analytical signal $s_{\rm TP}(t)$ is generally: | |
:$$S_{\rm TP}(f) = \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}(\phi_{\rm N}\hspace{0.05cm}+\hspace{0.05cm} 90^\circ) }\cdot \hspace{0.05cm} \delta (f - n \cdot f_{\rm N})\hspace{0.05cm}$$ | :$$S_{\rm TP}(f) = \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}(\phi_{\rm N}\hspace{0.05cm}+\hspace{0.05cm} 90^\circ) }\cdot \hspace{0.05cm} \delta (f - n \cdot f_{\rm N})\hspace{0.05cm}$$ | ||
| − | + | Here, $η = K_{\rm PM} · A_{\rm N}$ is called the modulation index. | |
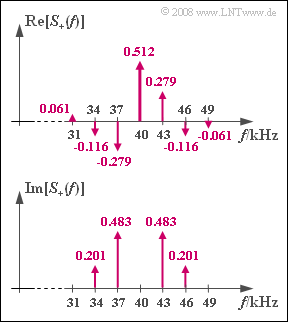
| − | In | + | In the graph, the real and imaginary parts of the spectrum $S_+(f)$ of the analytical signal $s_+(t)$ are shown separately. This should be used to determine the characteristics $f_{\rm T}$, $f_{\rm N}$, $ϕ_{\rm N}$ and $η$ . |
| Line 22: | Line 22: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Modulation_Methods/Phase_Modulation_(PM)|Phase Modulation]]. |
| − | * | + | *Particular reference is made to the page [[Modulation_Methods/Phase_Modulation_(PM)#Equivalent_low-pass_signal_in_phase_modulation|Equivalent low-pass signal in phase modulation]]. |
| − | * | + | *For the calculation of the modulation index, you can take advantage of the following property of the Bessel function: |
:$${\rm J}_n (\eta) = \frac{2 \cdot (n-1)}{\eta} \cdot {\rm J}_{n-1} (\eta) - {\rm J}_{n-2} (\eta) \hspace{0.3cm}\Rightarrow \hspace{0.3cm}{\rm J}_{2} (\eta)= {2}/{\eta} \cdot {\rm J}_{1} (\eta) - {\rm J}_{0} (\eta) \hspace{0.05cm}.$$ | :$${\rm J}_n (\eta) = \frac{2 \cdot (n-1)}{\eta} \cdot {\rm J}_{n-1} (\eta) - {\rm J}_{n-2} (\eta) \hspace{0.3cm}\Rightarrow \hspace{0.3cm}{\rm J}_{2} (\eta)= {2}/{\eta} \cdot {\rm J}_{1} (\eta) - {\rm J}_{0} (\eta) \hspace{0.05cm}.$$ | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What are the frequencies $f_{\rm T}$ and $f_{\rm N}$? |
|type="{}"} | |type="{}"} | ||
$f_{\rm T} \ = \ $ { 40 3% } $\ \rm kHz$ | $f_{\rm T} \ = \ $ { 40 3% } $\ \rm kHz$ | ||
$f_{\rm N} \ = \ $ { 3 3% } $\ \rm kHz$ | $f_{\rm N} \ = \ $ { 3 3% } $\ \rm kHz$ | ||
| − | { | + | {Calculate the magnitude and the phase of $S_{\rm TP}(f = 3 \ \rm kHz)$. |
|type="{}"} | |type="{}"} | ||
$|S_{\rm TP}(f = 3 \ \rm kHz)| \ = \ $ { 0.558 3% } | $|S_{\rm TP}(f = 3 \ \rm kHz)| \ = \ $ { 0.558 3% } | ||
${\rm arc} \ S_{\rm TP}(f = 3\ \rm kHz) \ = \ $ { 60 3% } $\ \rm Grad$ | ${\rm arc} \ S_{\rm TP}(f = 3\ \rm kHz) \ = \ $ { 60 3% } $\ \rm Grad$ | ||
| − | { | + | {Calculate the magnitude and the phase of $S_{\rm TP}(f = 6 \ \rm kHz)$. |
|type="{}"} | |type="{}"} | ||
$|S_{\rm TP}(f = 6 \ \rm kHz)| \ = \ $ { 0.232 3% } | $|S_{\rm TP}(f = 6 \ \rm kHz)| \ = \ $ { 0.232 3% } | ||
${\rm arc} \ S_{\rm TP}(f = 6\ \rm kHz) \ = \ $ { 120 3% } $\ \rm Grad$ | ${\rm arc} \ S_{\rm TP}(f = 6\ \rm kHz) \ = \ $ { 120 3% } $\ \rm Grad$ | ||
| − | { | + | {What is the phase of the source signal $q(t)$? |
|type="{}"} | |type="{}"} | ||
$ϕ_{\rm N} \ = \ $ { -30.9--29.1 } $\ \rm Grad$ | $ϕ_{\rm N} \ = \ $ { -30.9--29.1 } $\ \rm Grad$ | ||
| − | { | + | {What is the magnitude of the modulation index $η$ ? |
|type="{}"} | |type="{}"} | ||
$η \ = \ $ { 1.5 3% } | $η \ = \ $ { 1.5 3% } | ||
| Line 60: | Line 60: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Regarding $|S_+(f)|$ there is a symmetry with respect to the carrier frequency $f_{\rm T}\hspace{0.15cm}\underline { = 40 \ \rm kHz}$. The distance between the spectral lines is $f_{\rm N}\hspace{0.15cm}\underline { = 3 \ \rm kHz}$. |
| − | '''(2)''' | + | '''(2)''' Considering $S_{\rm TP}(f = 3{\ \rm kHz}) = S_+(f = 43 \ \rm kHz)$ , it holds that: |
:$$|S_{\rm TP}(f = 3\,{\rm kHz})| = \sqrt{0.279^2 + 0.483^2} \hspace{0.15cm}\underline {= 0.558}\hspace{0.05cm},$$ | :$$|S_{\rm TP}(f = 3\,{\rm kHz})| = \sqrt{0.279^2 + 0.483^2} \hspace{0.15cm}\underline {= 0.558}\hspace{0.05cm},$$ | ||
:$$ {\rm arc}\hspace{0.15cm} S_{\rm TP}(f = 3\,{\rm kHz}) = \arctan \frac{0.483}{0.279} = \arctan 1.732\hspace{0.15cm}\underline { = 60^\circ} \hspace{0.05cm}.$$ | :$$ {\rm arc}\hspace{0.15cm} S_{\rm TP}(f = 3\,{\rm kHz}) = \arctan \frac{0.483}{0.279} = \arctan 1.732\hspace{0.15cm}\underline { = 60^\circ} \hspace{0.05cm}.$$ | ||
| Line 72: | Line 72: | ||
| − | '''(3)''' | + | '''(3)''' Analogously to in question '''(2)''' , at frequency $f = 6 \ \rm kHz$ we obtain: |
:$$|S_{\rm TP}(f = 6\,{\rm kHz})| = \sqrt{(-0.116)^2 + 0.201^2} \hspace{0.15cm}\underline {= 0.232}\hspace{0.05cm},$$ | :$$|S_{\rm TP}(f = 6\,{\rm kHz})| = \sqrt{(-0.116)^2 + 0.201^2} \hspace{0.15cm}\underline {= 0.232}\hspace{0.05cm},$$ | ||
:$${\rm arc}\hspace{0.15cm} S_{\rm TP}(f = 6\,{\rm kHz}) = \arctan \frac{-0.116}{0.201} = 180^\circ - \arctan 1.732 \hspace{0.15cm}\underline {= 120^\circ} \hspace{0.05cm}.$$ | :$${\rm arc}\hspace{0.15cm} S_{\rm TP}(f = 6\,{\rm kHz}) = \arctan \frac{-0.116}{0.201} = 180^\circ - \arctan 1.732 \hspace{0.15cm}\underline {= 120^\circ} \hspace{0.05cm}.$$ | ||
| Line 78: | Line 78: | ||
| − | '''(4)''' | + | '''(4)''' When $n = 1$ ⇒ $f = 3 \ \rm kHz$ as in question '''(2)''', the phase is: |
:$$ \phi_{\rm N} + 90^\circ = 60^\circ \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\phi_{\rm N} = -30^\circ\hspace{0.05cm}.$$ | :$$ \phi_{\rm N} + 90^\circ = 60^\circ \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\phi_{\rm N} = -30^\circ\hspace{0.05cm}.$$ | ||
| − | * | + | *Checking this result when $n = 2$ ⇒ $f = 6 \ \rm kHz$ as in question '''(3)''' yields the same value: |
:$$ 2\cdot (\phi_{\rm N} + 90^\circ) = 120^\circ \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\phi_{\rm N} \hspace{0.15cm}\underline {= -30^\circ}\hspace{0.05cm}.$$ | :$$ 2\cdot (\phi_{\rm N} + 90^\circ) = 120^\circ \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\phi_{\rm N} \hspace{0.15cm}\underline {= -30^\circ}\hspace{0.05cm}.$$ | ||
| − | '''(5)''' | + | '''(5)''' The given equation can be rewritten as follows: |
:$$\eta = \frac{2 \cdot {\rm J}_{1}{(\eta)}}{{\rm J}_{0}(\eta) + {\rm J}_{2}(\eta)} \hspace{0.05cm}.$$ | :$$\eta = \frac{2 \cdot {\rm J}_{1}{(\eta)}}{{\rm J}_{0}(\eta) + {\rm J}_{2}(\eta)} \hspace{0.05cm}.$$ | ||
| − | * | + | *With ${\rm J}_0(η) = 0.512$, ${\rm J}_1(η) = 0.558$ and ${\rm J}_2(η) = 0.232$ we thus get: |
:$$ \eta = \frac{2 \cdot 0.558}{0.512 + 0.232}\hspace{0.15cm}\underline { = 1.5}\hspace{0.05cm}.$$ | :$$ \eta = \frac{2 \cdot 0.558}{0.512 + 0.232}\hspace{0.15cm}\underline { = 1.5}\hspace{0.05cm}.$$ | ||
| Line 95: | Line 95: | ||
| − | [[Category:Modulation Methods: Exercises|^3.1 | + | [[Category:Modulation Methods: Exercises|^3.1 Phase Modulation^]] |
Latest revision as of 17:03, 9 April 2022
Let us consider the phase modulation of the harmonic oscillation
- $$q(t) = A_{\rm N} \cdot \cos(2 \pi \cdot f_{\rm N} \cdot t + \phi_{\rm N}) \hspace{0.05cm},$$
which, given a normalized carrier amplitude $(A_{\rm T} = 1)$ , leads to the following transmitted signal:
- $$ s(t) = \cos \hspace{-0.1cm}\big[\omega_{\rm T} \cdot t + K_{\rm PM} \cdot q(t) \big]\hspace{0.05cm}.$$
The spectrum of the corresponding analytical signal $s_{\rm TP}(t)$ is generally:
- $$S_{\rm TP}(f) = \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}(\phi_{\rm N}\hspace{0.05cm}+\hspace{0.05cm} 90^\circ) }\cdot \hspace{0.05cm} \delta (f - n \cdot f_{\rm N})\hspace{0.05cm}$$
Here, $η = K_{\rm PM} · A_{\rm N}$ is called the modulation index.
In the graph, the real and imaginary parts of the spectrum $S_+(f)$ of the analytical signal $s_+(t)$ are shown separately. This should be used to determine the characteristics $f_{\rm T}$, $f_{\rm N}$, $ϕ_{\rm N}$ and $η$ .
Hints:
- This exercise belongs to the chapter Phase Modulation.
- Particular reference is made to the page Equivalent low-pass signal in phase modulation.
- For the calculation of the modulation index, you can take advantage of the following property of the Bessel function:
- $${\rm J}_n (\eta) = \frac{2 \cdot (n-1)}{\eta} \cdot {\rm J}_{n-1} (\eta) - {\rm J}_{n-2} (\eta) \hspace{0.3cm}\Rightarrow \hspace{0.3cm}{\rm J}_{2} (\eta)= {2}/{\eta} \cdot {\rm J}_{1} (\eta) - {\rm J}_{0} (\eta) \hspace{0.05cm}.$$
Questions
Solution
(2) Considering $S_{\rm TP}(f = 3{\ \rm kHz}) = S_+(f = 43 \ \rm kHz)$ , it holds that:
- $$|S_{\rm TP}(f = 3\,{\rm kHz})| = \sqrt{0.279^2 + 0.483^2} \hspace{0.15cm}\underline {= 0.558}\hspace{0.05cm},$$
- $$ {\rm arc}\hspace{0.15cm} S_{\rm TP}(f = 3\,{\rm kHz}) = \arctan \frac{0.483}{0.279} = \arctan 1.732\hspace{0.15cm}\underline { = 60^\circ} \hspace{0.05cm}.$$
(3) Analogously to in question (2) , at frequency $f = 6 \ \rm kHz$ we obtain:
- $$|S_{\rm TP}(f = 6\,{\rm kHz})| = \sqrt{(-0.116)^2 + 0.201^2} \hspace{0.15cm}\underline {= 0.232}\hspace{0.05cm},$$
- $${\rm arc}\hspace{0.15cm} S_{\rm TP}(f = 6\,{\rm kHz}) = \arctan \frac{-0.116}{0.201} = 180^\circ - \arctan 1.732 \hspace{0.15cm}\underline {= 120^\circ} \hspace{0.05cm}.$$
(4) When $n = 1$ ⇒ $f = 3 \ \rm kHz$ as in question (2), the phase is:
- $$ \phi_{\rm N} + 90^\circ = 60^\circ \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\phi_{\rm N} = -30^\circ\hspace{0.05cm}.$$
- Checking this result when $n = 2$ ⇒ $f = 6 \ \rm kHz$ as in question (3) yields the same value:
- $$ 2\cdot (\phi_{\rm N} + 90^\circ) = 120^\circ \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\phi_{\rm N} \hspace{0.15cm}\underline {= -30^\circ}\hspace{0.05cm}.$$
(5) The given equation can be rewritten as follows:
- $$\eta = \frac{2 \cdot {\rm J}_{1}{(\eta)}}{{\rm J}_{0}(\eta) + {\rm J}_{2}(\eta)} \hspace{0.05cm}.$$
- With ${\rm J}_0(η) = 0.512$, ${\rm J}_1(η) = 0.558$ and ${\rm J}_2(η) = 0.232$ we thus get:
- $$ \eta = \frac{2 \cdot 0.558}{0.512 + 0.232}\hspace{0.15cm}\underline { = 1.5}\hspace{0.05cm}.$$