Difference between revisions of "Aufgaben:Exercise 1.5Z: Probabilities of Default"
From LNTwww
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Statistical_Dependence_and_Independence}} |
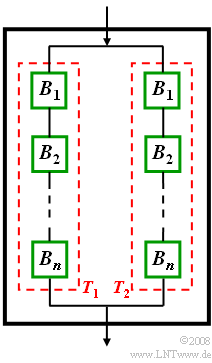
| − | [[File:P_ID87__Sto_Z_1_5.png|right|frame| | + | [[File:P_ID87__Sto_Z_1_5.png|right|frame|Functional circuit diagram of a device]] |
| − | + | A device part is composed of the components $B_1, \ B_2,\ \text{...} \ , B_n$ where the respective functionality can be assumed to be independent of all other components. | |
| − | * | + | *Assume that all components default with equal probability $p_{\rm A}$ . |
| − | * | + | *Part $T_1$ functions only if all $n$ components are functional. |
| − | + | To increase reliability, important assemblies are often duplicated. The device $G$ can thus be described in terms of set theory as follows: | |
:$$ G = T_1 \cup T_2.$$ | :$$ G = T_1 \cup T_2.$$ | ||
| − | + | This means: Device $G$ is already operational if at least one of the two identical subassemblies $(T_1$ or $T_2)$ is functional. | |
| Line 21: | Line 21: | ||
| − | + | Hints: | |
| − | * | + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Statistical_Dependence_and_Independence|Statistical dependence and independence]]. |
| − | * | + | *The topic of this chapter is illustrated with examples in the (German language) learning video |
| − | + | :[[Statistische_Abhängigkeit_und_Unabhängigkeit_(Lernvideo)|Statistische Abhängigkeit und Unabhängigkeit]] $\Rightarrow$ "Statistical dependence and independence". | |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {The default probability $p_{\rm G}$ of the total device must not be greater than $0.04\%$. <br>How large may then the default probabilities $p_{\rm T}$ of the two identical device parts existing in parallel be at most? |
|type="{}"} | |type="{}"} | ||
$p_\text{T, max} \ = \ $ { 2 3% } $ \ \%$ | $p_\text{T, max} \ = \ $ { 2 3% } $ \ \%$ | ||
| − | { | + | {Let the default probability of all components be $\underline{p_{\rm A} = 0.1}$. Let each subdevice consist of $n = 3$ components. <br>Calculate the probability $p_{\rm T}$ exactly that a subdevice defaults. |
|type="{}"} | |type="{}"} | ||
$p_{\rm T} \ = \ $ { 27.1 3% } $ \ \%$ | $p_{\rm T} \ = \ $ { 27.1 3% } $ \ \%$ | ||
| − | { | + | {What value is obtained for $\underline{p_{\rm A} = 0.01}$? In what form can you approximate $p_{\rm T}$ for small values of $p_{\rm A}$ ? |
|type="{}"} | |type="{}"} | ||
$p_{\rm T} \ = \ $ { 2.97 3% } $ \ \%$ | $p_{\rm T} \ = \ $ { 2.97 3% } $ \ \%$ | ||
| − | { | + | {Now apply $p_{\rm A} = 0.4\%$ for the default probability of all components. What is the maximum number of components the subdevice can contain $p_{\rm T} ≤ 2\%$ is to hold? |
|type="{}"} | |type="{}"} | ||
$n \ = \ $ { 5 3% } | $n \ = \ $ { 5 3% } | ||
| Line 50: | Line 50: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Since the two subdevices default independently, set-theoretically holds: |
:$$\rm Pr(\it G \rm \hspace{0.1cm}f\ddot{a}llt\hspace{0.1cm}aus) = Pr(\it T_{\rm 1}\rm \hspace{0.1cm} f\ddot{a}llt \hspace{0.1cm}aus) \cdot Pr(\it T_{\rm 2}\rm \hspace{0.1cm} f\ddot{a}llt \hspace{0.1cm}aus). $$ | :$$\rm Pr(\it G \rm \hspace{0.1cm}f\ddot{a}llt\hspace{0.1cm}aus) = Pr(\it T_{\rm 1}\rm \hspace{0.1cm} f\ddot{a}llt \hspace{0.1cm}aus) \cdot Pr(\it T_{\rm 2}\rm \hspace{0.1cm} f\ddot{a}llt \hspace{0.1cm}aus). $$ | ||
| − | * | + | *Moreover, since subdevices $T_1$ and $T_2$ are identical in construction, they default with the same probability $p_{\rm T}$ . It follows that: |
:$$p_{\rm G} = \it p_{\rm T}^{\rm 2} \hspace{0.5cm} \rm bzw. \hspace{0.5cm} \rm \it p_{\rm T,\hspace{0.1cm}max}= \sqrt{\it p_{\rm G}} \le \rm\sqrt{0.0004} \hspace{0.15cm}\underline {= 2\%}.$$ | :$$p_{\rm G} = \it p_{\rm T}^{\rm 2} \hspace{0.5cm} \rm bzw. \hspace{0.5cm} \rm \it p_{\rm T,\hspace{0.1cm}max}= \sqrt{\it p_{\rm G}} \le \rm\sqrt{0.0004} \hspace{0.15cm}\underline {= 2\%}.$$ | ||
| − | '''(2)''' | + | '''(2)''' This result is easier to determine using the complementary event: |
| − | :$$\rm Pr(\it T_{\rm 1}\hspace{0.1cm}\rm | + | :$$\rm Pr(\it T_{\rm 1}\hspace{0.1cm}\rm functions) = \rm Pr(\it B_{\rm 1} \hspace{0.1cm}\rm functions \cap \it B_{\rm 2} \hspace{0.1cm} \rm functions \cap \it B_{\rm 3}\hspace{0.1cm} \rm functions).$$ |
:$$\Rightarrow 1- p_{\rm T}= (1-p_{\rm A})^{3} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | :$$\Rightarrow 1- p_{\rm T}= (1-p_{\rm A})^{3} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ||
1-p_{\rm T}=(0.9)^3= 0.729 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_{\rm T}\hspace{0.15cm}\underline {= 0.271 = 27.1\%}.$$ | 1-p_{\rm T}=(0.9)^3= 0.729 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_{\rm T}\hspace{0.15cm}\underline {= 0.271 = 27.1\%}.$$ | ||
| Line 66: | Line 66: | ||
| − | '''(3)''' | + | '''(3)''' With $p_{\rm A} = 0.01$ , we obtain $p_{\rm T}\hspace{0.15cm}\underline {= 2.97\%}.$ |
| − | * | + | *In general, the approximation is: $p_{\rm T} \approx n \cdot p_{\rm A}\; (= 3\%)$. |
| − | '''(4)''' | + | '''(4)''' With the approximation of the last subtaks $\underline{n = 5}$ follows directly. |
| − | * | + | *For larger $p_{\rm A}$ , one would have to proceed as follows: |
:$$0.996^{\it n}\ge 0.98 \hspace{0.5cm} \rm\Rightarrow \hspace{0.5cm} \it n\le\rm\frac{log(0.98)}{log(0.996)} = 5.0406\hspace{0.15cm}\underline { \approx 5}.$$ | :$$0.996^{\it n}\ge 0.98 \hspace{0.5cm} \rm\Rightarrow \hspace{0.5cm} \it n\le\rm\frac{log(0.98)}{log(0.996)} = 5.0406\hspace{0.15cm}\underline { \approx 5}.$$ | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 23:03, 28 November 2021
A device part is composed of the components $B_1, \ B_2,\ \text{...} \ , B_n$ where the respective functionality can be assumed to be independent of all other components.
- Assume that all components default with equal probability $p_{\rm A}$ .
- Part $T_1$ functions only if all $n$ components are functional.
To increase reliability, important assemblies are often duplicated. The device $G$ can thus be described in terms of set theory as follows:
- $$ G = T_1 \cup T_2.$$
This means: Device $G$ is already operational if at least one of the two identical subassemblies $(T_1$ or $T_2)$ is functional.
Hints:
- The exercise belongs to the chapter Statistical dependence and independence.
- The topic of this chapter is illustrated with examples in the (German language) learning video
- Statistische Abhängigkeit und Unabhängigkeit $\Rightarrow$ "Statistical dependence and independence".
Questions
Solution
(1) Since the two subdevices default independently, set-theoretically holds:
- $$\rm Pr(\it G \rm \hspace{0.1cm}f\ddot{a}llt\hspace{0.1cm}aus) = Pr(\it T_{\rm 1}\rm \hspace{0.1cm} f\ddot{a}llt \hspace{0.1cm}aus) \cdot Pr(\it T_{\rm 2}\rm \hspace{0.1cm} f\ddot{a}llt \hspace{0.1cm}aus). $$
- Moreover, since subdevices $T_1$ and $T_2$ are identical in construction, they default with the same probability $p_{\rm T}$ . It follows that:
- $$p_{\rm G} = \it p_{\rm T}^{\rm 2} \hspace{0.5cm} \rm bzw. \hspace{0.5cm} \rm \it p_{\rm T,\hspace{0.1cm}max}= \sqrt{\it p_{\rm G}} \le \rm\sqrt{0.0004} \hspace{0.15cm}\underline {= 2\%}.$$
(2) This result is easier to determine using the complementary event:
- $$\rm Pr(\it T_{\rm 1}\hspace{0.1cm}\rm functions) = \rm Pr(\it B_{\rm 1} \hspace{0.1cm}\rm functions \cap \it B_{\rm 2} \hspace{0.1cm} \rm functions \cap \it B_{\rm 3}\hspace{0.1cm} \rm functions).$$
- $$\Rightarrow 1- p_{\rm T}= (1-p_{\rm A})^{3} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} 1-p_{\rm T}=(0.9)^3= 0.729 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_{\rm T}\hspace{0.15cm}\underline {= 0.271 = 27.1\%}.$$
(3) With $p_{\rm A} = 0.01$ , we obtain $p_{\rm T}\hspace{0.15cm}\underline {= 2.97\%}.$
- In general, the approximation is: $p_{\rm T} \approx n \cdot p_{\rm A}\; (= 3\%)$.
(4) With the approximation of the last subtaks $\underline{n = 5}$ follows directly.
- For larger $p_{\rm A}$ , one would have to proceed as follows:
- $$0.996^{\it n}\ge 0.98 \hspace{0.5cm} \rm\Rightarrow \hspace{0.5cm} \it n\le\rm\frac{log(0.98)}{log(0.996)} = 5.0406\hspace{0.15cm}\underline { \approx 5}.$$