Exercise 3.5: PM and FM for Rectangular Signals

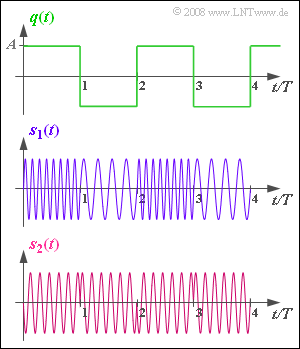
Two signal waveforms in angle modulation

Assume a bipolar and rectangular source signal $q(t)$ , as shown in the upper diagram. This signal can only take on the two signal values $±A = ±2 \ \rm V$ and the duration of the positive and negative rectangles are each $T = 1 \ \rm ms$ . The period of $q(t)$ is therefore $T_0 = 2 \ \rm ms$ .

The signals $s_1(t)$ and $s_2(t)$ display two transmitted signals with angle modulation $\rm (WM)$ , each of which can be represented as

$s(t) = A_{\rm T} \cdot \cos \hspace{-0.05cm}\big [\psi (t) \big ]$

Here, we distinguish between phase modulation $\rm (PM)$ with the angular function

$\psi(t) = \omega_{\rm T} \cdot t + \phi(t) = \omega_{\rm T} \cdot t + K_{\rm PM} \cdot q(t)$

and frequency modulation $\rm (FM)$ , where the instantaneous freqiency is linearly related to $q(t)$ :

$f_{\rm A}(t) = \frac{\omega_{\rm A}(t)}{2\pi}, \hspace{0.3cm} \omega_{\rm A}(t) = \frac{{\rm d}\hspace{0.03cm}\psi(t)}{{\rm d}t}= \omega_{\rm T} + K_{\rm FM} \cdot q(t)\hspace{0.05cm}.$

$K_{\rm PM}$ and $K_{\rm FM}$ denote the dimensionally constrained constants given by the realizations of the PM and FM modulators, respectively. The frequency deviation $Δf_{\rm A}$ indicates the maximum deviation of the instantaneous frequency from the carrier frequency.

Hints:

This exercise belongs to the chapter Frequency Modulation.
Reference is also made to the chapter Phase Modulation.

In anticipation of the fourth chapter, it should be mentioned that phase modulation with a digital input signal is also called Phase Shift Keying $\rm (PSK)$ and frequency modulation is analogously called Frequency Shift Keying $\rm (FSK)$ .

Questions

	$s_1(t)$ represents a phase modulation.
	$s_1(t)$ represents a frequency modulation.

$ϕ_{\rm T} \ = \$

$\ \rm Grad$

$f_{\rm T} · T \ = \$

$K_{\rm PM} \ = \$

$\ \rm V^{-1}$

$Δf_{\rm A} · T \ = \$

$K_{\rm FM} \ = \$

$\ \rm (Vs)^{-1}$

Solution

(1) Answer 2 is correct:

For a rectangular (digital) source signal, phase modulation (PM) can be recognised by the typical phase jumps – see the signal waveform $s_2(t)$ .
Frequency modulation (FM), on the other hand, has diverse instantaneous frequencies at different times, as in $s_1(t)$ .

(2) When $q(t) = 0$ , the equations provided for both PM and FM give

$s(t) = A_{\rm T} \cdot \cos (\omega_{\rm T} \cdot t ) \hspace{0.3cm}\Rightarrow\hspace{0.3cm} \phi_{\rm T} \hspace{0.15cm}\underline {= 0}\hspace{0.05cm}.$

(3) The carrier frequency $f_{\rm T}$ can be directly determined only from the PM signal $s_2(t)$ .

By counting the oscillations of $s_2(t)$ in the time interval $T$ , it can be seen that $f_{\rm T} · T\hspace{0.15cm}\underline{ = 6}$ was used.
When frequency modulating a bipolar source signal, $f_{\rm T}$ does not occur directly.
However, the graphs do indicate that $f_{\rm T} · T = 6$ is also used here.

(4) The amplitude value $A = 2 \ \rm V$ results in the phase $90^\circ$ or $π/2$ (minus sine wave). This gives:

$K_{\rm PM} = \frac {\pi /2}{2\,{\rm V}} \hspace{0.15cm}\underline {= 0.785\,{\rm V}^{-1}} \hspace{0.05cm}.$

(5) The graph for $s_1(t)$ shows that either four or eight oscillations arise within a time interval $T$ : $4 \le f_{\rm A}(t) \cdot T \le 8\hspace{0.05cm}.$

Considering the (normalized) carrier frequency $f_{\rm T} · T = 6$ , the (normalized) frequency deviation is:

$\Delta f_{\rm A} \cdot T \hspace{0.15cm}\underline {=2}\hspace{0.05cm}.$

(6) The frequency deviation can also be represented as follows:

$\Delta f_{\rm A} = \frac {K_{\rm FM}}{2\pi}\cdot A \hspace{0.05cm}.$

With $Δf_{\rm A} · {\rm A} = 2$ we thus get:

$K_{\rm FM} = \frac {2 \cdot 2\pi}{A \cdot T}= \frac {4\pi}{2\,{\rm V} \cdot 1\,{\rm ms}}\hspace{0.15cm}\underline {= 6283 \,{\rm V}^{-1}{\rm s}^{-1}} \hspace{0.05cm}.$