Exercise 3.9Z: Convolution of Gaussian Pulses
From LNTwww
(Redirected from Aufgabe 3.9Z: Gauß gefaltet mit Gauß)
The convolution result of two Gaussian functions is to be determined. We consider
- a Gaussian input pulse ${x(t)}$ with amplitude $x_0 = 1\,\text{V}$ and "equivalent pulse duration" $\Delta t_x = 4 \,\text{ms}$, as well as
- a likewise Gaussian impulse response ${h(t)}$, which has the "equivalent pulse duration" $\Delta t_h = 3 \,\text{ms}$ :
- $$x( t ) = x_0 \cdot {\rm{e}}^{ - {\rm{\pi }}( {t/\Delta t_x } )^2 } ,$$
- $$h( t ) = \frac{1}{\Delta t_h } \cdot {\rm{e}}^{ - {\rm{\pi }}( {t/\Delta t_h } )^2 } .$$
The output signal ${y(t)} = {x(t)} ∗{h(t)}$ is sought, whereby the diversions via the spectral functions is to be taken.
Hint:
- This exercise belongs to the chapter The Convolution Theorem and Operation.
Questions
Solution
(1) By Fourier transformation one obtains:
- $$X( f ) = x_0 \cdot \Delta t_x \cdot {\rm{e}}^{ - {\rm{\pi }}\left( {\Delta t_x \hspace{0.05cm}\cdot \hspace{0.05cm} f} \right)^2 } , \hspace{0.5cm}H(f) = {\rm{e}}^{ - {\rm{\pi }}\left( {\Delta t_h \hspace{0.05cm}\cdot \hspace{0.05cm}f} \right)^2 } .$$
- The values we are looking for are
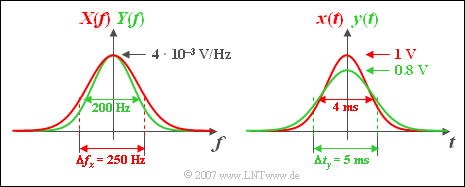
- $$X(f = 0)\;\underline{ = 4 \,\text{mV/Hz}}, \hspace{0.5cm} H(f = 0)\; \underline{= 1}.$$
(2) Convolution in time domain corresponds to multiplication in frequency domain:
- $$Y(f) = X(f) \cdot H(f) = x_0 \cdot \Delta t_x \cdot {\rm{e}}^{ - {\rm{\pi }}\left( {\Delta t_x^2 + \Delta t_h^2 } \right)f^2 } .$$
- With the abbreviation $\Delta t_y = (\Delta t_x^2 + \Delta t_h^2)^{1/2} = 5\, \text{ms}$ one can write for this:
- $$Y(f) = x_0 \cdot \Delta t_x \cdot {\rm{e}}^{ - {\rm{\pi }}\left( {\Delta t_y \hspace{0.05cm}\cdot \hspace{0.05cm} f} \right)^2 } .$$
- At frequency $f = 0$ , the spectral values at the input and output of the Gaussian filter are equal, so:
- $$Y(f = 0) \;\underline{= 4 \text{ mV/Hz}}.$$
- The function curve of ${Y(f)}$ is narrower than ${X(f)}$ and narrower than ${H(f)}$.
(3) The following Fourier correspondence holds:
- $${\rm{e}}^{ - {\rm{\pi }}\left( {\Delta t_y \hspace{0.05cm}\cdot \hspace{0.05cm} f} \right)^2 }\bullet\!\!\!-\!\!\!-\!\!\!-\!\!\circ\, \frac{1}{\Delta t_y } \cdot {\rm{e}}^{ - {\rm{\pi }}\left( {t/\Delta t_y } \right)^2 } .$$
- This gives:
- $$y(t) = x(t) * h(t) = x_0 \cdot \frac{\Delta t_x }{\Delta t_y } \cdot {\rm{e}}^{ - {\rm{\pi }}\left( {t/\Delta t_y } \right)^2 } .$$
- The maximum value of the signal ${y(t)}$ is also at $t = 0$ and is $y_0 \hspace{0.15cm}\underline{= 0.8 \text{ V} }$.
- The equivalent pulse duration results in $\Delta t_y \hspace{0.15cm}\underline{= 5 \text{ ms}}$ (see above graphic, right sketch).
- This means: The Gaussian ${H(f)}$ causes the output pulse ${y(t)}$ to be smaller and wider than the input pulse ${x(t)}$ .
- The pulse shape remains Gaussian. Because: Gaussian convoluted with Gaussian always results in Gaussian!