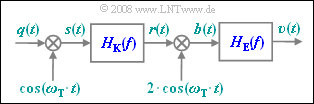
Exercise 2.5Z: Linear Distortions with DSB-AM

• Return to book

Considered system model

As in  Exercise 2.5  here we will also examine:

• the DSB–AM/synchronous demodulator combination,
• considerations involving a linear distorting channel.

Let the source signal  $q(t)$  be a cosine signal with amplitude  $A_{\rm N}$  and frequency  $f_{\rm N}$, such that the spectrum of the modulated signal is as follows:

$$S(f)= \frac{A_{\rm N}}{4} \cdot \big[\delta(f + f_{\rm O}) + \delta(f + f_{\rm U}) + \delta(f - f_{\rm U}) + \delta(f - f_{\rm O}) \big]\hspace{0.05cm}.$$

The abbreviations stand for  

• the upper sideband  (German:  "oberes Seitenband"   ⇒ subscript  "O")   $f_{\rm O} = f_{\rm T} + f_{\rm N}$,  and  
• the lower sideband  (German:  "unteres Seitenband"   ⇒ subscript  "U")  $f_{\rm U} = f_{\rm T} - f_{\rm N}$.

The channel frequency response  (German:  "Kanalfrequenzgang"   ⇒ subscript  "K")  is only given for these two frequencies and is:

$$H_{\rm K}(f_{\rm O}) = R_{\rm O} + {\rm j} \cdot I_{\rm O},\hspace{0.2cm}H_{\rm K}(f_{\rm U}) = R_{\rm U} + {\rm j} \cdot I_{\rm U} \hspace{0.05cm}.$$

For negative frequencies,  $H_{\rm K}(– f) = H_{\rm K}^*(f)$ always holds.

Use the following values for numerical calculations :

$$A_{\rm N} = 2\,{\rm V}, \hspace{0.15cm}f_{\rm N} = 3\,{\rm kHz}, \hspace{0.15cm}f_{\rm T} = 30\,{\rm kHz} \hspace{0.05cm},$$

$$R_{\rm U} = 0.8, \hspace{0.15cm}I_{\rm U} = -0.2, \hspace{0.15cm}R_{\rm O} = 0.4, \hspace{0.15cm}I_{\rm O} = -0.2 \hspace{0.05cm}.$$

In subtask  (3)  the solution should be found from the resulting frequency response of modulator, channel and demodulator:

$$H_{\rm MKD}(f) = {1}/{2} \cdot \big[ H_{\rm K}(f + f_{\rm T}) + H_{\rm K}(f - f_{\rm T})\big]\hspace{0.05cm}.$$

Finally,  in subtask  (4)  the following channel frequency response is considered  (this equationt is only valid for positive frequencies):

$$H_{\rm K}(f) = H_{\rm(4)}(f) = \frac{1}{1 + 3{\rm j} \cdot ({f}/{f_{\rm T}} - 1)}\hspace{0.05cm}.$$

Hints:

• This exercise belongs to the chapter   Synchronous Demodulation.
• Particular reference is made to the page  Influence of linear channel distortions.

Questions

1

Let  $R_{\rm U} = 0.8, \ I_{\rm U} = -0.2, \ R_{\rm O} = 0.4, \ I_{\rm O} = -0.2.$  Calculate and sketch the spectrum  $R(f)$  at the channel output.
What is the spectral line at  $-f_{\rm O}$?

${\rm Re}[R(-f_{\rm O})] \ = \ $ $\ \text{V}$

${\rm Im}[R(-f_{\rm O})] \ = \ $ $\ \text{V}$

2

What is the sink signal  $v(t)$?  Take the synchronous demodulator's low-pass into account during calculation.
What is the signal value when $t = 0$?

$v(t = 0) \ = \ $ $\ \text{V}$

3

Now calculate the sink signal  $v(t)$  over the resulting frequency response  $H_{\rm MKD}(f)$  and evaluate the calculation process.

	The calculation according to subtask (2)  leads to faster success.
	The calculation according to subtask (3)  leads to faster success.

4

Calculate  $v(t)$  for the channel frequency response  $H_{\rm K}(f) = H_{\rm(4)}(f)$.  What is the signal value when  $t = 0$?

$v(t = 0) \ = \ $ $\ \text{V}$

Solution

Solution

Spectrum  $R(f)$  of the received signal

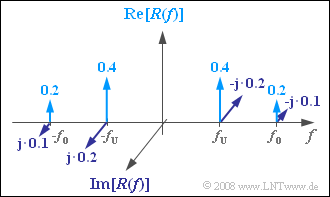
(1)  In general,  $R(f) = S(f) · H_K(f)$.  This gives the line spectrum as shown in the adjacent sketch  (all weights still have to be supplemented by the unit "Volt").

• For the weight of the spectral line at  $f = -f_{\rm O}$  it is valid:

$${\rm Re}[R(-f_{\rm O})]\hspace{0.15cm}\underline{=0.2 \ \rm V},$$

$${\rm Im}[R(-f_{\rm O})]\hspace{0.15cm}\underline{=0.1 \ \rm V}.$$

(2)  The spectral function   $V(f)$  of the sink signal  $v(t)$  is:

$$V(f) = \big[ R(f) \star \left[\delta(f - f_{\rm T}) + \delta(f + f_{\rm T}) \right]\big]\cdot H_{\rm E}(f).$$

• According to the laws of the Fourier transform, this can also be written as:

$$V(f) = \frac{A_{\rm N}}{4} \cdot (R_{\rm O} + {\rm j} \cdot I_{\rm O}) \cdot \delta(f - f_{\rm N}) + \frac{A_{\rm N}}{4} \cdot (R_{\rm U} + {\rm j} \cdot I_{\rm U}) \cdot \delta(f + f_{\rm N})+$$

$$\hspace{2.25cm}+ \frac{A_{\rm N}}{4} \cdot (R_{\rm O} - {\rm j} \cdot I_{\rm O}) \cdot \delta(f + f_{\rm N})+ \frac{A_{\rm N}}{4} \cdot (R_{\rm U} - {\rm j} \cdot I_{\rm U}) \cdot \delta(f - f_{\rm N}) \hspace{0.05cm}.$$

• All other terms are around twice the carrier frequency and are eliminated by the low-pass filter.
• Rearranging and combining the terms results in:

$$V(f) = A_{\rm N}\cdot \frac{R_{\rm U} +R_{\rm O}}{2}\cdot \frac{1}{2} \cdot \left[\delta(f - f_{\rm N}) + \delta(f + f_{\rm N}) \right] + A_{\rm N}\cdot \frac{I_{\rm U} - I_{\rm O}}{2}\cdot \frac{\rm j}{2} \cdot \left[-\delta(f - f_{\rm N}) + \delta(f + f_{\rm N}) \right]$$

$$\Rightarrow \hspace{0.3cm}v(t) = A_{\rm N}\cdot \frac{R_{\rm U} +R_{\rm O}}{2}\cdot\cos (\omega_{\rm N}\cdot t)+ A_{\rm N}\cdot \frac{I_{\rm U} -I_{\rm O}}{2}\cdot\sin (\omega_{\rm N}\cdot t)\hspace{0.05cm}.$$

• When   $R_{\rm U} = 0.8,\ I_{\rm U} = -0.2,\ R_{\rm O} = 0.4,\ I_{\rm O} = -0.2$  it follows:

$$v(t) = 0.6 \cdot A_{\rm N}\cdot \cos (\omega_{\rm N}\cdot t)\hspace{0.3cm}\Rightarrow \hspace{0.3cm} v(t=0) = 0.6 \cdot A_{\rm N}\hspace{0.15cm}\underline {= 1.2\,{\rm V}}\hspace{0.05cm}.$$

• There is attenuation by a factor of   $0.6$ compared to  $q(t)$.
• The synchronous demodulator receives more information about the source signal through the lower sideband than through the upper one.
• Because of the property  $I_{\rm O} = I_{\rm U}$,  $v(t)$  is also cosine-shaped.
• Accordingly,  it is valid either no delay occurs or the delay is an even multiple of the period.

(3)  The following equations apply here:

$$H_{\rm K}(f_{\rm N}+ f_{\rm T}) = R_{\rm O} + {\rm j} \cdot I_{\rm O} \hspace{0.05cm},$$

$$H_{\rm K}(f_{\rm N}- f_{\rm T}) = H_{\rm K}^{\star}(f_{\rm T}- f_{\rm N}) = R_{\rm U} - {\rm j} \cdot I_{\rm U}$$

$$\Rightarrow \hspace{0.2cm} H_{\rm MKD}(f_{\rm N}) = {1}/{2} \cdot \big[(R_{\rm O} +R_{\rm U}) + {\rm j} \cdot (I_{\rm O} -I_{\rm U}) \big]\hspace{0.05cm},\hspace{0.2cm} H_{\rm MKD}(-f_{\rm N}) = H_{\rm MKD}^\star(f_{\rm N}) = {1}/{2} \cdot \big[(R_{\rm O} +R_{\rm U}) - {\rm j} \cdot (I_{\rm O} -I_{\rm U}) \big]\hspace{0.05cm}.$$

• Thus,  one obtains the same result as in  (2),  but faster   ⇒   Answer 2.

(4)  For  $f > 0$  the resulting frequency response is:

$$H_{\rm MKD}(f) = {1}/{2} \cdot \left[ H_{\rm K}(f_{\rm T}+ f) + H_{\rm K}^\star(f_{\rm T}-f)\right]= {1}/{2} \cdot \left[ \frac{1}{1 + 3{\rm j} \cdot (\frac{f_{\rm T}+f}{f_{\rm T}} - 1)} + \frac{1}{1 - 3{\rm j} \cdot (\frac{f_{\rm T}-f}{f_{\rm T}} - 1)}\right]$$

$$\Rightarrow \hspace{0.3cm} H_{\rm MKD}(f) = \frac{1}{1 + {\rm j} \cdot {3f}/{f_{\rm T}} } \hspace{0.05cm}.$$

• Inserted at the point where  $f = f_{\rm N}$  this leads to the result:

$$H_{\rm MKD}(f_{\rm N}) = \frac{1}{1 + {\rm j} \cdot {3f_{\rm N}}/{f_{\rm T}} } \hspace{1.0cm} \Rightarrow \hspace{0.3cm}{\rm magnitude} = \frac{1}{\sqrt{1 + ({3f_{\rm N}}/{f_{\rm T}} )^2}} \hspace{0.05cm}, \hspace{0.3cm} {\rm phase} = {\rm arctan}\hspace{0.1cm}({3f_{\rm N}}/{f_{\rm T}}) \hspace{0.05cm}.$$

• When  $f_{\rm N}/f_{\rm T} = 0.1$  we get the magnitude  $0.958$  and the phase  $16.7^\circ$.  Thus, the sink signal is:

$$v(t) = 0.958 \cdot 2\,{\rm V}\cdot \cos (\omega_{\rm N}\cdot t + 16.7^\circ) \hspace{0.3cm} \Rightarrow \hspace{0.3cm} v(t=0)= 1.916\,{\rm V}\cdot \cos ( 16.7^\circ)\hspace{0.15cm}\underline { = 1.835\,{\rm V}}\hspace{0.05cm}.$$