Exercise 3.1Z: Influence of the Message Phase in Phase Modulation

Two PM signal waveforms

We will now consider the phase modulation of diverse oscillations

$$ q(t) = \cos(\omega_{\rm N} \cdot t + \phi_{\rm N})\hspace{0.05cm}.$$

The source signal is represented here in normalized form with $($amplitude $1)$ , so that the phase-modulated signal can be characterised by the modulation index (or phase deviation) $η$ as follows:

$$s(t) = A_{\rm T} \cdot \cos \hspace{-0.1cm}\big[\omega_{\rm T} \cdot t + \eta \cdot q(t) \big]\hspace{0.05cm}.$$

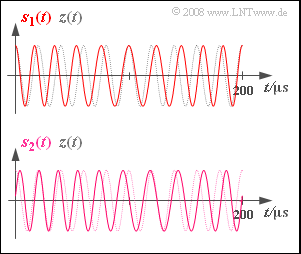
The signal $s_1(t)$ shown in the upper graph is characterized by the parameter values $ϕ_{\rm N} = -90^\circ$ and $η_1 = 2$ .
The frequency $f_{\rm N}$ of this sinusoidal source signal as well as the carrier frequency $f_{\rm T}$ can be determined from the signal section of duration $200 \ \rm µ s$ represented here.

The signal $s_2(t)$ possibly differs from $s_1(t)$ due to a different message phase $ϕ_{\rm N}$ and modulation index $η$. All other system parameters are unchanged from $s_1(t)$ .

Hints:

This exercise belongs to the chapter Phase Modulation.
Particular reference is made to the page Signal characteristics of phase modulation.

Questions

$f_{\rm N} \ = \ $

$\ \rm kHz$

$f_{\rm T} \ = \ $

$\ \rm kHz$

$ϕ_{\rm max} \ = \ $

$\ \rm rad$

$Δt_{\rm max} \ = \ $

$\ \rm µ s$

$η_2 \ = \ $

$ϕ_{\rm N2} \ = \ $

$\ \rm Grad$

Solution

(1) It can be seen from the sketch that the represented section of the signal of duration $200 \ \rm µ s$ corresponds exactly to the period duration of the sinusoidal source signal. From this follows $f_{\rm N}\hspace{0.15cm}\underline{ = 5 \ \rm kHz}$.

At times $t = 0$, $t = 100 \ \rm µ s$ and $t = 200 \ \rm µ s$ , the signals $z(t)$ and $s(t)$ are synchronous in phase.
In the first half-wave of $q(t)$ , the zero crossings of $s(t)$ come slightly earlier than those of the carrier signal $z(t)$ ⇒ positive phase.
In contrast, in the range from $t = 100 \ \rm µ s$ to $t = 200 \ \rm µ s$ , the phase $ϕ(t) < 0$.

(2) $f_{\rm T}\hspace{0.15cm}\underline{ = 50 \ \rm kHz}$, holds,

since exactly $10$ periods can be counted in the shown section of $z(t)$ of duration $200 \ \rm µ s$ .

(3) The maximum relative phase deviation is $ϕ_{\rm max} = η_1/(2π)\hspace{0.15cm}\underline{ ≈ 0.318}$.

(4) Since the period of the carrier is $T_0 = 20 \ \rm µ s$ , we obtain $Δt_{\rm max} = ϕ_{\rm max} ·T_0\hspace{0.15cm}\underline{ ≈ 6.37 \ \rm µ s}$.

(5) The maximum phase deviation (shift in the zero intercepts) is exactly the same for $s_2(t)$ as for $s_1(t)$.

From this, we can conclude that $η_2 = η_1\hspace{0.15cm}\underline{ = 2}$ .

(6) The signal $s_2(t)$ is shifted to the right by $25 \ \rm µ s$ compared to $s_1(t)$ . Therefore, the same must be true for the source signals:

$$ q_2(t) = q_1(t - 25\,{\rm \mu s}) = \cos \hspace{-0.1cm} \big[2 \pi f_{\rm N} (t - 25\,{\rm \mu s}) \big ] = \cos (\omega_{\rm N} \cdot t - 0.75 \cdot \pi)\hspace{0.05cm}.$$

This corresponds to the phase position $ϕ_{\rm N2}\hspace{0.15cm}\underline{ = -135^\circ}$.