Exercise 4.1Z: Appointment to Breakfast
From LNTwww
Ms. M. and Mr. S. are known to meet often for a joint breakfast:
- Both promise to come to such a meeting on a certain day between 8 am and 9 am.
- Further, they agree that each of them will arrive in this period (and only in this period) on "good luck"
and wait up to fifteen minutes for the other.
Hints:
- The exercise belongs to the chapter Two-Dimensional Random Variables.
- Use the minute of arrival time as the time in the following questions:
"Minute = 0" stands for 8 o'clock, "Minute = 60" for 9 o'clock. - The exercise arose before the 2002 German Bundestag elections, when both Dr. Angela Merkel and Dr. Edmund Stoiber wanted to become the CDU/CSU's candidate for chancellor.
- At a joint breakfast in Wolfratshausen, Ms. Merkel renounced. The later election was won by Gerhard Schröder (SPD).
Questions
Solution
(1) If Mr. S. arrives at 8:30, he will meet Ms. M. if she arrives between 8:15 and 8:45. Thus the probability:
- $$p_1 = \text{Pr(Mr. S. meets Ms. M.)}\hspace{0.15cm}\underline{=50\%}.$$
(2) If Ms. M. arrives at 8 a.m., she meets Mr. S. only if he arrives before 8:15.
- If Ms. M. arrives at 9 a.m., Mr. S. must arrive after 8:45 a.m. so that they can meet.
- The probability of meeting is the same in both cases:
- $$p_2 = \big[\text{Min Pr(Mr. S. meets Ms. M.)}\big]\hspace{0.15cm}\underline{=25\%}.$$
(3) Of the two arrival times calculated in (2), 9 o'clock $(\underline{\text{Minute = 60}})$ is more favorable,
since she – if Mr. S. is not there – can leave immediately.
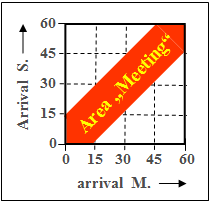
(4) The probability $p_4$ is given as the ratio of the red area in the graph to the total area $1$.
- Using the triangular areas, one obtains:
- $$p_4=\rm 1-2\cdot\frac{1}{2}\cdot\frac{3}{4}\cdot\frac{3}{4}=\frac{7}{16}\hspace{0.15cm}\underline{=\rm 43.75\%}.$$