Exercise 5.7Z: Matched Filter - All Gaussian

Given Gaussian pulse

At the input of a reception filter there is

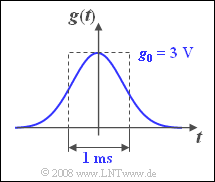
a Gaussian pulse $g(t)$ with amplitude $g_0$ and equivalent duration $\Delta t_g = 1\hspace{0.08cm} \rm ms$,

$$g(t) = g_0 \cdot {\rm{e}}^{ - {\rm{\pi }}\left( {t/\Delta t_g } \right)^2 } ,$$

superimposed by white Gaussian noise with power density $N_0 = 10^{-4}\hspace{0.08cm} \rm V^2 \hspace{-0.1cm}/Hz$.

The pulse energy is $E_g = 0.01\hspace{0.08cm} \rm V^2 s$. Let the reception filter be an acausal Gaussian low-pass filter with frequency response

$$H_{\rm E} (f) = {\rm{e}}^{ - {\rm{\pi }}\left( {f/\Delta f_{\rm E} } \right)^2 } .$$

The associated impulse response is thus:

$$h_{\rm E} (t) = \Delta f_{\rm E} \cdot {\rm{e}}^{ - {\rm{\pi }}\left( {\Delta f_{\rm E} \hspace{0.03cm}\cdot \hspace{0.03cm}t} \right)^2 } .$$

The system-theoretical filter bandwidth $\Delta f_{\rm E}$ should be chosen so that the Gaussian low-pass filter is optimally matched to the input impulse $g(t)$. This is then referred to as a: "matched filter".

Notes:

The exercise belongs to the chapter Matched Filter.
Use the following definite integral to solve:

$$\int_0^\infty {{\rm{e}}^{ - a^2 x^2 } {\rm{d}}x = \frac{{\sqrt {\rm{\pi }} }}{2a}} .$$

Questions

$g_0 \ = \ $

$\ \rm V$

$10 \cdot \lg\ \rho_d(T_\text{D, opt}\hspace{-0.05cm}) \ = \ $

$\ \rm dB$

$\Delta f_\text{E, opt}\ = \ $

$\ \rm kHz$

	The signal component $d_{\rm S}(T_\text{D, opt}\hspace{-0.05cm})$ is smaller than with matching.
	The noise power $\sigma_d^2$ is larger than with matching.
	The S/N ratio is smaller than calculated in subtask (2).

Solution

(1) For the energy of an pulse $g(t)$ applies in general, or for this example:

$$E_g = \int_{ - \infty }^{ + \infty } {g^2(t) \hspace{0.1cm}{\rm{d}}t} = g^2_0 \cdot \int_{ - \infty }^{ + \infty } {{\rm{e}}^{ - 2{\rm{\pi }}\left( {t/\Delta t_g } \right)^2 } \hspace{0.1cm}{\rm{d}}t} .$$

This equation can be transformed as follows:

$$E_g = 2 \cdot g_0 ^2 \cdot \int_0^\infty {{\rm{e}}^{ - \left( {\sqrt {2 \rm{\pi }} /\Delta t_g } \right)^2 \cdot \hspace{0.05cm} t^2 }\hspace{0.1cm} {\rm{d}}t} .$$

With $a = (2\pi)^{1/2}\cdot 1/\Delta t_g$ and the formula given, the following relationship holds:

$$E_g = 2 \cdot g_0 ^2 \cdot \frac{{\sqrt {\rm{\pi }} }}{2a} = \sqrt 2 \cdot g_0 ^2 \cdot \Delta t_g .$$

Solving this equation for $g_0$, the final result is:

$$g_0 = \sqrt {\frac{E_g }{\Delta t_g \cdot \sqrt 2 }} = \sqrt {\frac{{0.01\;{\rm{V}}^{\rm{2}} {\rm{s}}}}{{0.001\;{\rm{s}} \cdot 1.414}}} \hspace{0.15cm}\underline { = 2.659\;{\rm{V}}}.$$

(2) Assuming a matched filter, the S/N ratio at the output is:

$$\rho _{d} ( {T_{{\rm{D,}}\,{\rm{opt}}} } ) = \frac{2 \cdot E_g }{N_0 } = \frac{{2 \cdot 10^{ - 2} \;{\rm{V}}^{\rm{2}} {\rm{s}}}}{{10^{ - 4} \;{\rm{V}}^{\rm{2}} {\rm{/Hz}}}} = 200.$$

In logarithmic representation, the following result is obtained:

$$10 \cdot \lg \rho _{d} ( {T_{{\rm{D,}}\,{\rm{opt}}} } ) = 10 \cdot \lg \left( {200} \right) \hspace{0.15cm}\underline {\approx 23\;{\rm{dB}}}.$$

(3) A comparison between the input pulse and the filter frequency response shows that when $\Delta f_{\rm E} = 1/\Delta t_g$ is fitted, it must hold:

$$\Delta f_{{\rm{E,}}\,{\rm{opt}}} \hspace{0.15cm}\underline { = 1\;{\rm{kHz}}}.$$

(4) Solutions 1 and 3 are correct:

A smaller filter bandwidth is favorable with respect to interference,
but unfavorable with respect to the useful signal.
The negative influence (smaller useful signal) outweighs the positive influence (less noise).