Aufgaben:Exercise 1.3: Calculating with Complex Numbers

• Return to book

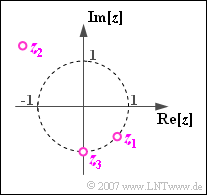
Nebenstehende Grafik zeigt einige Punkte in der komplexen Ebene, nämlich

$$z_1 = {\rm e}^{-{\rm j} 45^{ \circ}}, $$ $$z_2 = 2 \cdot{\rm e}^{{\rm j} 135^{ \circ}},$$ $$z_3 = -{\rm j} .$$

Im Verlauf dieser Aufgabe werden noch folgende komplexe Größen betrachtet: $$z_4 = z_2^2 + z_3^2,$$ $$z_5 = 1/z_2,$$ $$z_6 = \sqrt{z_3},$$ $$z_7 = {\rm e}^{z_2},$$ $$z_8 = {\rm e}^{z_2} + {\rm e}^{z_2^{\star}}.$$

Hinweise:

• Die Aufgabe gehört zum Kapitel Zum_Rechnen_mit_komplexen_Zahlen.
• Die Thematik wird auch im Lernvideo Rechnen mit komplexen Zahlen behandelt.
• Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.

Fragebogen

1

Welche der folgenden Gleichungen sind zutreffend?

	\(2 \cdot z_1 + z_2 =0.\)
	\(z_1^{\ast} \cdot z_2 +2=0.\)
	\((z_1/z_2) \cdot z_3\) ist rein reell.

2

Welchen Wert besitzt die Zufallsgröße \(z_4 = z_2^2 + z_3^2 = x_4 + {\rm j} \cdot y_4\)?

\( x_4 = \)

\( y_4 = \)

3

Berechnen Sie die komplexe Größe \(z_5 = 1/z_2 = x_5 + {\rm j} \cdot y_5\).

\( x_5 = \)

\( y_5 = \)

4

\(z_6\) hat als Quadratwurzel von \(z_3\) zwei Lösungen, beide mit dem Betrag \(|z_6| = 1\). Geben Sie die beiden möglichen Phasenwinkel von \(z_6\) an.

\( \phi_6 ({\rm zwischen\hspace{0.1cm} 0^{\circ} \hspace{0.1cm}und \hspace{0.1cm} 180^{\circ} \hspace{0.1cm}Grad}) \) = $\text{Grad}$

\( \phi_6 ({\rm zwischen\hspace{0.1cm} - \hspace{-0.15cm}180^{\circ} \hspace{0.1cm}und \hspace{0.1cm} 0^{\circ} \hspace{0.1cm}Grad}) \) = $\text{Grad}$

5

Berechnen Sie \(z_7 = {\rm e}^{z_2} = x_7 + {\rm j} \cdot y_7\).

\( x_7 = \)

\( y_7 = \)

6

Geben Sie die komplexe Größe \(z_8 = {\rm e}^{z_2} + {\rm e}^{z_2^{\ast}} = x_8 + {\rm j}\cdot y_8\).

\( x_8 = \)

\( y_8 = \)

Musterlösung

Solution

1. Entsprechend den Angaben gilt mit dem Signaldarstellung/Zum Rechnen mit komplexen Zahlen: 

\(2 \cdot z_1 + z_2 = 2 \cdot \cos(45^{ \circ}) - 2{\rm j}\cdot \sin(45^{ \circ})- 2 \cdot \cos(45^{ \circ}) + 2{\rm j} \cdot\sin(45^{ \circ}) = 0.\)

Der zweite Vorschlag ist ebenfalls richtig, da

\(z_1^{\star} \cdot z_2 = 1 \cdot{\rm e}^{{\rm j} 45^{ \circ}} \cdot 2 \cdot{\rm e}^{{\rm j} 135^{ \circ}} = 2 \cdot{\rm e}^{{\rm j} 180^{ \circ}}= -2.\)

Dagegen ist der dritte Vorschlag falsch. Die Division von \(z_1\) und \(z_2\) liefert: 

\(\frac{z_1}{z_2} = \frac{{\rm e}^{-{\rm j} 45^{ \circ}}}{2 \cdot{\rm e}^{{\rm j} 135^{ \circ}}} = 0.5 \cdot{\rm e}^{-{\rm j} 180^{ \circ}}= -0.5.\)

Die Multiplikation mit \(z_3 = -{\rm j} \) führt zum Ergebnis j/2, also zu einer rein imaginären Größe. Richtig sind also die Lösungsvorschläge 1 und 2.

2. Das Quadrat von \(z_2\) hat den Betrag \(|z_2|^{2}\) und die Phase \(2 \cdot \phi_2\): 

\(z_2^2 = 2^2 \cdot{\rm e}^{{\rm j} 270^{ \circ}}= 4 \cdot {\rm e}^{-{\rm j} 90^{ \circ}}=-4 \cdot {\rm j}.\)

Entsprechend gilt für das Quadrat von \(z_3\): 

\(z_3^2 = (-{\rm j})^2 = -1.\) Somit ist \(x_4 = –1\) und \(y_4 = –4.\)

3. Durch Anwendung der Divisionsregel erhält man: 

\(z_5 = \frac{1}{z_2} = \frac{1}{2 \cdot e^{j135^{\circ}}} = 0.5 \cdot e^{-j135^{\circ}} = 0.5 \cdot (cos(-135^{\circ}) + j \cdot sin(-135^{\circ}))\) \(\Rightarrow x_5 = y_5 = - \frac{\sqrt{2}}{4}= -0.354\)

4. Die angegeben Beziehung für \(z_6\) kann wie folgt umgeformt werden: 

\(z_6^2 = z_3 = e^{-90^{\circ}}\)

Man erkennt, dass es zwei Möglichkeiten für \(z_6\) gibt, die diese Gleichung erfüllen: 

\(z_6(1.Loesung) = \frac{z_2}{2}= 1 \cdot e^{j135^{\circ}} \Rightarrow \phi_6 = 135^{\circ}\)

\(z_6(2.Loesung) = z_1= 1 \cdot e^{-j45^{\circ}} \Rightarrow \phi_6 = -45^{\circ}\)

5. Die komplexe Größe \(z_2\) lautet in Realteil/imaginärteildarstellung: 

\(z_2 = x_2 + j \cdot y_2 = -\sqrt{2} + j \cdot \sqrt{2}\)

Damit ergibt sich für die komplexe Exponentialfunktion\[z_7 = e^{-\sqrt{2}+j \cdot \sqrt{2}} = e^{-\sqrt{2}} \cdot (cos(\sqrt{2} + j \cdot sin(\sqrt{2})\]

Mit

\(e^{-\sqrt{2}} = 0.243, \quad cos(\sqrt{2}) = 0.156, \quad sin(\sqrt{2}) = 0.988\)

erhält man somit: 

\(z_7 = 0.243 \cdot (0.156 + j \cdot 0.988) = 0.038 + j \cdot 0.24\)

6. Ausgehend vom Ergebnis 4. erhält man für \(z_8\): 

\(z_8 = e^{-\sqrt{2}} \cdot (cos(\sqrt{2}) + j \cdot sin(\sqrt{2}) + cos(\sqrt{2}) - j \cdot (\sqrt{2}))\)

\(2 \cdot e^{-\sqrt{2}} \cdot cos(\sqrt{2}) = 2 \cdot x_7\)

\(\Rightarrow x_8 = 0.076, \quad y_8 =0\)