Aufgaben:Exercise 5.7Z: Application of the IDFT

• Return to book

Drei Sätze „A”, „B” und „C” für die Spektralkoeffizienten

Bei der Diskreten Fouriertransformation (DFT) werden aus den Zeitabtastwerten $d(ν)$ mit der Laufvariablen $ν = 0$, ... , $N – 1$ die diskreten Spektralkoeffizienten $D(μ)$ mit $μ = 0$, ... , $N – 1$ wie folgt berechnet:

$$D(\mu) = \frac{1}{N} \cdot \sum_{\nu = 0 }^{N-1} d(\nu)\cdot {w}^{\hspace{0.05cm}\nu \hspace{0.03cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$

Hierbei ist mit $w$ der komplexe Drehfaktor abgekürzt, der wie folgt definiert ist:

$$w = {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2 \pi /N} = \cos \left( {2 \pi}/{N}\right)-{\rm j} \cdot \sin \left( {2 \pi}/{N}\right) \hspace{0.05cm}.$$

Entsprechend gilt für die Inverse Diskrete Fouriertransformation (IDFT) als Umkehrfunktion der DFT:

$$d(\nu) = \sum_{\mu = 0 }^{N-1} D(\mu) \cdot {w}^{-\nu \hspace{0.03cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$

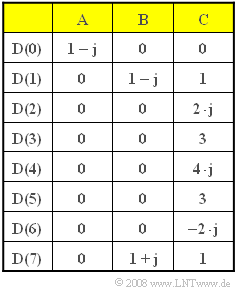
In dieser Aufgabe sollen für verschiedene komplexwertige Beispielfolgen $D(μ)$ – die in der Tabelle mit „A”, „B” und „C” bezeichnet sind – die Zeitkoeffizienten $d(ν)$ ermittelt werden. Es gilt somit stets $N = 8$.

Hinweise:

• Die Aufgabe gehört zum Kapitel Realisierung von OFDM-Systemen.
• Bezug genommen wird auch auf das Kapitel Diskrete Fouriertransformation im Buch „Signaldarstellung”.
• Sie können Ihre Ergebnisse mit dem Interaktionsmodul Diskrete Fouriertransformation kontrollieren.
• Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.

Fragebogen

1

Wie lauten die Zeitkoeffizienten $d(ν)$ für die Spektralkoeffizienten $D(μ)$ gemäß Spalte A?

${\rm Re}[d(1)] \ = \ $

${\rm Im}[d(1)] \ = \ $

2

Wie lauten die Zeitkoeffizienten $d(ν)$ für die Spektralkoeffizienten $D(μ)$ gemäß Spalte B?

${\rm Re}[d(1)] \ = \ $

${\rm Im}[d(1)] \ = \ $

3

Wie lauten die Zeitkoeffizienten $d(ν)$ für die Spektralkoeffizienten $D(μ)$ gemäß Spalte C?

${\rm Re}[d(1)] \ = \ $

${\rm Im}[d(1)] \ = \ $

Musterlösung

Solution

(1)  Wegen $D(μ) = 0$ für $μ ≠ 0$ sind alle Zeitkoeffizienten $d(ν) = D(0)$. Damit gilt auch:

$${\rm Re}[d(1)] \hspace{0.15cm}\underline {=+ 1}, \hspace{0.3cm}{\rm Im}[d(1)] \hspace{0.15cm}\underline {= -1}.$$

(2)  Hier sind alle Spektralkoeffizienten Null mit Ausnahme von $D_1 = 1 –{\rm j}$ und $D_7 = 1 + {\rm j}$. Daraus folgt für alle Zeitkoeffizienten ($0 ≤ ν ≤ 7$):

$$d(\nu) = (1 - {\rm j}) \cdot {\rm{e}}^{ - {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu} +(1 + {\rm j}) \cdot {\rm{e}}^{ - {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {7\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu}.$$

Aufgrund der Periodizität gilt aber auch:

$$d(\nu) = (1 - {\rm j}) \cdot {\rm{e}}^{ - {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu} +(1 + {\rm j}) \cdot {\rm{e}}^{ +{\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu}= \left[ {\rm{e}}^{ + {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu} + {\rm{e}}^{ - {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu}\right]+ {\rm{j}} \cdot\left[ {\rm{e}}^{ + {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu} - {\rm{e}}^{ - {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\nu}\right].$$

Mit dem Satz von Euler lässt sich dieser Ausdruck wie folgt umformen:

$$d(\nu) = 2 \cdot \cos \left( {\pi}/{4}\cdot \nu \right)+ 2 \cdot \sin \left( {\pi}/{4}\cdot \nu \right).$$

Diese Zeitfunktion $d(ν)$ ist rein reell und kennzeichnet eine harmonische Schwingung mit der Amplitude $ 2 \cdot \sqrt{2}$ und der Phase $φ = 45^\circ$. Der Zeitkoeffizient mit Index $ν = 1$ gibt das Maximum an:

$$ {\rm Re}[d(1)] = 2 \cdot \frac {\sqrt{2}}{2}+ 2 \cdot \frac {\sqrt{2}}{2} = 2 \cdot {\sqrt{2}} \hspace{0.15cm}\underline {\approx 2.828}, \hspace{0.5cm}{\rm Im}[d(1)] \hspace{0.15cm}\underline {= 0}.$$

(3)  Entsprechend der allgemeinen Gleichung gilt: $$d(1) = \sum\limits_{\mu = 0}^{7} D(\mu)\cdot {\rm{e}}^{ - {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}/4\hspace{0.04cm}\cdot \hspace{0.04cm}\mu} =$$ $$ = \left[ D(1) + D(7) \right]\cdot \cos \left( {\pi}/{4} \right) + \left[ D(3) + D(5) \right]\cdot \cos \left( {3\pi}/{4} \right)+$$ $$ + {\rm j} \cdot \left[ D(2) - D(6) \right]\cdot \sin \left( {\pi}/{2} \right) + D(4) \cdot {\rm{e}}^{ - {\rm{j}}\hspace{0.04cm}\cdot \hspace{0.04cm} {\rm{\pi}}}.$$ Die ersten drei Terme liefern rein reelle Ergebnisse: $${\rm Re}\{d(1)\} = (1+1) \cdot \frac{1}{\sqrt{2}}-(3+3) \cdot \frac{1}{\sqrt{2}}+ {\rm j} \cdot4{\rm j} \cdot 1 =$$ $$ = -\frac{4}{\sqrt{2}}-4\hspace{0.15cm}\underline { \approx -6.829}.$$ Für den Imaginärteil ergibt sich: $${\rm Im}\{d(1)\} = {\rm Im}\left\{4 \cdot{\rm j} \cdot (-1) \right\} \hspace{0.15cm}\underline {= -4}.$$