Aufgaben:Exercise 1.4: Maximum Likelihood Decision

• Return to book

Zur Maximum–Likelihood–Decodierung

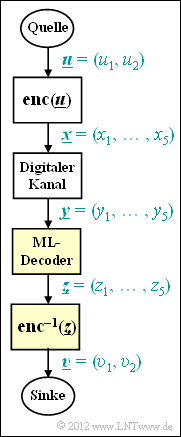
Wir betrachten das digitale Übertragungssystem entsprechend der Grafik. Berücksichtigt sind dabei:

• ein systematischer (5, 2)–Blockcode C mit den Codeworten

$$\underline{x}_{0} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (0, 0, 0, 0, 0) \hspace{0.05cm},$$ $$\underline{x}_{1} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (0, 1, 0, 1, 0) \hspace{0.05cm},$$ $$\underline{x}_{2} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (1, 0, 1, 0, 1) \hspace{0.05cm},$$ $$\underline{x}_{3} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} (1, 1, 1, 1, 1) \hspace{0.05cm},$$

• ein digitales (binäres) Kanalmodell, das den Vektor x ∈ GF($2^{5}$) in den Vektor $\underline{y} \in {\rm GF} (2^{5}$) verfälscht,
• ein Maximum–Likelihood–Decoder mit der Entscheidungsregel

$$\underline{z} = {\rm arg} \max_{\underline{x}_{\hspace{0.03cm}i} \hspace{0.05cm} \in \hspace{0.05cm} \mathcal{C}} \hspace{0.1cm} {\rm Pr}( \underline{x}_{\hspace{0.03cm}i} \hspace{0.05cm}|\hspace{0.05cm} \underline{y} ) = {\rm arg} \min_{\underline{x}_{\hspace{0.03cm}i} \hspace{0.05cm} \in \hspace{0.05cm} \mathcal{C}} \hspace{0.1cm} d_{\rm H}(\underline{y} \hspace{0.05cm}, \hspace{0.1cm}\underline{x}_{\hspace{0.03cm}i}).$$

Fragebogen

1

Multiple-Choice Frage

	Falsch
	Richtig

2

Input-Box Frage

$\alpha$ =

Musterlösung

Solution

1. 2. 3. 4. 5. 6. 7.