Difference between revisions of "Aufgaben:Exercise 1.08Z: Equivalent Codes"
From LNTwww
(Die Seite wurde neu angelegt: „{{quiz-Header|Buchseite=Kanalcodierung/Allgemeine Beschreibung linearer Blockcodes }} [[File:|right|]] ===Fragebogen=== <quiz display=simple> {Multiple-C…“) |
|||
| Line 3: | Line 3: | ||
}} | }} | ||
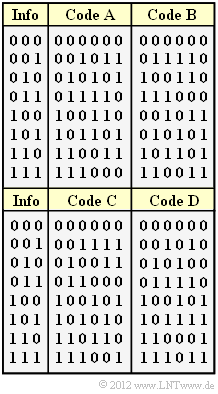
| − | [[File:|right|]] | + | [[File:P_ID2394__KC_Z_1_8.png|right|frame|Vier verschiedene (6, 3)–Blockcodes]] |
| + | In der Grafik sind die Zuordnungen $\underline{u} → \underline{x}$ für verschiedene Codes angegeben, die im Folgenden jeweils durch die Generatormatrix '''G''' und die Prüfmatrix '''H''' charakterisiert werden: | ||
| + | |||
| + | *$\color{red}{\boldsymbol{\rm Code \ A}}$: | ||
| + | :$${ \boldsymbol{\rm G}}_{\rm A} = \begin{pmatrix} 1 &0 &0 &1 &1 &0\\ 0 &1 &0 &1 &0 &1\\ 0 &0 &1 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$ | ||
| + | :$${ \boldsymbol{\rm H}}_{\rm A} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 1 &0 &1 &0 &1 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| + | |||
| + | *$\color{red}{\boldsymbol{\rm Code \ B}}$: | ||
| + | :$${ \boldsymbol{\rm G}}_{\rm B} = \begin{pmatrix} 0 &0 &1 &0 &1 &1\\ 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0 \end{pmatrix} \hspace{0.05cm},$$ | ||
| + | :$$ { \boldsymbol{\rm H}}_{\rm B} = \begin{pmatrix} 1 &0 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| + | |||
| + | *$\color{red}{\boldsymbol{\rm Code \ C}}$: | ||
| + | :$${ \boldsymbol{\rm G}}_{\rm C} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &0 &1 &1\\ 0 &0 &1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},{ \boldsymbol{\rm H}}_{\rm C} = \begin{pmatrix} 1 &0 &1 &1 &0 &0\\ 0 &1 &1 &0 &1 &0\\ 1 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm},$$ | ||
| + | |||
| + | *$\color{red}{\boldsymbol{\rm Code \ D}}$: | ||
| + | :$${ \boldsymbol{\rm G}}_{\rm D} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0 \end{pmatrix} \hspace{0.05cm},{ \boldsymbol{\rm H}}_{\rm D} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0\\ 1 &0 &0 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| + | |||
| + | In dieser Aufgabe soll untersucht werden, welche dieser Codes bzw. Codepaare | ||
| + | |||
| + | *systematisch sind, | ||
| + | *identisch sind (das heißt: Verschiedene Codes haben gleiche Codeworte), | ||
| + | *äquivalent sind (das heißt: Verschiedene Codes haben gleiche Codeparameter). | ||
| + | |||
| + | |||
| + | ''Hinweis'' : | ||
| + | |||
| + | Die Aufgabe gehört zum Themengebiet von Kapitel [[Kanalcodierung/Allgemeine_Beschreibung_linearer_Blockcodes|Allgemeine Beschreibung linearer | ||
| + | Blockcodes]] Anzumerken ist, dass die Angabe einer Prüfmatrix '''H''' nicht eindeutig ist. Verändert man die Reihenfolge der Prüfgleichungen, so entspricht dies einer Vertauschung von Zeilen. | ||
===Fragebogen=== | ===Fragebogen=== | ||
Revision as of 14:08, 2 December 2017
In der Grafik sind die Zuordnungen $\underline{u} → \underline{x}$ für verschiedene Codes angegeben, die im Folgenden jeweils durch die Generatormatrix G und die Prüfmatrix H charakterisiert werden:
- $\color{red}{\boldsymbol{\rm Code \ A}}$:
- $${ \boldsymbol{\rm G}}_{\rm A} = \begin{pmatrix} 1 &0 &0 &1 &1 &0\\ 0 &1 &0 &1 &0 &1\\ 0 &0 &1 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},$$
- $${ \boldsymbol{\rm H}}_{\rm A} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 1 &0 &1 &0 &1 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
- $\color{red}{\boldsymbol{\rm Code \ B}}$:
- $${ \boldsymbol{\rm G}}_{\rm B} = \begin{pmatrix} 0 &0 &1 &0 &1 &1\\ 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0 \end{pmatrix} \hspace{0.05cm},$$
- $$ { \boldsymbol{\rm H}}_{\rm B} = \begin{pmatrix} 1 &0 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
- $\color{red}{\boldsymbol{\rm Code \ C}}$:
- $${ \boldsymbol{\rm G}}_{\rm C} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &0 &1 &1\\ 0 &0 &1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},{ \boldsymbol{\rm H}}_{\rm C} = \begin{pmatrix} 1 &0 &1 &1 &0 &0\\ 0 &1 &1 &0 &1 &0\\ 1 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm},$$
- $\color{red}{\boldsymbol{\rm Code \ D}}$:
- $${ \boldsymbol{\rm G}}_{\rm D} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0 \end{pmatrix} \hspace{0.05cm},{ \boldsymbol{\rm H}}_{\rm D} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0\\ 1 &0 &0 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
In dieser Aufgabe soll untersucht werden, welche dieser Codes bzw. Codepaare
- systematisch sind,
- identisch sind (das heißt: Verschiedene Codes haben gleiche Codeworte),
- äquivalent sind (das heißt: Verschiedene Codes haben gleiche Codeparameter).
Hinweis :
Die Aufgabe gehört zum Themengebiet von Kapitel Allgemeine Beschreibung linearer Blockcodes Anzumerken ist, dass die Angabe einer Prüfmatrix H nicht eindeutig ist. Verändert man die Reihenfolge der Prüfgleichungen, so entspricht dies einer Vertauschung von Zeilen.
Fragebogen
Musterlösung
1.
2.
3.
4.
5.
6.
7.