Difference between revisions of "Aufgaben:Exercise 1.2: Signal Classification"
From LNTwww
| Line 3: | Line 3: | ||
[[File:P_ID341_Sig_A_1_2.png|right|frame|predetermined characteristics]] | [[File:P_ID341_Sig_A_1_2.png|right|frame|predetermined characteristics]] | ||
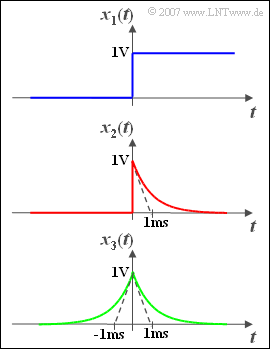
Three signal curves are shown on the Right: | Three signal curves are shown on the Right: | ||
| − | *The blue signal <math>x_1(t)</math> is switched on at time $t = 0$ and has | + | *The blue signal <math>x_1(t)</math> is switched on at time $t = 0$ and has at $t > 0$ the value $1\,\text{V}$. |
*The blue signal <math>x_2(t)</math> is for $t < 0$ equals zero, jumps at $t = 0$ to $1\,\text{V}$ and then falls down with the time constant $1\,\text{ms}$ . For $t > 0$ the following applies: | *The blue signal <math>x_2(t)</math> is for $t < 0$ equals zero, jumps at $t = 0$ to $1\,\text{V}$ and then falls down with the time constant $1\,\text{ms}$ . For $t > 0$ the following applies: | ||
Revision as of 21:40, 11 August 2020
Three signal curves are shown on the Right:
- The blue signal \(x_1(t)\) is switched on at time $t = 0$ and has at $t > 0$ the value $1\,\text{V}$.
- The blue signal \(x_2(t)\) is for $t < 0$ equals zero, jumps at $t = 0$ to $1\,\text{V}$ and then falls down with the time constant $1\,\text{ms}$ . For $t > 0$ the following applies:
- \[x_2(t) = 1\,\text{V} \cdot {\rm e}^{- {t}/(1\,\text{ms})}.\]
- Correspondingly, the signal shown in green applies to all times $t$:
- \[x_3(t) = 1\,\text{V} \cdot {\rm e}^{- {|\hspace{0.05cm}t\hspace{0.05cm}|}/(1\,\text{ms})}.\]
You will now classify these three signals according to the following criteria:
- deterministic or stochastic,
- causal or acausal,
- energy limited or power limited,
- value-continuous or value-discrete,
- time-continuous or time-discrete.
Notes:
- This exercise belongs to the chapter Klassifizierung von Signalen.
Questions
Solutions
(1) The solutions 1 and 3 are applicable:
- Alle Signale können in analytischer Form vollständig beschrieben werden; sie sind deshalb auch deterministisch.
- Alle Signale sind außerdem für alle Zeiten $t$ eindeutig definiert, nicht nur zu gewissen Zeitpunkten. Deshalb handelt es sich stets um zeitkontinuierliche Signale.
- Die Signalamplituden von \(x_2(t)\) und \(x_3(t)\) können alle beliebigen Werte zwischen $0$ und $1\,\text{V}$ annehmen; sie sind deshalb wertkontinuierlich.
- Dagegen sind beim Signal \(x_1(t)\) nur die zwei Signalwerte $0$ und $1\,\text{V}$ möglich; es liegt ein wertdiskretes Signal vor.
(2) Richtig sind die Lösungsvorschläge 1 und 2:
- Ein Signal bezeichnet man als kausal, wenn es für Zeiten $t < 0$ nicht existiert bzw. identisch Null ist. Dies gilt für die Signale \(x_1(t)\) und \(x_2(t)\).
- Dagegen gehört \(x_3(t)\) zur Klasse der akausalen Signale.
(3) Nach der allgemeinen Definition gilt:
- \[E_2=\lim_{T_{\rm M}\to\infty}\int^{T_{\rm M}/2}_{-T_{\rm M}/2}x^2_2(t)\,\hspace{0.1cm}{\rm d}t.\]
Im vorliegenden Fall ist die untere Integrationsgrenze Null und die obere Integrationsgrenze $+\infty$. Man erhält:
- \[E_2=\int^\infty_0 (1{\rm V})^2\cdot{\rm e}^{-2t/(1\rm ms)}\,\hspace{0.1cm}{\rm d}t = 5 \cdot 10^{-4}\hspace{0.1cm} \rm V^2s \hspace{0.15cm}\underline{= 0.5 \cdot 10^{-3}\hspace{0.1cm} \rm V^2s}. \]
Bei endlicher Energie ist die zugehörige Leistung stets verschwindend klein. Daraus folgt $P_2\hspace{0.15cm}\underline{ = 0}$.
(4) Richtig sind die Lösungsvorschläge 2 und 3:
- Wie bereits in der letzten Teilaufgabe berechnet wurde, besitzt \(x_2(t)\) eine endliche Energie:
- $$E_2= 0.5 \cdot 10^{-3}\hspace{0.1cm} {\rm V^2s}. $$
- Die Energie des Signals \(x_3(t)\) ist doppelt so groß, da nun der Zeitbereich $t < 0$ den gleichen Beitrag liefert wie der Zeitbereich $t > 0$. Also ist
- $$E_3= 10^{-3}\hspace{0.1cm} {\rm V^2s}.$$
- Beim Signal \(x_1(t)\) divergiert das Energieintegral: $E_1 \rightarrow \infty$. Dieses Signal weist eine endliche Leistung auf ⇒ $P_1= 0.5 \hspace{0.1cm} {\rm V}^2$.
- Das Ergebnis berücksichtigt auch, dass das Signal \(x_1(t)\) in der Hälfte der Zeit $(t < 0)$ identisch Null ist.
- Das Signal \(x_1(t)\) ist dementsprechend leistungsbegrenzt.