Difference between revisions of "Signal Representation/Fourier Series"
| Line 100: | Line 100: | ||
:A1=2T0⋅∫T000.4⋅cos(ω0t)dt+2T0⋅∫T000.6⋅cos2(ω0t)dt−2T0⋅∫T000.3⋅sin(3ω0t)⋅cos(ω0t)dt. | :A1=2T0⋅∫T000.4⋅cos(ω0t)dt+2T0⋅∫T000.6⋅cos2(ω0t)dt−2T0⋅∫T000.3⋅sin(3ω0t)⋅cos(ω0t)dt. | ||
| − | + | The last integral is equal to zero due to orthogonality; the first one is zero too (integral over one period). | |
| − | * | + | *Only the middle term contributes here to A1, namely $2 - 0.6 - 0.5 = 0.6. $ |
| − | * | + | *For all further (n≥2) cosine coefficients all three integrals return the value zero, and thus An≠1=0. |
| − | + | To determine the sine coefficients Bn with the determining equation: | |
:Bn=2T0⋅∫T000.4⋅sin(n ω0t)dt+2T0⋅∫T000.6⋅cos(ω0t)sin(nω0t)dt−2T0⋅∫T000.3⋅sin(3ω0t)sin(nω0t)dt. | :Bn=2T0⋅∫T000.4⋅sin(n ω0t)dt+2T0⋅∫T000.6⋅cos(ω0t)sin(nω0t)dt−2T0⋅∫T000.3⋅sin(3ω0t)sin(nω0t)dt. | ||
| − | * | + | *For n≠3 all three integral values are zero and therefore Bn≠3=0. |
| − | * | + | *On the other hand, for n=3 the last integral provides a contribution, and one gets for the sine coefficient B3=−0.3.}} |
==Exploitation of Symmetries== | ==Exploitation of Symmetries== | ||
<br> | <br> | ||
| − | + | Some insights into the Fourier coefficients An and Bn can already be read from the ''Symmetry properties'' of the time function x(t) . | |
| − | * | + | *If the time signal x(t) is an even function ⇒ axis-symmetrical around the ordinate (t=0), all sine coefficients Bn disappear, since the sine function itself is an odd function ⇒ sin(−α)=−sin(α): |
:Bn=0(n=1, 2, 3,...). | :Bn=0(n=1, 2, 3,...). | ||
| − | * | + | *An odd function x(t) is point symmetric around the coordinate origin (t=0; x=0). Therefore, all cosine coefficients (An=0) disappear here, since the cosine function itself is even. In this case, the constant component A0 is also always zero. |
:An=0(n=0, 1, 2, 3,...). | :An=0(n=0, 1, 2, 3,...). | ||
| − | * | + | *If a function without a constant component is present (A0=0) and if this function is odd within a period ⇒ it is x(t)=−x(t−T0/2), then only odd multiples of the fundamental frequency are present in the Fourier series representation. For the coefficients with an even index, however, the following always applies: |
:An=Bn=0(n=2, 4, 6,...). | :An=Bn=0(n=2, 4, 6,...). | ||
| − | * | + | *If all coefficients An and Bn with even-numbered index (n=2, 4, 6,...) equals zero and the coefficient A0≠0, then the symmetry property mentioned in the last point refers to the DC component and applies: |
:x(t)=2⋅A0−x(t−T0/2). | :x(t)=2⋅A0−x(t−T0/2). | ||
| − | '' | + | ''Remark'': Several of the named symmetry properties can be fulfilled at the same time. |
| − | + | The symmetry properties of the Fourier coefficients are explained in the first part of the german learning video | |
| − | [[Eigenschaften_der_Fourierreihendarstellung_(Lernvideo)| | + | [[Eigenschaften_der_Fourierreihendarstellung_(Lernvideo)|Properties and Accuracy of the fourier series]] . |
| − | [[File:EN_Sig_T_2_4_S3.png|right|frame| | + | [[File:EN_Sig_T_2_4_S3.png|right|frame|Symmetry Properties of the Fourier Coefficients]] |
{{GraueBox|TEXT= | {{GraueBox|TEXT= | ||
| − | $\text{ | + | $\text{Example 3:}$ |
| − | + | The above mentioned properties are now illustrated by three signal characteristics. | |
| − | *x1(t) | + | *x1(t) is an averaged function ⇒ A0≠0 and is also even, which is accordingly exclusively determined by cosine coefficients An (Bn=0). |
| − | * | + | *In contrast, the odd function x2(t) all An, (n≥0) are identical to zero. |
| − | * | + | *Also the odd function x3(t) contains only sine coefficients, but because of x3(t)=−x3(t−T0/2) exclusively for odd values of n.}} |
==Complex Fourier Series== | ==Complex Fourier Series== | ||
<br> | <br> | ||
| − | + | As shown on the page [[ Signal_Representation/Harmonic_Oscillation#Darstellung_mit_Cosinus-_und_Sinusanteil|3 Representation with Cosine and Sine Components]] in case of a harmonic oscillation any periodic signal | |
| + | |||
:x(t)=A0+∞∑n=1An⋅cos(nω0t)+∞∑n=1Bn⋅sin(nω0t) | :x(t)=A0+∞∑n=1An⋅cos(nω0t)+∞∑n=1Bn⋅sin(nω0t) | ||
| − | + | can also be displayed using the magnitude and phase coefficients: | |
:x(t)=C0+∞∑n=1Cn⋅cos(nω0t−φn). | :x(t)=C0+∞∑n=1Cn⋅cos(nω0t−φn). | ||
| − | + | These modified Fourier coefficients have the following properties: | |
| − | * | + | *The '''DC coefficient''' C0 is identical with A0. |
| − | * | + | *The '''magnitude coefficient''' read with n≥1: Cn=√A2n+B2n. |
| − | * | + | *For the '''phase coefficien''' applies: φn=arctan(Bn/An). |
| − | + | With the „Eulerian relationship” cos(x)+j⋅sin(x)=ejx we get a second representation variant of the Fourier series evolution, which starts from the complex exponential function. | |
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
Definition: | Definition: | ||
| − | + | The '''complex Fourier series''' of a periodic signal x(t) is as follows | |
:x(t)=+∞∑n=−∞Dn⋅ejnω0t. | :x(t)=+∞∑n=−∞Dn⋅ejnω0t. | ||
| − | + | Here Dn denote the '''complex Fourier coefficients''', which | |
| − | * | + | *from the cosine coefficients An and the sine coefficients Bn, or |
| − | * | + | *from the magnitude coefficients Cn and the phase coefficients φn |
| − | + | can be calculated as follows (valid for n≠0): | |
:Dn=1/2⋅(An−j⋅Bn)=1/2⋅Cn⋅e−jφn}} | :Dn=1/2⋅(An−j⋅Bn)=1/2⋅Cn⋅e−jφn}} | ||
| − | + | The complex Fourier coefficients can also be calculated directly using the following equation | |
:Dn=1T0⋅∫+T0/2−T0/2x(t)⋅e−jnω0tdt. | :Dn=1T0⋅∫+T0/2−T0/2x(t)⋅e−jnω0tdt. | ||
| − | + | As long as the integration interval T0 is preserved, it can be shifted randomly as with the coefficients An and Bn for example from t=0 to t=T0. | |
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
| − | Conclusion: | + | Conclusion: The coefficient D0=A0 is always real. For the complex coefficients with negative iterating index (n<0) applies: |
:D−n=D⋆n=1/2⋅(An+j⋅Bn).}} | :D−n=D⋆n=1/2⋅(An+j⋅Bn).}} | ||
| Line 199: | Line 200: | ||
==Periodic Signal Spectrum== | ==Periodic Signal Spectrum== | ||
<br> | <br> | ||
| − | + | Starting from the complex Fourier series just derived | |
:x(t)=+∞∑n=−∞Dn⋅ejnω0t | :x(t)=+∞∑n=−∞Dn⋅ejnω0t | ||
| − | + | and the [[Signal_Representation/Fourier_Transform_Laws#Verschiebungssatz|Displacement Theorem]] (for the frequency domain) one gets the following spectrum for the periodic signal x(t): | |
:X(f)=+∞∑n=−∞Dn⋅δ(f−n⋅f0). | :X(f)=+∞∑n=−∞Dn⋅δ(f−n⋅f0). | ||
| − | + | This means: | |
*Das Spektrum eines mit T0 periodischen Signals ist ein '''Linienspektrum''' bei ganzzahligen Vielfachen der Grundfrequenz f0=1/T0. | *Das Spektrum eines mit T0 periodischen Signals ist ein '''Linienspektrum''' bei ganzzahligen Vielfachen der Grundfrequenz f0=1/T0. | ||
*Der '''Gleichanteil''' liefert eine Diracfunktion bei f=0 mit dem Impulsgewicht A0. | *Der '''Gleichanteil''' liefert eine Diracfunktion bei f=0 mit dem Impulsgewicht A0. | ||
| Line 217: | Line 218: | ||
{{GraueBox|TEXT= | {{GraueBox|TEXT= | ||
| − | $\text{ | + | $\text{Example 4:}$ |
Wir betrachten – wie im [[Signal_Representation/Fourier_Series#Allgemeine_Beschreibung|Beispiel 1]] zu Beginn dieses Abschnitts - zwei periodische Rechtecksignale, jeweils mit Periodendauer T0 und Grundfrequenz f0=1/T0. | Wir betrachten – wie im [[Signal_Representation/Fourier_Series#Allgemeine_Beschreibung|Beispiel 1]] zu Beginn dieses Abschnitts - zwei periodische Rechtecksignale, jeweils mit Periodendauer T0 und Grundfrequenz f0=1/T0. | ||
Das obere Signal | Das obere Signal | ||
| Line 231: | Line 232: | ||
| − | [[File:P_ID528__Sig_T_2_4_S6_neu.png|center|frame| | + | [[File:P_ID528__Sig_T_2_4_S6_neu.png|center|frame|Spectrum of a periodic rectangle pulse]] |
| − | + | The function xu(t) shown below is odd: | |
:xu(t)=4/π⋅[sin(ω0t)+1/3⋅sin(3ω0t)+1/5⋅sin(5ω0t)+...]. | :xu(t)=4/π⋅[sin(ω0t)+1/3⋅sin(3ω0t)+1/5⋅sin(5ω0t)+...]. | ||
| Line 264: | Line 265: | ||
| − | [[File:P_ID2720__Sig_T_2_4_S7_neu.png|center|frame| | + | [[File:P_ID2720__Sig_T_2_4_S7_neu.png|center|frame|On the Gibbs Phenomenon]] |
| − | + | From the right graphic you can see that the flank and the inner area are marked with | |
| − | N=100 | + | N=100 but due to the Gibbs phenomenon there are still oscillations around 9% at the jumping point. |
| − | * | + | *Since the jump amplitudes here are equal to 2 the maximum values are approximately 1.18. |
| − | * | + | *With N=1000 the oscillations would be exactly the same size, but limited to a narrower space and possibly not recognizable with time-discrete representation.}} |
Revision as of 11:27, 27 October 2020
Contents
General Description
Every periodic function x(t) can be fragmented into a trigonometric series, which is called Fourier series, in all areas, where it is continuous or has only finite discontinuities.
Definition: The Fourier series of a periodic signal x(t) is defined as follows
- x(t)=A0+∞∑n=1An⋅cos(nω0t)+∞∑n=1Bn⋅sin(nω0t).
Here the symbols denote the following definitions:
- A0 the constant component of x(t),
- An the cosine coefficients with n≥1,
- Bn the sine coefficients mit n≥1,
- ω0=2π/T0 the angular frequency of the periodic signal (T0 is the period duration).
If the Fourier series is to exactly match the actual periodic signal x(t) , an infinite number of cosine and sine coefficients must generally be used for calculation.
- If the Fourier series is interrupted and only N of An and Bn coefficients is used, then a slightly different plot of the function results except for some special cases:
- xN(t)=A0+N∑n=1An⋅cos(nω0t)+N∑n=1Bn⋅sin(nω0t).
- The relation between the periodic signal x(t) and the Fourier series approximation xN(t) holds:
- x(t)=lim
- If N \cdot f_0 is the highest frequency occurring in the signal x(t) then of course x_N(t) = x(t).
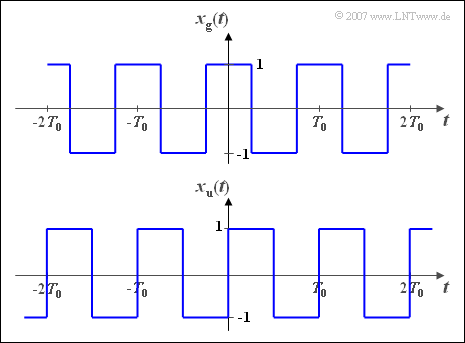
\text{Example 1:} We consider two periodic square wave signals, each with the period duration T_0 and the fundamental frequency \omega_0 = 2\pi/T_0.
- For the even time signal sketched above: x_{\rm g}(-t) = x_{\rm g}(t).
- The function shown below is odd: x_{\rm u}(-t) = -x_{\rm u}(t).
One finds the fourier series representations of both signals in formularies:
- x_{\rm g}(t)=\frac{4}{\pi}\left [ \cos(\omega_0 t)-\frac{1}{3}\cdot \cos(3 \omega_0 t)+\frac{1}{5}\cdot\cos(5 \omega_0 t)- \hspace{0.05cm}\text{...}\hspace{0.05cm} + \hspace{0.05cm}\text{...}\hspace{0.05cm}\right ],
- x_{\rm u}(t)=\frac{4}{\pi}\left [ \sin(\omega_0 t)+\frac{1}{3}\cdot\sin(3 \omega_0 t)+\frac{1}{5}\cdot\sin(5 \omega_0 t)+ \hspace{0.05cm}\text{...}\hspace{0.05cm} + \hspace{0.05cm}\text{...}\hspace{0.05cm} \right ].
- Because of the generally valid relationship
- 1-\frac{1}{3}+\frac{1}{5}-\frac{1}{7}+\frac{1}{9}\, {-}\, \hspace{0.05cm}\text{...}\hspace{0.05cm} \, {+} \hspace{0.05cm}\text{...}\hspace{0.05cm}=\frac{\pi}{4}
the amplitudes (maximum values) of the two rectangle pulses result to 1.
- This can also be verified using the signal curves in the above graphic:
- x_{\rm g}(t = 0) = x_{\rm u}(t = T_0/4) = 1.
Calculation of the Fourier Coefficients
The Fourier coefficient A_0 specifies the constant component which can be determined by averaging over the signal course x(t) . Due to the periodicity, averaging over one period is sufficient:
- A_0=\frac{1}{T_0}\cdot \int^{+T_0/2}_{-T_0/2}x(t)\,{\rm d}t.
The integration limits can also be selected from t = 0 to t = T_0 (or over a differently defined period of equal length).
The determination of the Fourier coefficients A_n and B_n (n \ge 1) is based on the property that the harmonic cosine functions and sine functions are so-called orthogonal functions . For them the following applies:
- \int^{+T_0/2}_{-T_0/2}\cos(n \omega_0 t)\cdot\cos(m \omega_0 t)\,{\rm d}t=\left \{{T_0/2\atop 0}{\rm\quad if \it \quad m=n,\atop \rm sonst} \right.
- \int ^{+T_0/2}_{-T_0/2}\sin(n\omega_0 t)\cdot\sin(m \omega_0 t)\,{\rm d}t=\left \{{T_0/2\atop 0}{\rm\quad if \it \quad m=n,\atop \rm sonst} \right.
- \int ^{+T_0/2}_{-T_0/2}\cos(n \omega_0 t)\cdot\sin(m \omega_0 t)\,{\rm d}t=0 \quad \rm f\ddot{u}r\quad alle \ \it m, \ n.
\text{Conclusion:} Considering these equations, the cosine coefficients A_n and the sine coefficients B_n result as follows
- A_{\it n}=\frac{2}{T_0}\cdot \int^{+T_0/2}_{-T_0/2}x(t)\cdot\cos(n \omega_0 t)\,{\rm d}t,
- B_{\it n}=\frac{2}{T_0}\cdot \int^{+T_0/2}_{-T_0/2}x(t)\cdot\sin(n \omega_0 t)\,{\rm d}t.
The german learning video Calculating the Fourier Coefficients illustrates these equations.
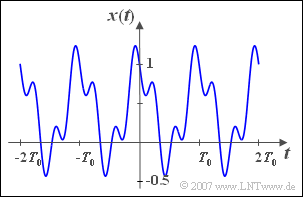
\text{Example 2:} We consider the drawn periodic time function
- x(t)=0.4+0.6\cdot \cos(\omega_0 t)-0.3\cdot\sin(3 \omega_0 t).
Since the integral of the cosine and sine functions is identical to zero over one period in each case, one obtains for the DC signal coefficient A_0 = 0.4.
One determines the cosine coefficient A_1 with the following equations (Integration limits from t = 0 to t = T_0):
- \begin{align*} A_{1}=\frac{2}{T_0}\cdot \int^{T_0}_{0}\hspace{-0.3cm}0.4\cdot\cos(\omega_0 t)\,{\rm d}t + \frac{2}{T_0}\cdot \int^{T_0}_{0}\hspace{-0.3cm}0.6\cdot\cos^2(\omega_0 t)\,{\rm d}t - \frac{2}{T_0}\cdot \int^{T_0}_{0}\hspace{-0.3cm}0.3\cdot\sin(3 \omega_0 t)\cdot \cos(\omega_0 t)\,{\rm d}t.\end{align*}
The last integral is equal to zero due to orthogonality; the first one is zero too (integral over one period).
- Only the middle term contributes here to A_1, namely 2 - 0.6 - 0.5 = 0.6.
- For all further (n \ge 2) cosine coefficients all three integrals return the value zero, and thus A_{n \hspace{0.05cm}\neq \hspace{0.05cm}1}=0.
To determine the sine coefficients B_n with the determining equation:
- \begin{align*} B_{\it n}=\frac{2}{T_0}\cdot \int^{T_0}_{0}\hspace{-0.3cm}0.4 \cdot \sin(n \ \omega_0 t)\,{\rm d}t + \frac{2}{T_0} \cdot \int^{T_0}_{0}\hspace{-0.3cm}0.6\cdot \cos(\omega_0 t) \sin(n \omega_0 t)\,{\rm d}t - \frac{2}{T_0}\cdot \int^{T_0}_{0}\hspace{-0.3cm}0.3\cdot \sin(3 \omega_0 t) \sin(n \omega_0 t )\,{\rm d}t. \end{align*}
- For n \hspace{0.05cm}\neq \hspace{0.05cm}3 all three integral values are zero and therefore B_{n \hspace{0.05cm}\neq \hspace{0.05cm}3} = 0.
- On the other hand, for n=3 the last integral provides a contribution, and one gets for the sine coefficient B_3 = -0.3.
Exploitation of Symmetries
Some insights into the Fourier coefficients A_n and B_n can already be read from the Symmetry properties of the time function x(t) .
- If the time signal x(t) is an even function ⇒ axis-symmetrical around the ordinate (t = 0), all sine coefficients B_n disappear, since the sine function itself is an odd function ⇒ \sin(-\alpha) = -\sin(\alpha):
- B_n = 0 \hspace{0.4cm}(n = 1, \ 2, \ 3, \text{...}).
- An odd function x(t) is point symmetric around the coordinate origin (t= 0; \ x =0). Therefore, all cosine coefficients (A_n = 0) disappear here, since the cosine function itself is even. In this case, the constant component A_0 is also always zero.
- A_n = 0 \hspace{0.4cm}(n = 0, \ 1, \ 2, \ 3, \text{...}).
- If a function without a constant component is present (A_0 = 0) and if this function is odd within a period ⇒ it is x(t) = -x(t - T_0/2), then only odd multiples of the fundamental frequency are present in the Fourier series representation. For the coefficients with an even index, however, the following always applies:
- A_n = B_n = 0 \hspace{0.4cm}(n = 2, \ 4, \ 6, \text{...}).
- If all coefficients A_n and B_n with even-numbered index (n = 2, \ 4, \ 6, \text{...}) equals zero and the coefficient A_0 \neq 0, then the symmetry property mentioned in the last point refers to the DC component and applies:
- x(t) = 2 \cdot A_0 - x (t - T_0/2).
Remark: Several of the named symmetry properties can be fulfilled at the same time.
The symmetry properties of the Fourier coefficients are explained in the first part of the german learning video Properties and Accuracy of the fourier series .
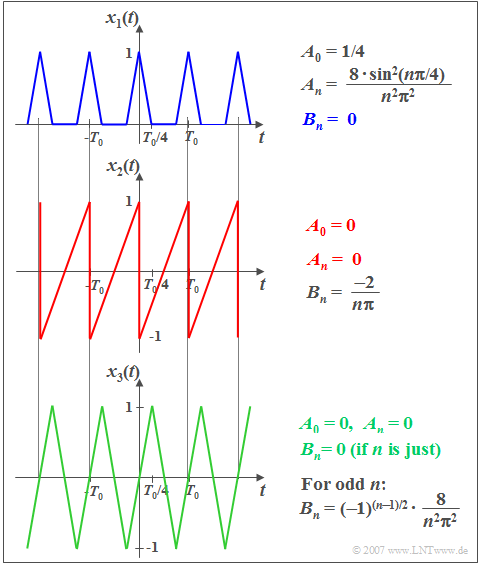
\text{Example 3:} The above mentioned properties are now illustrated by three signal characteristics.
- x_1(t) is an averaged function ⇒ A_0 \ne 0 and is also even, which is accordingly exclusively determined by cosine coefficients A_n (B_n = 0).
- In contrast, the odd function x_2(t) all A_n, \ ( n \ge 0) are identical to zero.
- Also the odd function x_3(t) contains only sine coefficients, but because of x_3(t) = -x_3(t - T_0/2) exclusively for odd values of n.
Complex Fourier Series
As shown on the page 3 Representation with Cosine and Sine Components in case of a harmonic oscillation any periodic signal
- x(t) =A_0+\sum^{\infty}_{n=1}A_{\it n} \cdot\cos(n \omega_0 t)+\sum^{\infty}_{n=1} B_n \cdot \sin(n \omega_0 t)
can also be displayed using the magnitude and phase coefficients:
- x(t) =C_0+\sum^{\infty}_{n=1}C_{\it n} \cdot\cos(n \omega_0 t-\varphi_n).
These modified Fourier coefficients have the following properties:
- The DC coefficient C_0 is identical with A_0.
- The magnitude coefficient read with n\ge 1: C_n = \sqrt{A_n^2 + B_n^2}.
- For the phase coefficien applies: \varphi_n = \arctan \hspace{0.05cm}(B_n/A_n).
With the „Eulerian relationship” \cos(x) + {\rm j} \cdot \sin(x) = {\rm e}^{{\rm j} \hspace{0.05cm}x} we get a second representation variant of the Fourier series evolution, which starts from the complex exponential function.
\text{Definition:} The complex Fourier series of a periodic signal x(t) is as follows
- x(t)=\sum^{+\infty}_{ n=- \infty}D_n\cdot {\rm e}^{ {\rm j} \hspace{0.05cm} n \hspace{0.05cm}\omega_0\hspace{0.05cm} t}.
Here D_n denote the complex Fourier coefficients, which
- from the cosine coefficients A_n and the sine coefficients B_n, or
- from the magnitude coefficients C_n and the phase coefficients \varphi_n
can be calculated as follows (valid for n \neq 0):
- D_n = 1/2\cdot (A_n - {\rm j}\cdot B_n) =1/2\cdot C_n\cdot {\rm e}^{- {\rm j} \hspace{0.05cm} \varphi_n }
The complex Fourier coefficients can also be calculated directly using the following equation
- D_n=\frac{1}{T_0}\cdot \int^{+T_0/2}_{-T_0/2}x(t) \cdot{\rm e}^{-\rm j \hspace{0.05cm}\it n \hspace{0.1cm}\omega_{\rm 0} \hspace{0.05cm}t}\, {\rm d}t.
As long as the integration interval T_0 is preserved, it can be shifted randomly as with the coefficients A_n and B_n for example from t = 0 to t = T_0.
\text{Conclusion:} The coefficient D_0 = A_0 is always real. For the complex coefficients with negative iterating index (n < 0) applies:
- D_{- n}=D_n^{\hspace{0.05cm}\star} =1/2 \cdot (A_n+ {\rm j}\cdot B_n).
Periodic Signal Spectrum
Starting from the complex Fourier series just derived
- x(t)=\sum^{+\infty}_{n=-\infty}D_{\it n}\cdot \rm e^{j \it n \omega_{\rm 0} t}
and the Displacement Theorem (for the frequency domain) one gets the following spectrum for the periodic signal x(t):
- X(f)=\sum^{+\infty}_{n=-\infty}D_n\cdot\delta(f-n\cdot f_0).
This means:
- Das Spektrum eines mit T_0 periodischen Signals ist ein Linienspektrum bei ganzzahligen Vielfachen der Grundfrequenz f_0 = 1/T_0.
- Der Gleichanteil liefert eine Diracfunktion bei f=0 mit dem Impulsgewicht A_0.
- Daneben gibt es Diracfunktionen \delta(f \pm n \cdot f_0) bei Vielfachen von f_0, wobei \delta(f - n \cdot f_0) eine Diracfunktion bei f= n \cdot f_0 (also im positiven Frequenzbereich) und \delta(f + n \cdot f_0) eine solche bei der Frequenz f= -n \cdot f_0 (im negativen Frequenzbereich) kennzeichnet.
- Die Impulsgewichte sind im allgemeinen komplex.
Diese Aussagen werden nun anhand zweier Beispiele verdeutlicht.
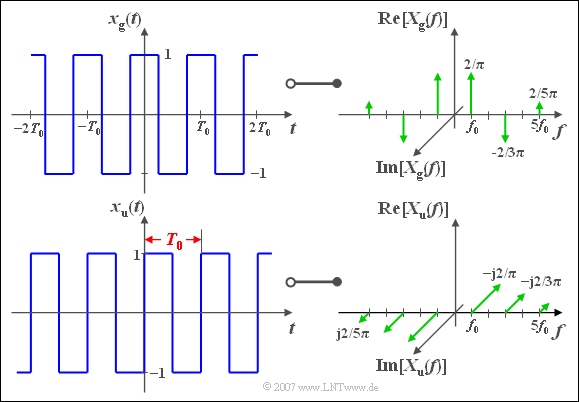
\text{Example 4:} Wir betrachten – wie im \text{Beispiel 1} zu Beginn dieses Abschnitts - zwei periodische Rechtecksignale, jeweils mit Periodendauer T_0 und Grundfrequenz f_0=1/T_0. Das obere Signal
- x_{\rm g}(t)={4}/{\pi} \cdot \big[\cos(\omega_0 t) - {1}/{3} \cdot \cos(3\omega_0 t)+{1}/{5}\cdot \cos(5\omega_0 t) - \, \text{...} \, + \, \text{...} \big]
ist eine gerade, aus verschiedenen Cosinusanteilen zusammengesetzte Funktion. Die zugehörige Spektralfunktion X_{\rm g}(f) ist damit rein reell.
Begründung: Wie auf der Seite Spektraldarstellung eines Cosinussignals bereits beschrieben wurde, liefert die Grundwelle zwei Diracfunktionen bei \pm f_0, jeweils gewichtet mit 2/\pi.
- Dieses Gewicht entspricht den (im Allgemeinen komplexen) Fourierkoeffizienten D_1 = D_{ - 1}^\ast, die nur im Sonderfall einer geraden Funktion reell sind.
- Weitere Diracfunktionen gibt es bei \pm 3f_0 (negativ), \pm 5f_0 (positiv), \pm 7f_0 (negativ) usw.
- Alle Phasenwerte \varphi_n sind aufgrund der alternierenden Vorzeichen entweder Null oder \pi.
The function x_{\rm u}(t) shown below is odd:
- x_{\rm u}(t)={4}/{\pi} \cdot \big[\sin(\omega_0 t)+{1}/{3} \cdot \sin(3\omega_0 t)+{1}/{5} \cdot \sin(5\omega_0 t)+ \, \text{...}\big].
Begründung: Wie im \text{Beispiel 4} auf der Seite Allgemeine Spektraldarstellung bereits beschrieben wurde, liefert hier die Grundwelle zwei Diracfunktionen bei +f_0 (gewichtet mit -\text{j}\cdot 2/\pi) bzw. bei -f_0 (gewichtet mit +\text{j}\cdot 2/\pi).
- Auch alle weiteren Diracfunktionen bei \pm 3f_0, \pm 5f_0, usw. sind rein imaginär und liegen in gleicher Richtung wie die Diracs bei \pm f_0.
- Die beiden Betragsspektren sind gleich: \vert X_{\rm u}(f)\vert = \vert X_{\rm g}(f) \vert.
The Gibbs Phenomenon
Nicht jedes Signal eignet sich für die Fourierreihendarstellung. Hier einige Einschränkungen:
- Eine wichtige Voraussetzung für die Konvergenz der Fourierreihe ist, dass das Signal nur endlich viele Unstetigkeitsstellen je Periode besitzen darf.
- An denjenigen Stellen t=t_i, an denen x(t) Sprünge aufweist, konvergiert die Reihe gegen den aus dem jeweiligen links– und rechtsseitigen Grenzwert gebildeten arithmetischen Mittelwert.
- In der Umgebung solcher Sprungstellen kommt es in der Reihendarstellung meist zu hochfrequenten Oszillationen. Dieser Fehler ist von prinzipieller Art, das heißt, er ließe sich auch nicht vermeiden, wenn man unendlich viele Summanden berücksichtigen würde. Man spricht vom Gibbschen Phänomen, benannt nach dem Physiker Josiah Willard Gibbs.
- Durch eine Erhöhung von N wird zwar der fehlerhafte Bereich kleiner, nicht jedoch die maximale Abweichung zwischen x(t) und der Fourierreihendarstellung x_N(t). Der maximale Fehler beträgt ca. 9\% der Sprungamplitude – und zwar unabhängig von N.
Das Gibbsche Phänomen und weitere interessante Aspekte zu vergleichbaren Effekten werden im Lernvideo
Eigenschaften der Fourierreihendarstellung behandelt.
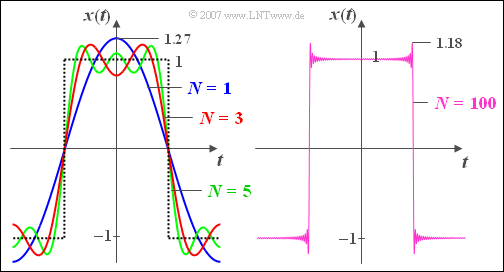
\text{Example 5:} In der linken Grafik sehen Sie gepunktet einen Ausschnitt eines periodischen \pm 1–Rechtecksignals und die dazugehörige Fourierreihendarstellung mit N = 1 (blau), N = 3 (rot) und N = 5 (grün) Summanden.
- Die Grundwelle hat hier den Amplitudenwert 4/\pi \approx 1.27.
- Auch mit N = 5 (das bedeutet wegen A_2 = A_4 = 0 drei „relevante” Summanden) unterscheidet sich die Fourierreihe vom anzunähernden Rechtecksignal noch deutlich, vor allem im Bereich der Flanke.
From the right graphic you can see that the flank and the inner area are marked with N = 100 but due to the Gibbs phenomenon there are still oscillations around 9\% at the jumping point.
- Since the jump amplitudes here are equal to 2 the maximum values are approximately 1.18.
- With N = 1000 the oscillations would be exactly the same size, but limited to a narrower space and possibly not recognizable with time-discrete representation.
Exercises for the Chapter
Aufgabe 2.4: Gleichgerichteter Cosinus
Aufgabe 2.5: Einweggleichrichtung
Aufgabe 2.6: Komplexe Fourierreihe
Aufgabe 2.6Z: Betrag und Phase