Difference between revisions of "Aufgaben:Exercise 2.1: Rectification"
| Line 5: | Line 5: | ||
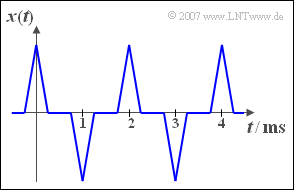
The graph shows the periodic signal $x(t)$. If $x(t)$ is applied to the input of a non-linearity with the characteristic curve | The graph shows the periodic signal $x(t)$. If $x(t)$ is applied to the input of a non-linearity with the characteristic curve | ||
| − | :$$y=g(x)=\left\{ {x \; \rm | + | :$$y=g(x)=\left\{ {x \; \rm for\; \it x \geq \rm 0, \atop {\rm 0 \;\;\; \rm else,}}\right.$$ |
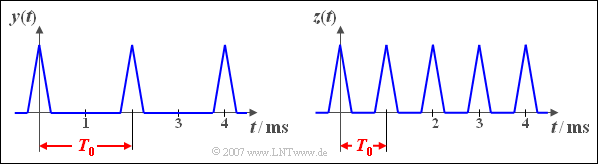
the signall $y(t)$ is obtained at the output. A second non-linear characteristic | the signall $y(t)$ is obtained at the output. A second non-linear characteristic | ||
| Line 29: | Line 29: | ||
{Which of the following statements are true? | {Which of the following statements are true? | ||
|type="[]"} | |type="[]"} | ||
| − | +$y = g(x)$ | + | +$y = g(x)$ describes a half-wave rectifier. |
| − | -$y = g(x)$ | + | -$y = g(x)$ describes a full-wave rectifier. |
| − | -$z = h(x)$ | + | -$z = h(x)$ describes a half-wave rectifier. |
| − | +$z = h(x)$ | + | +$z = h(x)$ describes a full-wave rectifier. |
| − | { | + | {What is the base frequency $f_0$ of the signal $x(t)$? |
|type="{}"} | |type="{}"} | ||
$f_0 \ = \ $ { 500 3% } $\text{Hz}$ | $f_0 \ = \ $ { 500 3% } $\text{Hz}$ | ||
| − | { | + | {What is the period $T_0$ of the signal $y(t)$? |
|type="{}"} | |type="{}"} | ||
$T_0 \ = \ $ { 2 3% } $\text{ms}$ | $T_0 \ = \ $ { 2 3% } $\text{ms}$ | ||
| − | { | + | {What is the base angular frequency $\omega_0$ of the signal $z(t)$? |
|type="{}"} | |type="{}"} | ||
$\omega_0 \ = \ $ { 6283 3% } $\text{1/s}$ | $\omega_0 \ = \ $ { 6283 3% } $\text{1/s}$ | ||
| Line 51: | Line 51: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
'''(1)''' Richtig sind die <u>Lösungsvorschläge 1 und 4</u>: | '''(1)''' Richtig sind die <u>Lösungsvorschläge 1 und 4</u>: | ||
Revision as of 00:07, 27 December 2020
The graph shows the periodic signal $x(t)$. If $x(t)$ is applied to the input of a non-linearity with the characteristic curve
- $$y=g(x)=\left\{ {x \; \rm for\; \it x \geq \rm 0, \atop {\rm 0 \;\;\; \rm else,}}\right.$$
the signall $y(t)$ is obtained at the output. A second non-linear characteristic
- $$z=h(x)=|x|$$
delivers the signal $z(t)$.
Hint:
- This exercise belongs to the chapter General description of periodic signals.
Questions
Solution
- Die nichtlineare Kennlinie $y = g(x)$ beschreibt einen Einweggleichrichter.
- $z = h(x) = |x|$ beschreibt einen Zweiweggleichrichter.
(2) Die Periodendauer des gegebenen Signals $x(t)$ beträgt $T_0 = 2\,\text{ms}$. Der Kehrwert hiervon ergibt die Grundfrequenz $f_0 \hspace{0.1cm}\underline{ = 500\,\text{Hz}}$.
(3) Die Einweggleichrichtung ändert nichts an der Periodendauer, siehe linke Skizze. Somit gilt weiterhin $T_0 \hspace{0.1cm}\underline{= 2\,\text{ms}}$.
(4) Das Signal $z(t)$ nach der Doppelweggleichrichtung hat dagegen die doppelte Frequenz (siehe rechte Darstellung). Hier gelten folgende Werte:
- $$T_0 = 1\,\text{ms}, \hspace{0.5cm} f_0 = 1\,\text{kHz}, \hspace{0.5cm} \omega_0 \hspace{0.1cm}\underline{= 6283\,\text{1/s}}.$$