Difference between revisions of "Aufgaben:Exercise 2.2Z: Non-Linearities"
From LNTwww
m (Guenter moved page Exercise 2.2Z: Nonlinearities to Exercise 2.2Z: Non-Linearities) |
|
(No difference)
| |
Revision as of 16:57, 12 April 2021
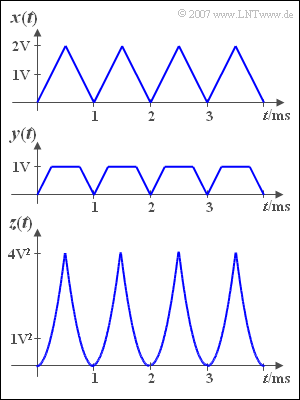
We start from the triangular signal x(t) according to the figure above.
If we apply this signal to an amplitude limiter, we get the signal
- y(t)={x(t)1Vforx(t)≤1Velse.
A second non-linearity provides the signal
- z(t)=x2(t).
The DC signal components are designated x0, y0 and z0 in the following.
Hint:
- This exercise belongs to the chapter Direct Current Signal - Limit Case of a Periodic Signal.
Questions
Solution
(1) The DC signal x0 is the mean value of the signal x(t). Averaging over a period duration T0=1ms is sufficient. One obtains:
- x0=1T0∫T00x(t)dt=1V_.
(2) In half the time y(t)=1V, in the other half is is between 0 and 1V with the mean value at 0.5V ⇒ y0=0.75V_.
(3) Due to the periodicity and symmetry, averaging in the range from 0 bis T0/2 is sufficient.
- With the corresponding characteristic curve, the following then applies::
- z0=1T0/2∫T0/20x2(t)dt=4V2T0/2∫T0/20(2t/T0)2dt=4/3V2≈1.333V2_.