Difference between revisions of "Aufgaben:Exercise 2.2Z: Non-Linearities"
From LNTwww
(Die Seite wurde neu angelegt: „ {{quiz-Header|Buchseite=Signaldarstellung/Allgemeine Beschreibung }} ==Z2.2 Nichtlinearitäten== right| Wir gehen von dem dre…“) |
|||
| (23 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Signal Representation/General Description |
}} | }} | ||
| − | + | ||
| − | [[File:P_ID322__Sig_Z_2_2.png|right|]] | + | [[File:P_ID322__Sig_Z_2_2.png|right|frame|DC component after non-linearities]] |
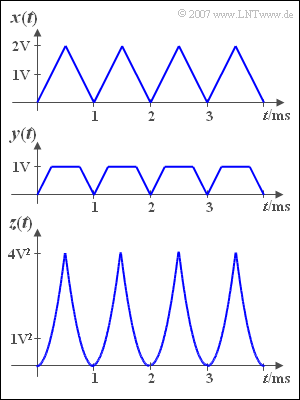
| − | + | We start from the triangular signal x(t) according to the figure above. | |
| − | :$$y(t)=\left\{ {x(t)\atop \rm 1V}{\hspace{0.5cm} {\rm | + | |
| − | + | *If we apply this signal to an amplitude limiter, we get the signal | |
| + | :$$y(t)=\left\{ {x(t)\atop \rm 1V}{\hspace{0.5cm} {\rm for}\quad x(t)\le \rm 1V \atop {\rm else}}\right..$$ | ||
| + | *Another non-linearity provides the signal | ||
:z(t)=x2(t). | :z(t)=x2(t). | ||
| − | + | The DC signal components are designated x0, y0 and z0 in the following. | |
| + | |||
| + | |||
| − | |||
| − | === | + | |
| + | ''Hint:'' | ||
| + | *This task belongs to chapter [[Signal_Representation/Direct_Current_Signal_-_Limit_Case_of_a_Periodic_Signal|Direct Current Signal - Limit Case of a Periodic Signal]]. | ||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Determine the DC signal component x0 of the signal x(t). |
|type="{}"} | |type="{}"} | ||
| − | x0 | + | $x_0\ = \ $ { 1 3% } V |
| − | { | + | {Determine the DC signal component y0 of the signal y(t). |
|type="{}"} | |type="{}"} | ||
| − | y0 | + | $y_0\ = \ $ { 0.75 3% } V |
| − | { | + | {Determine the DC signal component z0 of the signal z(t). |
|type="{}"} | |type="{}"} | ||
| − | z0 | + | $z_0\ = \ $ { 1.333 3% } V2 |
| Line 34: | Line 43: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' The DC signal x0 is the mean of signal x(t). Averaging over a period duration $T_0 = 1 \, \text{ms}$ is sufficient. One obtains: |
| − | :x0=1T0∫T00x(t)dt=1V_. | + | :$$x_0=\frac{1}{T_0}\int^{T_0}_0 x(t)\,{\rm d} t \hspace{0.15cm}\underline{=1\,\rm V}.$$ |
| + | |||
| + | |||
| + | |||
| + | '''(2)''' In half the time y(t)=1V, in the other half is is between 0 and 1V with the mean at 0.5V ⇒ y0=0.75V_. | ||
| + | |||
| − | |||
| − | '''3 | + | '''(3)''' Due to the periodicity and symmetry, averaging in the range from 0 bis T0/2 is sufficient. |
| + | * With the corresponding characteristic curve, the following then applies: | ||
:$$z_0=\frac{1}{T_0/2}\int^{T_0/2}_0 x^2(t)\,{\rm d}t=\frac{4\rm V^2}{T_0/2}\int^{T_0/2}_0 ({2t}/{T_0})^2\, {\rm d}t={4}/{3}\rm \;V^2 | :$$z_0=\frac{1}{T_0/2}\int^{T_0/2}_0 x^2(t)\,{\rm d}t=\frac{4\rm V^2}{T_0/2}\int^{T_0/2}_0 ({2t}/{T_0})^2\, {\rm d}t={4}/{3}\rm \;V^2 | ||
\hspace{0.15cm}\underline{\approx1.333\rm \;V^2}.$$ | \hspace{0.15cm}\underline{\approx1.333\rm \;V^2}.$$ | ||
| Line 48: | Line 62: | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^2.2 Direct Current Signal^]] |
Latest revision as of 13:24, 14 April 2021
We start from the triangular signal x(t) according to the figure above.
- If we apply this signal to an amplitude limiter, we get the signal
- y(t)={x(t)1Vforx(t)≤1Velse.
- Another non-linearity provides the signal
- z(t)=x2(t).
The DC signal components are designated x0, y0 and z0 in the following.
Hint:
- This task belongs to chapter Direct Current Signal - Limit Case of a Periodic Signal.
Questions
Solution
(1) The DC signal x0 is the mean of signal x(t). Averaging over a period duration T0=1ms is sufficient. One obtains:
- x0=1T0∫T00x(t)dt=1V_.
(2) In half the time y(t)=1V, in the other half is is between 0 and 1V with the mean at 0.5V ⇒ y0=0.75V_.
(3) Due to the periodicity and symmetry, averaging in the range from 0 bis T0/2 is sufficient.
- With the corresponding characteristic curve, the following then applies:
- z0=1T0/2∫T0/20x2(t)dt=4V2T0/2∫T0/20(2t/T0)2dt=4/3V2≈1.333V2_.