Difference between revisions of "Aufgaben:Exercise 1.4Z: Entropy of the AMI Code"
| Line 34: | Line 34: | ||
*Reference is made in particular to the page [[Information_Theory/Nachrichtenquellen_mit_Gedächtnis#Die_Entropie_des_AMI.E2.80.93Codes|Die Entropie des AMI–Codes]]. | *Reference is made in particular to the page [[Information_Theory/Nachrichtenquellen_mit_Gedächtnis#Die_Entropie_des_AMI.E2.80.93Codes|Die Entropie des AMI–Codes]]. | ||
| − | * | + | *In general, the following relations exist between the decision content $H_0$, the entropy $H$ $($here equal to $H_{\rm C})$ und den Entropienäherungen: |
:$$H \le \ \text{...} \ \le H_3 \le H_2 \le H_1 \le H_0 | :$$H \le \ \text{...} \ \le H_3 \le H_2 \le H_1 \le H_0 | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | *In [[Aufgaben:1.4_Entropienäherungen_für_den_AMI-Code| | + | *In [[Aufgaben:1.4_Entropienäherungen_für_den_AMI-Code|task 1.4]] for equally probable symbols $\rm L$ and $\rm H$ the entropy approximations were calculated as follows (each in „bit/symbol”): |
:$$H_1 = 1.500\hspace{0.05cm},\hspace{0.2cm} H_2 = 1.375\hspace{0.05cm},\hspace{0.2cm}H_3 = 1.292 | :$$H_1 = 1.500\hspace{0.05cm},\hspace{0.2cm} H_2 = 1.375\hspace{0.05cm},\hspace{0.2cm}H_3 = 1.292 | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| Line 46: | Line 46: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Let the source symbols be equally probable $(p_{\rm L} = p_{\rm H}= 1/2)$. What is the entropy $H_{\rm C}$ of the code symbol sequence $\langle c_\nu \rangle$? |
|type="{}"} | |type="{}"} | ||
| − | $H_{\rm C} \ = \ $ { 1 3% } $\ \rm bit/ | + | $H_{\rm C} \ = \ $ { 1 3% } $\ \rm bit/ternary symbol$ |
| − | { | + | {What is the relative redundancy of the code symbol sequence? |
|type="{}"} | |type="{}"} | ||
$r_{\rm C} \ = \ $ { 36.9 3% } $\ \rm \%$ | $r_{\rm C} \ = \ $ { 36.9 3% } $\ \rm \%$ | ||
| − | { | + | {For the binary source, $p_{\rm L} = 1/4$ and $p_{\rm H} = 3/4$. What is the entropy of the code symbol sequence? |
|type="{}"} | |type="{}"} | ||
| − | $H_{\rm C} \ = \ $ { 0.811 3% } $\ \rm bit/ | + | $H_{\rm C} \ = \ $ { 0.811 3% } $\ \rm bit/ternary symbol$ |
| − | { | + | {What is the relative redundancy of the code symbol sequence? |
| + | |||
|type="{}"} | |type="{}"} | ||
$r_{\rm C} \ = \ $ { 48.8 3% } $\ \rm \%$ | $r_{\rm C} \ = \ $ { 48.8 3% } $\ \rm \%$ | ||
| Line 74: | Line 75: | ||
| − | { | + | {Calculate the approximation $H_{\rm 1}$ der Coderentropie für $p_{\rm L} = 3/4$ und $p_{\rm H} = 1/4$. |
|type="{}"} | |type="{}"} | ||
| − | $H_{\rm 1} \ = \ $ { 1.06 3% } $\ \rm bit/ | + | $H_{\rm 1} \ = \ $ { 1.06 3% } $\ \rm bit/ternary symbol$ |
| Line 82: | Line 83: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
'''(1)''' Da durch den AMI–Code weder neue Information hinzukommt noch Information verschwindet, ist die Entropie $H_{\rm C}$ der Codesymbolfolge $\langle c_\nu \rangle$ gleich der Quellenentropie $H_{\rm Q}$. | '''(1)''' Da durch den AMI–Code weder neue Information hinzukommt noch Information verschwindet, ist die Entropie $H_{\rm C}$ der Codesymbolfolge $\langle c_\nu \rangle$ gleich der Quellenentropie $H_{\rm Q}$. | ||
Revision as of 16:02, 22 May 2021
We assume similar prerequisites as in task 1.4 :
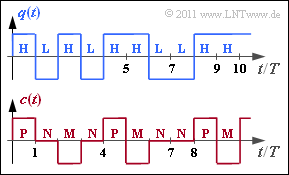
A binary source provides the source symbol sequence $\langle q_\nu \rangle$ with $q_\nu \in \{ {\rm L}, {\rm H} \}$, where there are no statistical ties between the individual sequence elements.
For the symbol probabilities, let:
- $p_{\rm L} =p_{\rm H} = 1/2$ (in subtasks 1 und 2),
- $p_{\rm L} = 1/4, \, p_{\rm H} = 3/4$ (subtasks 3, 4 and 5),
- $p_{\rm L} = 3/4, \, p_{\rm H} = 1/4$ (subtask 6).
The presented code signal $c(t)$ and the corresponding symbol sequence $\langle c_\nu \rangle$ with $c_\nu \in \{{\rm P}, {\rm N}, {\rm M} \}$ results from the AMI coding (Alternate Mark Inversion) according to the following rule:
- The binary symbol $\rm L$ ⇒ Low is always represented by the ternary symbol $\rm N$ ⇒ Null .
- The binary symbol $\rm H$ ⇒ High is also coded deterministically but alternately (hence the name „AMI”) by the symbols $\rm P$ ⇒ Plus and $\rm M$ ⇒ Minus codiert.
In this task, the decision content $H_0$ and the resulting entropy $H_{\rm C}$ the code symbol sequence $\langle c_\nu \rangle$ are to be determined for the three parameter sets mentioned above. The relative redundancy of the code sequence results from this according to the equation
- $$r_{\rm C} = \frac{H_{\rm 0}-H_{\rm C}}{H_{\rm C}} \hspace{0.05cm}.$$
Hints:
- The task belongs to the chapter Sources with Memory.
- Reference is made in particular to the page Die Entropie des AMI–Codes.
- In general, the following relations exist between the decision content $H_0$, the entropy $H$ $($here equal to $H_{\rm C})$ und den Entropienäherungen:
- $$H \le \ \text{...} \ \le H_3 \le H_2 \le H_1 \le H_0 \hspace{0.05cm}.$$
- In task 1.4 for equally probable symbols $\rm L$ and $\rm H$ the entropy approximations were calculated as follows (each in „bit/symbol”):
- $$H_1 = 1.500\hspace{0.05cm},\hspace{0.2cm} H_2 = 1.375\hspace{0.05cm},\hspace{0.2cm}H_3 = 1.292 \hspace{0.05cm}.$$
Questions
Solution
- Bei gleichwahrscheinlichen und statistisch voneinander unabhängigen Quellensymbolen gilt deshalb:
- $$H_{\rm Q} {= 1 \,{\rm bit/Bin\ddot{a}rsymbol}} \hspace{0.3cm} \Rightarrow\hspace{0.3cm} H_{\rm C} \hspace{0.15cm} \underline {= 1 \,{\rm bit/Tern\ddot{a}rsymbol}} \hspace{0.05cm}.$$
(2) Der Entscheidungsgehalt einer ternären Quelle beträgt $H_0 = \log_2 \; (3) = 1.585\; \rm bit/Symbol$.
- Damit ergibt sich für die relative Redundanz
- $$r_{\rm C} =1 -{H_{\rm C}/H_{\rm 0}}=1-1/{\rm log}_2\hspace{0.05cm}(3) \hspace{0.15cm} \underline {= 36.9 \,\%} \hspace{0.05cm}.$$
(3) Es gilt weiter $H_{\rm C} = H_{\rm Q}$. Wegen den ungleichen Symbolwahrscheinlichkeiten ist aber nun $H_{\rm Q}$ kleiner:
- $$H_{\rm Q} = \frac{1}{4} \cdot {\rm log}_2\hspace{0.05cm} (4) + \frac{3}{4} \cdot {\rm log}_2\hspace{0.1cm} (4/3) {= 0.811 \,{\rm bit/Bin\ddot{a}rsymbol}} \hspace{0.3cm} \Rightarrow\hspace{0.3cm} H_{\rm C} = H_{\rm Q} \hspace{0.15cm} \underline {= 0.811 \,{\rm bit/Tern\ddot{a}rsymbol}} \hspace{0.05cm}.$$
(4) In Analogie zur Teilaufgabe (2) gilt nun $r_{\rm C} = 1 - 0.811/1.585 \hspace{0.15cm} \underline {= 48.8 \,\%} \hspace{0.05cm}.$
- Man kann dieses Ergebnis verallgemeinern. Es gilt nämlich:
- $$(1-0.488) = (1- 0.189) \cdot (1- 0.369)\hspace{0.3cm} \Rightarrow\hspace{0.3cm} (1-r_{\rm Codefolge}) = (1-r_{\rm Quelle}) \cdot (1- r_{\rm AMI-Code}) \hspace{0.05cm}.$$
(5) Da jedes $\rm L$ auf $\rm N$ abgebildet wird und $\rm H$ alternierend auf $\rm M$ und $\rm P$, gilt
- $$p_{\rm N} = p_{\rm L} = 1/4\hspace{0.05cm},\hspace{0.2cm}p_{\rm P} = p_{\rm M} = p_{\rm H}/2 = 3/8\hspace{0.3cm} \Rightarrow\hspace{0.3cm} H_1 = {1}/{4} \cdot {\rm log}_2\hspace{0.1cm} (4) + 2 \cdot {3}/{8} \cdot {\rm log}_2\hspace{0.1cm}(8/3) \hspace{0.15cm} \underline {= 1.56 \,{\rm bit/Tern\ddot{a}rsymbol}} \hspace{0.05cm}.$$
(6) Nun ergeben sich die Auftrittswahrscheinlichkeiten der Ternärsymbole zu $p_{\rm N} = 3/4$ sowie $p_{\rm P} = p_{\rm M} =1/8$. Somit gilt:
- $$H_1 = {3}/{4} \cdot {\rm log}_2\hspace{0.1cm} (4/3) + 2 \cdot {1}/{8} \cdot {\rm log}_2\hspace{0.1cm}(8) \hspace{0.15cm} \underline {= 1.06 \,{\rm bit/Tern\ddot{a}rsymbol}} \hspace{0.05cm}.$$
Interpretation:
- Für $p_{\rm L} = 1/4, \ p_{\rm H} = 3/4$ ergibt sich $H_1 = 1.56 \; \rm bit/Symbol$.
- Für $p_{\rm L} = 3/4, \ p_{\rm H} = 1/4$ ergibt sich dagegen mit $H_1 = 1.06 \; \rm bit/Symbol$ ein deutlich kleinerer Wert.
- Für beide Parameterkombinationen gilt aber gleichermaßen:
- $$H_0 = 1.585 \,{\rm bit/Symbol}\hspace{0.05cm},\hspace{0.2cm}H_{\rm C} = \lim_{k \rightarrow \infty } H_k = 0.811 \,{\rm bit/Symbol} \hspace{0.05cm}.$$
Daraus folgt:
- Betrachtet man zwei Nachrichtenquellen $\rm Q1$ und $\rm Q2$ mit gleichem Symbolumfang $M$ ⇒ Entscheidungsgehalt $H_0 = \rm const.$, wobei bei der Quelle $\rm Q1$ die Entropienäherung erster Ordnung $(H_1)$ deutlich größer ist als bei der Quelle $\rm Q2$, so kann man daraus noch lange nicht schließen, dass die Entropie von $\rm Q1$ tatsächlich größer ist als die Entropie von $\rm Q2$.
- Vielmehr muss man für beide Quellen
- genügend viele Entropienäherungen $H_1$, $H_2$, $H_3$, ... berechnen, und
- daraus (grafisch oder analytisch) den Grenzwert von $H_k$ für $k \to \infty$ bestimmen.
- Erst dann ist eine endgültige Aussage über die Entropieverhältnisse möglich.