Difference between revisions of "Aufgaben:Exercise 1.4Z: Everything Rectangular"
From LNTwww
| Line 2: | Line 2: | ||
{{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/System_Description_in_Time_Domain}} | {{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/System_Description_in_Time_Domain}} | ||
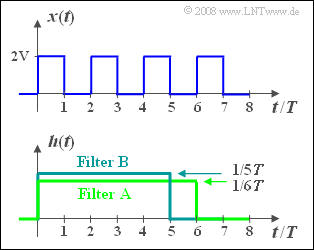
| − | [[File:P_ID834__LZI_Z_1_4.png |right|frame| | + | [[File:P_ID834__LZI_Z_1_4.png |right|frame|Periodic rectangular signal and <br>filter with rectangular impulse response]] |
| − | + | We consider the periodic rectangular signal $x(t)$ , whose periodic duration is $T_0 = 2T$ , according to the sketch above. | |
| − | * | + | *This signal has spectral components at the fundamental frequency $f_0 = 1/T_0 = 1/(2T)$ and at all odd multiples thereof, that is, at $3f_0$, $5f_0,$ and so on. In addition, there is a direct component. |
| − | * | + | *For this purpose, we consider two filters $\rm A$ and $\rm B$ each with rectangular impulse response $h_{\rm A}(t)$ with duration $6T$ and $h_{\rm B}(t)$ with duration $5T$, respectively. |
| − | * | + | *The heights of the two impulse responses are such that the areas of the rectangles each add up to $1$ . |
| Line 18: | Line 18: | ||
''Please note:'' | ''Please note:'' | ||
*The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/System_Description_in_Time_Domain|System Description in Time Domain]]. | *The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/System_Description_in_Time_Domain|System Description in Time Domain]]. | ||
| − | * | + | *For information on convolution, see the chapter [[Signal_Representation/The_Convolution_Theorem_and_Operation|convolution theorem and operation]] in the book "Signal Representation”. |
| − | * | + | *We also refer you to the interactive applet [[Applets:Zur_Verdeutlichung_der_grafischen_Faltung|Zur Verdeutlichung der graphischen Faltung]]. |
Revision as of 01:23, 19 July 2021
We consider the periodic rectangular signal $x(t)$ , whose periodic duration is $T_0 = 2T$ , according to the sketch above.
- This signal has spectral components at the fundamental frequency $f_0 = 1/T_0 = 1/(2T)$ and at all odd multiples thereof, that is, at $3f_0$, $5f_0,$ and so on. In addition, there is a direct component.
- For this purpose, we consider two filters $\rm A$ and $\rm B$ each with rectangular impulse response $h_{\rm A}(t)$ with duration $6T$ and $h_{\rm B}(t)$ with duration $5T$, respectively.
- The heights of the two impulse responses are such that the areas of the rectangles each add up to $1$ .
Please note:
- The exercise belongs to the chapter System Description in Time Domain.
- For information on convolution, see the chapter convolution theorem and operation in the book "Signal Representation”.
- We also refer you to the interactive applet Zur Verdeutlichung der graphischen Faltung.
Questions
Solution
(1) Das Ausgangssignal ist das Ergebnis der Faltungsoperation zwischen $x(t)$ und $h_{\rm A}(t)$:
- $$y_{\rm A}(t) = x (t) * h_{\rm A} (t) = \int_{ - \infty }^{ + \infty } {x ( \tau )} \cdot h_{\rm A} ( {t - \tau } ) \hspace{0.1cm}{\rm d}\tau.$$
- Aufgrund der Rechteckfunktion und der Dauer $6T$ kann hierfür auch geschrieben werden:
- $$y_{\rm A}(t) = \frac{1}{6T}\cdot \int_{t-6T}^{t}x(\tau)\hspace{0.15cm} {\rm d}\tau.$$
- Man erkennt, dass diese Gleichung für alle $t$ das gleiche Ergebnis $y_{\rm A}(t) \rm \underline{\: = 1V}$ liefert.
(2) Der Betragsfrequenzgang lautet $|H_{\rm A}(f)| = |{\rm si}(\pi \cdot f \cdot 6T)|.$ Dieser weist Nullstellen im Abstand $1/(6T)$ auf.
- Somit liegen auch bei $f_0$, $3f_0$, $5f_0$ usw. jeweils Nullstellen vor.
- Insbesondere gilt auch $|H_{\rm A}(f = f_0)| \underline{\: = 0}$.
- Vom Spektrum $X(f)$ bleibt somit nur der Gleichanteil $1 \hspace{0.05cm} \rm V$ unverändert erhalten.
- Dagegen sind alle anderen Spektrallinien in $Y_{\rm A}(f)$ nicht mehr enthalten.
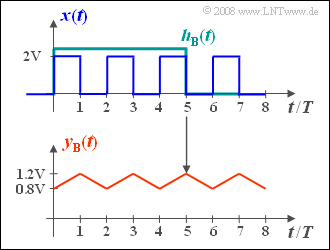
(3) Analog zur Teilaufgabe (1) kann man hier für das Ausgangssignal schreiben:
- $$y_{\rm B}(t) = \frac{1}{5T}\cdot \int_{t-5T}^{t}x(\tau)\hspace{0.15cm} {\rm d}\tau.$$
- Es ergibt sich ein um den Mittelwert $1 \ \rm V$ schwankender dreieckförmiger Verlauf ⇒ siehe untere Grafik.
- Da jeweils zwei Rechtecke und drei Lücken ins Integrationsintervall fallen, gilt für $t = 0, t = 2T,$ usw.:
- $$y_{\rm B}(t) = \frac{2\,{\rm V} \cdot 2T }{5T} \hspace{0.15cm}\underline{= 0.8\,{\rm V} =y_{\rm B}(t=0) }.$$
- Bei $t = T,\ 3T, \ 5T, $ usw. sind jeweils drei Rechtecke und zwei Lücken zu berücksichtigen: Man erhält:
- $$y_{\rm B}(t) \underline{\: = 1.2 \: {\rm V}=y_{\rm B}(t=T)}.$$
(4) Die Betragsfunktion lautet nun allgemein bzw. bei den Frequenzen $f = f_0 = 1/(2T)$ und $f = 3f_0$:
- $$\begin{align*} |H_{\rm B}(f)| & = |{\rm si}(\pi \cdot f \cdot 5T)|, \\ |H_{\rm B}(f = f_0)| & = |{\rm si}(\pi \frac{5T}{2T})| = |{\rm si}(2.5\pi )| = \frac{1}{2.5 \pi} \hspace{0.15cm}\underline{= 0.127}, \\ |H_{\rm B}(f = 3f_0)| & = |{\rm si}(7.5\pi )| = \frac{1}{7.5 \pi} \hspace{0.15cm}\underline{=0.042}.\end{align*}$$
Interpretation:
- Die Spektralanteile des Rechtecksignals bei $f_0, 3f_0,$ usw. werden zwar nun nicht mehr unterdrückt, aber mit steigender Frequenz immer mehr abgeschwächt und zwar in der Form, dass der Rechteckverlauf in ein periodisches Dreiecksignal gewandelt wird. Der Gleichanteil $(1 \hspace{0.05cm} \rm V)$ bleibt auch hier unverändert.
- Beide Filter liefern also den Mittelwert des Eingangssignals. Beim vorliegenden Signal $x(t)$ ist für die Bestimmung des Mittelwertes das Filter $\rm A$ besser geeignet als das Filter $\rm B$, da bei Ersterem die Länge der Impulsantwort ein Vielfaches der Periodendauer $T_0 = 2T$ ist.
- Ist diese Bedingung – wie beim Filter $\rm B$ – nicht erfüllt, so überlagert sich dem Mittelwert noch ein (in diesem Beispiel dreieckförmiges) Fehlersignal.