Difference between revisions of "Aufgaben:Exercise 2.13: Inverse Burrows-Wheeler Transformation"
| Line 3: | Line 3: | ||
}} | }} | ||
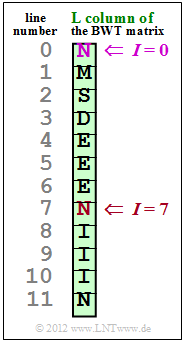
| − | [[File:EN_Inf_A_2_14.png|right|frame| | + | [[File:EN_Inf_A_2_14.png|right|frame|To be analysed <br>BWT result]] |
| − | Die ''Burrows–Wheeler–Transformation'' – | + | Die ''Burrows–Wheeler–Transformation'' – abbreviated $\rm BWT$ – causes a blockwise sorting of the characters of a text with the aim of preparing the text for efficient data compression with the help of run-length coding or entropy coding. |
| − | * | + | * First, an $N×N$– matrix is generated from a block of length $N$ , with each row of this first–matrix resulting from the preceding row by cyclic left shift. |
| − | * | + | * Then the matrix is sorted lexicographically (without special characters: alphabetically) . The result of the BWT is the last row of the new BWT matrix, the so-called $\text{L–column}$ (from "Last", last row of the BWT–matrix). |
| − | * | + | * Further, this task refers to the $\text{F–column}$ (from "First", first row of the BWT matrix), which is needed for the inverse Burrows–Wheeler–Transformation ⇒ reconstruction of the original text from the L–column. |
| − | * | + | * For the inverse BWT, the so-called <i>primary index</i> $I$ is also required. This indicates the row of the BWT matrix in which the algorithm must be started. |
| − | + | The graphic shows the result of a BWT, more precisely its L–column. The original text is to be reconstructed from this according to the description on the theory page [[Information_Theory/Weitere_Quellencodierverfahren#Burrows.E2.80.93Wheeler.E2.80.93Transformation|Burrows–Wheeler Transformation]] , | |
| − | * | + | *where in subtask '''(2)''' from the primary index $I = 7$ |
| − | * | + | *and in subtask '''(3)''' $I = 0$ is to be assumed. |
| Line 25: | Line 25: | ||
| − | + | Hints: | |
| − | * | + | *The task belongs to the chapter [[Information_Theory/Weitere_Quellencodierverfahren|Other source coding methods]]. |
| − | * | + | *In particular, reference is made to the page [[Information_Theory/Weitere_Quellencodierverfahren#Burrows.E2.80.93Wheeler.E2.80.93Transformation|Burrows–Wheeler Transformation]]. |
| − | * | + | *Further information can be found in the two publications mentioned below: |
: '''(1)''' Abel, J.: ''Verlustlose Datenkompression auf Grundlage der Burrows-Wheeler-Transformation''. <br> In: PIK - Praxis der Informationsverarbeitung und Kommunikation, no. 3, vol. 26, S. 140–144, Sept. 2003. | : '''(1)''' Abel, J.: ''Verlustlose Datenkompression auf Grundlage der Burrows-Wheeler-Transformation''. <br> In: PIK - Praxis der Informationsverarbeitung und Kommunikation, no. 3, vol. 26, S. 140–144, Sept. 2003. | ||
: '''(2)''' Abel, J.: ''Grundlagen des Burrows-Wheeler-Kompressionsalgorithmus''. <br> In: Informatik Forschung & Entwicklung, no. 2, vol. 18, S. 80–87, Jan. 2004. | : '''(2)''' Abel, J.: ''Grundlagen des Burrows-Wheeler-Kompressionsalgorithmus''. <br> In: Informatik Forschung & Entwicklung, no. 2, vol. 18, S. 80–87, Jan. 2004. | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the $\text{F–Spalte}$ associated with the given $\text{L–Spalte}$ ? |
|type="()"} | |type="()"} | ||
- $\rm SEINMEINDEIN$, | - $\rm SEINMEINDEIN$, | ||
| Line 43: | Line 43: | ||
| − | { | + | {What is the result of the reconstruction with primary index $\underline{I = 7}$? |
|type="()"} | |type="()"} | ||
+ $\rm MEINDEINSEIN$, | + $\rm MEINDEINSEIN$, | ||
| Line 50: | Line 50: | ||
| − | { | + | {What happens if the reconstruction $\text{(BWT back transformation})$ starts from the wrong primary index $I = 0$ ? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The result is the original text, read from back to front. |
| − | + | + | + The result is a cyclic permutation of the original. |
| − | + | Why is the Burrows–Wheeler transform better suited than the original with regard to a later data compression? | |
|type="[]"} | |type="[]"} | ||
| − | - | + | - It results in more favourable character frequencies. |
| − | - | + | - All characters are sorted lexicographically. |
| − | + | + | + Identical characters follow each other more often in the BWT. |
| Line 66: | Line 66: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solutions=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Solution suggestion 3</u> is correct: |
| − | * | + | *The first column of the BWT matrix is also called the F–column and the last column the L–column (from "<i>First</i>" or "<i>Last</i>"). |
| − | * | + | *Only the L–column is passed on to the next coding level. |
| − | * | + | *The F–column, which is also needed for the reverse transformation, results from the L–column by lexicographic sorting. |
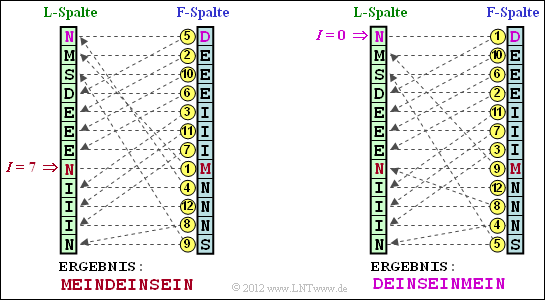
| − | '''(2)''' | + | '''(2)''' <u>Solution suggestion 1</u> is correct: <b>MEINDEINSEIN</b> is correct, as can be seen from the left-hand representation of the following diagram. <br>Note that the top line represents the line number $I = 0$ in each case. For explanation: |
| − | :[[File:P_ID2479__Inf_A_2_14b.png|right|frame| | + | :[[File:P_ID2479__Inf_A_2_14b.png|right|frame|BW back transformation with $I = 7$ (left) or $I = 0$ (right)]] |
| − | * | + | * Start the decoding with the line $I = 7$ of the F–column. The content is "M". |
| − | * | + | * Search for the corresponding "M" in the L–column and find it in line number "1". |
| − | * | + | * From line 1 of the L–column one goes horizontally to the F–column and finds the symbol "E". |
| − | * | + | * Similarly, one finds the third output symbol "I" in line 4 of theF–column. |
| − | * | + | * The decoding algorithm ends with the output symbol "N" in the third last row. |
| − | '''(3)''' | + | '''(3)''' Correct is the <u>proposed solution 2</u>: <br><br>$\rm DEINSEINMEIN$, as shown in the graph on the right. |
<br clear=all> | <br clear=all> | ||
| − | '''(4)''' | + | '''(4)''' Correct is the <u>suggested solution 3</u>: |
| − | * | + | *In BWT, four characters here are equal to their predecessors, in the original none. |
| − | *In | + | *In the F–column , even more characters would be the same as their respective predecessors (6 in total) due to the lexicographical sorting, but this sorting cannot be reversed without loss. |
| − | * | + | *Solution suggestion 1 is also wrong: the original and BWT contain exactly the same characters $($three times $\rm E$, three times $\rm I$, three times $\rm N$ and one each of $\rm D$, $\rm M$ and $\rm S)$. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Revision as of 13:11, 9 August 2021
Die Burrows–Wheeler–Transformation – abbreviated $\rm BWT$ – causes a blockwise sorting of the characters of a text with the aim of preparing the text for efficient data compression with the help of run-length coding or entropy coding.
- First, an $N×N$– matrix is generated from a block of length $N$ , with each row of this first–matrix resulting from the preceding row by cyclic left shift.
- Then the matrix is sorted lexicographically (without special characters: alphabetically) . The result of the BWT is the last row of the new BWT matrix, the so-called $\text{L–column}$ (from "Last", last row of the BWT–matrix).
- Further, this task refers to the $\text{F–column}$ (from "First", first row of the BWT matrix), which is needed for the inverse Burrows–Wheeler–Transformation ⇒ reconstruction of the original text from the L–column.
- For the inverse BWT, the so-called primary index $I$ is also required. This indicates the row of the BWT matrix in which the algorithm must be started.
The graphic shows the result of a BWT, more precisely its L–column. The original text is to be reconstructed from this according to the description on the theory page Burrows–Wheeler Transformation ,
- where in subtask (2) from the primary index $I = 7$
- and in subtask (3) $I = 0$ is to be assumed.
Hints:
- The task belongs to the chapter Other source coding methods.
- In particular, reference is made to the page Burrows–Wheeler Transformation.
- Further information can be found in the two publications mentioned below:
- (1) Abel, J.: Verlustlose Datenkompression auf Grundlage der Burrows-Wheeler-Transformation.
In: PIK - Praxis der Informationsverarbeitung und Kommunikation, no. 3, vol. 26, S. 140–144, Sept. 2003. - (2) Abel, J.: Grundlagen des Burrows-Wheeler-Kompressionsalgorithmus.
In: Informatik Forschung & Entwicklung, no. 2, vol. 18, S. 80–87, Jan. 2004.
Questions
Solutions
- The first column of the BWT matrix is also called the F–column and the last column the L–column (from "First" or "Last").
- Only the L–column is passed on to the next coding level.
- The F–column, which is also needed for the reverse transformation, results from the L–column by lexicographic sorting.
(2) Solution suggestion 1 is correct: MEINDEINSEIN is correct, as can be seen from the left-hand representation of the following diagram.
Note that the top line represents the line number $I = 0$ in each case. For explanation:
- Start the decoding with the line $I = 7$ of the F–column. The content is "M".
- Search for the corresponding "M" in the L–column and find it in line number "1".
- From line 1 of the L–column one goes horizontally to the F–column and finds the symbol "E".
- Similarly, one finds the third output symbol "I" in line 4 of theF–column.
- The decoding algorithm ends with the output symbol "N" in the third last row.
(3) Correct is the proposed solution 2:
$\rm DEINSEINMEIN$, as shown in the graph on the right.
(4) Correct is the suggested solution 3:
- In BWT, four characters here are equal to their predecessors, in the original none.
- In the F–column , even more characters would be the same as their respective predecessors (6 in total) due to the lexicographical sorting, but this sorting cannot be reversed without loss.
- Solution suggestion 1 is also wrong: the original and BWT contain exactly the same characters $($three times $\rm E$, three times $\rm I$, three times $\rm N$ and one each of $\rm D$, $\rm M$ and $\rm S)$.