Difference between revisions of "Aufgaben:Exercise 4.7Z: About the Water Filling Algorithm"
m (Text replacement - "”" to """) |
|||
| (8 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Information_Theory/AWGN_Channel_Capacity_for_Continuous_Input |
}} | }} | ||
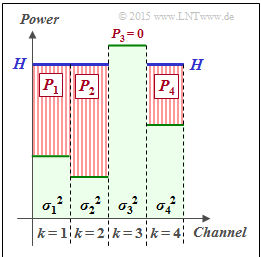
| − | [[File: | + | [[File:EN_Inf_Z_4_7.png|right|frame|Water-filling principle (K=4)]] |
| − | + | We consider K parallel Gaussian channels $\rm (AWGN)$ with different interference powers σ2k, where 1≤k≤K . The graph illustrates this constellation using K=4 as an example. | |
| − | + | The transmission power in the individual channels is denoted by Pk, the sum of which must not exceed the specified value PX : | |
:$$P_1 +\text{...}\hspace{0.05cm}+ P_K = \hspace{0.1cm} \sum_{k= 1}^K | :$$P_1 +\text{...}\hspace{0.05cm}+ P_K = \hspace{0.1cm} \sum_{k= 1}^K | ||
\hspace{0.1cm}{\rm E} \left [ X_k^2\right ] \le P_{X} \hspace{0.05cm}.$$ | \hspace{0.1cm}{\rm E} \left [ X_k^2\right ] \le P_{X} \hspace{0.05cm}.$$ | ||
| − | + | If the random variables X1, ... , XK are Gaussian, then for the (total) mutual information between the input X and the output Y can be written: | |
:$$I(X_1,\text{...} \hspace{0.05cm}, X_K\hspace{0.05cm};\hspace{0.05cm}Y_1, \text{...}\hspace{0.05cm}, Y_K) | :$$I(X_1,\text{...} \hspace{0.05cm}, X_K\hspace{0.05cm};\hspace{0.05cm}Y_1, \text{...}\hspace{0.05cm}, Y_K) | ||
= 1/2 \cdot \sum_{k= 1}^K \hspace{0.1cm} {\rm log}_2 \hspace{0.1cm} ( 1 + \frac{P_k}{\sigma_k^2})\hspace{0.05cm},\hspace{0.5cm} | = 1/2 \cdot \sum_{k= 1}^K \hspace{0.1cm} {\rm log}_2 \hspace{0.1cm} ( 1 + \frac{P_k}{\sigma_k^2})\hspace{0.05cm},\hspace{0.5cm} | ||
| − | {\rm | + | {\rm Result\hspace{0.15cm} in \hspace{0.15cm} \text{"bit"} } |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | The maximum for this is the '''channel capacity of the total system''', where the maximization refers to the division of the total power PX among the individual channels: | |
| − | :$$C_K(P_X) = \max_{P_k\hspace{0.05cm},\hspace{0.15cm}{\rm | + | :$$C_K(P_X) = \max_{P_k\hspace{0.05cm},\hspace{0.15cm}{\rm with} \hspace{0.15cm}P_1 + ... \hspace{0.05cm}+ P_K = P_X} \hspace{-0.5cm} I(X_1, \text{...} \hspace{0.05cm}, X_K\hspace{0.05cm};\hspace{0.05cm}Y_1, \text{...}\hspace{0.05cm}, Y_K) \hspace{0.05cm}.$$ |
| + | This maximization can be done with the "water–filling algorithm" shown in the above graph for K=4. | ||
| − | + | In the present exercise, this algorithm is to be applied, assuming the following: | |
| + | * Two parallel Gaussian channels ⇒ K=2, | ||
| + | * normalized noise powers $\sigma_1^2 = 1 and \sigma_2^2 = 4$, | ||
| + | * normalized transmission powers PX=10 and PX=3 respectively. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | Hints: | |
| − | + | *The exercise belongs to the chapter [[Information_Theory/AWGN–Kanalkapazität_bei_wertkontinuierlichem_Eingang|AWGN channel capacity with continuous value input]]. | |
| − | * | + | *Reference is made in particular to the page [[Information_Theory/AWGN–Kanalkapazität_bei_wertkontinuierlichem_Eingang#Parallel_Gaussian_channels|Parallel Gaussian Channels]]. |
| − | * | + | *Since the results are to be given in "bit", the logarithm to base 2 is used in the equations: log2. |
| − | * | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which power allocation strategies are useful? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - A very noisy channel k (with large noise power σ2k) should be allocated a large effective power Pk. |
| − | + | + | + A very noisy channel k (with large noise power σ2k) should be assigned only a small useful power $P_k$. |
| − | + | + For $K$ equally good channels ⇒ σ21=...=σ2K=σ2N the power Pk should be evenly distributed. | |
| − | { | + | {What is the mutual information obtained by distributing the transmission power PX=10 equally to both channels (P1=P2=5)? |
|type="{}"} | |type="{}"} | ||
I(X1,X2;Y1,Y2) = { 1.877 3% } bit | I(X1,X2;Y1,Y2) = { 1.877 3% } bit | ||
| − | { | + | {Let PX=P1+P2=10 be further valid. Which optimal power distribution results according to the "water–filling algorithm"? |
|type="{}"} | |type="{}"} | ||
P1 = { 6.5 3% } | P1 = { 6.5 3% } | ||
| Line 58: | Line 57: | ||
| − | { | + | {What is the channel capacity for K=2_ and PX=10_? |
|type="{}"} | |type="{}"} | ||
C = { 1.907 3% } bit | C = { 1.907 3% } bit | ||
| − | { | + | {What mutual information results if the transmit power PX=3 is distributed equally to both channels (P1=P2=1.5)? |
|type="{}"} | |type="{}"} | ||
I(X1,X2;Y1,Y2) = { 0.891 3% } bit | I(X1,X2;Y1,Y2) = { 0.891 3% } bit | ||
| − | { | + | {What is the channel capacity for K=2_ and PX=3_? |
|type="{}"} | |type="{}"} | ||
C = { 1 3% } bit | C = { 1 3% } bit | ||
| Line 74: | Line 73: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Proposed solutions 2 and 3</u> are correct: |
| − | * | + | *According to the explanations in the theory section "Water–Filling" ⇒ <u>Proposal 2</u> is to be applied when unequal conditions exist. |
| − | * | + | * However, <u>solution proposal 3</u> is also correct: If the channels are equally good, there is nothing to prevent all K channels from being filled with the same power ⇒ P1=P2= ... =PK=PX/K. |
| − | '''(2)''' | + | '''(2)''' For the mutual information, with equal power distribution, the following applies: |
:$$I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) \ = \ {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{5}{1} \right ) | :$$I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) \ = \ {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{5}{1} \right ) | ||
+{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{5}{4} \right )=1.292\,{\rm bit}+ 0.585\,{\rm bit} | +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{5}{4} \right )=1.292\,{\rm bit}+ 0.585\,{\rm bit} | ||
| Line 90: | Line 89: | ||
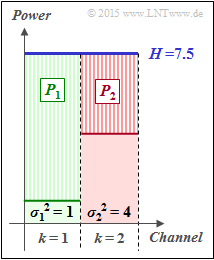
| − | [[File: | + | [[File:EN_Inf_Z_4_7b.png|right|frame|Best possible distribution of transmit power PX=10]] |
| − | '''(3)''' | + | '''(3)''' According to the adjacent sketch, the following must apply: |
:P2=P1−(σ22−σ21)=P1−3wobei P1+P2=PX=10 | :P2=P1−(σ22−σ21)=P1−3wobei P1+P2=PX=10 | ||
:$$\Rightarrow \hspace{0.3cm} | :$$\Rightarrow \hspace{0.3cm} | ||
| Line 99: | Line 98: | ||
\underline{P_1 = 6.5}\hspace{0.05cm}, | \underline{P_1 = 6.5}\hspace{0.05cm}, | ||
\hspace{0.3cm}\underline{P_2 = 3.5}\hspace{0.05cm}.$$ | \hspace{0.3cm}\underline{P_2 = 3.5}\hspace{0.05cm}.$$ | ||
| + | For the "water level height" here holds: | ||
| + | :H=P1+σ21=P2+σ22=7.5=6.5+1=3.5+4. | ||
| − | '''(4)''' | + | '''(4)''' The channel capacity indicates the maximum mutual information. The maximum is already fixed by the best possible power sharing according to subtask '''(3)'''. For PX=10 holds: |
:$$C={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{6.5}{1} \right ) | :$$C={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{6.5}{1} \right ) | ||
+{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{3.5}{4} \right )$$ | +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{3.5}{4} \right )$$ | ||
| Line 111: | Line 112: | ||
| − | '''(5)''' | + | '''(5)''' For PX=3, with the same power splitting (P1=P2=1.5), we obtain: |
:$$I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) ={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{1.5}{1} \right ) | :$$I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) ={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{1.5}{1} \right ) | ||
+{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{1.5}{4} \right ) | +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{1.5}{4} \right ) | ||
| Line 119: | Line 120: | ||
| − | [[File: | + | [[File:EN_Inf_Z_4_7e.png|right|frame|Best possible distribution of the transmit power PX=3]] |
| − | '''(6)''' | + | '''(6)''' According to the water–filling algorithm, the total transmission power PX=3 is now fully allocated to the first channel: |
:$${P_1 = 3}\hspace{0.05cm}, | :$${P_1 = 3}\hspace{0.05cm}, | ||
\hspace{0.3cm}{P_2 = 0}\hspace{0.05cm}.$$ | \hspace{0.3cm}{P_2 = 0}\hspace{0.05cm}.$$ | ||
| − | * | + | *So here for the "water level height": |
| + | :H=4=P1+σ21=P2+σ22=3+1=0+4. | ||
| + | |||
| + | *This gives for the channel capacity: | ||
:$$C ={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{3}{1} \right ) | :$$C ={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{3}{1} \right ) | ||
+{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{0}{4} \right )=1\,{\rm bit}+ 0\,{\rm bit} | +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{0}{4} \right )=1\,{\rm bit}+ 0\,{\rm bit} | ||
| Line 130: | Line 134: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | Further notes: | |
| − | * | + | *While for PX=10 the difference between even and best power splitting was only 0.03 bit, for PX=3 the difference is larger: 0.109 bit. |
| − | * | + | *For even larger PX>10 , the difference between even and best power splitting becomes even smaller. |
| − | + | For example, for PX=100 the difference is only 0.001 bit as the following calculation shows: | |
| − | * | + | *For P1=P2=50 one obtains: |
:$$I = I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) = {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{50}{1} \right ) | :$$I = I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) = {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{50}{1} \right ) | ||
+{1}/{2}\cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{50}{4} \right )= 2.836\,{\rm bit}+ 1.877\,{\rm bit} | +{1}/{2}\cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{50}{4} \right )= 2.836\,{\rm bit}+ 1.877\,{\rm bit} | ||
\hspace{0.15cm}\underline{= 4.713\,{\rm bit}} | \hspace{0.15cm}\underline{= 4.713\,{\rm bit}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *In contrast, the best possible split gives ⇒ P1=51.5, P2=48.5: |
:$$C = {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{51.5}{1} \right ) | :$$C = {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{51.5}{1} \right ) | ||
+{1}/{2}\cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{48.5}{4} \right )= 2.857\,{\rm bit}+ 1.857\,{\rm bit} | +{1}/{2}\cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{48.5}{4} \right )= 2.857\,{\rm bit}+ 1.857\,{\rm bit} | ||
| Line 150: | Line 154: | ||
| − | [[Category:Information Theory: Exercises|^4.2 AWGN | + | [[Category:Information Theory: Exercises|^4.2 AWGN and Value-Continuous Input^]] |
Latest revision as of 15:10, 7 October 2021
We consider K parallel Gaussian channels (AWGN) with different interference powers σ2k, where 1≤k≤K . The graph illustrates this constellation using K=4 as an example.
The transmission power in the individual channels is denoted by Pk, the sum of which must not exceed the specified value PX :
- P1+...+PK=K∑k=1E[X2k]≤PX.
If the random variables X1, ... , XK are Gaussian, then for the (total) mutual information between the input X and the output Y can be written:
- I(X1,...,XK;Y1,...,YK)=1/2⋅K∑k=1log2(1+Pkσ2k),Resultin"bit".
The maximum for this is the channel capacity of the total system, where the maximization refers to the division of the total power PX among the individual channels:
- CK(PX)=max
This maximization can be done with the "water–filling algorithm" shown in the above graph for K = 4.
In the present exercise, this algorithm is to be applied, assuming the following:
- Two parallel Gaussian channels ⇒ K = 2,
- normalized noise powers \sigma_1^2 = 1 and \sigma_2^2 = 4,
- normalized transmission powers P_X = 10 and P_X = 3 respectively.
Hints:
- The exercise belongs to the chapter AWGN channel capacity with continuous value input.
- Reference is made in particular to the page Parallel Gaussian Channels.
- Since the results are to be given in "bit", the logarithm to base 2 is used in the equations: \log_2.
Questions
Solution
- According to the explanations in the theory section "Water–Filling" ⇒ Proposal 2 is to be applied when unequal conditions exist.
- However, solution proposal 3 is also correct: If the channels are equally good, there is nothing to prevent all K channels from being filled with the same power ⇒ P_1 = P_2 = ... = P_K = P_X/K.
(2) For the mutual information, with equal power distribution, the following applies:
- I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) \ = \ {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{5}{1} \right ) +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{5}{4} \right )=1.292\,{\rm bit}+ 0.585\,{\rm bit} \hspace{0.15cm}\underline{= 1.877\,{\rm bit}} \hspace{0.05cm}.
(3) According to the adjacent sketch, the following must apply:
- P_2 = P_1 - (\sigma_2^2 - \sigma_1^2) = P_1 -3\hspace{0.3cm}\text{wobei }\hspace{0.3cm}P_1 + P_2 = P_X = 10
- \Rightarrow \hspace{0.3cm} P_1 + (P_1 -3) = 10\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 2 \cdot P_1 = 13 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \underline{P_1 = 6.5}\hspace{0.05cm}, \hspace{0.3cm}\underline{P_2 = 3.5}\hspace{0.05cm}.
For the "water level height" here holds:
- H= P_1 + \sigma_1^2= P_2 + \sigma_2^2 = 7.5 = 6.5+1 = 3.5+4.
(4) The channel capacity indicates the maximum mutual information. The maximum is already fixed by the best possible power sharing according to subtask (3). For P_X = 10 holds:
- C={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{6.5}{1} \right ) +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{3.5}{4} \right )
- \Rightarrow\hspace{0.3cm} C=1.453\,{\rm bit}+ 0.453\,{\rm bit} \hspace{0.15cm}\underline{= 1.906\,{\rm bit}} \hspace{0.05cm}.
(5) For P_X = 3, with the same power splitting (P_1 = P_2 =1.5), we obtain:
- I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) ={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{1.5}{1} \right ) +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{1.5}{4} \right ) \hspace{0.15cm}\underline{= 0.891\,{\rm bit}} \hspace{0.05cm}.
(6) According to the water–filling algorithm, the total transmission power P_X = 3 is now fully allocated to the first channel:
- {P_1 = 3}\hspace{0.05cm}, \hspace{0.3cm}{P_2 = 0}\hspace{0.05cm}.
- So here for the "water level height":
- H= 4= P_1 + \sigma_1^2= P_2 + \sigma_2^2= 3+1=0+4.
- This gives for the channel capacity:
- C ={1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{3}{1} \right ) +{1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{0}{4} \right )=1\,{\rm bit}+ 0\,{\rm bit} \hspace{0.15cm}\underline{= 1\,{\rm bit}} \hspace{0.05cm}.
Further notes:
- While for P_X = 10 the difference between even and best power splitting was only 0.03 bit, for P_X = 3 the difference is larger: 0.109 bit.
- For even larger P_X > 10 , the difference between even and best power splitting becomes even smaller.
For example, for P_X = 100 the difference is only 0.001 bit as the following calculation shows:
- For P_1 = P_2 = 50 one obtains:
- I = I(X_1, X_2\hspace{0.05cm};\hspace{0.05cm}Y_1, Y_2) = {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{50}{1} \right ) +{1}/{2}\cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{50}{4} \right )= 2.836\,{\rm bit}+ 1.877\,{\rm bit} \hspace{0.15cm}\underline{= 4.713\,{\rm bit}} \hspace{0.05cm}.
- In contrast, the best possible split gives ⇒ P_1 = 51.5, \ P_2 = 48.5:
- C = {1}/{2} \cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{51.5}{1} \right ) +{1}/{2}\cdot {\rm log}_2\hspace{0.05cm}\left ( 1 + \frac{48.5}{4} \right )= 2.857\,{\rm bit}+ 1.857\,{\rm bit} \hspace{0.15cm}\underline{= 4.714\,{\rm bit}} \hspace{0.05cm}.