Difference between revisions of "Aufgaben:Exercise 4.2: Triangular PDF"
From LNTwww
m (Text replacement - "[[Informationstheorie" to "[[Information_Theory") |
m (Text replacement - "value-continuous" to "continuous") |
||
| (10 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Information_Theory/Differential_Entropy |
}} | }} | ||
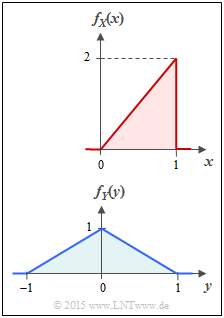
| − | [[File:P_ID2865__Inf_A_4_2.png|right|frame| | + | [[File:P_ID2865__Inf_A_4_2.png|right|frame|Two triangular PDFs]] |
| − | + | Two probability density functions $\rm (PDF)$ with triangular shapes are considered. | |
| − | * | + | * The random variable X is limited to the range from 0 to 1 , and it holds for the PDF (upper sketch): |
| − | :$$f_X(x) = \left\{ 2x0 \right. \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \hspace{0.1cm} 0 \le x \le 1 \\ {\rm | + | :$$f_X(x) = \left\{ 2x0 \right. \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \hspace{0.1cm} 0 \le x \le 1 \\ {\rm else} \\ \end{array} |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | * According to the lower sketch, the random variable Y has the following PDF: |
| − | :$$f_Y(y) = \left\{ 1−|y|0 \right. \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \hspace{0.1cm} |\hspace{0.03cm}y\hspace{0.03cm}| \le 1 \\ {\rm | + | :$$f_Y(y) = \left\{ 1−|y|0 \right. \begin{array}{*{20}c} {\rm{f\ddot{u}r}} \hspace{0.1cm} |\hspace{0.03cm}y\hspace{0.03cm}| \le 1 \\ {\rm else} \\ \end{array} |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | For both random variables, the [[Information_Theory/Differentielle_Entropie|differential entropy]] is to be determined in each case. | |
| − | + | For example, the corresponding equation for the random variable X is: | |
:$$h(X) = | :$$h(X) = | ||
\hspace{0.1cm} - \hspace{-0.45cm} \int\limits_{{\rm supp}\hspace{0.03cm}(\hspace{-0.03cm}f_X)} \hspace{-0.35cm} f_X(x) \cdot {\rm log} \hspace{0.1cm} \big [ f_X(x) \big ] \hspace{0.1cm}{\rm d}x | \hspace{0.1cm} - \hspace{-0.45cm} \int\limits_{{\rm supp}\hspace{0.03cm}(\hspace{-0.03cm}f_X)} \hspace{-0.35cm} f_X(x) \cdot {\rm log} \hspace{0.1cm} \big [ f_X(x) \big ] \hspace{0.1cm}{\rm d}x | ||
| − | \hspace{0.6cm}{\rm | + | \hspace{0.6cm}{\rm with}\hspace{0.6cm} {\rm supp}(f_X) = \{ x\text{:} \ f_X(x) > 0 \} |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *If the "natural logarithm", the pseudo-unit "nat" must be added. |
| − | * | + | *If, on the other hand, the result is asked in "bit" then the "dual logarithm" ⇒ "log2" is to be used. |
| − | In | + | In the fourth subtask, the new random variable Z=A⋅Y is considered. Here, the PDF parameter A is to be determined in such a way that the differential entropy of the new random variable Z yields exactly 1 bit :<br> |
| − | :$$h(Z) = h (A \cdot Y) = h (Y) + {\rm log}_2 \hspace{0.1cm} (A) = 1\ | + | :h(Z)=h(A⋅Y)=h(Y)+log2(A)=1 bit. |
| Line 32: | Line 32: | ||
| − | + | Hints: | |
| − | * | + | *The task belongs to the chapter [[Information_Theory/Differentielle_Entropie|Differential Entropy]]. |
| − | * | + | *Useful hints for solving this task and further information on continuous random variables can be found in the third chapter "Continuous Random Variables" of the book [[Theory of Stochastic Signals]]. |
| − | * | + | *Given the following indefinite integral: |
:$$\int \xi \cdot {\rm ln} \hspace{0.1cm} (\xi)\hspace{0.1cm}{\rm d}\xi = | :$$\int \xi \cdot {\rm ln} \hspace{0.1cm} (\xi)\hspace{0.1cm}{\rm d}\xi = | ||
\xi^2 \cdot \big [1/2 \cdot {{\rm ln} \hspace{0.1cm} (\xi)} - | \xi^2 \cdot \big [1/2 \cdot {{\rm ln} \hspace{0.1cm} (\xi)} - | ||
| Line 43: | Line 43: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the differential entropy of the random variable X in "nat". |
|type="{}"} | |type="{}"} | ||
h(X) = { -0.199--0.187 } nat | h(X) = { -0.199--0.187 } nat | ||
| − | { | + | {What result is obtained with the pseudo-unit "bit"? |
|type="{}"} | |type="{}"} | ||
h(X) = { -0.288--0.270 } bit | h(X) = { -0.288--0.270 } bit | ||
| − | { | + | {Calculate the differential entropy of the random variable Y. |
|type="{}"} | |type="{}"} | ||
h(Y) = { 0.721 3% } bit | h(Y) = { 0.721 3% } bit | ||
| − | { | + | {Determine the PDF parameter A such that h(Z)=h(A⋅Y)=1 bit_ . |
|type="{}"} | |type="{}"} | ||
A = { 1.213 3% } | A = { 1.213 3% } | ||
| Line 65: | Line 65: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' For the probability density function, in the range 0≤X≤1 , it is agreed that: |
:$$f_X(x) = 2x = C \cdot x | :$$f_X(x) = 2x = C \cdot x | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *Here we have replaced "2" by C ⇒ generalization in order to be able to use the following calculation again in subtask (3) . |
| − | * | + | *Since the differential entropy is sought in "nat", we use the natural logarithm. With the substitution ξ=C⋅x we obtain: |
:$$h_{\rm nat}(X) = \hspace{0.1cm} - \int_{0}^{1} \hspace{0.1cm} C \cdot x \cdot {\rm ln} \hspace{0.1cm} \big[ C \cdot x \big] \hspace{0.1cm}{\rm d}x = | :$$h_{\rm nat}(X) = \hspace{0.1cm} - \int_{0}^{1} \hspace{0.1cm} C \cdot x \cdot {\rm ln} \hspace{0.1cm} \big[ C \cdot x \big] \hspace{0.1cm}{\rm d}x = | ||
\hspace{0.1cm} - \hspace{0.1cm}\frac{1}{C} \cdot \int_{0}^{C} \hspace{0.1cm} \xi \cdot {\rm ln} \hspace{0.1cm} [ \xi ] \hspace{0.1cm}{\rm d}\xi | \hspace{0.1cm} - \hspace{0.1cm}\frac{1}{C} \cdot \int_{0}^{C} \hspace{0.1cm} \xi \cdot {\rm ln} \hspace{0.1cm} [ \xi ] \hspace{0.1cm}{\rm d}\xi | ||
| Line 79: | Line 79: | ||
\frac{1}{4}\right ]_{\xi = 0}^{\xi = C} | \frac{1}{4}\right ]_{\xi = 0}^{\xi = C} | ||
\hspace{0.05cm}$$ | \hspace{0.05cm}$$ | ||
| − | * | + | *Here the indefinite integral given in the front was used. After inserting the limits, considering C=2, we obtain:: |
:$$h_{\rm nat}(X) = | :$$h_{\rm nat}(X) = | ||
- C/2 \cdot | - C/2 \cdot | ||
| Line 95: | Line 95: | ||
| − | '''(2)''' | + | '''(2)''' In general: |
| + | [[File:P_ID2866__Inf_A_4_2c.png|right|frame|To calculate h(Y)]] | ||
| + | |||
:$$h_{\rm bit}(X) = \frac{h_{\rm nat}(X)}{{\rm ln} \hspace{0.1cm} (2)\,{\rm nat/bit}} = - 0.279 | :$$h_{\rm bit}(X) = \frac{h_{\rm nat}(X)}{{\rm ln} \hspace{0.1cm} (2)\,{\rm nat/bit}} = - 0.279 | ||
\hspace{0.3cm} \Rightarrow\hspace{0.3cm} | \hspace{0.3cm} \Rightarrow\hspace{0.3cm} | ||
| Line 101: | Line 103: | ||
\hspace{0.15cm}\underline {= - 0.279\,{\rm bit}} | \hspace{0.15cm}\underline {= - 0.279\,{\rm bit}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *You can save this conversion if you directly replace (1) direct "ln" by "log<sub>2</sub>" already in the analytical result of subtask: |
| + | |||
:$$h(X) = \ {\rm log}_2 \hspace{0.1cm} (\sqrt{\rm e}/2)\hspace{0.05cm}, \hspace{1.3cm} | :$$h(X) = \ {\rm log}_2 \hspace{0.1cm} (\sqrt{\rm e}/2)\hspace{0.05cm}, \hspace{1.3cm} | ||
| − | {\rm | + | {\rm pseudo-unit\hspace{-0.1cm}:\hspace{0.15cm} bit} |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
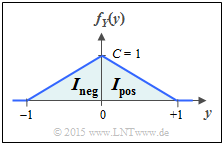
| − | + | '''(3)''' We again use the natural logarithm and divide the integral into two partial integrals: | |
| − | '''(3)''' | ||
:$$h(Y) = | :$$h(Y) = | ||
\hspace{0.1cm} - \hspace{-0.45cm} \int\limits_{{\rm supp} | \hspace{0.1cm} - \hspace{-0.45cm} \int\limits_{{\rm supp} | ||
| Line 115: | Line 117: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *The first integral for the range −1≤y≤0 is identical in form to that of subtask (1) and only shifted with respect to it, which does not affect the result. |
| − | * | + | *Now the height C=1 instead of C=2 has to be considered: |
:$$I_{\rm neg} =- C/2 \cdot | :$$I_{\rm neg} =- C/2 \cdot | ||
\big [ {\rm ln} \hspace{0.1cm} (C) - 1/2 | \big [ {\rm ln} \hspace{0.1cm} (C) - 1/2 | ||
| Line 124: | Line 126: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *The second integrand is identical to the first except for a shift and reflection. Moreover, the integration intervals do not overlap ⇒ Ipos=Ineg: |
:$$h_{\rm nat}(Y) = 2 \cdot I_{\rm neg} = 1/2 \cdot | :$$h_{\rm nat}(Y) = 2 \cdot I_{\rm neg} = 1/2 \cdot | ||
{\rm ln} \hspace{0.1cm} ({\rm e}) = {\rm ln} \hspace{0.1cm} (\sqrt{\rm e}) \hspace{0.3cm} | {\rm ln} \hspace{0.1cm} ({\rm e}) = {\rm ln} \hspace{0.1cm} (\sqrt{\rm e}) \hspace{0.3cm} | ||
| Line 133: | Line 135: | ||
| − | '''(4)''' | + | '''(4)''' For the differential entropy of the random variable Z=A⋅Y holds in general: |
:h(Z)=h(A⋅Y)=h(Y)+log2(A). | :h(Z)=h(A⋅Y)=h(Y)+log2(A). | ||
| − | * | + | *Thus, from the requiremen h(Z)=1 bit and the result of subtask (3) follows: |
:$${\rm log}_2 \hspace{0.1cm} (A) = 1\,{\rm bit} - 0.721 \,{\rm bit} = 0.279 \,{\rm bit} | :$${\rm log}_2 \hspace{0.1cm} (A) = 1\,{\rm bit} - 0.721 \,{\rm bit} = 0.279 \,{\rm bit} | ||
\hspace{0.3cm} \Rightarrow\hspace{0.3cm} A = 2^{0.279}\hspace{0.15cm}\underline | \hspace{0.3cm} \Rightarrow\hspace{0.3cm} A = 2^{0.279}\hspace{0.15cm}\underline | ||
| Line 144: | Line 146: | ||
| − | [[Category: | + | [[Category:Information Theory: Exercises|^4.1 Differential Entropy^]] |
Latest revision as of 10:27, 11 October 2021
Two probability density functions (PDF) with triangular shapes are considered.
- The random variable X is limited to the range from 0 to 1 , and it holds for the PDF (upper sketch):
- fX(x)={2x0f¨ur0≤x≤1else.
- According to the lower sketch, the random variable Y has the following PDF:
- fY(y)={1−|y|0f¨ur|y|≤1else.
For both random variables, the differential entropy is to be determined in each case.
For example, the corresponding equation for the random variable X is:
- h(X)=−∫supp(fX)fX(x)⋅log[fX(x)]dxwithsupp(fX)={x: fX(x)>0}.
- If the "natural logarithm", the pseudo-unit "nat" must be added.
- If, on the other hand, the result is asked in "bit" then the "dual logarithm" ⇒ "log2" is to be used.
In the fourth subtask, the new random variable Z=A⋅Y is considered. Here, the PDF parameter A is to be determined in such a way that the differential entropy of the new random variable Z yields exactly 1 bit :
- h(Z)=h(A⋅Y)=h(Y)+log2(A)=1 bit.
Hints:
- The task belongs to the chapter Differential Entropy.
- Useful hints for solving this task and further information on continuous random variables can be found in the third chapter "Continuous Random Variables" of the book Theory of Stochastic Signals.
- Given the following indefinite integral:
- ∫ξ⋅ln(ξ)dξ=ξ2⋅[1/2⋅ln(ξ)−1/4].
Questions
Solution
(1) For the probability density function, in the range 0≤X≤1 , it is agreed that:
- fX(x)=2x=C⋅x.
- Here we have replaced "2" by C ⇒ generalization in order to be able to use the following calculation again in subtask (3) .
- Since the differential entropy is sought in "nat", we use the natural logarithm. With the substitution ξ=C⋅x we obtain:
- hnat(X)=−∫10C⋅x⋅ln[C⋅x]dx=−1C⋅∫C0ξ⋅ln[ξ]dξ=−ξ2C⋅[ln(ξ)2−14]ξ=Cξ=0
- Here the indefinite integral given in the front was used. After inserting the limits, considering C=2, we obtain::
- hnat(X)=−C/2⋅[ln(C)−1/2]=−ln(2)+1/2=−ln(2)+1/2⋅ln(e)=ln(√e/2)=−0.193⇒h(X)=−0.193nat_.
(2) In general:
- hbit(X)=hnat(X)ln(2)nat/bit=−0.279⇒h(X)=−0.279bit_.
- You can save this conversion if you directly replace (1) direct "ln" by "log2" already in the analytical result of subtask:
- h(X)= log2(√e/2),pseudo−unit:bit.
(3) We again use the natural logarithm and divide the integral into two partial integrals:
- h(Y)=−∫supp(fY)fY(y)⋅ln[fY(y)]dy=Ineg+Ipos.
- The first integral for the range −1≤y≤0 is identical in form to that of subtask (1) and only shifted with respect to it, which does not affect the result.
- Now the height C=1 instead of C=2 has to be considered:
- Ineg=−C/2⋅[ln(C)−1/2]=−1/2⋅[ln(1)−1/2⋅ln(e)]=1/4⋅ln(e).
- The second integrand is identical to the first except for a shift and reflection. Moreover, the integration intervals do not overlap ⇒ Ipos=Ineg:
- hnat(Y)=2⋅Ineg=1/2⋅ln(e)=ln(√e)⇒hbit(Y)=log2(√e)⇒h(Y)=log2(1.649)=0.721bit_.
(4) For the differential entropy of the random variable Z=A⋅Y holds in general:
- h(Z)=h(A⋅Y)=h(Y)+log2(A).
- Thus, from the requiremen h(Z)=1 bit and the result of subtask (3) follows:
- log2(A)=1bit−0.721bit=0.279bit⇒A=20.279=1.213_.