Difference between revisions of "Aufgaben:Exercise 1.2Z: Sets of Digits"
From LNTwww
| (3 intermediate revisions by 2 users not shown) | |||
| Line 2: | Line 2: | ||
{{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Set_Theory_Basics}} | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Set_Theory_Basics}} | ||
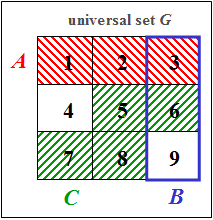
| − | [[File:EN_Sto_Z_1_2_neu.png|right|frame|Sets of digits A, B, C]] | + | [[File:EN_Sto_Z_1_2_neu.png|right|frame|Sets of digits: A, B, C]] |
Let the universal set G be the set of all digits between 1 and 9. Given are the following subsets: | Let the universal set G be the set of all digits between 1 and 9. Given are the following subsets: | ||
:A=[digits⩽ | :A = \big[\text{digits} \leqslant 3\big], | ||
: B = \big[\text{digits divisible by 3}\big], | : B = \big[\text{digits divisible by 3}\big], | ||
| − | :$$ C = \big[\text{digits 5, 6, 7, 8}\big] | + | :$$ C = \big[\text{digits 5, 6, 7, 8}\big].$$ |
| − | Besides these, let other sets be defined: | + | Besides these, let other sets be defined: |
:D = (A \cap \overline B) \cup (\overline A \cap B), | :D = (A \cap \overline B) \cup (\overline A \cap B), | ||
:E = (A \cup B) \cap (\overline A \cup \overline B), | :E = (A \cup B) \cap (\overline A \cup \overline B), | ||
:F = (A \cup C) \cap \overline B, | :F = (A \cup C) \cap \overline B, | ||
| − | :$$ | + | :$$H = (\overline A \cap \overline C) \cup (A \cap B \cap C).$$ |
First consider which digits belong to the sets D, E, F and H and then answer the following questions. <br>Justify your answers in terms of set theory. | First consider which digits belong to the sets D, E, F and H and then answer the following questions. <br>Justify your answers in terms of set theory. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| Line 25: | Line 21: | ||
Hints: | Hints: | ||
| − | *The task belongs to the chapter [[Theory_of_Stochastic_Signals/Set_Theory_Basics|Set | + | *The task belongs to the chapter [[Theory_of_Stochastic_Signals/Set_Theory_Basics|Set Theory Basics]]. |
| − | *The topic of this chapter is illustrated with examples in the (German language) learning video[[Mengentheoretische_Begriffe_und_Gesetzmäßigkeiten_(Lernvideo)|Mengentheoretische Begriffe und Gesetzmäßigkeiten]] \Rightarrow. | + | *The topic of this chapter is illustrated with examples in the (German language) learning video |
| + | |||
| + | :[[Mengentheoretische_Begriffe_und_Gesetzmäßigkeiten_(Lernvideo)|Mengentheoretische Begriffe und Gesetzmäßigkeiten]] $\Rightarrow$ "Set Theoretical Concepts and Laws". | ||
| Line 44: | Line 42: | ||
+ The complementary set to A \cap B \cap C gives the universal set G. | + The complementary set to A \cap B \cap C gives the universal set G. | ||
| − | {Which of the following statements | + | {Which of the following statements are correct? |
|type="[]"} | |type="[]"} | ||
+ The complementary sets of D and E are identical. | + The complementary sets of D and E are identical. | ||
| − | + F is a subset of the complementary set of B. | + | + F is a subset of the complementary set of B. |
- The sets B, C and D form a complete system. | - The sets B, C and D form a complete system. | ||
+ The sets A, C and H form a complete system. | + The sets A, C and H form a complete system. | ||
| Line 63: | Line 61: | ||
: E = (A \cup B) \cap (\overline A \cup \overline B) = (A \cap \overline A) \cup (A \cap \overline B) \cup (\overline A \cap B) \cup (\overline A \cap \overline B) = (A \cap \overline B) \cup (\overline A \cap B) = D = \{1, 2, 6, 9\}, | : E = (A \cup B) \cap (\overline A \cup \overline B) = (A \cap \overline A) \cup (A \cap \overline B) \cup (\overline A \cap B) \cup (\overline A \cap \overline B) = (A \cap \overline B) \cup (\overline A \cap B) = D = \{1, 2, 6, 9\}, | ||
| − | :$$F = (A \cup C | + | :$$F = (A \cup C) \cap \overline B = \{1, 2, 3, 5, 6, 7, 8\} \cap \{1, 2, 4, 5, 7, 8\} = \{1, 2, 5, 7, 8\},$$ |
:H = (\bar A \cap \overline C) \cup (A \cap B \cap C) = (\overline A \cap \overline C) \cup \phi = \{4, 9\}. | :H = (\bar A \cap \overline C) \cup (A \cap B \cap C) = (\overline A \cap \overline C) \cup \phi = \{4, 9\}. | ||
| − | '''(1)''' Only the <u>proposed solution 2</u> is correct: | + | '''(1)''' Only the <u>proposed solution 2</u> is correct: |
* A and C have no common element. | * A and C have no common element. | ||
* A and B each contain a 3. | * A and B each contain a 3. | ||
| Line 74: | Line 72: | ||
| − | '''(2)''' Correct is the <u>proposed solution 2</u>: | + | '''(2)''' Correct is the <u>proposed solution 2</u>: |
*No digit is contained in A, B and C at the same time ⇒ A \cap B \cap C = \phi ⇒ \overline{A \cap B \cap C} = \overline{\phi} = G. | *No digit is contained in A, B and C at the same time ⇒ A \cap B \cap C = \phi ⇒ \overline{A \cap B \cap C} = \overline{\phi} = G. | ||
| − | *The first proposition, on the other hand, is wrong. It is missing a 4. | + | *The first proposition, on the other hand, is wrong. It is missing a 4. |
| − | '''(3)''' Correct are the <u>proposed | + | '''(3)''' Correct are the <u>proposed solutions 1, 2 and 4</u>: |
*The first proposal is correct: The sets D and E contain exactly the same elements and thus also their complementary sets. | *The first proposal is correct: The sets D and E contain exactly the same elements and thus also their complementary sets. | ||
| − | *The second proposal is also correct: In general, i.e. for any X and B the following holds: X \cap \overline B \subset \overline B \ \Rightarrow With X = A \cup C it follows that F \subset \overline B. | + | *The second proposal is also correct: In general, i.e. for any X and B the following holds: $(X \cap \overline B) \subset \overline B \ \Rightarrow With X = A \cup C it follows that F \subset \overline B$. |
| − | *The last proposal is also correct: A = \{1, 2, 3\}, C = \{5, 6, 7, 8\} and H = \{4, 9\} form a "complete system". | + | *The last proposal is also correct: A = \{1, 2, 3\}, C = \{5, 6, 7, 8\} and H = \{4, 9\} form a "complete system". |
| − | *The third suggestion, on the other hand, is wrong because B and C are not disjoint. | + | *The third suggestion, on the other hand, is wrong because B and C are not disjoint. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Latest revision as of 15:13, 25 November 2021
Let the universal set G be the set of all digits between 1 and 9. Given are the following subsets:
- A = \big[\text{digits} \leqslant 3\big],
- B = \big[\text{digits divisible by 3}\big],
- C = \big[\text{digits 5, 6, 7, 8}\big].
Besides these, let other sets be defined:
- D = (A \cap \overline B) \cup (\overline A \cap B),
- E = (A \cup B) \cap (\overline A \cup \overline B),
- F = (A \cup C) \cap \overline B,
- H = (\overline A \cap \overline C) \cup (A \cap B \cap C).
First consider which digits belong to the sets D, E, F and H and then answer the following questions.
Justify your answers in terms of set theory.
Hints:
- The task belongs to the chapter Set Theory Basics.
- The topic of this chapter is illustrated with examples in the (German language) learning video
- Mengentheoretische Begriffe und Gesetzmäßigkeiten \Rightarrow "Set Theoretical Concepts and Laws".
Questions
Solution
For the other sets defined in the problem holds:
- D = (A \cap \overline B) \cup (\overline A \cap B) =\big[\{1, 2, 3\} \cap \{1, 2, 4, 5, 7, 8\}\big] \cup \big[\{4, 5, 6, 7, 8, 9\} \cap \{3, 6, 9\}\big] = \{1, 2, 6, 9\},
- E = (A \cup B) \cap (\overline A \cup \overline B) = (A \cap \overline A) \cup (A \cap \overline B) \cup (\overline A \cap B) \cup (\overline A \cap \overline B) = (A \cap \overline B) \cup (\overline A \cap B) = D = \{1, 2, 6, 9\},
- F = (A \cup C) \cap \overline B = \{1, 2, 3, 5, 6, 7, 8\} \cap \{1, 2, 4, 5, 7, 8\} = \{1, 2, 5, 7, 8\},
- H = (\bar A \cap \overline C) \cup (A \cap B \cap C) = (\overline A \cap \overline C) \cup \phi = \{4, 9\}.
(1) Only the proposed solution 2 is correct:
- A and C have no common element.
- A and B each contain a 3.
- B and C each contain a 6.
(2) Correct is the proposed solution 2:
- No digit is contained in A, B and C at the same time ⇒ A \cap B \cap C = \phi ⇒ \overline{A \cap B \cap C} = \overline{\phi} = G.
- The first proposition, on the other hand, is wrong. It is missing a 4.
(3) Correct are the proposed solutions 1, 2 and 4:
- The first proposal is correct: The sets D and E contain exactly the same elements and thus also their complementary sets.
- The second proposal is also correct: In general, i.e. for any X and B the following holds: (X \cap \overline B) \subset \overline B \ \Rightarrow With X = A \cup C it follows that F \subset \overline B.
- The last proposal is also correct: A = \{1, 2, 3\}, C = \{5, 6, 7, 8\} and H = \{4, 9\} form a "complete system".
- The third suggestion, on the other hand, is wrong because B and C are not disjoint.