Difference between revisions of "Aufgaben:Exercise 1.4Z: Sum of Ternary Quantities"
From LNTwww
| (7 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Statistical_Dependence_and_Independence}} |
| − | [[File:P_ID79__Sto_Z_1_4.png|right|frame| | + | [[File:P_ID79__Sto_Z_1_4.png|right|frame|Sum of two ternary variables $x$ and $y$]] |
| − | + | Let be given the ternary random variables | |
:$$x ∈ {–2, \ 0, +2},$$ | :$$x ∈ {–2, \ 0, +2},$$ | ||
| − | |||
:$$y ∈ {–1, \ 0, +1}.$$ | :$$y ∈ {–1, \ 0, +1}.$$ | ||
| − | + | *These two ternary values each occur with equal probability. | |
| − | + | *From this, the sum $s = x + y$ is formed as a new random variable. | |
| − | + | *The adjacent scheme shows that the sum $s$ can take all integer values between $–3$ and $+3$ : | |
| − | |||
:$$ s \in \{-3, -2, -1, \ 0, +1, +2, +3\}.$$ | :$$ s \in \{-3, -2, -1, \ 0, +1, +2, +3\}.$$ | ||
| Line 20: | Line 18: | ||
| − | + | Hints: | |
| − | + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Statistical_Dependence_and_Independence|Statistical dependence and independence]]. | |
| − | * | ||
| − | * | + | *The topic of this chapter is illustrated with examples in the (German language) learning video |
| − | ::[[Statistische_Abhängigkeit_und_Unabhängigkeit_(Lernvideo)|Statistische Abhängigkeit und Unabhängigkeit]]. | + | ::[[Statistische_Abhängigkeit_und_Unabhängigkeit_(Lernvideo)|Statistische Abhängigkeit und Unabhängigkeit]] $\Rightarrow$ "Statistical dependence and independence". |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the probability that the sum $s$ is positive: |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(s>0) \ = \ $ { 0.4444 3% } | ${\rm Pr}(s>0) \ = \ $ { 0.4444 3% } | ||
| − | { | + | {Calculate the probability that both the input $x$ and the sum $s$ are positive: |
|type="{}"} | |type="{}"} | ||
${\rm Pr}\big [(x>0) \cap (s>0)\big] \ = \ $ { 0.3333 3% } | ${\rm Pr}\big [(x>0) \cap (s>0)\big] \ = \ $ { 0.3333 3% } | ||
| − | { | + | {Calculate the conditional probability that the input variable $x > 0$, when $s > 0$ holds: |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(x>0\hspace{0.05cm}|\hspace{0.05cm}s>0)\ = \ $ { 0.75 3% } | ${\rm Pr}(x>0\hspace{0.05cm}|\hspace{0.05cm}s>0)\ = \ $ { 0.75 3% } | ||
| − | { | + | {Calculate the conditional probability that the sum $s$ is positive, when the input variable is $x > 0$ : |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(s>0\hspace{0.05cm}|\hspace{0.05cm}x>0)\ = \ $ { 1 } | ${\rm Pr}(s>0\hspace{0.05cm}|\hspace{0.05cm}x>0)\ = \ $ { 1 } | ||
| Line 50: | Line 47: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | [[File:P_ID99__Sto_Z_1_4_a.png|right|frame| | + | [[File:P_ID99__Sto_Z_1_4_a.png|right|frame|Ternary variables in the Venn diagram]] |
| − | In | + | In the adjacent graph |
| − | * | + | *the three fields belonging to the event $\big[x > 0\big]$ are outlined in purple, |
| − | * | + | *the fields for $\big[ s > 0\big]$ are highlighted in yellow. |
| − | + | All sought probabilities can be determined here with the help of the classical definition. | |
<br><br> | <br><br> | ||
| − | '''(1)''' | + | '''(1)''' This event is marked by the fields with yellow background: |
:$$\rm Pr (\it s > \rm 0) = \rm 4/9 \hspace{0.15cm}\underline { \approx \rm 0.444}.$$ | :$$\rm Pr (\it s > \rm 0) = \rm 4/9 \hspace{0.15cm}\underline { \approx \rm 0.444}.$$ | ||
| − | '''(2)''' | + | '''(2)''' The following facts hold here: |
:$$\rm Pr \big[(\it x > \rm 0) \cap (\it s>\rm 0) \big ] = \rm Pr(\it x > \rm 0) =\rm 3/9\hspace{0.15cm}\underline { \approx \rm 0.333}. $$ | :$$\rm Pr \big[(\it x > \rm 0) \cap (\it s>\rm 0) \big ] = \rm Pr(\it x > \rm 0) =\rm 3/9\hspace{0.15cm}\underline { \approx \rm 0.333}. $$ | ||
| − | '''(3)''' | + | '''(3)''' Using the results of subtasks '''(1)''' and '''(2)''', it follows: |
:$$\rm Pr \big[(\it x > \rm 0) \hspace{0.05cm}| \hspace{0.05cm} (\it s > \rm 0)\big] = \frac{{\rm Pr} [(\it x > \rm 0) \cap (\it s > \rm 0)]}{{\rm Pr}(\it s > \rm 0)}= \frac{3/9}{4/9}\hspace{0.15cm}\underline {= 0.75}.$$ | :$$\rm Pr \big[(\it x > \rm 0) \hspace{0.05cm}| \hspace{0.05cm} (\it s > \rm 0)\big] = \frac{{\rm Pr} [(\it x > \rm 0) \cap (\it s > \rm 0)]}{{\rm Pr}(\it s > \rm 0)}= \frac{3/9}{4/9}\hspace{0.15cm}\underline {= 0.75}.$$ | ||
| − | '''(4)''' | + | '''(4)''' Analogous to subtask '''(3)''' now holds: |
:$$\rm Pr(\it s > \rm 0 \hspace{0.05cm} | \hspace{0.05cm} \it x > \rm 0)=\frac{Pr \big[(\it x > \rm 0) \cap (\it s > \rm 0) \big]}{Pr(\it x >\rm 0)}=\rm \frac{3/9}{3/9}\hspace{0.15cm}\underline {= 1}.$$ | :$$\rm Pr(\it s > \rm 0 \hspace{0.05cm} | \hspace{0.05cm} \it x > \rm 0)=\frac{Pr \big[(\it x > \rm 0) \cap (\it s > \rm 0) \big]}{Pr(\it x >\rm 0)}=\rm \frac{3/9}{3/9}\hspace{0.15cm}\underline {= 1}.$$ | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 81: | Line 78: | ||
| − | [[Category: | + | [[Category:Theory of Stochastic Signals: Exercises|^1.3 Statistical Dependence/Independence^]] |
Latest revision as of 15:41, 30 November 2021
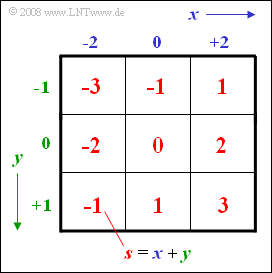
Let be given the ternary random variables
- $$x ∈ {–2, \ 0, +2},$$
- $$y ∈ {–1, \ 0, +1}.$$
- These two ternary values each occur with equal probability.
- From this, the sum $s = x + y$ is formed as a new random variable.
- The adjacent scheme shows that the sum $s$ can take all integer values between $–3$ and $+3$ :
- $$ s \in \{-3, -2, -1, \ 0, +1, +2, +3\}.$$
Hints:
- The exercise belongs to the chapter Statistical dependence and independence.
- The topic of this chapter is illustrated with examples in the (German language) learning video
- Statistische Abhängigkeit und Unabhängigkeit $\Rightarrow$ "Statistical dependence and independence".
Questions
Solution
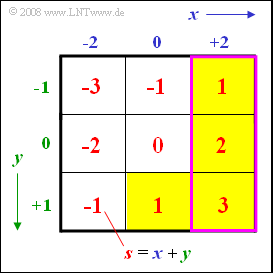
In the adjacent graph
- the three fields belonging to the event $\big[x > 0\big]$ are outlined in purple,
- the fields for $\big[ s > 0\big]$ are highlighted in yellow.
All sought probabilities can be determined here with the help of the classical definition.
(1) This event is marked by the fields with yellow background:
- $$\rm Pr (\it s > \rm 0) = \rm 4/9 \hspace{0.15cm}\underline { \approx \rm 0.444}.$$
(2) The following facts hold here:
- $$\rm Pr \big[(\it x > \rm 0) \cap (\it s>\rm 0) \big ] = \rm Pr(\it x > \rm 0) =\rm 3/9\hspace{0.15cm}\underline { \approx \rm 0.333}. $$
(3) Using the results of subtasks (1) and (2), it follows:
- $$\rm Pr \big[(\it x > \rm 0) \hspace{0.05cm}| \hspace{0.05cm} (\it s > \rm 0)\big] = \frac{{\rm Pr} [(\it x > \rm 0) \cap (\it s > \rm 0)]}{{\rm Pr}(\it s > \rm 0)}= \frac{3/9}{4/9}\hspace{0.15cm}\underline {= 0.75}.$$
(4) Analogous to subtask (3) now holds:
- $$\rm Pr(\it s > \rm 0 \hspace{0.05cm} | \hspace{0.05cm} \it x > \rm 0)=\frac{Pr \big[(\it x > \rm 0) \cap (\it s > \rm 0) \big]}{Pr(\it x >\rm 0)}=\rm \frac{3/9}{3/9}\hspace{0.15cm}\underline {= 1}.$$