Difference between revisions of "Aufgaben:Exercise 2.3: Algebraic Sum of Binary Numbers"
From LNTwww
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Binomial_Distribution |
}} | }} | ||
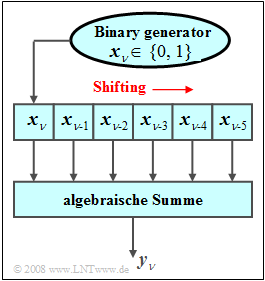
| − | [[File:EN_Sto_A_2_3.png|right|frame| | + | [[File:EN_Sto_A_2_3.png|right|frame|Considered random generator]] |
| − | + | A random number generator outputs a binary random number $x_\nu$ at each clock time $(\nu)$ , which can be $0$ or $1$ . | |
| − | * | + | *The value "1" occurs with probability $p = 0.25$ . |
| − | * | + | *The individual values $x_\nu$ are statistically independent of each other. |
| − | + | The binary numbers are stored in a shift register withnbsp; $I = 6$ memory cells. | |
| − | + | At each clock instant, the contents of this shift register are shifted one place to the right and the algebraic sum $y_\nu$ of the shift register contents is formed in each case: | |
:$$y_{\nu}=\sum\limits_{i=0}^{5}x_{\nu-i}=x_{\nu}+x_{\nu-1}+\ \text{...} \ +x_{\nu-5}.$$ | :$$y_{\nu}=\sum\limits_{i=0}^{5}x_{\nu-i}=x_{\nu}+x_{\nu-1}+\ \text{...} \ +x_{\nu-5}.$$ | ||
| Line 20: | Line 20: | ||
| − | + | Hints: | |
| − | * | + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Binomial_Distribution|binomial distribution]]. |
| − | * | + | *To check your results you can use the interactive applet [[Applets:Binomial-_und_Poissonverteilung_(Applet)|Binomial and Poisson distribution]] . |
| Line 29: | Line 29: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What values can the sum $y$ take? What is the largest possible value? |
|type="{}"} | |type="{}"} | ||
$y_\max \ = \ $ { 6 3% } | $y_\max \ = \ $ { 6 3% } | ||
| − | { | + | {Calculate the probability that $y$ is greater than $2$ . |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(y > 2) \ = \ $ { 0.169 3% } | ${\rm Pr}(y > 2) \ = \ $ { 0.169 3% } | ||
| − | { | + | {What is the mean value of the random variable $y$ ? |
|type="{}"} | |type="{}"} | ||
$m_y \ =$ { 1.5 3% } | $m_y \ =$ { 1.5 3% } | ||
| − | { | + | {Find the ***standard deviation*** of the random variable $y$. |
|type="{}"} | |type="{}"} | ||
$\sigma_y \ = \ $ { 1.061 3% } | $\sigma_y \ = \ $ { 1.061 3% } | ||
| − | { | + | {Are the random numbers $y_\nu$ statistically independent? Justify your result. |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The random numbers are statistically independent. |
| − | + | + | + The random numbers are statistically dependent. |
| − | { | + | {What is the conditional probability that $y_\nu$ equals $\mu$ again if $y_{\nu-1} = \mu$ occured previously? $(\mu = 0, \ 1, \ \text{...} \ , \ I)$. |
|type="{}"} | |type="{}"} | ||
${\rm Pr}(y_\nu = \mu \hspace{0.05cm} | \hspace{0.05cm} y_{\nu-1} = \mu ) \ = \ $ { 0.625 3% } | ${\rm Pr}(y_\nu = \mu \hspace{0.05cm} | \hspace{0.05cm} y_{\nu-1} = \mu ) \ = \ $ { 0.625 3% } | ||
| Line 65: | Line 65: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
'''(1)''' In jeder Zelle kann eine $0$ oder eine $1$ stehen. Deshalb kann die Summe alle ganzzahligen Werte zwischen $0$ und $6$ annehmen: | '''(1)''' In jeder Zelle kann eine $0$ oder eine $1$ stehen. Deshalb kann die Summe alle ganzzahligen Werte zwischen $0$ und $6$ annehmen: | ||
Revision as of 13:03, 10 December 2021
Considered random generator
A random number generator outputs a binary random number $x_\nu$ at each clock time $(\nu)$ , which can be $0$ or $1$ .
- The value "1" occurs with probability $p = 0.25$ .
- The individual values $x_\nu$ are statistically independent of each other.
The binary numbers are stored in a shift register withnbsp; $I = 6$ memory cells.
At each clock instant, the contents of this shift register are shifted one place to the right and the algebraic sum $y_\nu$ of the shift register contents is formed in each case:
- $$y_{\nu}=\sum\limits_{i=0}^{5}x_{\nu-i}=x_{\nu}+x_{\nu-1}+\ \text{...} \ +x_{\nu-5}.$$
Hints:
- The exercise belongs to the chapter binomial distribution.
- To check your results you can use the interactive applet Binomial and Poisson distribution .
Questions
Solution
(1) In jeder Zelle kann eine $0$ oder eine $1$ stehen. Deshalb kann die Summe alle ganzzahligen Werte zwischen $0$ und $6$ annehmen:
- $$y_{\nu}\in\{0,1,\ \text{...} \ ,6\}\hspace{0.3cm}\Rightarrow\hspace{0.3cm} y_{\rm max} \hspace{0.15cm} \underline{= 6}.$$
(2) Es liegt eine Binomialverteilung vor. Daher gilt mit $p = 0.25$:
- $${\rm Pr}(y =0)=(1-p)^{\it I}=0.75^6=0.178,$$
- $${\rm Pr}(y=1)=\left({ I \atop {1}}\right)\cdot (1-p)^{I-1}\cdot p= \rm 6\cdot 0.75^5\cdot 0.25=0.356,$$
- $${\rm Pr}(y=2)=\left({ I \atop { 2}}\right)\cdot (1-p)^{I-2}\cdot p^{\rm 2}= \rm 15\cdot 0.75^4\cdot 0.25^2=0.297,$$
- $$\Rightarrow \hspace{0.3cm}{\rm Pr}(y>2)=1-{\rm Pr}(y=0)-{\rm Pr}( y=1)-{\rm Pr}( y=2)\hspace{0.15cm} \underline{=\rm 0.169}.$$
(3) Nach der allgemeinen Gleichung gilt für den Mittelwert der Binomialverteilung:
- $$m_y= I\cdot p\hspace{0.15cm} \underline{=\rm 1.5}.$$
(4) Entsprechend gilt für die Streuung der Binomialverteilung:
- $$\sigma_y=\sqrt{ I \cdot p \cdot( 1- p)} \hspace{0.15cm} \underline{= \rm 1.061}.$$
(5) Richtig ist der Lösungsvorschlag 2:
- Ist $y_\nu = 0$, so können zum nächsten Zeitpunkt nur die Werte $0$ und $1$ folgen, nicht aber $2$, ... , $6$.
- Das heißt: Die Folge $ \langle y_\nu \rangle$ weist (starke) statistische Bindungen auf.
(6) Die gesuchte Wahrscheinlichkeit ist identisch mit der Wahrscheinlichkeit dafür, dass das neue Binärsymbol gleich dem aus dem Schieberegister herausgefallenen Symbol ist. Daraus folgt:
- $${\rm Pr} (y_{\nu} = \mu\hspace{0.05cm}| \hspace{0.05cm} y_{\nu-{1}} = \mu) = {\rm Pr}(x_{\nu}= x_{\nu-6}). $$
- Da die Symbole $x_\nu$ statistisch voneinander unabhängig sind, kann hierfür auch geschrieben werden:
- $${\rm Pr}(x_{\nu} = x_{\nu-6}) = {\rm Pr}\big[(x_{\nu}= 1)\hspace{0.05cm}\cap\hspace{0.05cm}(x_{\nu-6}= 1)\hspace{0.05cm}\cup \hspace{0.05cm}(x_\nu=0)\hspace{0.05cm}\cap\hspace{0.05cm}(x_{\nu-6} =0)\big]= p^{2}+(1- p)^{2}=\rm 0.25^2 + 0.75^2\hspace{0.15cm} \underline{ = 0.625}. $$
