Aufgaben:Exercise 2.10: SSB-AM with Channel Distortions

• Return to book

Transmission spectrum of the analytical signal and channel frequency response

Let us consider the transmission of the source signal

$$q(t) = 2\,{\rm V} \cdot \cos(2 \pi f_2 t) + 2\,{\rm V} \cdot \cos(2 \pi f_4 t)$$

over a Gaussian bandpass channel with center frequency  $f_{\rm M} = 48 \ \rm kHz$.  This is different from the carrier frequency  $f_{\rm T} = 50 \ \rm kHz$ used in modulation.  The frequencies  $f_2$  and  $f_4$  stand fro  $f = 2 \ \rm kHz$  und  $f = 4 \ \rm kHz$, respectively.

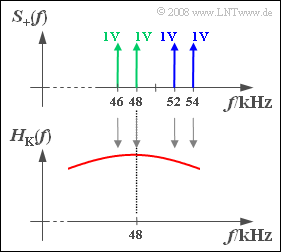
We will now investigate the following modulation methods with respect to the spectrum  $S_+(f)$  of the analytical signal as shown in the upper graph:

• DSB–AM  $($all four spectral lines at  $46 \ \rm kHz$,  $48 \ \rm kHz$,  $52 \ \rm kHz$  and  $54 \ \rm kHz)$,
• USB–AM  $($only blue spectral lines at  $52 \ \rm kHz$  and  $54 \ \rm kHz)$,
• LSB–AM  $($only green spectral lines at  $46 \ \rm kHz$  and  $48 \ \rm kHz)$.

In each case, a synchronous demodulator is used to first convert the receiver-side carrier signal

$$ z_{\rm E} (t) = \left\{ \begin{array}{c} 2 \cdot z(t) \\ 4 \cdot z(t) \\ \end{array} \right.\quad \begin{array}{*{10}c} {\rm{bei}} \\ {\rm{with}} \\ \end{array}\begin{array}{*{20}c} {\rm ZSB} \hspace{0.05cm}, \\ {\rm USB, LSB} \hspace{0.05cm} \\ \end{array}$$

by multiplication and then completely suppresses the components by twice the carrier frequency. Thus, with an ideal channel  $H_{\rm K}(f) = 1$ ,  $v(t) = q(t)$  would hold in all cases.

The Gaussian channel considered here is given by the following auxiliary values:

$$ H_{\rm K}(f = 46\ {\rm kHz}) = 0.968,\hspace{0.3cm}H_{\rm K}(f = 48\ {\rm kHz}) = 1.000,\hspace{0.3cm} H_{\rm K}(f = 52\ {\rm kHz}) = 0.882,\hspace{0.3cm}H_{\rm K}(f = 54\ {\rm kHz}) = 0.754\hspace{0.05cm}.$$

In each case, write the sink signal in the form.

$$v(t) = A_2 \cdot \cos(2 \pi f_2 \cdot (t - \tau_2)) + A_4 \cdot \cos(2 \pi f_4 \cdot (t - \tau_4))\hspace{0.05cm}.$$

All calculations are to be carried out for both a perfect phase synchronization  $(Δϕ_{\rm T} = 0)$  as well as for a phase offset of  $Δϕ_{\rm T} = 30^\circ$ .  This is present, for example, if the transmit-side carrier signal is cosine-shaped and the receiver-side carrier is:

$$ z_{\rm E} (t) = A_{\rm E} \cdot \cos(\omega_{\rm T} \cdot t - 30^\circ) . $$

Hints:

• This exercise belongs to the chapter   Single-Sideband Modulation.
• Reference will also be made to the chapter nbsp; Synchronous Demodulation.

Questions

1

Calculate the amplitudes for   DSB–AM  and  perfect synchronization  $(Δϕ_{\rm T} = 0)$.

$A_2 \ = \ $ $\ \rm V$

$A_4 \ = \ $ $\ \rm V$

2

What are the values for  $A_2$  and  $τ_2$  for  DSB–AM  and a  phase offset of $(Δϕ_{\rm T} = 30^\circ)$?

$A_2 \ = \ $ $\ \rm V$

$τ_2 \hspace{0.25cm} = \ $ $\ \rm µ s$

3

Calculate the amplitudes $A_2$  and  $A_4$  for  USB–AM  and  perfect synchronization  with  $(Δϕ_{\rm T} = 0)$.

$A_2 \ = \ $ $\ \rm V$

$A_4 \ = \ $ $\ \rm V$

4

Give the signal amplitudes for   LSB–AM  and  perfect synchronization with  $(Δϕ_{\rm T} = 0)$.

$A_2 \ = \ $ $\ \rm V$

$A_4 \ = \ $ $\ \rm V$

5

In contrast, what are the signal parameters for  LSB–AM  and a  phase offset  of  $(Δϕ_{\rm T} = 30^\circ)$?

$A_2 \ = \ $ $\ \rm V$

$τ_2 \hspace{0.25cm} = \ $ $\ \rm µ s$

$A_4 \ = \ $ $\ \rm V$

$τ_4 \hspace{0.25cm} = \ $ $\ \rm µ s$

6

Which of these statements are true given your results?  Here, "channel distortions" should always be understood as attenuation distortions.

	In DSB-AM, each channel distortion leads to attenuation distortions.
	In SSB-AM, each channel distortion leads to phase distortions.
	In DSB-AM, a phase offset leads to attenuation distortions.
	In SSB-AM, a phase offset leads to phase distortions.

Solution

Solution

(1)  Bei der ZSB–AM sind folgende Dämpfungsfaktoren zu berücksichtigen:

$$\alpha_2 = {1}/{2} \cdot \left[ H_{\rm K}(f = 48\,{\rm kHz}) + H_{\rm K}(f = 52\,{\rm kHz})\right] = 0.981,$$

$$\alpha_4 = {1}{2} \cdot \left[ H_{\rm K}(f = 46\,{\rm kHz}) + H_{\rm K}(f = 54\,{\rm kHz})\right] = 0.861\hspace{0.05cm}.$$

• Damit ergeben sich die Amplituden  $A_2\hspace{0.15cm}\underline{ = 1.882 \ \rm V}$  und  $A_4\hspace{0.15cm}\underline{ = 1.722 \ \rm V}$.

(2)  Bei ZSB führt ein Phasenversatz zwischen den Trägerfrequenzen von Sender und Empfänger nur zu einer für alle Frequenzen gleichen Dämpfung:

$$A_2 = \cos (30^\circ) \cdot 1.882\,{\rm V} \hspace{0.15cm}\underline {= 1.630\,{\rm V}},$$

$$A_4 = \cos (30^\circ) \cdot 1.722\,{\rm V} = 1.491\,{\rm V}\hspace{0.05cm}.$$

• Die Laufzeiten sind  $τ_2\hspace{0.15cm}\underline {= 0}$  und  $τ_4 = 0$.

(3)  Bei OSB–AM wird der Dämpfungsfaktor  $α_2$  allein von  $H_{\rm K}(f = 52\ \rm kHz)$  bestimmt.

• Da der prinzipielle Amplitudenverlust der OSB um den Faktor  $2$  durch eine größere Trägeramplitude ausgeglichen wird, gilt:

$$A_2 = 0.882 \cdot 2\,{\rm V}\hspace{0.15cm}\underline {= 1.764\,{\rm V}},$$

$$A_4 = 0.754 \cdot 2\,{\rm V}\hspace{0.15cm}\underline {= 1.508\,{\rm V}} \hspace{0.05cm}.$$

(4)  Analog zur Lösung der Teilaufgabe  (3)  erhält man hier:

$$ A_2 = H_{\rm K}(f = 48\,{\rm kHz}) \cdot 2\,{\rm V}\hspace{0.15cm}\underline {= 2\,{\rm V}},$$

$$A_4 = H_{\rm K}(f = 46\,{\rm kHz}) \cdot 2\,{\rm V}\hspace{0.15cm}\underline {= 1.936\,{\rm V}} \hspace{0.05cm}.$$

(5)  Bei der USB–AM lautet das Empfangssignal:

$$r(t) = 1\,{\rm V} \cdot \cos( \omega_{\rm 48} \cdot t) + 0.968\,{\rm V} \cdot \cos( \omega_{\rm 46} \cdot t)\hspace{0.05cm}.$$

• Durch Multiplikation mit dem empfangsseitigen Trägersignal  $z_{\rm E}(t) = 4 \cdot \cos( \omega_{\rm 50} \cdot t - \Delta \phi_{\rm T})$  erhält man nach Anwendung des trigonometrischen Additionstheorems:

$$v(t) = r(t) \cdot z_{\rm E}(t) = \hspace{0.15cm}\underline { 2.000\,{\rm V}} \cdot \cos( \omega_{\rm 2} \cdot t - \Delta \phi_{\rm T})+\hspace{0.15cm}\underline { 1.936\,{\rm V}} \cdot \cos( \omega_{\rm 4} \cdot t - \Delta \phi_{\rm T}) + {\rm Anteile \hspace{0.15cm}um \hspace{0.15cm}} 2f_{\rm T}\hspace{0.05cm}$$

$$ \Rightarrow \hspace{0.3cm} A_2 \hspace{0.15cm}\underline {= 2\,{\rm V}},\hspace{0.5cm} A_4 \hspace{0.15cm}\underline {= 1.936\,{\rm V}}.$$

• Unter Berücksichtigung des nachfolgenden Tiefpassfilters kann hierfür auch geschrieben werden:

$$ v(t) = A_2 \cdot \cos( \omega_{\rm 2} \cdot (t - \tau_2))+ A_4 \cdot \cos( \omega_{\rm 4} \cdot (t - \tau_4))\hspace{0.05cm}.$$

• Die Amplituden sind gegenüber Teilaufgabe  (4)  unverändert.  Für die Laufzeiten erhält man mit  $Δϕ_{\rm T} = π/6$:

$$ \tau_2 = \frac {\Delta \phi_{\rm T}}{2 \pi \cdot f_2} = \frac {\pi /6}{2 \pi \cdot 2\,{\rm kHz}} \hspace{0.15cm}\underline {\approx 41.6\,{\rm µ s}},\hspace{0.5cm} \tau_4 = \frac {\Delta \phi_{\rm T}}{2 \pi \cdot f_4}= \frac {\tau_2}{2}\hspace{0.15cm}\underline {\approx 20.8\,{\rm µ s}} \hspace{0.05cm}.$$

(6)  Richtig sind der erste und der letzte Lösungsvorschlag:

• Auch bei ESB führen Dämpfungsverzerrungen auf dem Kanal ausschließlich zu Dämpfungsverzerrungen bezüglich  $v(t)$.
• Phasenverzerrungen gibt es nur bei einem Demodulator mit Phasenversatz bei eine Einseitenbandmodulation.
• Bei der ZSB–AM hätte ein solcher Phasenversatz keine Verzerrungen zur Folge, sondern nur eine frequenzunabhängige Dämpfung.
• Zu Phasenverzerrungen bezüglich  $v(t)$  kommt es bei ZSB–AM und ESB–AM auch, wenn solche bereits auf dem Kanal auftreten.