Difference between revisions of "Aufgaben:Exercise 3.10Z: Rayleigh? Or Rice?"
From LNTwww
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Further_Distributions |
}} | }} | ||
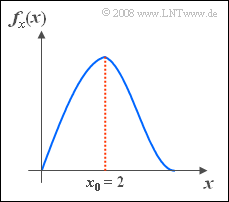
| − | [[File:P_ID149__Sto_Z_3_10.png|right|frame| | + | [[File:P_ID149__Sto_Z_3_10.png|right|frame|Does the present PDF describe Rayleigh or Rice?]] |
| − | + | The probability density function of the random variable $x$ is given as follows: | |
:$$f_x(x)=\frac{\it x}{\lambda^{2}}\cdot{\rm e}^{-x^{\rm 2}/(\lambda^{\rm 2})}.$$ | :$$f_x(x)=\frac{\it x}{\lambda^{2}}\cdot{\rm e}^{-x^{\rm 2}/(\lambda^{\rm 2})}.$$ | ||
| − | + | Correspondingly, for the associated distribution function: | |
:$$F_x(r)= {\rm Pr}(x \le r) = 1-{\rm e}^{- r^{\rm 2}/(2 \lambda^{\rm 2})}.$$ | :$$F_x(r)= {\rm Pr}(x \le r) = 1-{\rm e}^{- r^{\rm 2}/(2 \lambda^{\rm 2})}.$$ | ||
| − | * | + | *It is known that the value $x_0 = 2$ occurs most frequently. |
| − | * | + | *This also means that the PDF $f_x(x)$ is maximum at $x = x_0 $ . |
| Line 19: | Line 19: | ||
| − | + | Hints: | |
| − | + | This exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Further_Distributions|Further Distributions]]. | |
*Insbesondere wird auf die Seiten [[Theory_of_Stochastic_Signals/Weitere_Verteilungen#Rayleighverteilung|Rayleighverteilung]] und [[Theory_of_Stochastic_Signals/Weitere_Verteilungen#Riceverteilung|Riceverteilung]] Bezug genommen . | *Insbesondere wird auf die Seiten [[Theory_of_Stochastic_Signals/Weitere_Verteilungen#Rayleighverteilung|Rayleighverteilung]] und [[Theory_of_Stochastic_Signals/Weitere_Verteilungen#Riceverteilung|Riceverteilung]] Bezug genommen . | ||
| − | * | + | *You can check your results with interactive applet [[Applets:WDF,_VTF_und_Momente_spezieller_Verteilungen_(Applet)|PDF, CDF and moments of special distributions]] . |
| − | * | + | *Consider the following definite integral in the solution: |
:$$\int_{0}^{\infty}x^{\rm 2}\cdot {\rm e}^{ -x^{\rm 2}/\rm 2} \, {\rm d}x=\sqrt{{\pi}/{\rm 2}}.$$ | :$$\int_{0}^{\infty}x^{\rm 2}\cdot {\rm e}^{ -x^{\rm 2}/\rm 2} \, {\rm d}x=\sqrt{{\pi}/{\rm 2}}.$$ | ||
| Line 30: | Line 30: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
Revision as of 15:30, 12 January 2022
The probability density function of the random variable $x$ is given as follows:
- $$f_x(x)=\frac{\it x}{\lambda^{2}}\cdot{\rm e}^{-x^{\rm 2}/(\lambda^{\rm 2})}.$$
Correspondingly, for the associated distribution function:
- $$F_x(r)= {\rm Pr}(x \le r) = 1-{\rm e}^{- r^{\rm 2}/(2 \lambda^{\rm 2})}.$$
- It is known that the value $x_0 = 2$ occurs most frequently.
- This also means that the PDF $f_x(x)$ is maximum at $x = x_0 $ .
Hints: This exercise belongs to the chapter Further Distributions.
- Insbesondere wird auf die Seiten Rayleighverteilung und Riceverteilung Bezug genommen .
- You can check your results with interactive applet PDF, CDF and moments of special distributions .
- Consider the following definite integral in the solution:
- $$\int_{0}^{\infty}x^{\rm 2}\cdot {\rm e}^{ -x^{\rm 2}/\rm 2} \, {\rm d}x=\sqrt{{\pi}/{\rm 2}}.$$
Questions
Musterlösung
(1) Richtig ist allein der zweite Lösungsvorschlag.
- Aufgrund der gegebenen WDF liegt keine Riceverteilung, sondern eine Rayleighverteilung vor.
- Diese ist um den Mittelwert $m_x$ unsymmetrisch, so dass $\mu_3 \ne 0$ ist.
- Nur bei einer gaußverteilten Zufallsgröße gilt für die Kurtosis $K = 3$.
- Bei der Rayleighverteilung ergibt sich aufgrund ausgeprägterer WDF–Ausläufer ein größerer Wert $(K = 3.245)$, und zwar unabhängig von $\lambda$.
(2) Die Ableitung der WDF nach $x$ liefert:
- $$\frac{{\rm d} f_x(x)}{{\rm d} x} = \frac{\rm 1}{\lambda^{\rm 2}}\cdot{\rm e}^{ -{x^{\rm 2}}/({2 \lambda^{\rm 2}})}+\frac{ x}{ \lambda^{\rm 2}}\cdot{\rm e}^{ -{x^{\rm 2}}/({ 2 \lambda^{\rm 2}})}\cdot(-\frac{2 x}{2 \lambda^{\rm 2}}).$$
- Daraus folgt als Bestimmungsgleichung für $x_0$ (nur die positive Lösung ist sinnvoll):
- $$\frac{1}{\lambda^{\rm 2}}\cdot{\rm e}^{ -{x_{\rm 0}^{\rm 2}}/{(2 \lambda^{\rm 2}})}\cdot(\rm 1-{\it x_{\rm 0}^{\rm 2}}/{\it \lambda^{\rm 2}})=0 \quad \Rightarrow \quad {\it x}_0=\it \lambda.$$
- Somit erhält man für den Verteilungsparameter $\lambda = x_0\hspace{0.15cm}\underline{= 2}$.
(3) Die gesuchte Wahrscheinlichkeit ist gleich der Verteilungsfunktion an der Stelle $r = x_0 = \lambda$:
- $${\rm Pr}(x<x_{\rm 0})={\rm Pr}( x \le x_{\rm 0})= F_x(x_{\rm 0})=1-{\rm e}^{-{\lambda^{\rm 2}}/({ 2 \lambda^{\rm 2}})}=1-{\rm e}^{-0.5}\hspace{0.15cm}\underline{=\rm 39.3\%}.$$
(4) Der Mittelwert kann beispielsweise nach folgender Gleichung ermittelt werden:
- $$m_x=\int_{-\infty}^{+\infty}\hspace{-0.45cm}x\cdot f_x(x)\,{\rm d}x=\int_{\rm 0}^{\infty}\frac{\it x^{\rm 2}}{\it \lambda^{\rm 2}} \cdot \rm e^{-{\it x^{\rm 2}}/({\rm 2\it \lambda^{\rm 2}})}\,{\rm d}\it x = \sqrt{{\rm \pi}/{\rm 2}}\cdot \it \lambda\hspace{0.15cm}\underline{=\rm 2.506}.$$
- Der Mittelwert $m_x$ ist natürlich größer als $x_0$ $(=$ Maximalwert der WDF$)$, da die WDF zwar nach unten, aber nicht nach oben begrenzt ist.
(5) Allgemein gilt für die gesuchte Wahrscheinlichkeit:
- $${\rm Pr}(x>m_x)=1- F_x(m_x).$$
- Mit der angegebenen Verteilungsfunktion und dem Ergebnis der Teilaufgabe (4) erhält man:
- $${\rm Pr}(x>m_x)={\rm e}^{-{m_x^{\rm 2}}/({ 2\lambda^{\rm 2})}}={\rm e}^{-\pi/ 4}\hspace{0.15cm}\underline{\approx \rm 45.6\%}.$$