Difference between revisions of "Aufgaben:Exercise 3.5Z: Antenna Areas"
m (Text replacement - "rms value" to "standard deviation") |
|||
| (16 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Uniformly_Distributed_Random_Variables |
}} | }} | ||
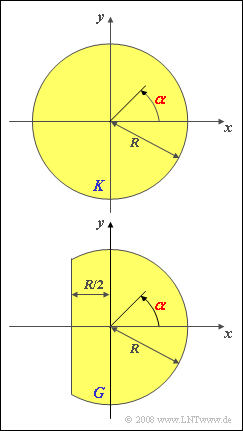
| − | [[File:P_ID188__Sto_Z_3_5.png|right|frame| | + | [[File:P_ID188__Sto_Z_3_5.png|right|frame|Two antenna areas: K and G]] |
| − | + | We first consider – as sketched in the image above – a receiving antenna serving a circular area K. It is assumed that this antenna can detect all signals incident at different angles α equally well: | |
| − | * | + | *According to the sketch, the angle α refers to the x–axis. |
| − | * | + | *The value α=0 therefore means that the signal is moving towards the antenna in the direction of the negative x–axis. |
| − | + | Further we assume: | |
| − | * | + | *The range of values of the angle of incidence α with this definition −π<α≤+π. |
| − | * | + | *There are very many users in the coverage area whose positions (x,y) are "statistically distributed" over the area K. |
| − | + | From subtask '''(5)''' we assume the coverage area G outlined below. | |
| − | * | + | *Because of an obstacle, the x–coordinate of all participants must now be greaterö&space;than −R/2. |
| − | * | + | *Also in the coverage area G the subscribers would again be "statistically distributed". |
| Line 22: | Line 22: | ||
| − | + | Hint: | |
| − | * | + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Uniformly_Distributed_Random_Variables|Uniformly Distributed Random Variables]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the PDF fα(α) for the area K? What PDF–value results for α=0? |
|type="{}"} | |type="{}"} | ||
fα(α=0) = { 0.159 3% } | fα(α=0) = { 0.159 3% } | ||
| − | { | + | {Which of the two statements is correct? Note in particular also the asymmetric definition range of −π<α≤+π. |
| − | |type=" | + | |type="()"} |
| − | + | + | + The expected value is E[α]=0. |
| − | - | + | - The expected value is E[α]≠0. |
| − | { | + | {What value results for the standard deviation of the random variable α in the area K? |
|type="{}"} | |type="{}"} | ||
| − | $\sigma_\alpha \ = | + | σα = { 1.814 3% } |
| − | { | + | {What is the probability that in area K the antenna locates a user at an angle between ±45∘ ? |
|type="{}"} | |type="{}"} | ||
| − | ${\rm Pr}( | + | ${\rm Pr}(-π/4 ≤ α ≤ +π/4) \ = \ $ { 25 3% } % |
| − | { | + | {Now let's consider the coverage area G. In which area −α0≤α≤+α0 does the PDF fα(α) have a constant value? |
|type="{}"} | |type="{}"} | ||
| − | α0 = | + | α0 = { 2.094 3% } rad |
| − | α0 = | + | α0 = { 120 3% } $ \ \rm degrees$ |
| − | { | + | {What statements are now valid with respect to fα(α) in the range |α|>α0 ? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The PDF has the same course "outside" as "inside". |
| − | - | + | - The PDF is "outside" identically zero. |
| − | + | + | + The PDF decreases towards the edges in this area. |
| − | - | + | - The PDF increases towards the edges in this area. |
| − | { | + | {Calculate for the area G the probability that the antenna locates a user at an angle between ±45∘ . |
|type="{}"} | |type="{}"} | ||
| − | ${\rm Pr}( | + | ${\rm Pr}(-π/4 ≤ α ≤ +π/4) \ = \ $ { 31.1 3% } % |
| − | { | + | {What is now the PDF value at the position α=0? |
|type="{}"} | |type="{}"} | ||
| − | $f_\alpha(\alpha = | + | $f_\alpha(\alpha = 0) \ = \ $ { 0.198 3% } |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' There is a uniform distribution and it is true for the PDF in the range −π<α≤+π: |
| − | fα(α)=1/(2⋅π). | + | :$$f_\alpha(\alpha)={\rm 1}/({\rm 2\cdot \pi}).$$ |
| + | * For α=0 this gives – as for all allowed values also – the PDF value :$$f_\alpha(\alpha =0) \hspace{0.15cm}\underline{=0.159}.$$ | ||
| − | |||
| − | '''(2)''' | + | '''(2)''' It holds ${\rm E}\big[\alpha\big] = 0$ ⇒ <u>Answer 1</u>. |
| + | *It has no effect that α=+π is allowed, but α=−π is excluded. | ||
| − | |||
| − | |||
| − | '''( | + | '''(3)''' For the variance of the angle of incidence α holds: |
| + | :$$\sigma_{\alpha}^{\rm 2}=\int_{-\rm\pi}^{\rm\pi}\hspace{-0.1cm}\it\alpha^{\rm 2}\cdot \it f_{\alpha}(\alpha)\,\,{\rm d} \alpha=\frac{\rm 1}{\rm 2\cdot\it \pi}\cdot \frac{\alpha^{\rm 3}}{\rm 3}\Bigg|_{\rm -\pi}^{\rm\pi}=\frac{\rm 2\cdot\pi^{3}}{\rm 2\cdot\rm \pi\cdot \rm 3}=\frac{\rm \pi^2}{\rm 3} = \rm 3. 29. \hspace{0.5cm}\Rightarrow \hspace{0.5cm}\sigma_{\alpha}\hspace{0.15cm}\underline{=1.814}.$$ | ||
| − | + | '''(4)''' Since the given section of the circle is exactly one quarter of the total circle area, the probability we are looking for is | |
| − | '''( | + | :$${\rm Pr}(-π/4 ≤ α ≤ +π/4)\hspace{0.15cm}\underline{=25\%}.$$ |
| − | $$ | ||
| − | |||
| − | '''(6)''' | + | |
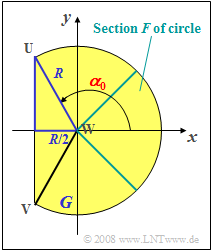
| − | * | + | [[File:EN_Sto_Z_3_5_e.png|right|frame|The area G]] |
| − | * | + | '''(5)''' From simple geometrical ¨considerations (right-angled triangle, marked dark blue in the adjacent sketch) one obtains the equation of determination for the angle α0: |
| − | * | + | :cos(π−α0)=R/2R=1/2⇒π−α0=π3(60∘). |
| − | * | + | *It follows α0=π/3=2.094_. |
| + | *This corresponds α0=120∘_. | ||
| + | |||
| + | |||
| + | |||
| + | '''(6)''' Correct is <u>the suggested solution 3</u>: | ||
| + | *The PDF fα(α) is f for a given angle α directly proportional to the distance A between the antenna and the boundary line. | ||
| + | *For $\alpha = \pm 2\pi/3 = \pm 120^\circ$ against A=R, for $\alpha \pm \pi = \pm 180^\circ$ against A=R/2. | ||
| + | *In between the distance becomes successively smaller. This means: The PDF decreases towards the boundary. | ||
| + | *The decrease follows the following course: | ||
:A=R/2cos(π−α). | :A=R/2cos(π−α). | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | '''(7)''' The area G can be calculated from the sum of the 240∘–sector and the triangle formed by the vertices UVW : | |
| + | :$$G=\frac{\rm 2}{\rm 3}\cdot \it R^{\rm 2}\cdot{\rm \pi} \ {\rm +} \ \frac{\it R}{\rm 2}\cdot \it R\cdot \rm sin(\rm 60^{\circ}) = \it R^{\rm 2}\cdot \rm\pi\cdot (\frac{\rm 2}{\rm 3}+\frac{\rm \sqrt{3}}{\rm 4\cdot\pi}).$$ | ||
| + | |||
| + | *The probability we are looking for is given by the ratio of the areas $F$ and $G$ (see sketch): | ||
| + | :$$\rm Pr(\rm -\pi/4\le\it\alpha\le+\rm\pi/4)=\frac{\it F}{\it G}=\frac{1/4}{2/3+{\rm sin(60^{\circ})}/({\rm 2\pi})}=\frac{\rm 0.25}{\rm 0.805}\hspace{0.15cm}\underline{=\rm 31.1\%}.$$ | ||
| + | |||
| + | *Although nothing has changed from point '''(4)''' at the area F the probability now becomes larger by a factor $1/0.805 ≈ 1.242$ due to the smaller area G . | ||
| + | |||
| + | |||
| − | '''(8)''' | + | '''(8)''' Since the overall PDF area is constantly equal 1 and the PDF decreases at the boundaries, it must have a larger value in the range |α|<2π/3 than in '''(1)'''. |
| − | fα(α=0)=1/(2π)2/3+sin(60∘)/(2π)=14⋅π/3+sin(60∘)≈0.198_. | + | * With the results from '''(1)''' and '''(7)''' holds: |
| + | :fα(α=0)=1/(2π)2/3+sin(60∘)/(2π)=14⋅π/3+sin(60∘)≈0.198_. | ||
| − | + | *Like the probability in '''(7)''' also simultaneously the PDF value in the range |α|<2π/3 increases by a factor 1.242 as the coverage area becomes smaller. | |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 123: | Line 135: | ||
| − | [[Category: | + | [[Category:Theory of Stochastic Signals: Exercises|^3.4 Uniformly Distributed Random Variable^]] |
Latest revision as of 13:11, 17 February 2022
We first consider – as sketched in the image above – a receiving antenna serving a circular area K. It is assumed that this antenna can detect all signals incident at different angles α equally well:
- According to the sketch, the angle α refers to the x–axis.
- The value α=0 therefore means that the signal is moving towards the antenna in the direction of the negative x–axis.
Further we assume:
- The range of values of the angle of incidence α with this definition −π<α≤+π.
- There are very many users in the coverage area whose positions (x,y) are "statistically distributed" over the area K.
From subtask (5) we assume the coverage area G outlined below.
- Because of an obstacle, the x–coordinate of all participants must now be greaterö&space;than −R/2.
- Also in the coverage area G the subscribers would again be "statistically distributed".
Hint:
- The exercise belongs to the chapter Uniformly Distributed Random Variables.
Questions
Solution
- f_\alpha(\alpha)={\rm 1}/({\rm 2\cdot \pi}).
- For \alpha = 0 this gives – as for all allowed values also – the PDF value :f_\alpha(\alpha =0) \hspace{0.15cm}\underline{=0.159}.
(2) It holds {\rm E}\big[\alpha\big] = 0 ⇒ Answer 1.
- It has no effect that \alpha = +\pi is allowed, but \alpha = -\pi is excluded.
(3) For the variance of the angle of incidence \alpha holds:
- \sigma_{\alpha}^{\rm 2}=\int_{-\rm\pi}^{\rm\pi}\hspace{-0.1cm}\it\alpha^{\rm 2}\cdot \it f_{\alpha}(\alpha)\,\,{\rm d} \alpha=\frac{\rm 1}{\rm 2\cdot\it \pi}\cdot \frac{\alpha^{\rm 3}}{\rm 3}\Bigg|_{\rm -\pi}^{\rm\pi}=\frac{\rm 2\cdot\pi^{3}}{\rm 2\cdot\rm \pi\cdot \rm 3}=\frac{\rm \pi^2}{\rm 3} = \rm 3. 29. \hspace{0.5cm}\Rightarrow \hspace{0.5cm}\sigma_{\alpha}\hspace{0.15cm}\underline{=1.814}.
(4) Since the given section of the circle is exactly one quarter of the total circle area, the probability we are looking for is
- {\rm Pr}(-π/4 ≤ α ≤ +π/4)\hspace{0.15cm}\underline{=25\%}.
(5) From simple geometrical ¨considerations (right-angled triangle, marked dark blue in the adjacent sketch) one obtains the equation of determination for the angle \alpha_0:
- \cos(\pi-\alpha_{\rm 0}) = \frac{R/ 2}{R}={\rm 1}/{\rm 2}\hspace{0.5cm}\Rightarrow\hspace{0.5cm}\rm\pi-\it\alpha_{\rm 0}=\frac{\rm\pi}{\rm 3} \hspace{0.2cm}\rm( 60^{\circ}).
- It follows \alpha_0 = \pi/3\hspace{0.15cm}\underline{=2.094}.
- This corresponds \alpha_0 \hspace{0.15cm}\underline{=120^\circ}.
(6) Correct is the suggested solution 3:
- The PDF f_\alpha(\alpha) is f for a given angle \alpha directly proportional to the distance A between the antenna and the boundary line.
- For \alpha = \pm 2\pi/3 = \pm 120^\circ against A = R, for \alpha \pm \pi = \pm 180^\circ against A = R/2.
- In between the distance becomes successively smaller. This means: The PDF decreases towards the boundary.
- The decrease follows the following course:
- \it A=\frac{\it R/\rm 2}{\rm cos(\rm \pi-\it\alpha)}.
(7) The area G can be calculated from the sum of the 240^\circ–sector and the triangle formed by the vertices \rm UVW :
- G=\frac{\rm 2}{\rm 3}\cdot \it R^{\rm 2}\cdot{\rm \pi} \ {\rm +} \ \frac{\it R}{\rm 2}\cdot \it R\cdot \rm sin(\rm 60^{\circ}) = \it R^{\rm 2}\cdot \rm\pi\cdot (\frac{\rm 2}{\rm 3}+\frac{\rm \sqrt{3}}{\rm 4\cdot\pi}).
- The probability we are looking for is given by the ratio of the areas F and G (see sketch):
- \rm Pr(\rm -\pi/4\le\it\alpha\le+\rm\pi/4)=\frac{\it F}{\it G}=\frac{1/4}{2/3+{\rm sin(60^{\circ})}/({\rm 2\pi})}=\frac{\rm 0.25}{\rm 0.805}\hspace{0.15cm}\underline{=\rm 31.1\%}.
- Although nothing has changed from point (4) at the area F the probability now becomes larger by a factor 1/0.805 ≈ 1.242 due to the smaller area G .
(8) Since the overall PDF area is constantly equal 1 and the PDF decreases at the boundaries, it must have a larger value in the range |\alpha| < 2\pi/3 than in (1).
- With the results from (1) and (7) holds:
- f_{\alpha}(\alpha = 0)=\frac{1/(2\pi)}{2/3+{\rm sin(\rm 60^{\circ})}/({\rm 2\pi})} = \frac{\rm 1}{{\rm 4\cdot\pi}/{\rm 3}+\rm sin(60^{\circ})}\hspace{0.15cm}\underline{\approx \rm 0.198}.
- Like the probability in (7) also simultaneously the PDF value in the range |\alpha| < 2\pi/3 increases by a factor 1.242 as the coverage area becomes smaller.