Difference between revisions of "Aufgaben:Exercise 5.2: Determination of the Frequency Response"
From LNTwww
| (21 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Stochastic_System_Theory |
}} | }} | ||
| − | [[File: | + | [[File:EN_Sto_A_5_2_neu2.png|right|frame|Measuring the frequency response $H(f)$]] |
| − | + | We consider the illustrated measurement setup for the determination of the frequency response $H(f)$ highlighted in blue. | |
| − | * | + | *The input signal $x(t)$ is white Gaussian noise with the noise power density $N_0 = 10^{-10} \hspace{0.05cm} \rm W/Hz$. |
| + | *Thus, the auto-correlation function $\rm (ACF)$ is: | ||
:$$\varphi _x ( \tau ) = {N_0 }/{2} \cdot \delta ( \tau ).$$ | :$$\varphi _x ( \tau ) = {N_0 }/{2} \cdot \delta ( \tau ).$$ | ||
| − | * | + | *The measured cross-correlation function $\rm (CCF)$ between the signals $x(t)$ and $y(t)$ can be approximated as follows <br>$($valid only for positive times $t)$: |
| − | :$$\varphi _{xy} \left( \tau \right) = K \cdot {\rm{e}}^{ - \tau /T_0 } .$$ | + | :$$\varphi _{xy} \left( \tau \right) = K \cdot {\rm{e}}^{ - \tau /T_0 },\hspace{0.5cm}\text{with } \ K = 0.628 \cdot 10^{-12} \hspace{0.05cm} {\rm W}, \ T_0 = 1 \hspace{0.05cm} \rm ms.$$ |
| + | *The ACF $\varphi_y(\tau)$ of the output signal $y(t)$ is also measured. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | Notes: | ||
| + | *The exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Stochastic_System_Theory|Stochastic System Theory]]. | ||
| + | *Reference is also made to the chapter [[Theory_of_Stochastic_Signals/Power-Spectral_Density|Power-Spectral Density]]. | ||
| + | *Please also note the following Fourier transform $($in $\omega)$: | ||
| + | :$$H( \omega ) = \frac{1}{{1 + {\rm{j}}\cdot \omega /\omega _0 }}\hspace{0.15cm}\bullet\!\!\!-\!\!\!-\!\!\!-\!\!\circ\,\hspace{0.15cm}h(t) = \omega _0 \cdot {\rm{e}}^{ - \omega _0 t} \hspace{0.3cm}(t \ge 0).$$ | ||
| + | :For negative $t$–values, on the other hand, $h(t) =0$ at all times. | ||
| − | === | + | |
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the following statements is true? One can completely determine the frequency response $H(f)$ by magnitude and phase if |
|type="[]"} | |type="[]"} | ||
| − | - | + | - the functions $\varphi_x(\tau)$ and $\varphi_y(\tau)$ are known, |
| − | + | + | + the functions $\varphi_x(\tau)$ and $\varphi_{xy}(\tau)$ are known, |
| − | + | + | + the functions $\varphi_{xy}(\tau)$ and $\varphi_y(\tau)$ are known. |
| − | { | + | {Calculate the impulse response $h(t)$. What is the value for $t=T_0$? |
|type="{}"} | |type="{}"} | ||
| − | $h(t = T_0) \ = $ { 4.62 3% } $\ \cdot 10^{-3} \ \rm 1/s$ | + | $h(t = T_0) \ = \ $ { 4.62 3% } $\ \cdot 10^{-3} \ \rm 1/s$ |
| − | { | + | {What is the frequency response $H(f)$? What value results for $f= 0$? |
|type="{}"} | |type="{}"} | ||
| − | $H(f = 0) \ = $ { 0.5 3% } | + | $H(f = 0) \ = \ $ { 0.5 3% } |
| − | { | + | {Calculate the power-spectral density of the output signal $y(t)$. What value results for frequency $f = 1/(2\pi T_0)$? |
|type="{}"} | |type="{}"} | ||
| − | ${\it \Phi}_y(f = 1/(2\pi T_0)) \ = $ { 6.25 3% } $\ \cdot 10^{-12}\ \rm W/Hz$ | + | ${\it \Phi}_y(f = 1/(2\pi T_0)) \ = \ $ { 6.25 3% } $\ \cdot 10^{-12}\ \rm W/Hz$ |
| Line 51: | Line 52: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | + | '''(1)''' <u>Statements 2 and 3</u> are true. | |
| − | + | *The following equations are valid: | |
| − | |||
:$$\varphi _{xy} ( \tau ) = h( \tau ) * \varphi _x ( \tau )\quad \Rightarrow \quad H( f ) = \frac{{{\it \Phi} _{xy} ( f )}}{{{\it \Phi} _x ( f )}},$$ | :$$\varphi _{xy} ( \tau ) = h( \tau ) * \varphi _x ( \tau )\quad \Rightarrow \quad H( f ) = \frac{{{\it \Phi} _{xy} ( f )}}{{{\it \Phi} _x ( f )}},$$ | ||
:$$\varphi _y ( \tau) = \varphi _{xy} ( \tau) * h(- \tau)\quad \Rightarrow \quad H^{\star}( f ) = \frac{{{\it \Phi} _y ( f )}}{{{\it \Phi} _{xy} ( f )}}.$$ | :$$\varphi _y ( \tau) = \varphi _{xy} ( \tau) * h(- \tau)\quad \Rightarrow \quad H^{\star}( f ) = \frac{{{\it \Phi} _y ( f )}}{{{\it \Phi} _{xy} ( f )}}.$$ | ||
| + | *In contrast, the first statement is false: The phase relations are lost in the ACF calculation. | ||
| + | *The associated spectral functions to $\varphi_x(\tau)$ and $\varphi_x(\tau)$ – namely ${\it \Phi}_x(f)$ and ${\it \Phi}_y(f)$ – are purely real, so only the magnitude $|H(f)|$ can be given. | ||
| + | |||
| + | |||
| + | |||
| + | '''(2)''' For Dirac-shaped input ACF $\varphi_x(\tau)$, the impulse response $h(t)$ is equal in shape to the CCF: | ||
| + | :$$h(t) = \frac{{K \cdot {\rm{e}}^{ - t/T_0 } }}{N_0 /2} = 1.256 \cdot 10^{ - 2} \frac{1}{{\rm{s}}} \cdot {\rm{e}}^{ - t/T_0 } \hspace{0.3cm}\Rightarrow \hspace{0.3cm}h(t = T_0)\hspace{0.15cm}\underline{ = 4.62 \cdot 10^{-3}\ \rm 1/s}.$$ | ||
| − | |||
| − | |||
| − | |||
| − | + | '''(3)''' The Fourier correspondence given is with $T_0 = 1/\omega_0$ and the constant $C= N_0/2 \cdot T_0/K$: | |
| − | :$$h(t) = \frac{C}{T_0 } \cdot {\rm{e}}^{ - t/T_0 }\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, H( \omega ) = \frac{C}{{1 + {\rm{j}}\omega T_0 }}.$$ | + | :$$h(t) = \frac{C}{T_0 } \cdot {\rm{e}}^{ - t/T_0 }\hspace{0.15cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\,\hspace{0.15cm} H( \omega ) = \frac{C}{{1 + {\rm{j}}\cdot \omega T_0 }}.$$ |
| − | + | *The constant results in $C = 0.08$. With $H(f) = 2 \pi \cdot H(\omega)$ it follows: | |
| − | :$$H(f) = \frac{0.5}{1 + {\rm{ | + | :$$H(f) = \frac{0.5}{1 + {\rm{j\cdot 2\pi }}\cdot f \cdot T_0 } \hspace{0.3cm}\Rightarrow \hspace{0.3cm} H(f= 0) \hspace{0.15cm}\underline{=0.5}.$$ |
| − | |||
| − | |||
| − | |||
| − | + | '''(4)''' For the output PSD, in general or specifically here: | |
| − | :$${\it \Phi}_y (f) = {N_0 }/{8} \cdot \frac{1}{1 + \left( {{\rm{2\pi }} | + | :$${\it \Phi}_y (f) = {\it \Phi} _x (f) \cdot \left| {H(f)} \right|^2 = \frac{N_0 }{2} \cdot \frac{0.5^2 }{{\left( {1 + {\rm{j\cdot 2\pi }}\cdot f \cdot T_0 } \right)\left( {1 - {\rm{j\cdot 2\pi }}\cdot f \cdot T_0 } \right)}} = {N_0 }/{8} \cdot \frac{1}{1 + \left( {{\rm{2\pi }}\cdot f\cdot T_0 } \right)^2 }.$$ |
| − | + | *At the given frequency $f = 1/(2\pi T_0)$, ${\it \Phi}_y (f)$ has dropped by half compared to its maximum at $f=0$ : | |
:$${\it \Phi}_y (f = 1/(2 \pi T_0)) ={N_0 }/{16}\hspace{0.15cm} \underline{ = 6.25 \cdot 10^{ - 12} \;{\rm{W/Hz}}}.$$ | :$${\it \Phi}_y (f = 1/(2 \pi T_0)) ={N_0 }/{16}\hspace{0.15cm} \underline{ = 6.25 \cdot 10^{ - 12} \;{\rm{W/Hz}}}.$$ | ||
| Line 85: | Line 86: | ||
| − | [[Category: | + | [[Category:Theory of Stochastic Signals: Exercises|^5.1 Stochastic Systems Theory^]] |
Latest revision as of 16:54, 22 February 2022
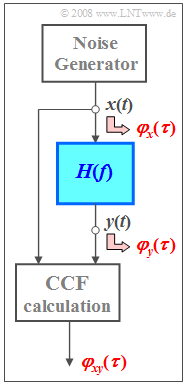
We consider the illustrated measurement setup for the determination of the frequency response $H(f)$ highlighted in blue.
- The input signal $x(t)$ is white Gaussian noise with the noise power density $N_0 = 10^{-10} \hspace{0.05cm} \rm W/Hz$.
- Thus, the auto-correlation function $\rm (ACF)$ is:
- $$\varphi _x ( \tau ) = {N_0 }/{2} \cdot \delta ( \tau ).$$
- The measured cross-correlation function $\rm (CCF)$ between the signals $x(t)$ and $y(t)$ can be approximated as follows
$($valid only for positive times $t)$:
- $$\varphi _{xy} \left( \tau \right) = K \cdot {\rm{e}}^{ - \tau /T_0 },\hspace{0.5cm}\text{with } \ K = 0.628 \cdot 10^{-12} \hspace{0.05cm} {\rm W}, \ T_0 = 1 \hspace{0.05cm} \rm ms.$$
- The ACF $\varphi_y(\tau)$ of the output signal $y(t)$ is also measured.
Notes:
- The exercise belongs to the chapter Stochastic System Theory.
- Reference is also made to the chapter Power-Spectral Density.
- Please also note the following Fourier transform $($in $\omega)$:
- $$H( \omega ) = \frac{1}{{1 + {\rm{j}}\cdot \omega /\omega _0 }}\hspace{0.15cm}\bullet\!\!\!-\!\!\!-\!\!\!-\!\!\circ\,\hspace{0.15cm}h(t) = \omega _0 \cdot {\rm{e}}^{ - \omega _0 t} \hspace{0.3cm}(t \ge 0).$$
- For negative $t$–values, on the other hand, $h(t) =0$ at all times.
Questions
Solution
(1) Statements 2 and 3 are true.
- The following equations are valid:
- $$\varphi _{xy} ( \tau ) = h( \tau ) * \varphi _x ( \tau )\quad \Rightarrow \quad H( f ) = \frac{{{\it \Phi} _{xy} ( f )}}{{{\it \Phi} _x ( f )}},$$
- $$\varphi _y ( \tau) = \varphi _{xy} ( \tau) * h(- \tau)\quad \Rightarrow \quad H^{\star}( f ) = \frac{{{\it \Phi} _y ( f )}}{{{\it \Phi} _{xy} ( f )}}.$$
- In contrast, the first statement is false: The phase relations are lost in the ACF calculation.
- The associated spectral functions to $\varphi_x(\tau)$ and $\varphi_x(\tau)$ – namely ${\it \Phi}_x(f)$ and ${\it \Phi}_y(f)$ – are purely real, so only the magnitude $|H(f)|$ can be given.
(2) For Dirac-shaped input ACF $\varphi_x(\tau)$, the impulse response $h(t)$ is equal in shape to the CCF:
- $$h(t) = \frac{{K \cdot {\rm{e}}^{ - t/T_0 } }}{N_0 /2} = 1.256 \cdot 10^{ - 2} \frac{1}{{\rm{s}}} \cdot {\rm{e}}^{ - t/T_0 } \hspace{0.3cm}\Rightarrow \hspace{0.3cm}h(t = T_0)\hspace{0.15cm}\underline{ = 4.62 \cdot 10^{-3}\ \rm 1/s}.$$
(3) The Fourier correspondence given is with $T_0 = 1/\omega_0$ and the constant $C= N_0/2 \cdot T_0/K$:
- $$h(t) = \frac{C}{T_0 } \cdot {\rm{e}}^{ - t/T_0 }\hspace{0.15cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\,\hspace{0.15cm} H( \omega ) = \frac{C}{{1 + {\rm{j}}\cdot \omega T_0 }}.$$
- The constant results in $C = 0.08$. With $H(f) = 2 \pi \cdot H(\omega)$ it follows:
- $$H(f) = \frac{0.5}{1 + {\rm{j\cdot 2\pi }}\cdot f \cdot T_0 } \hspace{0.3cm}\Rightarrow \hspace{0.3cm} H(f= 0) \hspace{0.15cm}\underline{=0.5}.$$
(4) For the output PSD, in general or specifically here:
- $${\it \Phi}_y (f) = {\it \Phi} _x (f) \cdot \left| {H(f)} \right|^2 = \frac{N_0 }{2} \cdot \frac{0.5^2 }{{\left( {1 + {\rm{j\cdot 2\pi }}\cdot f \cdot T_0 } \right)\left( {1 - {\rm{j\cdot 2\pi }}\cdot f \cdot T_0 } \right)}} = {N_0 }/{8} \cdot \frac{1}{1 + \left( {{\rm{2\pi }}\cdot f\cdot T_0 } \right)^2 }.$$
- At the given frequency $f = 1/(2\pi T_0)$, ${\it \Phi}_y (f)$ has dropped by half compared to its maximum at $f=0$ :
- $${\it \Phi}_y (f = 1/(2 \pi T_0)) ={N_0 }/{16}\hspace{0.15cm} \underline{ = 6.25 \cdot 10^{ - 12} \;{\rm{W/Hz}}}.$$