Aufgaben:Exercise 3.2: Spectrum with Angle Modulation

• Return to book

Table of Bessel functions

The following equations are assumed here:

• Source signal:

$$q(t) = 2\,{\rm V} \cdot \sin(2 \pi \cdot 3\,{\rm kHz} \cdot t)\hspace{0.05cm},$$

• Transmit signal:

$$s(t) = 1\,{\rm V} \cdot \cos\hspace{-0.1cm}\big[2 \pi \cdot 100\,{\rm kHz} \cdot t + K_{\rm M} \cdot q(t)\big ]\hspace{0.05cm},$$

• Received signal (ideal channel):

$$r(t) = s(t) = 1\,{\rm V} \cdot \cos\hspace{-0.1cm}\big[2 \pi \cdot 100\,{\rm kHz} \cdot t + \phi(t)\big ]\hspace{0.05cm},$$

• ideal demodulator:

$$v(t) = \frac{1}{ K_{\rm M}} \cdot \phi(t)\hspace{0.05cm}.$$

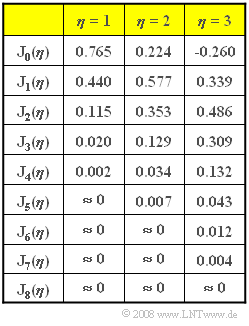
The graphs shows the   $n$–th order Bessel functions of the first kind   ${\rm J}_n (\eta)$  in table form.

Hints:

• This exercise belongs to the chapter  Phase Modulation.
• Particular reference is made to the pages  Spectral function of a phase-modulated sine signal  and  Interpretation of the Bessel spectrum.

Questions

1

Which modulation method is used here?

	Amplitude modulation.
	Phase modulation.
	Frequency modulation.

2

Which modulation method would you choose if the channel bandwidth was only  $B_{\rm K} = 10 \ \rm kHz$ ?

	Amplitude modulation.
	Phase modulation.
	Frequency modulation.

3

How should one choose the modulator constant $K_{\rm M}$  for a phase deviation of  $η = 1$ ?

$K_{\rm M} \ = \ $ $\ \rm 1/V$

4

Calculate the spectrum  $S_{\rm TP}(f)$  of the equivalent low-pass signal  $s_{\rm TP}(t)$.  What are the weights of the spectral lines at  $f = 0$  and  $f = -3 \ \rm kHz$?

$S_{\rm TP}(f = 0)\ = \ $ $\ \rm V$

$S_{\rm TP}(f = -3\ \rm kHz) \ = \ $ $\ \rm V$

5

Calculate the spectra of the analytical signal $s_{\rm +}(t)$  and the physical signal  $s(t)$.  What are the weights of the spectral lines at  $f = 97 \ \rm kHz$?

$S_+(f = 97 \ \rm kHz)\ = \ $ $\ \rm V$

$S(f = 97 \ \rm kHz)\hspace{0.32cm} = \ $ $\ \rm V$

6

What is the required channel bandwidth  $B_{\rm K}$  for  $η = 1$, if one ignores pulse weights smaller (in magnitude) than $0.01$ ?

$η = 1\text{:} \ \ \ B_{\rm K}\ = \ $ $\ \rm kHz$

7

What would be the channel bandwidths for  $η = 2$  and  $η = 3$ ?

$η = 2\text{:} \ \ \ B_{\rm K}\ = \ $ $\ \rm kHz$

$η = 3\text{:} \ \ \ B_{\rm K}\ = \ $ $\ \rm kHz$

Solution

Solution

(1)  Die Phase  $ϕ(t)$  ist proportional zum Quellensignal  $q(t)$   ⇒   es handelt sich um eine Phasenmodulation   ⇒   Antwort 2.

(2)  Eine Winkelmodulation  (PM, FM)  führt bei bandbegrenztem Kanal stets zu nichtlinearen Verzerrungen.

• Bei Zweiseitenband-Amplitudenmodulation  (ZSB-AM)  ist hier dagegen bereits mit  $B_{\rm K} = 6 \ \rm kHz$  eine verzerrungsfreie Übertragung möglich   ⇒   Antwort 1.

(3)  Der Modulationsindex (oder Phasenhub) ist bei Phasenmodulation gleich  $η = K_{\rm M} · A_{\rm N}$.

• Somit ist die Modulatorkonstante  $K_{\rm M} = 1/A_{\rm N}\hspace{0.15cm}\underline { = 0.5 \rm \cdot {1}/{V}}$  zu wählen, damit sich  $η = 1$  ergibt.

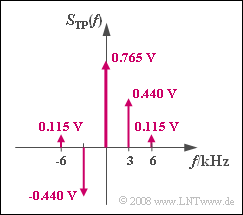
(4)  Es liegt ein sogenanntes Besselspektrum vor:

$$S_{\rm TP}(f) = A_{\rm T} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot \delta (f - n \cdot f_{\rm N})\hspace{0.05cm}.$$

• Dieses ist ein diskretes Spektrum mit Anteilen bei  $f = n · f_{\rm N}$, wobei  $n$  ganzzahlig ist.
• Die Gewichte der Diracfunktionen sind durch die Besselfunktionen gegeben.  Mit  $A_{\rm T} = 1\ \rm V$  erhält man:

PM–Spektrum im äquivalenten Tiefpass–Bereich

$$S_{\rm TP}(f = 0) = A_{\rm T} \cdot {\rm J}_0 (\eta = 1) \hspace{0.15cm}\underline {= 0.765\,{\rm V}},$$

$$S_{\rm TP}(f = f_{\rm N}) = A_{\rm T} \cdot {\rm J}_1 (\eta = 1)\hspace{0.15cm} = 0.440\,{\rm V},$$

$$S_{\rm TP}(f = 2 \cdot f_{\rm N}) = A_{\rm T} \cdot {\rm J}_2 (\eta = 1) = 0.115\,{\rm V} \hspace{0.05cm}.$$

• Aufgrund der Symmetrie  ${\rm J}_{-n} (\eta) = (-1)^n \cdot {\rm J}_{n} (\eta)$  erhält man für die Spektrallinie bei  $f = -3 \ \rm kHz$:

$$S_{\rm TP}(f = -f_{\rm N}) = -S_{\rm TP}(f = +f_{\rm N}) =\hspace{-0.01cm}\underline { -0.440\,{\rm V} \hspace{0.05cm}}.$$

Anmerkung:  Eigentlich müsste man für den Spektralwert bei  $f = 0$  schreiben:

$$S_{\rm TP}(f = 0) = 0.765\,{\rm V} \cdot \delta (f) \hspace{0.05cm}.$$

• Dieser ist somit aufgrund der Diracfunktion unendlich groß, lediglich das Gewicht der Diracfunktion ist endlich.
• Gleiches gilt für alle diskreten Spektrallinien.

(5)  $S_+(f)$  ergibt sich aus  $S_{\rm TP}(f)$  durch Verschiebung um  $f_{\rm T}$  nach rechts.  Deshalb ist

$$S_{\rm +}(f = 97\,{\rm kHz}) = S_{\rm TP}(f = -3\,{\rm kHz}) \hspace{0.15cm}\underline {=-0.440\,{\rm V}} \hspace{0.05cm}.$$

• Das tatsächliche Spektrum unterscheidet sich von  $S_+(f)$  bei positiven Frequenzen um den Faktor  $1/2$:

$$S(f = 97\,{\rm kHz}) = {1}/{2} \cdot S_{\rm +}(f = 97\,{\rm kHz}) \hspace{0.15cm}\underline {=-0.220\,{\rm V}} \hspace{0.05cm}.$$

• Allgemein kann geschrieben werden:

$$S(f) = \frac{A_{\rm T}}{2} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot \delta (f \pm (f_{\rm T}+ n \cdot f_{\rm N}))\hspace{0.05cm}.$$

(6)  Unter der vorgeschlagenen Vernachlässigung können alle Bessellinien  ${\rm J}_{|n|>3}$  außer Acht gelassen werden.

• Damit erhält man  $B_{\rm K} = 2 · 3 · f_{\rm N}\hspace{0.15cm}\underline { = 18 \ \rm kHz}$.

(7)  Die Zahlenwerte in der Tabelle auf der Angabenseite zeigen, dass nun folgende Kanalbandbreiten erforderlich wären:

• für $η = 2$:     $B_{\rm K} \hspace{0.15cm}\underline { = 24 \ \rm kHz}$,
• für $η = 3$:     $B_{\rm K} \hspace{0.15cm}\underline { = 36 \ \rm kHz}$.