Difference between revisions of "Aufgaben:Exercise 3.10Z: Amplitude and Angle Modulation in Comparison"
m (Text replacement - "Category:Aufgaben zu Modulationsverfahren" to "Category:Modulation Methods: Exercises") |
m |
||
| (11 intermediate revisions by 3 users not shown) | |||
| Line 3: | Line 3: | ||
}} | }} | ||
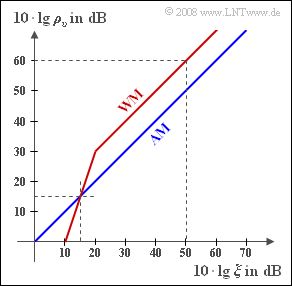
| − | [[File:P_ID1112__Mod_Z_3_9.png|right|frame| | + | [[File:P_ID1112__Mod_Z_3_9.png|right|frame|Characteristic curves illustrating the noise behavior for $\rm AM$ and $\rm WM$]] |
| − | + | Consider the transmission of a cosine signal with amplitude modulation $\rm (AM)$ and angle modulation $\rm (WM)$. The following boundary conditions apply:: | |
| − | * | + | * Message frequency $f_{\rm N} = 10 \ \rm kHz$, |
| − | * | + | * Transmission power $P_{\rm S} = 100 \ \rm kW$, |
| − | * | + | * Channel transmission factor $20 · \lg α_{\rm K} = -120 \ \rm dB$, |
| − | * | + | * noise power density $N_0 = 10^{–16} \ \rm W/Hz$. |
| − | + | These system parameters are conveniently combined to form the performance parameter: | |
| − | + | $$ \xi = \frac{\alpha_{\rm K}^2 \cdot P_{\rm S}}{N_0 \cdot B_{\rm NF}}$$ | |
| − | + | The graph shows the resulting sink-to-noise ratio $10 · \lg ρ_v$ as a function of the logarithmized performance parameter $ξ$. | |
| Line 22: | Line 22: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Modulation_Methods/Influence_of_Noise_on_Systems_with_Angle_Modulation#System_comparison_of_AM.2C_PM_and_FM_with_respect_to_noise]]. |
| − | * | + | *Reference is also made to the page [[Modulation_Methods/Synchronous_Demodulation#Sink_SNR_and_the_performance_parameter|Sink SNR and the performance parameter]] and the chapter [[Modulation_Methods/Frequency_Modulation_(FM)|Frequency Modulation]]. |
| − | * | + | *The following relationships hold: |
| − | :$$\rho_{v } = \left\{ \begin{array}{c} \xi \\ {\eta^2}/2 \cdot\xi \\ 3{\eta^2}/2 \cdot\xi \\ \end{array} \right.\quad \begin{array}{*{10}c} {\rm{ | + | :$$\rho_{v } = \left\{ \begin{array}{c} \xi \\ {\eta^2}/2 \cdot\xi \\ 3{\eta^2}/2 \cdot\xi \\ \end{array} \right.\quad \begin{array}{*{10}c} {\rm{for}} \\ {\rm{for}} \\ {\rm{for}} \\ \end{array}\begin{array}{*{20}l} {\rm DSB/SSB-AM \hspace{0.15cm}without \hspace{0.15cm}carrier} \hspace{0.05cm}, \\ {\rm PM \hspace{0.15cm}with \hspace{0.15cm}modulation\hspace{0.15cm}depth \hspace{0.15cm} \eta } \hspace{0.05cm}, \\ {\rm FM \hspace{0.15cm}with \hspace{0.15cm}modulation\hspace{0.15cm}depth \hspace{0.15cm} \eta }\hspace{0.05cm}. \\ \end{array}$$ |
| − | * | + | * The bandwidths $B_{\rm K}$ for angle modulation shall be selected according to the "Carson rule" to guarantee a distortion factor of $K < 1\%$ : |
:$$ B_{\rm K} = 2 \cdot f_{\rm N} \cdot (\eta +2) \hspace{0.05cm}.$$ | :$$ B_{\rm K} = 2 \cdot f_{\rm N} \cdot (\eta +2) \hspace{0.05cm}.$$ | ||
| Line 34: | Line 34: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the logarithmized power parameter $ξ$. |
|type="{}"} | |type="{}"} | ||
$10 · \lg \ ξ \ = \ $ { 50 3% } $\ \rm dB$ | $10 · \lg \ ξ \ = \ $ { 50 3% } $\ \rm dB$ | ||
| − | { | + | {What is the sink-to-noise ratio for the AM system? |
|type="{}"} | |type="{}"} | ||
$10 · \lg ρ_v \ = \ $ { 50 3% } $\ \rm dB$ | $10 · \lg ρ_v \ = \ $ { 50 3% } $\ \rm dB$ | ||
| − | { | + | {What special kind of AM might be present here? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + It could be a DSB-AM. |
| − | + | + | + It could be a SSB-AM. |
| − | + | + | + It could be an AM without a carrier. |
| − | - | + | - It could be an AM with an added carrier. |
| − | { | + | {In the case of the DSB-AM, what is the required channel bandwidth $B_{\rm K}$? |
|type="{}"} | |type="{}"} | ||
$B_{\rm K} \ = \ $ { 20 3% } $\ \rm kHz$ | $B_{\rm K} \ = \ $ { 20 3% } $\ \rm kHz$ | ||
| − | { | + | {What is the sink-to-noise ratio for the WM system? |
|type="{}"} | |type="{}"} | ||
$10 · \lg ρ_v \ = \ $ { 60 3% } $\ \rm dB$ | $10 · \lg ρ_v \ = \ $ { 60 3% } $\ \rm dB$ | ||
| − | { | + | {What is the minimum bandwidth required for the given PM system if $K < 1\%$ is to apply? |
|type="{}"} | |type="{}"} | ||
$B_{\rm K} \ = \ $ { 130 3% } $\ \rm kHz$ | $B_{\rm K} \ = \ $ { 130 3% } $\ \rm kHz$ | ||
| − | { | + | {For $K < 1\%$ , die erforderliche Bandbreite, what is the required bandwidth if the WM system implements frequency modulation?? |
|type="{}"} | |type="{}"} | ||
$B_{\rm K} \ = \ $ { 91.6 3% } $\ \rm kHz$ | $B_{\rm K} \ = \ $ { 91.6 3% } $\ \rm kHz$ | ||
| − | { | + | {All other parameters being equal, what is the minimum transmit power $P_{\rm S}$ required for the WM system to be no worse than the AM system? |
|type="{}"} | |type="{}"} | ||
$P_{\rm S,\hspace{0.05cm}min} \ = \ $ { 30 3% } $\ \rm W$ | $P_{\rm S,\hspace{0.05cm}min} \ = \ $ { 30 3% } $\ \rm W$ | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' From $20 · \lg α_{\rm K} = -120 \ \rm dB$ we get $α_{\rm K} = 10^{–6}$. Thus, with $B_{\rm NF} = f_{\rm N}$, one obtains: |
:$$ \xi = \frac{\alpha_{\rm K}^2 \cdot P_{\rm S}}{N_0 \cdot B_{\rm NF}}= \frac{10^{-12} \cdot 10^{5}\;{\rm W}}{10^{-16}\;{\rm W/Hz} \cdot 10^{4}\;{\rm Hz}}= 10^5 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}10 \cdot {\rm lg} \hspace{0.15cm}\xi \hspace{0.15cm}\underline {= 50\,{\rm dB}}\hspace{0.05cm}.$$ | :$$ \xi = \frac{\alpha_{\rm K}^2 \cdot P_{\rm S}}{N_0 \cdot B_{\rm NF}}= \frac{10^{-12} \cdot 10^{5}\;{\rm W}}{10^{-16}\;{\rm W/Hz} \cdot 10^{4}\;{\rm Hz}}= 10^5 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}10 \cdot {\rm lg} \hspace{0.15cm}\xi \hspace{0.15cm}\underline {= 50\,{\rm dB}}\hspace{0.05cm}.$$ | ||
| − | '''(2)''' | + | '''(2)''' From the graph, it can be seen that $ρ_v = ξ$ holds for the AM system. Thus, the sinking signal-to-noise ratio is: |
:$$10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }\hspace{0.15cm}\underline {= 50\,{\rm dB}}\hspace{0.05cm}.$$ | :$$10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }\hspace{0.15cm}\underline {= 50\,{\rm dB}}\hspace{0.05cm}.$$ | ||
| − | '''(3)''' | + | '''(3)''' The <u>first three answers</u> are correct: |
| − | * | + | *It is a DSB-AM or a SSB-AM without a carrier. |
| − | * | + | *DSB–AM and SSB–AM with a carrier can be ruled out. In these instances, it would always be the case that $ρ_v < \xi$ . |
| − | '''(4)''' | + | '''(4)''' For DSB–AM, $B_{\rm K} ≥ 2 · f_{\rm N}\hspace{0.15cm}\underline { = 20 \ \rm kHz}$ must hold. |
| − | '''(5)''' | + | '''(5)''' From the given graph, it can be seen that from about $20 \ \rm dB$ onwards: |
:$$10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }= 10 \cdot {\rm lg} \hspace{0.15cm}\xi + 10\,{\rm dB}. \hspace{0.3cm}{\rm Mit}\hspace{0.15cm}10 \cdot {\rm lg} \hspace{0.15cm}\xi = 50\,{\rm dB}\hspace{0.05cm}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }\hspace{0.15cm}\underline {= 60\,{\rm dB}}.$$ | :$$10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }= 10 \cdot {\rm lg} \hspace{0.15cm}\xi + 10\,{\rm dB}. \hspace{0.3cm}{\rm Mit}\hspace{0.15cm}10 \cdot {\rm lg} \hspace{0.15cm}\xi = 50\,{\rm dB}\hspace{0.05cm}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }\hspace{0.15cm}\underline {= 60\,{\rm dB}}.$$ | ||
| − | '''(6)''' | + | '''(6)''' In the case of phase modulation: |
:$$ \rho_{v }= \frac{\eta^2}{2} \cdot \xi \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta^2 = \frac{2 \cdot \rho_{v }}{\xi} = 20\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta \approx 4.47 \hspace{0.05cm}.$$ | :$$ \rho_{v }= \frac{\eta^2}{2} \cdot \xi \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta^2 = \frac{2 \cdot \rho_{v }}{\xi} = 20\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta \approx 4.47 \hspace{0.05cm}.$$ | ||
| − | * | + | *Thus, the channel bandwidth needed for 𝐾<1% must be: |
:$$B_{\rm K} \ge 2 \cdot f_{\rm N} \cdot (\eta +2) = 20\,{\rm kHz}\cdot 6.47 \hspace{0.15cm}\underline { \approx 130\,{\rm kHz}}\hspace{0.05cm}.$$ | :$$B_{\rm K} \ge 2 \cdot f_{\rm N} \cdot (\eta +2) = 20\,{\rm kHz}\cdot 6.47 \hspace{0.15cm}\underline { \approx 130\,{\rm kHz}}\hspace{0.05cm}.$$ | ||
| − | '''(7)''' | + | '''(7)''' Here, a smaller modulation index is sufficient, and thus a smaller bandwidth: |
:$${3}/{2}\cdot \eta^2 = 10\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta \approx 2.58 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}B_{\rm K} = 20\,{\rm kHz}\cdot 4.58 \hspace{0.15cm}\underline {\approx 91.6\,{\rm kHz}}\hspace{0.05cm}.$$ | :$${3}/{2}\cdot \eta^2 = 10\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta \approx 2.58 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}B_{\rm K} = 20\,{\rm kHz}\cdot 4.58 \hspace{0.15cm}\underline {\approx 91.6\,{\rm kHz}}\hspace{0.05cm}.$$ | ||
| − | '''(8)''' In | + | '''(8)''' In the graph, one can see the so-called FM threshold effect. |
| − | * | + | *For $10 · \lg \hspace{0.08cm} ξ = 15 \ \rm dB$ one obtains exactly the same sink SNR for the WM system as for the AM system. |
| − | * | + | *Thus, the transmit power can be $35 \ \rm dB$ less than s $100 \ \rm kW$: |
:$$ 10 \cdot {\rm lg} \hspace{0.15cm}\frac{P_{\rm S,\hspace{0.05cm}min}}{100\,{\rm kW}}= -35\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \frac{P_{\rm S,\hspace{0.05cm}min}}{100\,{\rm kW}}\approx 0.0003\hspace{0.3cm}\Rightarrow \hspace{0.3cm}P_{\rm S,\hspace{0.05cm}min} \hspace{0.15cm}\underline {\approx 30\,{\rm W}}\hspace{0.05cm}.$$ | :$$ 10 \cdot {\rm lg} \hspace{0.15cm}\frac{P_{\rm S,\hspace{0.05cm}min}}{100\,{\rm kW}}= -35\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \frac{P_{\rm S,\hspace{0.05cm}min}}{100\,{\rm kW}}\approx 0.0003\hspace{0.3cm}\Rightarrow \hspace{0.3cm}P_{\rm S,\hspace{0.05cm}min} \hspace{0.15cm}\underline {\approx 30\,{\rm W}}\hspace{0.05cm}.$$ | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 120: | Line 120: | ||
| − | [[Category:Modulation Methods: Exercises|^3.3 | + | [[Category:Modulation Methods: Exercises|^3.3 Noise Influence with PM and FM^]] |
Latest revision as of 18:28, 17 March 2022
Consider the transmission of a cosine signal with amplitude modulation $\rm (AM)$ and angle modulation $\rm (WM)$. The following boundary conditions apply::
- Message frequency $f_{\rm N} = 10 \ \rm kHz$,
- Transmission power $P_{\rm S} = 100 \ \rm kW$,
- Channel transmission factor $20 · \lg α_{\rm K} = -120 \ \rm dB$,
- noise power density $N_0 = 10^{–16} \ \rm W/Hz$.
These system parameters are conveniently combined to form the performance parameter:
$$ \xi = \frac{\alpha_{\rm K}^2 \cdot P_{\rm S}}{N_0 \cdot B_{\rm NF}}$$
The graph shows the resulting sink-to-noise ratio $10 · \lg ρ_v$ as a function of the logarithmized performance parameter $ξ$.
Hints:
- This exercise belongs to the chapter Modulation Methods/Influence of Noise on Systems with Angle Modulation.
- Reference is also made to the page Sink SNR and the performance parameter and the chapter Frequency Modulation.
- The following relationships hold:
- $$\rho_{v } = \left\{ \begin{array}{c} \xi \\ {\eta^2}/2 \cdot\xi \\ 3{\eta^2}/2 \cdot\xi \\ \end{array} \right.\quad \begin{array}{*{10}c} {\rm{for}} \\ {\rm{for}} \\ {\rm{for}} \\ \end{array}\begin{array}{*{20}l} {\rm DSB/SSB-AM \hspace{0.15cm}without \hspace{0.15cm}carrier} \hspace{0.05cm}, \\ {\rm PM \hspace{0.15cm}with \hspace{0.15cm}modulation\hspace{0.15cm}depth \hspace{0.15cm} \eta } \hspace{0.05cm}, \\ {\rm FM \hspace{0.15cm}with \hspace{0.15cm}modulation\hspace{0.15cm}depth \hspace{0.15cm} \eta }\hspace{0.05cm}. \\ \end{array}$$
- The bandwidths $B_{\rm K}$ for angle modulation shall be selected according to the "Carson rule" to guarantee a distortion factor of $K < 1\%$ :
- $$ B_{\rm K} = 2 \cdot f_{\rm N} \cdot (\eta +2) \hspace{0.05cm}.$$
Questions
Solution
- $$ \xi = \frac{\alpha_{\rm K}^2 \cdot P_{\rm S}}{N_0 \cdot B_{\rm NF}}= \frac{10^{-12} \cdot 10^{5}\;{\rm W}}{10^{-16}\;{\rm W/Hz} \cdot 10^{4}\;{\rm Hz}}= 10^5 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}10 \cdot {\rm lg} \hspace{0.15cm}\xi \hspace{0.15cm}\underline {= 50\,{\rm dB}}\hspace{0.05cm}.$$
(2) From the graph, it can be seen that $ρ_v = ξ$ holds for the AM system. Thus, the sinking signal-to-noise ratio is:
- $$10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }\hspace{0.15cm}\underline {= 50\,{\rm dB}}\hspace{0.05cm}.$$
(3) The first three answers are correct:
- It is a DSB-AM or a SSB-AM without a carrier.
- DSB–AM and SSB–AM with a carrier can be ruled out. In these instances, it would always be the case that $ρ_v < \xi$ .
(4) For DSB–AM, $B_{\rm K} ≥ 2 · f_{\rm N}\hspace{0.15cm}\underline { = 20 \ \rm kHz}$ must hold.
(5) From the given graph, it can be seen that from about $20 \ \rm dB$ onwards:
- $$10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }= 10 \cdot {\rm lg} \hspace{0.15cm}\xi + 10\,{\rm dB}. \hspace{0.3cm}{\rm Mit}\hspace{0.15cm}10 \cdot {\rm lg} \hspace{0.15cm}\xi = 50\,{\rm dB}\hspace{0.05cm}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}10 \cdot {\rm lg} \hspace{0.15cm}\rho_{v }\hspace{0.15cm}\underline {= 60\,{\rm dB}}.$$
(6) In the case of phase modulation:
- $$ \rho_{v }= \frac{\eta^2}{2} \cdot \xi \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta^2 = \frac{2 \cdot \rho_{v }}{\xi} = 20\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta \approx 4.47 \hspace{0.05cm}.$$
- Thus, the channel bandwidth needed for 𝐾<1% must be:
- $$B_{\rm K} \ge 2 \cdot f_{\rm N} \cdot (\eta +2) = 20\,{\rm kHz}\cdot 6.47 \hspace{0.15cm}\underline { \approx 130\,{\rm kHz}}\hspace{0.05cm}.$$
(7) Here, a smaller modulation index is sufficient, and thus a smaller bandwidth:
- $${3}/{2}\cdot \eta^2 = 10\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \eta \approx 2.58 \hspace{0.3cm}\Rightarrow \hspace{0.3cm}B_{\rm K} = 20\,{\rm kHz}\cdot 4.58 \hspace{0.15cm}\underline {\approx 91.6\,{\rm kHz}}\hspace{0.05cm}.$$
(8) In the graph, one can see the so-called FM threshold effect.
- For $10 · \lg \hspace{0.08cm} ξ = 15 \ \rm dB$ one obtains exactly the same sink SNR for the WM system as for the AM system.
- Thus, the transmit power can be $35 \ \rm dB$ less than s $100 \ \rm kW$:
- $$ 10 \cdot {\rm lg} \hspace{0.15cm}\frac{P_{\rm S,\hspace{0.05cm}min}}{100\,{\rm kW}}= -35\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} \frac{P_{\rm S,\hspace{0.05cm}min}}{100\,{\rm kW}}\approx 0.0003\hspace{0.3cm}\Rightarrow \hspace{0.3cm}P_{\rm S,\hspace{0.05cm}min} \hspace{0.15cm}\underline {\approx 30\,{\rm W}}\hspace{0.05cm}.$$