Difference between revisions of "Aufgaben:Exercise 1.1: Basic Transmission Pulses"
| (26 intermediate revisions by 7 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/System_Components_of_a_Baseband_Transmission_System |
}} | }} | ||
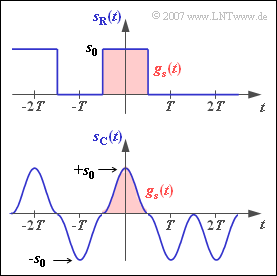
| − | [[File:P_ID1256__Dig_A_1_1.png|right|frame| | + | [[File:P_ID1256__Dig_A_1_1.png|right|frame|Considered basic transmission pulses]] |
| − | + | In this exercise, we examine the two transmitted signals $s_{\rm R}(t)$ and $s_{\rm C}(t)$ with rectangular resp. cosine–square basic transmission pulse, shown in the diagram. | |
| − | * | + | |
| + | In particular, the following characteristics are to be calculated for the respective basic transmission pulses $g_s(t)$: | ||
| + | *the equivalent pulse duration of $g_s(t)$: | ||
:$$\Delta t_{\rm S} = \frac {\int ^{+\infty} _{-\infty} \hspace{0.15cm} g_s(t)\,{\rm | :$$\Delta t_{\rm S} = \frac {\int ^{+\infty} _{-\infty} \hspace{0.15cm} g_s(t)\,{\rm | ||
d}t}{{\rm Max} \hspace{0.05cm}[g_s(t)]} \hspace{0.05cm},$$ | d}t}{{\rm Max} \hspace{0.05cm}[g_s(t)]} \hspace{0.05cm},$$ | ||
| − | * | + | *the energy of $g_s(t)$: |
:$$E_g = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm | :$$E_g = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm | ||
d}t \hspace{0.05cm},$$ | d}t \hspace{0.05cm},$$ | ||
| − | * | + | *the power of the transmitted signal $s(t)$: |
:$$P_{\rm S} = \lim_{T_{\rm M} \to \infty} \frac{1}{+T_{\rm M}} \cdot \int^{+T_{\rm M}/2} _{-T_{\rm M}/2} s^2(t)\,{\rm | :$$P_{\rm S} = \lim_{T_{\rm M} \to \infty} \frac{1}{+T_{\rm M}} \cdot \int^{+T_{\rm M}/2} _{-T_{\rm M}/2} s^2(t)\,{\rm | ||
d}t \hspace{0.05cm}.$$ | d}t \hspace{0.05cm}.$$ | ||
| − | + | Always assume in your calculations that the two possible amplitude coefficients are equally likely and that the distance between adjacent symbols is $T = 1 \ \rm µ s$. This corresponds to a bit rate of $R = 1 \ \rm Mbit/s$. | |
| − | * | + | *The (positive) maximum value of the transmitted signal is the same in both cases: |
:$$s_0 = \sqrt{0.5\, {\rm W}} \hspace{0.05cm}.$$ | :$$s_0 = \sqrt{0.5\, {\rm W}} \hspace{0.05cm}.$$ | ||
| − | * | + | *Assuming that the transmitter is terminated with a $50\ \rm Ω$ resistor, this corresponds to the following voltage value: |
| − | s_0 = \ | + | :$$s_0^2 = 0.5\, {\rm W} \cdot 50\, {\rm \Omega} = 25\, {\rm V}^2 \hspace{0.15cm} \Rightarrow \hspace{0.15cm} s_0 =5\, {\rm V} \hspace{0.05cm}.$$ |
| + | |||
| + | |||
| − | + | ||
| − | * | + | Notes: |
| − | * | + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/System_Components_of_a_Baseband_Transmission_System|"System Components of a Baseband Transmission System"]]. |
| − | * | + | *Reference is made in particular to the section [[Digital_Signal_Transmission/System_Components_of_a_Baseband_Transmission_System#Characteristics_of_the_digital_transmitter|"Characteristics of the digital transmitter"]]. |
| − | + | *Given is the following indefinite integral: | |
:$$\int \cos^4(a x)\,{\rm d}x = \frac{3}{8} \cdot x + \frac{1}{4a} \cdot \sin(2 a x)+ \frac{1}{32a} \cdot \sin(4 a | :$$\int \cos^4(a x)\,{\rm d}x = \frac{3}{8} \cdot x + \frac{1}{4a} \cdot \sin(2 a x)+ \frac{1}{32a} \cdot \sin(4 a | ||
x)\hspace{0.05cm}.$$ | x)\hspace{0.05cm}.$$ | ||
| Line 35: | Line 39: | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | + | Are $s_{\rm R}(t)$ and $s_{\rm C}(t)$ unipolar or bipolar signals? | |
| − | |type=" | + | |type="()"} |
| − | - $s_{\rm R}(t)$ | + | - $s_{\rm R}(t)$ is a bipolar signal and $s_{\rm C}(t)$ is a unipolar signal. |
| − | + $s_{\rm | + | + $s_{\rm C}(t)$ is a bipolar signal and $s_{\rm R}(t)$ is a unipolar signal. |
| − | { | + | {What is the equivalent pulse duration $\Delta t_{\rm S}$, normalized to the symbol duration $T$? |
|type="{}"} | |type="{}"} | ||
| − | $\text{ | + | $\text{For the signal}\ \ s_{\rm R}(t) \text{:} \ \ \Delta t_{\rm S}/T \ = \ $ { 1 3% } |
| − | $\text{ | + | $\text{For the signal}\ \ s_{\rm C}(t) \text{:} \ \ \Delta t_{\rm S}/T \ = \ $ { 0.5 3% } |
| − | { | + | {What is the energy of the rectangular basic transmission pulse $g_s(t)$? |
|type="{}"} | |type="{}"} | ||
$E_g \ = \ $ { 0.5 } $\ \cdot 10^{-6}\ \rm Ws$ | $E_g \ = \ $ { 0.5 } $\ \cdot 10^{-6}\ \rm Ws$ | ||
| − | { | + | {What is the power of the rectangular transmitted signal $s_{\rm R}(t)$? |
|type="{}"} | |type="{}"} | ||
$P_{\rm S} \ = \ $ { 0.25 3% } $\ \rm W$ | $P_{\rm S} \ = \ $ { 0.25 3% } $\ \rm W$ | ||
| − | { | + | {What is the energy of the cosine–square basic transmission pulse $g_s(t)$? |
|type="{}"} | |type="{}"} | ||
$E_g \ = \ $ { 0.1875 3% } $\ \cdot 10^{-6}\ \rm Ws$ | $E_g \ = \ $ { 0.1875 3% } $\ \cdot 10^{-6}\ \rm Ws$ | ||
| − | { | + | {What is the power of the transmitted signal $s_{\rm C}(t)$? |
|type="{}"} | |type="{}"} | ||
$P_{\rm S} \ = \ $ { 0.1875 3% } $\ \rm W$ | $P_{\rm S} \ = \ $ { 0.1875 3% } $\ \rm W$ | ||
| Line 68: | Line 72: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Solution 2</u> is correct: |
| + | *In both cases, the transmitted signal can be represented in the following form: | ||
| + | :$$s(t) = \sum_{(\nu)} a_\nu \cdot g_s ( t - \nu \cdot T)$$ | ||
| + | *For the signal $s_{\rm R}(t)$, the amplitude coefficients $a_ν$ are either $0$ or $1$. Thus, a unipolar signal is present. | ||
| + | *In contrast, for the bipolar signal $s_{\rm R}(t)$ ⇒ $a_ν ∈ \{–1, +1\}$ holds. | ||
| − | '''(2)''' | + | '''(2)''' The signal $s_{\rm R}(t)$ is NRZ ("non-return-to-zero") rectangular. |
| − | :$$ | + | *Accordingly, both the absolute pulse duration $T_{\rm S}$ and the equivalent pulse duration $\Delta t_{\rm S}$ are equal to the symbol duration $T$: |
| + | :$$T_{\rm S} / T = 1\hspace{0.05cm},\hspace{0.3cm}\Delta t_{\rm S} / T \hspace{0.1cm}\underline{ = 1 }\hspace{0.05cm}.$$ | ||
| + | *The basic transmission pulse for the signal $s_{\rm C}(t)$ is: | ||
| + | :$$g_s(t) = \left\{ \begin{array}{c} s_0 \cdot \cos^2(\pi \cdot \frac{t}{T}) \\ | ||
| + | 0 \\ \end{array} \right.\quad | ||
| + | \begin{array}{*{1}c} {\rm{for}} | ||
| + | \\ \\ \end{array}\begin{array}{*{20}c} | ||
| + | -T/2 \le t \le +T/2 \hspace{0.05cm}, \\ | ||
| + | {\rm else} \hspace{0.05cm}. \\ | ||
| + | \end{array}$$ | ||
| + | *From the diagram on the information section, we can see that the following values apply to the cosine–square pulse: | ||
| + | :$$T_{\rm S} / T = 1\hspace{0.05cm},\hspace{0.3cm}\Delta t_{\rm S} / T \hspace{0.1cm}\underline{ = 0.5} \hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | '''(3)''' For the energy of the rectangular pulse holds: | ||
| + | :$$E_g = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm | ||
| + | d}t = s_0^2 \cdot T = 0.5\, {\rm W} \cdot 1\, {\rm µ s} \hspace{0.1cm}\underline{= 0.5 \cdot 10^{-6}\, {\rm | ||
| + | Ws}}\hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | '''(4)''' For a bipolar rectangular signal, the following would apply: | ||
| + | :$$s_{\rm R}^2(t)= s_0^2 = {\rm const.} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} P_s = s_0^2 \cdot | ||
| + | \lim_{T_{\rm M} \to \infty} \frac{1}{T_{\rm M}} \cdot \int ^{T_{\rm M}/2} _{-T_{\rm M}/2} \,{\rm | ||
| + | d}t = s_0^2 \hspace{0.05cm}.$$ | ||
| + | *However, since the signal $s_{\rm R}(t)$ is unipolar here, in half the time $s_{\rm R}(t)= 0$. Thus, we get: | ||
| + | :$$P_{\rm S} = {1}/{2} \cdot s_0^2 \hspace{0.1cm}\underline{= 0.25 \,{\rm | ||
| + | W}} \hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | '''(5)''' For the energy of the cosine–square pulse holds: | ||
| + | :$$E_g = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm | ||
| + | d}t = 2 \cdot s_0^2 \cdot \int^{T/2} _{0} \cos^4(\pi \cdot {t}/{T})\,{\rm | ||
| + | d}t \hspace{0.05cm}.$$ | ||
| + | *Here, the formula derived in subtask '''(3)''' and the symmetry of $g_s(t)$ about time $t = 0$ are considered. | ||
| + | *The integral is given in the task description, where $a = π/T$ is to be set: | ||
| + | :$$E_g = 2 \cdot s_0^2 \cdot \left [ \frac{3}{8} \cdot t + \frac{T}{4\pi} \cdot \sin(2 \pi \frac{t}{T})+ \frac{T}{32\pi} \cdot | ||
| + | \sin(4 \pi \frac{t}{T})\right ]_{0}^{T/2}\hspace{0.05cm}.$$ | ||
| + | *The lower bound $t = 0$ always yields the result $0$. With respect to the upper bound, only the first term yields a result different from $0$. Thus: | ||
| + | :$$E_g = 2 \cdot s_0^2 \cdot \frac{3}{8} \cdot \frac{T}{2} = \frac{3}{8} \cdot 5 \cdot 10^{-7}\, {\rm | ||
| + | Ws} \hspace{0.1cm}\underline{ = 0.1875 \cdot 10^{-6}\, {\rm | ||
| + | Ws}}\hspace{0.05cm}.$$ | ||
| − | |||
| − | |||
| − | '''( | + | '''(6)''' The following relationship holds for the bipolar signal $s_{\rm C}(t)$: |
| + | :$$P_{\rm S} = \frac{ E_g}{T} = \frac{ 1.875 \cdot 10^{-7}\, {\rm | ||
| + | Ws}}{10^{-6}\, {\rm s}}\hspace{0.1cm}\underline{ = 0.1875 \,{\rm W}} \hspace{0.05cm}.$$ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^1.1 Baseband System Components^]] |
Latest revision as of 14:13, 9 May 2022
In this exercise, we examine the two transmitted signals $s_{\rm R}(t)$ and $s_{\rm C}(t)$ with rectangular resp. cosine–square basic transmission pulse, shown in the diagram.
In particular, the following characteristics are to be calculated for the respective basic transmission pulses $g_s(t)$:
- the equivalent pulse duration of $g_s(t)$:
- $$\Delta t_{\rm S} = \frac {\int ^{+\infty} _{-\infty} \hspace{0.15cm} g_s(t)\,{\rm d}t}{{\rm Max} \hspace{0.05cm}[g_s(t)]} \hspace{0.05cm},$$
- the energy of $g_s(t)$:
- $$E_g = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm d}t \hspace{0.05cm},$$
- the power of the transmitted signal $s(t)$:
- $$P_{\rm S} = \lim_{T_{\rm M} \to \infty} \frac{1}{+T_{\rm M}} \cdot \int^{+T_{\rm M}/2} _{-T_{\rm M}/2} s^2(t)\,{\rm d}t \hspace{0.05cm}.$$
Always assume in your calculations that the two possible amplitude coefficients are equally likely and that the distance between adjacent symbols is $T = 1 \ \rm µ s$. This corresponds to a bit rate of $R = 1 \ \rm Mbit/s$.
- The (positive) maximum value of the transmitted signal is the same in both cases:
- $$s_0 = \sqrt{0.5\, {\rm W}} \hspace{0.05cm}.$$
- Assuming that the transmitter is terminated with a $50\ \rm Ω$ resistor, this corresponds to the following voltage value:
- $$s_0^2 = 0.5\, {\rm W} \cdot 50\, {\rm \Omega} = 25\, {\rm V}^2 \hspace{0.15cm} \Rightarrow \hspace{0.15cm} s_0 =5\, {\rm V} \hspace{0.05cm}.$$
Notes:
- The exercise belongs to the chapter "System Components of a Baseband Transmission System".
- Reference is made in particular to the section "Characteristics of the digital transmitter".
- Given is the following indefinite integral:
- $$\int \cos^4(a x)\,{\rm d}x = \frac{3}{8} \cdot x + \frac{1}{4a} \cdot \sin(2 a x)+ \frac{1}{32a} \cdot \sin(4 a x)\hspace{0.05cm}.$$
Questions
Solution
- In both cases, the transmitted signal can be represented in the following form:
- $$s(t) = \sum_{(\nu)} a_\nu \cdot g_s ( t - \nu \cdot T)$$
- For the signal $s_{\rm R}(t)$, the amplitude coefficients $a_ν$ are either $0$ or $1$. Thus, a unipolar signal is present.
- In contrast, for the bipolar signal $s_{\rm R}(t)$ ⇒ $a_ν ∈ \{–1, +1\}$ holds.
(2) The signal $s_{\rm R}(t)$ is NRZ ("non-return-to-zero") rectangular.
- Accordingly, both the absolute pulse duration $T_{\rm S}$ and the equivalent pulse duration $\Delta t_{\rm S}$ are equal to the symbol duration $T$:
- $$T_{\rm S} / T = 1\hspace{0.05cm},\hspace{0.3cm}\Delta t_{\rm S} / T \hspace{0.1cm}\underline{ = 1 }\hspace{0.05cm}.$$
- The basic transmission pulse for the signal $s_{\rm C}(t)$ is:
- $$g_s(t) = \left\{ \begin{array}{c} s_0 \cdot \cos^2(\pi \cdot \frac{t}{T}) \\ 0 \\ \end{array} \right.\quad \begin{array}{*{1}c} {\rm{for}} \\ \\ \end{array}\begin{array}{*{20}c} -T/2 \le t \le +T/2 \hspace{0.05cm}, \\ {\rm else} \hspace{0.05cm}. \\ \end{array}$$
- From the diagram on the information section, we can see that the following values apply to the cosine–square pulse:
- $$T_{\rm S} / T = 1\hspace{0.05cm},\hspace{0.3cm}\Delta t_{\rm S} / T \hspace{0.1cm}\underline{ = 0.5} \hspace{0.05cm}.$$
(3) For the energy of the rectangular pulse holds:
- $$E_g = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm d}t = s_0^2 \cdot T = 0.5\, {\rm W} \cdot 1\, {\rm µ s} \hspace{0.1cm}\underline{= 0.5 \cdot 10^{-6}\, {\rm Ws}}\hspace{0.05cm}.$$
(4) For a bipolar rectangular signal, the following would apply:
- $$s_{\rm R}^2(t)= s_0^2 = {\rm const.} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} P_s = s_0^2 \cdot \lim_{T_{\rm M} \to \infty} \frac{1}{T_{\rm M}} \cdot \int ^{T_{\rm M}/2} _{-T_{\rm M}/2} \,{\rm d}t = s_0^2 \hspace{0.05cm}.$$
- However, since the signal $s_{\rm R}(t)$ is unipolar here, in half the time $s_{\rm R}(t)= 0$. Thus, we get:
- $$P_{\rm S} = {1}/{2} \cdot s_0^2 \hspace{0.1cm}\underline{= 0.25 \,{\rm W}} \hspace{0.05cm}.$$
(5) For the energy of the cosine–square pulse holds:
- $$E_g = \int^{+\infty} _{-\infty} g_s^2(t)\,{\rm d}t = 2 \cdot s_0^2 \cdot \int^{T/2} _{0} \cos^4(\pi \cdot {t}/{T})\,{\rm d}t \hspace{0.05cm}.$$
- Here, the formula derived in subtask (3) and the symmetry of $g_s(t)$ about time $t = 0$ are considered.
- The integral is given in the task description, where $a = π/T$ is to be set:
- $$E_g = 2 \cdot s_0^2 \cdot \left [ \frac{3}{8} \cdot t + \frac{T}{4\pi} \cdot \sin(2 \pi \frac{t}{T})+ \frac{T}{32\pi} \cdot \sin(4 \pi \frac{t}{T})\right ]_{0}^{T/2}\hspace{0.05cm}.$$
- The lower bound $t = 0$ always yields the result $0$. With respect to the upper bound, only the first term yields a result different from $0$. Thus:
- $$E_g = 2 \cdot s_0^2 \cdot \frac{3}{8} \cdot \frac{T}{2} = \frac{3}{8} \cdot 5 \cdot 10^{-7}\, {\rm Ws} \hspace{0.1cm}\underline{ = 0.1875 \cdot 10^{-6}\, {\rm Ws}}\hspace{0.05cm}.$$
(6) The following relationship holds for the bipolar signal $s_{\rm C}(t)$:
- $$P_{\rm S} = \frac{ E_g}{T} = \frac{ 1.875 \cdot 10^{-7}\, {\rm Ws}}{10^{-6}\, {\rm s}}\hspace{0.1cm}\underline{ = 0.1875 \,{\rm W}} \hspace{0.05cm}.$$