Difference between revisions of "Aufgaben:Exercise 3.3Z: Optimization of a Coaxial Cable System"
| (22 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Digital_Signal_Transmission/Consideration_of_Channel_Distortion_and_Equalization |
}} | }} | ||
| − | [[File:P_ID1409__Dig_Z_3_3.png|right|frame| | + | [[File:P_ID1409__Dig_Z_3_3.png|right|frame|Normalized system parameters for different cutoff frequencies]] |
| − | + | We consider a redundancy-free binary transmission system with the following specifications: | |
| − | * | + | * The transmission pulses are NRZ rectangular and have energy $E_{\rm B} = s_0^2 \cdot T$. |
| − | * | + | |
| − | + | * The channel is a coaxial cable with characteristic cable attenuation $a_* = 40 \, {\rm dB}$. | |
| − | |||
| + | * AWGN noise with (one-sided) noise power density $N_0 = 0.0001 \cdot E_{\rm B}$ is present. | ||
| − | + | * The receiver frequency response $H_{\rm E}(f)$ includes an ideal channel equalizer $H_{\rm K}^{\rm -1}(f)$ and a Gaussian low-pass filter $H_{\rm G}(f)$ with cutoff frequency $f_{\rm G}$ for noise power limitation. | |
| + | |||
| + | |||
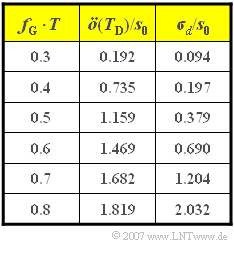
| + | The table shows the eye opening $\ddot{o}(T_{\rm D})$ as well as the detection noise rms value $\sigma_{\rm d}$ – each normalized to the transmitted amplitude $s_0$ – for different cutoff frequencies $f_{\rm G}$. The cutoff frequency is to be chosen such that the worst-case error probability is as small as possible, with the following definition: | ||
:$$p_{\rm U} = {\rm Q} \left( \frac{\ddot{o}(T_{\rm D})/2}{ \sigma_d} | :$$p_{\rm U} = {\rm Q} \left( \frac{\ddot{o}(T_{\rm D})/2}{ \sigma_d} | ||
\right) \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U} = {\rm Q} \left( \sqrt{\rho_{\rm U}}\right)$$ | \right) \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U} = {\rm Q} \left( \sqrt{\rho_{\rm U}}\right)$$ | ||
| − | + | *This quantity represents an upper bound for the mean error probability $p_{\rm S} \le p_{\rm U}$. | |
| − | :$ | + | |
| − | + | *For $f_{\rm G} \cdot T ≥ 0.4$, a lower bound can also be given: $p_{\rm S} \ge p_{\rm U}/4$. | |
| + | |||
| + | |||
| + | Notes: | ||
| + | *The exercise belongs to the chapter [[Digital_Signal_Transmission/Consideration_of_Channel_Distortion_and_Equalization|"Consideration of Channel Distortion and Equalization"]]. | ||
| − | + | * Use the interaction module [[Applets:Komplementäre_Gaußsche_Fehlerfunktionen|"Complementary Gaussian Error Functions"]] for numerical evaluation of the Q-function. | |
| − | * | + | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Within the given grid, determine the optimal cutoff frequency with respect to the "worst-case error probability" criterion. |
|type="{}"} | |type="{}"} | ||
| − | $f_{ | + | $f_\text{G, opt} \cdot T \ = \ $ { 0.4 3% } |
| − | { | + | {What values does this give for the "worst-case signal-to-noise ratio" and the worst-case error probability? |
|type="{}"} | |type="{}"} | ||
| − | $f_{\ | + | $f_\text{G} = \text{G, opt:}\hspace{0.4cm} 10 \cdot {\rm lg} \, \rho_{\rm U} \ = \ $ { 5.41 3% } ${\ \rm dB}$ |
| − | $ | + | $\hspace{4.07cm}p_{\rm U} \ = \ $ { 3.1 3% } $\ \rm \%$ |
| − | { | + | {To what value would we need to reduce the noise power density $N_0$ (with respect to signal energy) so that $p_{\rm U}$ is not greater than $10^{\rm -6}$? |
|type="{}"} | |type="{}"} | ||
| − | $N_0/E_{\rm B}$ | + | $N_0/E_{\rm B} \ = \ $ { 1.53 3% } $\ \cdot 10^{\rm -5}$ |
| − | { | + | {For the assumptions made in '''(3)''', give a lower and an upper bound for the "average error probability" $p_{\rm S}$. |
|type="{}"} | |type="{}"} | ||
| − | $p_{ | + | $p_\text{ S, min}\hspace{0.02cm} \ = \ $ { 0.25 3% } $\ \cdot 10^{\rm -6}$ |
| − | $p_{ | + | $p_\text{ S, max} \ = \ $ { 1 3% } $\ \cdot 10^{\rm -6}$ |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' For the optimization it is sufficient to maximize the quotient $\ddot{o}(T_{\rm D})/\sigma_d$: |
| + | *This is maximized from the values given in the table for the cutoff frequency $f_{\rm G, opt} \cdot T \underline {= 0.4}$ with $0.735/0.197 \approx 3.73$. | ||
| + | *As a comparison: For $f_{\rm G} \cdot T = 0.3$ the result is $0.192/0.094 \approx 2.04$ due to the smaller eye opening. | ||
| + | *For $f_{\rm G} \cdot T = 0.5$ the quotient is also smaller than for the optimum: $1.159/0.379 \approx 3.05$. | ||
| + | *An even larger cutoff frequency leads to a very large noise rms value without simultaneously increasing the vertical eye opening in the same way. | ||
| − | |||
| − | '''(2)''' | + | '''(2)''' Using the result from '''(1)''', we further obtain: |
:$$\rho_{\rm U} = \left ( {3.73}/{2} \right )^2 \approx 3.48 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} | :$$\rho_{\rm U} = \left ( {3.73}/{2} \right )^2 \approx 3.48 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} | ||
10 \cdot {\rm | 10 \cdot {\rm | ||
| − | lg}\hspace{0.1cm}\rho_{\rm U} \hspace{0.15cm}\underline { = 5.41\,{\rm dB}} | + | lg}\hspace{0.1cm}\rho_{\rm U} \hspace{0.15cm}\underline { = 5.41\,{\rm dB}}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_{\rm U} = {\rm Q}\left ( |
| − | |||
{3.73}/{2} \right) \hspace{0.15cm}\underline {\approx 0.031} \hspace{0.05cm}.$$ | {3.73}/{2} \right) \hspace{0.15cm}\underline {\approx 0.031} \hspace{0.05cm}.$$ | ||
| − | '''(3)''' | + | '''(3)''' With the given $10 \cdot {\rm lg} \, E_{\rm B}/N_0 = 40 \ \rm dB$, i.e. $E_{\rm B}/N_0 = 10^4$, the worst-case signal-to-noise ratio has been found to be $10 \cdot {\rm lg} \, \rho_{\rm U} \approx 5.41 \, {\rm dB}$. |
| + | *However, for the worst-case error probability $p_{\rm U} = 10^{\rm -6}$ ⇒ $10 \cdot {\rm lg} \, \rho_{\rm U} > 13.55 \, {\rm dB}$ must be obtained. | ||
| + | *This is achieved by increasing the quotient $E_{\rm B}/N_0$ accordingly: | ||
:$$10 \cdot {\rm | :$$10 \cdot {\rm | ||
lg}\hspace{0.1cm}{E_{\rm B}}/{N_0} = 40\,{\rm dB} | lg}\hspace{0.1cm}{E_{\rm B}}/{N_0} = 40\,{\rm dB} | ||
\hspace{0.1cm}+\hspace{0.1cm}13.55\,{\rm dB} | \hspace{0.1cm}+\hspace{0.1cm}13.55\,{\rm dB} | ||
| − | \hspace{0.1cm}-\hspace{0.1cm}5.41\,{\rm dB}= 48.14\,{\rm dB} | + | \hspace{0.1cm}-\hspace{0.1cm}5.41\,{\rm dB}= 48.14\,{\rm dB}\hspace{0.3cm} |
| − | + | \Rightarrow | |
\hspace{0.3cm} {E_{\rm B}}/{N_0} = 10^{4.814}\approx 65163 | \hspace{0.3cm} {E_{\rm B}}/{N_0} = 10^{4.814}\approx 65163 | ||
\hspace{0.3cm}\Rightarrow \hspace{0.3cm} {N_0}/{E_{\rm B}}\hspace{0.15cm}\underline { = | \hspace{0.3cm}\Rightarrow \hspace{0.3cm} {N_0}/{E_{\rm B}}\hspace{0.15cm}\underline { = | ||
| Line 77: | Line 83: | ||
| − | '''(4)''' | + | '''(4)''' |
| + | *The upper bound for $p_{\rm S}$ is equal to the worst-case error probability $p_{\rm U} = \underline {10^{\rm -6}}$. | ||
| + | |||
| + | *The lower bound is $\underline {0.25 \cdot 10^{\rm -6}}$, which is smaller by a factor of $4$. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Digital Signal Transmission: Exercises|^3.3 Channel Distortion and Equalization^]] |
Latest revision as of 14:06, 28 June 2022
We consider a redundancy-free binary transmission system with the following specifications:
- The transmission pulses are NRZ rectangular and have energy $E_{\rm B} = s_0^2 \cdot T$.
- The channel is a coaxial cable with characteristic cable attenuation $a_* = 40 \, {\rm dB}$.
- AWGN noise with (one-sided) noise power density $N_0 = 0.0001 \cdot E_{\rm B}$ is present.
- The receiver frequency response $H_{\rm E}(f)$ includes an ideal channel equalizer $H_{\rm K}^{\rm -1}(f)$ and a Gaussian low-pass filter $H_{\rm G}(f)$ with cutoff frequency $f_{\rm G}$ for noise power limitation.
The table shows the eye opening $\ddot{o}(T_{\rm D})$ as well as the detection noise rms value $\sigma_{\rm d}$ – each normalized to the transmitted amplitude $s_0$ – for different cutoff frequencies $f_{\rm G}$. The cutoff frequency is to be chosen such that the worst-case error probability is as small as possible, with the following definition:
- $$p_{\rm U} = {\rm Q} \left( \frac{\ddot{o}(T_{\rm D})/2}{ \sigma_d} \right) \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U} = {\rm Q} \left( \sqrt{\rho_{\rm U}}\right)$$
- This quantity represents an upper bound for the mean error probability $p_{\rm S} \le p_{\rm U}$.
- For $f_{\rm G} \cdot T ≥ 0.4$, a lower bound can also be given: $p_{\rm S} \ge p_{\rm U}/4$.
Notes:
- The exercise belongs to the chapter "Consideration of Channel Distortion and Equalization".
- Use the interaction module "Complementary Gaussian Error Functions" for numerical evaluation of the Q-function.
Questions
Solution
- This is maximized from the values given in the table for the cutoff frequency $f_{\rm G, opt} \cdot T \underline {= 0.4}$ with $0.735/0.197 \approx 3.73$.
- As a comparison: For $f_{\rm G} \cdot T = 0.3$ the result is $0.192/0.094 \approx 2.04$ due to the smaller eye opening.
- For $f_{\rm G} \cdot T = 0.5$ the quotient is also smaller than for the optimum: $1.159/0.379 \approx 3.05$.
- An even larger cutoff frequency leads to a very large noise rms value without simultaneously increasing the vertical eye opening in the same way.
(2) Using the result from (1), we further obtain:
- $$\rho_{\rm U} = \left ( {3.73}/{2} \right )^2 \approx 3.48 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm U} \hspace{0.15cm}\underline { = 5.41\,{\rm dB}}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} p_{\rm U} = {\rm Q}\left ( {3.73}/{2} \right) \hspace{0.15cm}\underline {\approx 0.031} \hspace{0.05cm}.$$
(3) With the given $10 \cdot {\rm lg} \, E_{\rm B}/N_0 = 40 \ \rm dB$, i.e. $E_{\rm B}/N_0 = 10^4$, the worst-case signal-to-noise ratio has been found to be $10 \cdot {\rm lg} \, \rho_{\rm U} \approx 5.41 \, {\rm dB}$.
- However, for the worst-case error probability $p_{\rm U} = 10^{\rm -6}$ ⇒ $10 \cdot {\rm lg} \, \rho_{\rm U} > 13.55 \, {\rm dB}$ must be obtained.
- This is achieved by increasing the quotient $E_{\rm B}/N_0$ accordingly:
- $$10 \cdot {\rm lg}\hspace{0.1cm}{E_{\rm B}}/{N_0} = 40\,{\rm dB} \hspace{0.1cm}+\hspace{0.1cm}13.55\,{\rm dB} \hspace{0.1cm}-\hspace{0.1cm}5.41\,{\rm dB}= 48.14\,{\rm dB}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} {E_{\rm B}}/{N_0} = 10^{4.814}\approx 65163 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} {N_0}/{E_{\rm B}}\hspace{0.15cm}\underline { = 1.53 \cdot 10^{-5}} \hspace{0.05cm}.$$
(4)
- The upper bound for $p_{\rm S}$ is equal to the worst-case error probability $p_{\rm U} = \underline {10^{\rm -6}}$.
- The lower bound is $\underline {0.25 \cdot 10^{\rm -6}}$, which is smaller by a factor of $4$.