Difference between revisions of "Aufgaben:Exercise 2.3Z: Polynomial Division"
| (19 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Extension_Field}} |
| − | [[File:P_ID2504__KC_Z_2_3.png|right|frame| | + | [[File:P_ID2504__KC_Z_2_3.png|right|frame|Multiplication and division of polynomials in GF(2) <br><u>Note.</u> remainder r(x)]] |
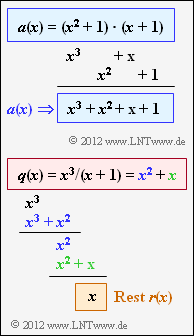
| − | In | + | In this exercise we deal with the multiplication and especially the division of polynomials in the Galois field GF(2). In the graph the procedure is indicated by a simple and (hopefully) self-explanatory example: |
| − | * | + | * Multiplying the two polynomials x2+1 and x+1 yields the result a(x)=x3+x2+x+1. |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | * Dividing the polynomial b(x)=x3 by p(x)=x+1 gives the quotient q(x)=x2+x and the remainder r(x)=x. | |
| − | * | ||
| + | * One can check the latter result as follows: | ||
| + | :b(x) = p(x)⋅q(x)+r(x)=[(x+1)⋅(x2+x)]+x=[x3+x2+x2+x]+x=x3. | ||
| + | Hint: This exercise belongs to the chapter [[Channel_Coding/Extension_Field| "Extension field"]]. | ||
| − | === | + | |
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the result a(x)=(x3+x+1)⋅(x2+1)? |
| − | |type=" | + | |type="()"} |
- a(x)=x5+x3+x2+1, | - a(x)=x5+x3+x2+1, | ||
+ a(x)=x5+x2+x+1. | + a(x)=x5+x2+x+1. | ||
- a(x)=x6+x3+x2+1- | - a(x)=x6+x3+x2+1- | ||
| − | { | + | {Which of the polynomial divisions do not yield a remainder $r(x) \ne 0$? |
|type="[]"} | |type="[]"} | ||
+ (x5+x2+x+1)/(x3+x+1). | + (x5+x2+x+1)/(x3+x+1). | ||
| Line 33: | Line 33: | ||
- (x5+x2+x)/(x2+1). | - (x5+x2+x)/(x2+1). | ||
| − | { | + | {It is a(x)=x6+x5+1 and p(x)=x3+x2+1. <br>Determine q(x) and r(x) according to the description equation a(x)=p(x)⋅q(x)+r(x). |
| − | |type=" | + | |type="()"} |
- q(x)=x3+x2+1,r(x)=0, | - q(x)=x3+x2+1,r(x)=0, | ||
- q(x)=x3+1,r(x)=0, | - q(x)=x3+1,r(x)=0, | ||
| Line 40: | Line 40: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The modulo-2 multiplication of the two polynomials leads to the result |
| − | :$$a(x) \hspace{-0.15cm} \ = \ \hspace{-0.15cm} (x^3+ x+1) \cdot (x^2+1)= | + | :a(x) = (x3+x+1)⋅(x2+1)=x5+x3+x2+x3+x+1=x5+x2+x+1. |
| − | |||
| − | + | *Thus the <u>proposed solution 2</u> is correct. | |
| + | *The last solution suggestion cannot be valid already alone, since the degree of the product polynomial would be unequal 5. | ||
| − | '''(2)''' | + | |
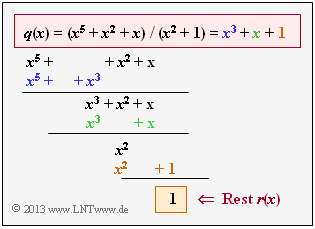
| + | [[File:P_ID2506__KC_Z_2_3b_neu.png|right|frame|'''Example 1''' for polynomial division]] | ||
| + | '''(2)''' With the abbreviations | ||
:a(x)=x5+x2+x+1,p(x)=x3+x+1,q(x)=x2+1 | :a(x)=x5+x2+x+1,p(x)=x3+x+1,q(x)=x2+1 | ||
| − | + | and the result from subtask '''(1)''' we get a(x)=p(x)⋅q(x). | |
| − | + | ||
| + | That is: The divisions a(x)/p(x) and a(x)/q(x) are free of remainders | ||
| + | ⇒ Correct are the <u>solutions 1 and 2</u>. | ||
| − | + | Even without calculation one recognizes that a(x)/x2 must result in a remainder. After calculation it results explicitly: | |
| + | :$$(x^5 + x^2+x+1)/(x^2) = x^3 + 1 \hspace{0.05cm},\hspace{0.4cm}{\rm remainder}\hspace{0.15cm} r(x) = x+1\hspace{0.05cm}.$$ | ||
| − | + | To the last proposed solution: We use for shortcut b(x)=x5+x2+x=a(x)+1. This is the given quotient: | |
:b(x)/q(x)=a(x)/q(x)+1/q(x). | :b(x)/q(x)=a(x)/q(x)+1/q(x). | ||
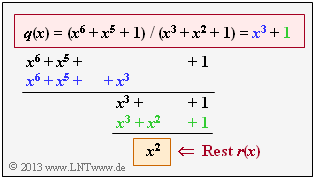
| − | + | [[File:P_ID2505__KC_Z_2_3c.png|Right|frame|'''Example 2''' for polynomial division]] | |
| + | *The first quotient a(x)/q(x) gives exactly p(x) without remainder, the second quotient 0, remainder 1. | ||
| + | |||
| + | *Thus the remainder of the quotient b(x)/q(x) is equal to r(x)=1, as the calculation in Example 1 shows. | ||
| − | '''(3)''' | + | '''(3)''' The polynomial division is explained in detail in Example 2. Correct is the <u>proposed solution 3</u>. |
| + | |||
| − | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Channel Coding: Exercises|^2.2 Extension Field^]] |
Latest revision as of 18:17, 29 September 2022
In this exercise we deal with the multiplication and especially the division of polynomials in the Galois field GF(2). In the graph the procedure is indicated by a simple and (hopefully) self-explanatory example:
- Multiplying the two polynomials x2+1 and x+1 yields the result a(x)=x3+x2+x+1.
- Dividing the polynomial b(x)=x3 by p(x)=x+1 gives the quotient q(x)=x2+x and the remainder r(x)=x.
- One can check the latter result as follows:
- b(x) = p(x)⋅q(x)+r(x)=[(x+1)⋅(x2+x)]+x=[x3+x2+x2+x]+x=x3.
Hint: This exercise belongs to the chapter "Extension field".
Questions
Solution
- a(x) = (x3+x+1)⋅(x2+1)=x5+x3+x2+x3+x+1=x5+x2+x+1.
- Thus the proposed solution 2 is correct.
- The last solution suggestion cannot be valid already alone, since the degree of the product polynomial would be unequal 5.
(2) With the abbreviations
- a(x)=x5+x2+x+1,p(x)=x3+x+1,q(x)=x2+1
and the result from subtask (1) we get a(x)=p(x)⋅q(x).
That is: The divisions a(x)/p(x) and a(x)/q(x) are free of remainders ⇒ Correct are the solutions 1 and 2.
Even without calculation one recognizes that a(x)/x2 must result in a remainder. After calculation it results explicitly:
- (x5+x2+x+1)/(x2)=x3+1,remainderr(x)=x+1.
To the last proposed solution: We use for shortcut b(x)=x5+x2+x=a(x)+1. This is the given quotient:
- b(x)/q(x)=a(x)/q(x)+1/q(x).
- The first quotient a(x)/q(x) gives exactly p(x) without remainder, the second quotient 0, remainder 1.
- Thus the remainder of the quotient b(x)/q(x) is equal to r(x)=1, as the calculation in Example 1 shows.
(3) The polynomial division is explained in detail in Example 2. Correct is the proposed solution 3.