Difference between revisions of "Aufgaben:Exercise 1.1: ISDN Supply Lines"
From LNTwww
| (3 intermediate revisions by the same user not shown) | |||
| Line 2: | Line 2: | ||
{{quiz-Header|Buchseite=Examples_of_Communication_Systems/General_Description_of_ISDN | {{quiz-Header|Buchseite=Examples_of_Communication_Systems/General_Description_of_ISDN | ||
}} | }} | ||
| + | [[File:EN_Bei_A_1_1.png|right|frame|Main bundle, basic bundle, and star quad]] | ||
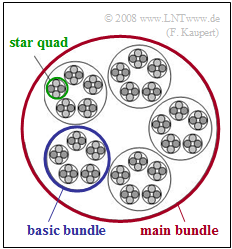
| + | In ISDN ("Integrated Services Digital Network") the final branch (near the subscriber) is connected to a "local exchange" $\rm (LE)$ by a copper twisted pair, whereby two twisted pairs are twisted into a so-called "star quad". Several such star quads are then combined to form a "basic bundle", and several basic bundles are combined to form a "main bundle" (see graphic). | ||
| − | + | In the network of "Deutsche Telekom" (formerly: "Deutsche Bundespost"), mostly copper lines with $0.4$ mm core diameter are found, for whose attenuation and phase function the following equations are given in '''[PW95]''': | |
| − | In | ||
| − | |||
| − | |||
:$$\frac{a_{\rm K}(f)}{\rm dB} = \left [ 5.1 + 14.3 \cdot \left (\frac{f}{\rm MHz}\right )^{0.59}\right ]\cdot\frac{l}{\rm km} | :$$\frac{a_{\rm K}(f)}{\rm dB} = \left [ 5.1 + 14.3 \cdot \left (\frac{f}{\rm MHz}\right )^{0.59}\right ]\cdot\frac{l}{\rm km} | ||
\hspace{0.05cm},$$ | \hspace{0.05cm},$$ | ||
:$$\frac{b_{\rm K}(f)}{\rm rad} = \left [ 32.9 \cdot \frac{f}{\rm MHz} + 2.26 \cdot \left (\frac{f}{\rm MHz}\right )^{0.5}\right ]\cdot\frac{l}{\rm km} \hspace{0.05cm}.$$ | :$$\frac{b_{\rm K}(f)}{\rm rad} = \left [ 32.9 \cdot \frac{f}{\rm MHz} + 2.26 \cdot \left (\frac{f}{\rm MHz}\right )^{0.5}\right ]\cdot\frac{l}{\rm km} \hspace{0.05cm}.$$ | ||
| − | Here $l$ denotes the | + | Here $l$ denotes the cable length. |
| + | |||
| + | |||
| + | <u>Notes:</u> | ||
| + | *The exercise belongs to the chapter [[Examples_of_Communication_Systems/General_Description_of_ISDN|"General Description of ISDN"]]. | ||
| + | *In particular, reference is made to the section [[Examples_of_Communication_Systems/General_Description_of_ISDN#Network_infrastructure_for_ISDN|"Network infrastructure for ISDN"]]. | ||
| + | *Further information on the attenuation of copper lines can be found in the chapter "Properties of Electrical Cables" of the book [[Lineare zeitinvariante Systeme|"Linear and Time Invariant Systems"]]. | ||
| − | '' | + | *'''[PW95]''' refers to the following (German language) publication: Pollakowski, P.; Wellhausen, H.-W.: Eigenschaften symmetrischer Ortsanschlusskabel im Frequenzbereich bis 30 MHz. Deutsche Telekom AG, Forschungs- und Technologiezentrum Darmstadt, 1995. |
| − | |||
| − | |||
| − | |||
| − | |||
| Line 30: | Line 31: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | {How many subscribers ( | + | {How many subscribers $(N)$ can be connected to an ISDN local exchange through the main cable shown? |
|type="{}"} | |type="{}"} | ||
$N \ = \ $ { 50 3% } | $N \ = \ $ { 50 3% } | ||
| Line 40: | Line 41: | ||
- Intersymbol interference occurs. | - Intersymbol interference occurs. | ||
| − | {A DC signal is attenuated by a factor of $4$. What is the cable length $l$ ? | + | {A DC signal is attenuated by a factor of $4$. What is the cable length $l$ ? |
|type="{}"} | |type="{}"} | ||
$l \ = \ $ { 2.36 3% } $\ \rm km$ | $l \ = \ $ { 2.36 3% } $\ \rm km$ | ||
| Line 53: | Line 54: | ||
===Solution=== | ===Solution=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' Two-wire transmission is used in the connection area. The possible connections are equal to the number of pairs in the main cable: $\underline{N = 50}$. | + | '''(1)''' Two-wire transmission is used in the connection area. The possible connections are equal to the number of pairs in the main cable: |
| + | :$$\underline{N = 50}.$$ | ||
| + | |||
| + | |||
| + | '''(2)''' <u>Solutions 1 and 2</u> are correct: | ||
| + | *Two-wire transmission requires a directional separation method, namely the so-called "fork circuit". This has the task that at receiver $\rm A$ only the transmitted signal of subscriber $\rm B$ arrives, but not the own transmitted signal. This is generally quite successful with narrowband signals – for example, speech – but not completely. | ||
| + | *Due to inductive and capacitive couplings, crosstalk can occur from the twin wire located in the same star quad, whereby "near-end crosstalk" $($i.e. the interfering transmitter and the interfered receiver are located together$)$ leads to greater impairments than "far-end crosstalk". | ||
| − | + | *On the other hand, the last solution is not applicable. Intersymbol interference – i.e. the mutual interference of neighboring symbols – can certainly occur, but it is not related to two-wire transmission. The reason for this are rather (linear) distortions due to the specific attenuation and phase curves. | |
| − | |||
| − | |||
| − | *On the other hand, the last solution is not applicable. Intersymbol interference – i.e. the mutual interference of neighboring symbols – can certainly occur, but it is not related to two-wire transmission. The reason for this are rather (linear) distortions due to the specific attenuation and phase curves. | ||
| Line 65: | Line 69: | ||
'''(3)''' The DC signal attenuation by a factor of $4$ can be expressed as follows: | '''(3)''' The DC signal attenuation by a factor of $4$ can be expressed as follows: | ||
:$$a_{\rm K}(f = 0) = 20 \cdot {\rm lg}\,\,(4) = 12.04\,{\rm dB}\hspace{0.05cm}.$$ | :$$a_{\rm K}(f = 0) = 20 \cdot {\rm lg}\,\,(4) = 12.04\,{\rm dB}\hspace{0.05cm}.$$ | ||
| − | *With the given coefficient $\text{5.1 dB/km}$, this gives the | + | *With the given coefficient $\text{5.1 dB/km}$, this gives the cable length $l = 12.04/5.1\hspace{0.15cm}\underline{ = 2.36 \ \rm km}$. |
| − | '''(4)''' Using the given equations and $ l = 2.36 \ \rm km$, we obtain: | + | '''(4)''' Using the given equations and $ l = 2.36 \ \rm km$, we obtain: |
:$$a_{\rm K}(f = 120\,{\rm kHz})= (5.1 + 14.3 \cdot 0.12^{\hspace{0.05cm}0.59}) \cdot 2.36\,{\rm dB} \hspace{0.15cm}\underline{\approx 21.7\,{\rm dB}}\hspace{0.05cm},$$ | :$$a_{\rm K}(f = 120\,{\rm kHz})= (5.1 + 14.3 \cdot 0.12^{\hspace{0.05cm}0.59}) \cdot 2.36\,{\rm dB} \hspace{0.15cm}\underline{\approx 21.7\,{\rm dB}}\hspace{0.05cm},$$ | ||
:$$b_{\rm K}(f = 120\,{\rm kHz}) = (32.9 \cdot 0.12 + 2.26 \cdot 0.12^{\hspace{0.05cm}0.5}) \cdot 2.36\,{\rm rad}\hspace{0.15cm}\underline{ \approx 11.2\,{\rm rad}}\hspace{0.05cm}.$$ | :$$b_{\rm K}(f = 120\,{\rm kHz}) = (32.9 \cdot 0.12 + 2.26 \cdot 0.12^{\hspace{0.05cm}0.5}) \cdot 2.36\,{\rm rad}\hspace{0.15cm}\underline{ \approx 11.2\,{\rm rad}}\hspace{0.05cm}.$$ | ||
Latest revision as of 15:18, 26 October 2022
Main bundle, basic bundle, and star quad
In ISDN ("Integrated Services Digital Network") the final branch (near the subscriber) is connected to a "local exchange" $\rm (LE)$ by a copper twisted pair, whereby two twisted pairs are twisted into a so-called "star quad". Several such star quads are then combined to form a "basic bundle", and several basic bundles are combined to form a "main bundle" (see graphic).
In the network of "Deutsche Telekom" (formerly: "Deutsche Bundespost"), mostly copper lines with $0.4$ mm core diameter are found, for whose attenuation and phase function the following equations are given in [PW95]:
- $$\frac{a_{\rm K}(f)}{\rm dB} = \left [ 5.1 + 14.3 \cdot \left (\frac{f}{\rm MHz}\right )^{0.59}\right ]\cdot\frac{l}{\rm km} \hspace{0.05cm},$$
- $$\frac{b_{\rm K}(f)}{\rm rad} = \left [ 32.9 \cdot \frac{f}{\rm MHz} + 2.26 \cdot \left (\frac{f}{\rm MHz}\right )^{0.5}\right ]\cdot\frac{l}{\rm km} \hspace{0.05cm}.$$
Here $l$ denotes the cable length.
Notes:
- The exercise belongs to the chapter "General Description of ISDN".
- In particular, reference is made to the section "Network infrastructure for ISDN".
- Further information on the attenuation of copper lines can be found in the chapter "Properties of Electrical Cables" of the book "Linear and Time Invariant Systems".
- [PW95] refers to the following (German language) publication: Pollakowski, P.; Wellhausen, H.-W.: Eigenschaften symmetrischer Ortsanschlusskabel im Frequenzbereich bis 30 MHz. Deutsche Telekom AG, Forschungs- und Technologiezentrum Darmstadt, 1995.
Questions
Solution
(1) Two-wire transmission is used in the connection area. The possible connections are equal to the number of pairs in the main cable:
- $$\underline{N = 50}.$$
(2) Solutions 1 and 2 are correct:
- Two-wire transmission requires a directional separation method, namely the so-called "fork circuit". This has the task that at receiver $\rm A$ only the transmitted signal of subscriber $\rm B$ arrives, but not the own transmitted signal. This is generally quite successful with narrowband signals – for example, speech – but not completely.
- Due to inductive and capacitive couplings, crosstalk can occur from the twin wire located in the same star quad, whereby "near-end crosstalk" $($i.e. the interfering transmitter and the interfered receiver are located together$)$ leads to greater impairments than "far-end crosstalk".
- On the other hand, the last solution is not applicable. Intersymbol interference – i.e. the mutual interference of neighboring symbols – can certainly occur, but it is not related to two-wire transmission. The reason for this are rather (linear) distortions due to the specific attenuation and phase curves.
(3) The DC signal attenuation by a factor of $4$ can be expressed as follows:
- $$a_{\rm K}(f = 0) = 20 \cdot {\rm lg}\,\,(4) = 12.04\,{\rm dB}\hspace{0.05cm}.$$
- With the given coefficient $\text{5.1 dB/km}$, this gives the cable length $l = 12.04/5.1\hspace{0.15cm}\underline{ = 2.36 \ \rm km}$.
(4) Using the given equations and $ l = 2.36 \ \rm km$, we obtain:
- $$a_{\rm K}(f = 120\,{\rm kHz})= (5.1 + 14.3 \cdot 0.12^{\hspace{0.05cm}0.59}) \cdot 2.36\,{\rm dB} \hspace{0.15cm}\underline{\approx 21.7\,{\rm dB}}\hspace{0.05cm},$$
- $$b_{\rm K}(f = 120\,{\rm kHz}) = (32.9 \cdot 0.12 + 2.26 \cdot 0.12^{\hspace{0.05cm}0.5}) \cdot 2.36\,{\rm rad}\hspace{0.15cm}\underline{ \approx 11.2\,{\rm rad}}\hspace{0.05cm}.$$
