Difference between revisions of "Aufgaben:Exercise 3.1Z: Convolution Codes of Rate 1/2"
From LNTwww
| (19 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Basics_of_Convolutional_Coding}} |
| − | [[File: | + | [[File:EN_KC_Z_3_1_jetztaber.png|right|frame|Convolutional codes of rate $1/2$]] |
| − | + | The graphic shows two convolutional encoders of rate $R = 1/2$: | |
| − | + | *At the input there is the information sequence $\underline {u} = (u_1, u_2, \ \text{...} \ , u_i, \ \text{...})$. | |
| − | |||
| − | |||
| − | |||
| − | + | *From this, modulo-2 operations generate the two sequences | |
| + | :$$\underline{\it x}^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \big( \hspace{0.05cm}x_1^{(1)}\hspace{0.05cm},\hspace{0.05cm} x_2^{(1)}\hspace{0.05cm},\hspace{0.05cm} \text{...} \hspace{0.05cm},\hspace{0.05cm} | ||
| + | x_i^{(1)} \hspace{0.05cm},\text{...} \hspace{0.05cm} \big )\hspace{0.05cm},$$ | ||
| + | :$$\underline{\it x}^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \big( \hspace{0.05cm}x_1^{(2)}\hspace{0.05cm},\hspace{0.05cm} x_2^{(2)}\hspace{0.05cm},\hspace{0.05cm}\text{...} \hspace{0.05cm},\hspace{0.05cm} | ||
| + | x_i^{(2)} \hspace{0.05cm}, \text{...} \hspace{0.05cm} \big )$$ | ||
| − | + | :where $x_i^{(j)}$ with $j = 1$ resp. $j = 2$ may depend except from $u_i$ also from the previous information bits $u_{i-1}, \ \text{...} \ , u_{i-m}$. | |
| − | + | *One refers $m$ as the "memory" and $\nu = m + 1$ as the "influence length" of the code $($or of the encoder$)$. | |
| − | + | * The considered encoders $\rm A$ and $\rm B$ differ with respect to these quantities. | |
| − | * | ||
| − | === | + | |
| + | <u>Hints:</u> | ||
| + | *The exercise refers to the chapter [[Channel_Coding/Basics_of_Convolutional_Coding| "Basics of Convolutional Coding"]]. | ||
| + | |||
| + | *Not shown in the diagram is the multiplexing of the two subsequences $\underline {x}^{(1)}$ and $\underline {x}^{(2)}$ to the resulting code sequence | ||
| + | :$$\underline {x} = (x_1^{(1)}, x_1^{(2)}, x_2^{(1)}, x_2^{(2)}, \ \text{...}).$$ | ||
| + | *In subtasks '''(3)''' to '''(5)''' you are to determine the start of the sequences $\underline {x}^{(1)}, \underline{x}^{(2)}$ and $\underline{x}$ assuming the information sequence | ||
| + | :$$\underline{u} = (1, 0, 1, 1, 0, 0, \ \text{. ..}).$$ | ||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | {In | + | {In which code parameters do encoder $\rm A$ and encoder $\rm B$ differ? |
|type="[]"} | |type="[]"} | ||
| − | - $k$: | + | - $k$: Number of information bits processed per coding step, |
| − | - $n$: | + | - $n$: Number of code bits output per coding step, |
| − | + $m$: | + | + $m$: memory order of the code, |
| − | + $\nu$: | + | + $\nu$: influence length of the code. |
| − | { | + | {Which encoder exhibits the memory $m = 2$? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - Encoder $\rm A$, |
| − | + | + | + encoder $\rm B$. |
| − | { | + | {What is the partial code sequence $\underline {x}^{(1)}$ of encoder $\rm B$ for $\underline {u} = (1, 0, 1, 1, 0, 0, \ \text{...})$? |
| − | |type=" | + | |type="()"} |
+ $\underline {x}^{(1)} = (1, 1, 0, 0, 0, 1, 0, 0, \ ...)$, | + $\underline {x}^{(1)} = (1, 1, 0, 0, 0, 1, 0, 0, \ ...)$, | ||
- $\underline {x}^{(1)} = (1, 0, 1, 1, 0, 0, 0, 0, \ ...)$. | - $\underline {x}^{(1)} = (1, 0, 1, 1, 0, 0, 0, 0, \ ...)$. | ||
| − | { | + | {What is the partial code sequence $\underline {x}^{(2)}$ of encoder $\rm B$ for $\underline {u} = (1, 0, 1, 1, 0, 0, \ \text{...})$ |
| − | |type=" | + | |type="()"} |
| − | - $\underline{x}^{(2)} = (1, 1, 0, 0, 0, 1, 0, 0, \ ...)$, | + | - $\underline{x}^{(2)} = (1, 1, 0, 0, 0, 1, 0, 0, \ \text{...})$, |
| − | + $\underline{x}^{(2)} = (1, 0, 0, 1, 1, 1, 0, 0, \ ...)$. | + | + $\underline{x}^{(2)} = (1, 0, 0, 1, 1, 1, 0, 0, \ \text{...})$. |
| − | { | + | {How does the entire code sequence $\underline {x}$ start from encoder $\rm B$ after multiplexing? |
| − | |type=" | + | |type="()"} |
| − | + $\underline {x} = (1, 1, 1, 0, 0, 0, 0, 1, 0, 1, 1, 1, \ ...)$, | + | + $\underline {x} = (1, 1, 1, 0, 0, 0, 0, 1, 0, 1, 1, 1, \ \text{...})$, |
| − | - $\underline {x} = (1, 0, 1, 1, 1, 0, 0, 0, 1, 0, 1, 0, \ ...)$. | + | - $\underline {x} = (1, 0, 1, 1, 1, 0, 0, 0, 1, 0, 1, 0, \ \text{...})$. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' For both encoders, $k = 1$ and $n = 2$. |
| + | |||
| + | *The memory $m$ and the influence length $\nu$ are different ⇒ <u>Answers 3 and 4</u>. | ||
| − | '''(2)''' | + | '''(2)''' The shift register of encoder $\rm A$ does contain two memory cells. However, since $x_i^{(1)} = u_i$ and $x_i^{(2)} = u_i + u_{i-1}$ is influenced only by the immediately preceding bit $u_{i-1}$ besides the current information bit $u_i$, |
| + | [[File:EN_KC_A_3_1.png|right|frame|Equivalent encoder representations]] | ||
| + | *the memory is $m = 1$, and | ||
| + | |||
| + | *the influence length is $\nu = m + 1 = 2$. | ||
| − | |||
| − | + | The graphic shows the two encoders in another representation, whereby the "memory cells" are highlighted in yellow. | |
| + | *For the encoder $\rm A$ one recognizes only one memory ⇒ $m = 1$. | ||
| + | |||
| + | *In contrast, for the encoder $\rm B$ actually $m = 2$ and $\nu = 3$. | ||
| + | *Thus, the <u>proposed solution 2</u> is correct. | ||
| − | '''(3)''' | + | |
| + | |||
| + | '''(3)''' For the upper output of encoder $\rm B$ applies in general: | ||
:$$x_i^{(1)} = u_{i} + u_{i-1}+ u_{i-2} \hspace{0.05cm}.$$ | :$$x_i^{(1)} = u_{i} + u_{i-1}+ u_{i-2} \hspace{0.05cm}.$$ | ||
| − | + | *Considering the preassignment ($(u_0 = u_{-1} = 0)$, we obtain with the above data: | |
| − | :$$x_1^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{1} + u_{0}+ u_{-1} = 1+0+0 = 1 \hspace{0.05cm},\hspace{ | + | :$$x_1^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{1} + u_{0}+ u_{-1} = 1+0+0 = 1 \hspace{0.05cm},\hspace{1cm}x_2^{(1)} = u_{2} + u_{1}+ u_{0} = 0+1+0 = 1\hspace{0.05cm},$$ |
| − | :$$x_3^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{3} + u_{2}+ u_{1} \hspace{0.25cm}= 1+0+1 = 0 \hspace{0.05cm},\hspace{ | + | :$$x_3^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{3} + u_{2}+ u_{1} \hspace{0.25cm}= 1+0+1 = 0 \hspace{0.05cm},\hspace{1cm}x_4^{(1)} = u_{4} + u_{3}+ u_{2} = 1+1+0 = 0\hspace{0.05cm},$$ |
| − | :$$x_5^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{5} + u_{4}+ u_{3} \hspace{0.25cm}= 0+1+1 = 0 \hspace{0.05cm},\hspace{ | + | :$$x_5^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{5} + u_{4}+ u_{3} \hspace{0.25cm}= 0+1+1 = 0 \hspace{0.05cm},\hspace{1cm}x_6^{(1)} = u_{6} + u_{5}+ u_{4} = 0+0+1 = 1\hspace{0.05cm},$$ |
| − | :$$x_7^{(1)} \hspace{-0.15cm} | + | :$$x_7^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} x_8^{(1)} = \text{...} \hspace{0.05cm}= 0 \hspace{0.05cm}.$$ |
| − | + | *The <u>proposed solution 1</u> is correct. The second solution suggestion ⇒ $\underline {x}^{(1)} = \underline {u}$ would only be valid for a systematic code $($which is not present here$)$. | |
| − | '''(4)''' | + | '''(4)''' Analogous to subtask '''(3)''' ⇒ $x_i^{(2)} = u_i + u_{i–2}$: |
:$$x_1^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 1+0 = 1 \hspace{0.05cm},\hspace{0.2cm}x_2^{(2)} = 0+0 = 0\hspace{0.05cm}, | :$$x_1^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 1+0 = 1 \hspace{0.05cm},\hspace{0.2cm}x_2^{(2)} = 0+0 = 0\hspace{0.05cm}, | ||
\hspace{0.2cm}x_3^{(3)} = 1+1 = 0\hspace{0.05cm},\hspace{0.2cm}x_4^{(2)} = 1+0 = 1 \hspace{0.05cm},$$ | \hspace{0.2cm}x_3^{(3)} = 1+1 = 0\hspace{0.05cm},\hspace{0.2cm}x_4^{(2)} = 1+0 = 1 \hspace{0.05cm},$$ | ||
:$$x_5^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 0+1 = 1\hspace{0.05cm}, | :$$x_5^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 0+1 = 1\hspace{0.05cm}, | ||
\hspace{0.2cm}x_6^{(2)} = 0+1 = 1\hspace{0.05cm},\hspace{0.2cm} | \hspace{0.2cm}x_6^{(2)} = 0+1 = 1\hspace{0.05cm},\hspace{0.2cm} | ||
| − | x_7^{(2)} = x_8^{(2)} = ... \hspace{0.05cm}= 0 \hspace{0.05cm}.$$ | + | x_7^{(2)} = x_8^{(2)} = \text{...} \hspace{0.05cm}= 0 \hspace{0.05cm}.$$ |
| + | |||
| + | *The correct solution is the <u>proposed solution 2</u>. | ||
| − | |||
| − | '''(5)''' | + | '''(5)''' For the $($entire$)$ code sequence, one can formally write: |
| − | :$$\underline{\it x} = \big( \hspace{0.05cm}\underline{\it x}_1\hspace{0.05cm}, \hspace{0.05cm} \underline{\it x}_2\hspace{0.05cm}, \hspace{0.05cm}...\hspace{0.05cm} \underline{\it x}_i \hspace{0.05cm}, ... | + | :$$\underline{\it x} = \big( \hspace{0.05cm}\underline{\it x}_1\hspace{0.05cm}, \hspace{0.05cm} \underline{\it x}_2\hspace{0.05cm}, \hspace{0.05cm}\text{...}\hspace{0.05cm} \underline{\it x}_i \hspace{0.05cm}, \text{...} \hspace{0.05cm} \big )\hspace{0.05cm}, \hspace{0.3cm} \underline{\it x}_i = \big( x_i^{(1)}\hspace{0.05cm}, x_i^{(2)} \big) |
| − | \hspace{0.4cm}\Rightarrow \hspace{0.4cm} \underline{\it x} = \big( \hspace{0.05cm}x_1^{(1)}\hspace{0.01cm},\hspace{0.05cm} x_2^{(1)}\hspace{0.01cm},\hspace{0.05cm} x_1^{(2)}\hspace{0.01cm},\hspace{0.05cm} x_2^{(2)}\hspace{0.01cm}, \hspace{0.05cm} ... | + | \hspace{0.4cm}\Rightarrow \hspace{0.4cm} \underline{\it x} = \big( \hspace{0.05cm}x_1^{(1)}\hspace{0.01cm},\hspace{0.05cm} x_2^{(1)}\hspace{0.01cm},\hspace{0.05cm} x_1^{(2)}\hspace{0.01cm},\hspace{0.05cm} x_2^{(2)}\hspace{0.01cm}, \hspace{0.05cm} \text{...} \hspace{0.05cm} \big )\hspace{0.05cm}. $$ |
| − | + | *A comparison with the solutions of subtasks '''(3)''' and '''(4)''' shows the correctness of the <u>proposed solution 1</u>. | |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Channel Coding: Exercises|^3.1 Basics of Convolutional Coding^]] |
Latest revision as of 18:26, 16 November 2022
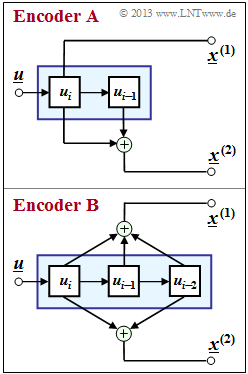
The graphic shows two convolutional encoders of rate $R = 1/2$:
- At the input there is the information sequence $\underline {u} = (u_1, u_2, \ \text{...} \ , u_i, \ \text{...})$.
- From this, modulo-2 operations generate the two sequences
- $$\underline{\it x}^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \big( \hspace{0.05cm}x_1^{(1)}\hspace{0.05cm},\hspace{0.05cm} x_2^{(1)}\hspace{0.05cm},\hspace{0.05cm} \text{...} \hspace{0.05cm},\hspace{0.05cm} x_i^{(1)} \hspace{0.05cm},\text{...} \hspace{0.05cm} \big )\hspace{0.05cm},$$
- $$\underline{\it x}^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} \big( \hspace{0.05cm}x_1^{(2)}\hspace{0.05cm},\hspace{0.05cm} x_2^{(2)}\hspace{0.05cm},\hspace{0.05cm}\text{...} \hspace{0.05cm},\hspace{0.05cm} x_i^{(2)} \hspace{0.05cm}, \text{...} \hspace{0.05cm} \big )$$
- where $x_i^{(j)}$ with $j = 1$ resp. $j = 2$ may depend except from $u_i$ also from the previous information bits $u_{i-1}, \ \text{...} \ , u_{i-m}$.
- One refers $m$ as the "memory" and $\nu = m + 1$ as the "influence length" of the code $($or of the encoder$)$.
- The considered encoders $\rm A$ and $\rm B$ differ with respect to these quantities.
Hints:
- The exercise refers to the chapter "Basics of Convolutional Coding".
- Not shown in the diagram is the multiplexing of the two subsequences $\underline {x}^{(1)}$ and $\underline {x}^{(2)}$ to the resulting code sequence
- $$\underline {x} = (x_1^{(1)}, x_1^{(2)}, x_2^{(1)}, x_2^{(2)}, \ \text{...}).$$
- In subtasks (3) to (5) you are to determine the start of the sequences $\underline {x}^{(1)}, \underline{x}^{(2)}$ and $\underline{x}$ assuming the information sequence
- $$\underline{u} = (1, 0, 1, 1, 0, 0, \ \text{. ..}).$$
Questions
Solution
(1) For both encoders, $k = 1$ and $n = 2$.
- The memory $m$ and the influence length $\nu$ are different ⇒ Answers 3 and 4.
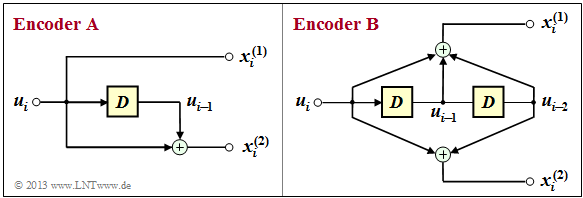
(2) The shift register of encoder $\rm A$ does contain two memory cells. However, since $x_i^{(1)} = u_i$ and $x_i^{(2)} = u_i + u_{i-1}$ is influenced only by the immediately preceding bit $u_{i-1}$ besides the current information bit $u_i$,
- the memory is $m = 1$, and
- the influence length is $\nu = m + 1 = 2$.
The graphic shows the two encoders in another representation, whereby the "memory cells" are highlighted in yellow.
- For the encoder $\rm A$ one recognizes only one memory ⇒ $m = 1$.
- In contrast, for the encoder $\rm B$ actually $m = 2$ and $\nu = 3$.
- Thus, the proposed solution 2 is correct.
(3) For the upper output of encoder $\rm B$ applies in general:
- $$x_i^{(1)} = u_{i} + u_{i-1}+ u_{i-2} \hspace{0.05cm}.$$
- Considering the preassignment ($(u_0 = u_{-1} = 0)$, we obtain with the above data:
- $$x_1^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{1} + u_{0}+ u_{-1} = 1+0+0 = 1 \hspace{0.05cm},\hspace{1cm}x_2^{(1)} = u_{2} + u_{1}+ u_{0} = 0+1+0 = 1\hspace{0.05cm},$$
- $$x_3^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{3} + u_{2}+ u_{1} \hspace{0.25cm}= 1+0+1 = 0 \hspace{0.05cm},\hspace{1cm}x_4^{(1)} = u_{4} + u_{3}+ u_{2} = 1+1+0 = 0\hspace{0.05cm},$$
- $$x_5^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} u_{5} + u_{4}+ u_{3} \hspace{0.25cm}= 0+1+1 = 0 \hspace{0.05cm},\hspace{1cm}x_6^{(1)} = u_{6} + u_{5}+ u_{4} = 0+0+1 = 1\hspace{0.05cm},$$
- $$x_7^{(1)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} x_8^{(1)} = \text{...} \hspace{0.05cm}= 0 \hspace{0.05cm}.$$
- The proposed solution 1 is correct. The second solution suggestion ⇒ $\underline {x}^{(1)} = \underline {u}$ would only be valid for a systematic code $($which is not present here$)$.
(4) Analogous to subtask (3) ⇒ $x_i^{(2)} = u_i + u_{i–2}$:
- $$x_1^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 1+0 = 1 \hspace{0.05cm},\hspace{0.2cm}x_2^{(2)} = 0+0 = 0\hspace{0.05cm}, \hspace{0.2cm}x_3^{(3)} = 1+1 = 0\hspace{0.05cm},\hspace{0.2cm}x_4^{(2)} = 1+0 = 1 \hspace{0.05cm},$$
- $$x_5^{(2)} \hspace{-0.15cm} \ = \ \hspace{-0.15cm} 0+1 = 1\hspace{0.05cm}, \hspace{0.2cm}x_6^{(2)} = 0+1 = 1\hspace{0.05cm},\hspace{0.2cm} x_7^{(2)} = x_8^{(2)} = \text{...} \hspace{0.05cm}= 0 \hspace{0.05cm}.$$
- The correct solution is the proposed solution 2.
(5) For the $($entire$)$ code sequence, one can formally write:
- $$\underline{\it x} = \big( \hspace{0.05cm}\underline{\it x}_1\hspace{0.05cm}, \hspace{0.05cm} \underline{\it x}_2\hspace{0.05cm}, \hspace{0.05cm}\text{...}\hspace{0.05cm} \underline{\it x}_i \hspace{0.05cm}, \text{...} \hspace{0.05cm} \big )\hspace{0.05cm}, \hspace{0.3cm} \underline{\it x}_i = \big( x_i^{(1)}\hspace{0.05cm}, x_i^{(2)} \big) \hspace{0.4cm}\Rightarrow \hspace{0.4cm} \underline{\it x} = \big( \hspace{0.05cm}x_1^{(1)}\hspace{0.01cm},\hspace{0.05cm} x_2^{(1)}\hspace{0.01cm},\hspace{0.05cm} x_1^{(2)}\hspace{0.01cm},\hspace{0.05cm} x_2^{(2)}\hspace{0.01cm}, \hspace{0.05cm} \text{...} \hspace{0.05cm} \big )\hspace{0.05cm}. $$
- A comparison with the solutions of subtasks (3) and (4) shows the correctness of the proposed solution 1.