Difference between revisions of "Aufgaben:Exercise 4.3: Algebraic and Modulo Sum"
From LNTwww
m (Textersetzung - „\*\s*Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0\.” ein.“ durch „ “) |
|||
| (19 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Theory_of_Stochastic_Signals/Two-Dimensional_Random_Variables |
}} | }} | ||
| − | [[File: | + | [[File:EN_Sto_A_4_3_neu2.png|right|frame|Algebraic & modulo–2 sum]] |
| − | |||
| − | + | [[File:P_ID254__Sto_A_4_3Tab.png|right|frame|Table for moment calculation]] | |
| + | A "clocked" random number generator returns a sequence $\langle x_\nu \rangle$ of binary random numbers. | ||
| + | *It is assumed that the binary numbers 0 and 1 occur with equal probabilities and that the individual random numbers do not depend on each other. | ||
| + | *The random numbers $ x_\nu \in \{0, 1\}$ are entered into the first memory location of a shift register and shifted down one digit with each clock pulse. | ||
| − | * | + | |
| + | Two new random sequences ⟨aν⟩ and ⟨mν⟩ are formed from the contents of the three-digit shift register. Here denotes: | ||
| + | |||
| + | * the "algebraic sum" aν: | ||
:aν=xν+xν−1+xν−2, | :aν=xν+xν−1+xν−2, | ||
| − | *mν | + | *the "modulo–2 sum" mν: |
:mν=xν⊕xν−1⊕xν−2. | :mν=xν⊕xν−1⊕xν−2. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | <br><br><br><br><br><br> |
| + | Hints: | ||
| + | *This exercise belongs to the chapter [[Theory_of_Stochastic_Signals/Two-Dimensional_Random_Variables|Two-Dimensional Random Variables]]. | ||
| + | *Use the following table for moment calculation. | ||
| + | <br clear=all> | ||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the probabilities of the random variable mν. What is the probability that the modulo-2 sum is equal to 0 ? |
|type="{}"} | |type="{}"} | ||
| − | Pr(mν=0) = | + | ${\rm Pr}(m_\nu = 0) \ = \ $ { 0.5 3% } |
| − | { | + | {Are there statistical dependencies within the sequence ⟨mν⟩? |
| − | |type=" | + | |type="()"} |
| − | + | + | + The sequence elements mν are statistically independent. |
| − | - | + | - There are statistical bindings within the sequence ⟨mν⟩. |
| − | { | + | {Determine the 2D–PDF fxm(xν,mν). Based on the result, evaluate the following statements. |
|type="[]"} | |type="[]"} | ||
| − | - | + | - The random variables xν and mν are statistically dependent. |
| − | + | + | + The random variables xν and mν are statistically independent. |
| − | - | + | - The random variables xν and mν are correlated. |
| − | + | + | + The random variables xν and mν are uncorrelated. |
| − | { | + | {Do statistical dependencies exist within the sequence ⟨aν⟩ ? |
| − | |type=" | + | |type="()"} |
| − | - | + | - The sequence elements aν are statistically independent. |
| − | + | + | + There are statistical bindings within the sequence ⟨aν⟩. |
| − | { | + | {Determine the 2D–PDF fam(aν,mν) and the correlation coefficient ρam. Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The random variables aν and mν are statistically dependent. |
| − | - | + | - The random variables aν and mν are statistically independent. |
| − | - | + | - The random variables aν and mν are correlated. |
| − | + | + | + The random variables aν and mν are uncorrelated. |
| Line 62: | Line 64: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' It can be seen from the table in the information section that for the modulo–2 sum, the two values 0 and 1 have equal probability: |
| + | :$${\rm Pr}(m_\nu = 0) = {\rm Pr}(m_\nu = 1)\hspace{0.15cm}\underline{=0.5}.$$ | ||
| + | |||
| + | |||
| + | |||
| + | '''(2)''' The table shows that for each preassignment ⇒ (xν−1,xν−2)=(0,0),(0,1),(1,0),(1,1), the values mν=0 and mν=1 resp. are equally likely. | ||
| + | *Expressed differently: Pr(mν|mν−1)=Pr(mν). | ||
| + | *This exactly matches the definition of "statistical independence" ⇒ <u>Answer 1</u>. | ||
| + | |||
| + | |||
| + | |||
| + | [[File:P_ID224__Sto_A_4_3_c.png|right|frame|2D–PDF of x and $m$]] | ||
| + | '''(3)''' Correct are <u>the second and the last suggested solutions</u>. | ||
| + | *The 2D–PDF consists of four Dirac delta functions, each with weight 1/4. | ||
| + | *One obtains this result, for example, by evaluating the table in the data section. | ||
| + | *Since fxm(xν,mν)=fx(xν)⋅fm(mν), the quantities xν and mν are statistically independent. | ||
| + | *Statistically independent random variables, however, are also linearly statistically independent, so they are certainly uncorrelated. | ||
| + | |||
| + | |||
| − | |||
| − | |||
| − | |||
| − | + | '''(4)''' Within the sequence ⟨aν⟩ of algebraic sum there are statistical bindings ⇒ <u>Answer 2</u>. | |
| − | '''( | + | *You can see this because the unconditional probability is $ {\rm Pr}( a_{\nu} = 0) =1/8$, |
| − | * | + | *while, for example, ${\rm Pr}(a_{\nu} = 0\hspace{0.05cm}|\hspace{0.05cm}a_{\nu-1} = 3) =0$ holds. |
| − | * | ||
| − | |||
| − | |||
| − | [[File:P_ID225__Sto_A_4_3_e.png|right|2D | + | [[File:P_ID225__Sto_A_4_3_e.png|right|frame|2D–PDF of $a and m$]] |
| − | '''(5)''' | + | '''(5)''' Correct are <u>the first and the last suggested solutions</u>: |
| − | * | + | *As in the subtask '''(3)''' there are again four Dirac delta functions, but this time not with equal Dirac weights 1/4. |
| − | * | + | *The two-dimensional PDF thus cannot be written as a product of the two marginal probability densities. |
| − | * | + | *But this means that statistical bindings must exist between aν and mν. |
| − | :E[a⋅m]=18⋅0⋅0+38⋅2⋅0+38⋅1⋅1+18⋅3⋅1=34. | + | *For the joint expected value, one obtains: |
| − | * | + | :$${\rm E}\big[a\cdot m \big] = \rm \frac{1}{8}\cdot 0 \cdot 0 +\frac{3}{8}\cdot 2 \cdot 0 +\frac{3}{8}\cdot 1 \cdot 1 + \frac{1}{8}\cdot 3 \cdot 1 = \frac{3}{4}.$$ |
| − | :μam=E[a⋅m]−E[a]⋅E[m]=0.75−1.5⋅0.5=0. | + | *With the linear means ${\rm E}\big[a \big] = 1.5$ and E[m]=0.5 it follows for the covariance: |
| − | * | + | :$$\mu_{am}= {\rm E}\big[ a\cdot m \big] - {\rm E}\big[ a \big]\cdot {\rm E} \big[ m \big] = \rm 0.75-1.5\cdot 0.5 = \rm 0.$$ |
| − | * | + | *Thus, the correlation coefficient ρam=0. That is: The dependencies present are nonlinear. |
| + | *The quantities aν and mν are statistically dependent, but still uncorrelated. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Theory of Stochastic Signals: Exercises|^4.1 Two-Dimensional Random Variables^]] |
Latest revision as of 14:49, 17 November 2022
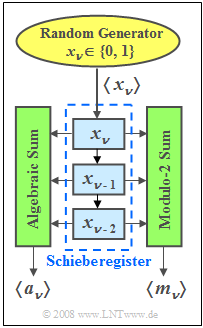
A "clocked" random number generator returns a sequence ⟨xν⟩ of binary random numbers.
- It is assumed that the binary numbers 0 and 1 occur with equal probabilities and that the individual random numbers do not depend on each other.
- The random numbers xν∈{0,1} are entered into the first memory location of a shift register and shifted down one digit with each clock pulse.
Two new random sequences ⟨aν⟩ and ⟨mν⟩ are formed from the contents of the three-digit shift register. Here denotes:
- the "algebraic sum" aν:
- aν=xν+xν−1+xν−2,
- the "modulo–2 sum" mν:
- mν=xν⊕xν−1⊕xν−2.
Hints:
- This exercise belongs to the chapter Two-Dimensional Random Variables.
- Use the following table for moment calculation.
Questions
Solution
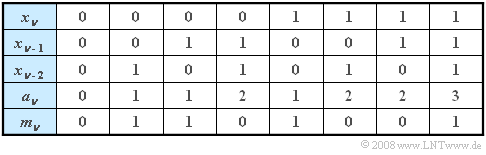
(1) It can be seen from the table in the information section that for the modulo–2 sum, the two values 0 and 1 have equal probability:
- Pr(mν=0)=Pr(mν=1)=0.5_.
(2) The table shows that for each preassignment ⇒ (xν−1,xν−2)=(0,0),(0,1),(1,0),(1,1), the values mν=0 and mν=1 resp. are equally likely.
- Expressed differently: Pr(mν|mν−1)=Pr(mν).
- This exactly matches the definition of "statistical independence" ⇒ Answer 1.
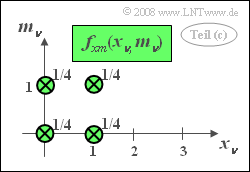
(3) Correct are the second and the last suggested solutions.
- The 2D–PDF consists of four Dirac delta functions, each with weight 1/4.
- One obtains this result, for example, by evaluating the table in the data section.
- Since fxm(xν,mν)=fx(xν)⋅fm(mν), the quantities xν and mν are statistically independent.
- Statistically independent random variables, however, are also linearly statistically independent, so they are certainly uncorrelated.
(4) Within the sequence ⟨aν⟩ of algebraic sum there are statistical bindings ⇒ Answer 2.
- You can see this because the unconditional probability is Pr(aν=0)=1/8,
- while, for example, Pr(aν=0|aν−1=3)=0 holds.
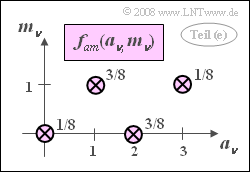
(5) Correct are the first and the last suggested solutions:
- As in the subtask (3) there are again four Dirac delta functions, but this time not with equal Dirac weights 1/4.
- The two-dimensional PDF thus cannot be written as a product of the two marginal probability densities.
- But this means that statistical bindings must exist between aν and mν.
- For the joint expected value, one obtains:
- E[a⋅m]=18⋅0⋅0+38⋅2⋅0+38⋅1⋅1+18⋅3⋅1=34.
- With the linear means E[a]=1.5 and E[m]=0.5 it follows for the covariance:
- μam=E[a⋅m]−E[a]⋅E[m]=0.75−1.5⋅0.5=0.
- Thus, the correlation coefficient ρam=0. That is: The dependencies present are nonlinear.
- The quantities aν and mν are statistically dependent, but still uncorrelated.