Difference between revisions of "Aufgaben:Exercise 3.3: Sum of two Oscillations"
m (Text replacement - "[[Modulationsverfahren" to "[[Modulation_Methods") |
|||
| (15 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Phase_Modulation_(PM) |
}} | }} | ||
| − | [[File:P_ID1084__Mod_A_3_3.png|right|frame| | + | [[File:P_ID1084__Mod_A_3_3.png|right|frame|Two different Bessel spectra]] |
| − | + | The equivalent low-pass signal with phase modulation, when normalized to the carrier amplitude $(A_{\rm T} = 1)$ is: | |
:$$ s_{\rm TP}(t) = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}K_{\rm PM}\hspace{0.05cm}\cdot \hspace{0.05cm}q(t) }\hspace{0.05cm},$$ | :$$ s_{\rm TP}(t) = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}K_{\rm PM}\hspace{0.05cm}\cdot \hspace{0.05cm}q(t) }\hspace{0.05cm},$$ | ||
| − | + | The modulator constant is assumed to be $K_{\rm PM} = \rm 1/V$ throughout the task. | |
| − | + | The upper graph shows the corresponding spectral function $B_1(f)$, when the source signal is | |
:$$q_1(t) = 0.9\,{\rm V} \cdot \sin(2 \pi \cdot 1\,{\rm kHz} \cdot t)$$ | :$$q_1(t) = 0.9\,{\rm V} \cdot \sin(2 \pi \cdot 1\,{\rm kHz} \cdot t)$$ | ||
| − | + | The weights of the Bessel-Dirac delta lines when $η_1 = 0.9$ are obtained as follows: | |
:$${\rm J}_0 (0.9) = 0.808 \approx 0.8,\hspace{1cm} | :$${\rm J}_0 (0.9) = 0.808 \approx 0.8,\hspace{1cm} | ||
{\rm J}_1 (0.9) = 0.406 \approx 0.4,$$ | {\rm J}_1 (0.9) = 0.406 \approx 0.4,$$ | ||
:$${\rm J}_2 (0.9) = 0.095 \approx 0.1,\hspace{1cm} | :$${\rm J}_2 (0.9) = 0.095 \approx 0.1,\hspace{1cm} | ||
{\rm J}_3 (0.9) \approx {\rm J}_4 (0.9) \approx\ \text{ ...} \ \approx 0 \hspace{0.05cm}.$$ | {\rm J}_3 (0.9) \approx {\rm J}_4 (0.9) \approx\ \text{ ...} \ \approx 0 \hspace{0.05cm}.$$ | ||
| − | + | Use the approximations given in the graph to simplify the calculations. | |
| − | + | The Bessel function $B_2(f)$ is obtained for the source signal | |
:$$q_2(t) = 0.65\,{\rm V} \cdot \cos(2 \pi \cdot 3\,{\rm kHz} \cdot t)$$ | :$$q_2(t) = 0.65\,{\rm V} \cdot \cos(2 \pi \cdot 3\,{\rm kHz} \cdot t)$$ | ||
| − | + | The numerical values of the Dirac delta lines are obtained from the following: | |
:$${\rm J}_0 (0.65) = 0.897 \approx 0.9,\hspace{0.3cm}{\rm J}_1 (0.65) = 0.308 \approx 0.3, \hspace{0.3cm}{\rm J}_2 (0.65) = 0.051 \approx 0\hspace{0.05cm}.$$ | :$${\rm J}_0 (0.65) = 0.897 \approx 0.9,\hspace{0.3cm}{\rm J}_1 (0.65) = 0.308 \approx 0.3, \hspace{0.3cm}{\rm J}_2 (0.65) = 0.051 \approx 0\hspace{0.05cm}.$$ | ||
| − | + | From the graph, it can be seen that due to the cosine source signal $q_2(t)$ and the cosine carrier signal $z(t)$ , the spectral lines at $±3 \ \rm kHz$ are both positive and imaginary. | |
| − | + | In the context of this task, we will now investigate the case where the source signal | |
:$$q(t) = q_1(t) + q_2(t)$$ | :$$q(t) = q_1(t) + q_2(t)$$ | ||
| − | + | is applied to the input of the phase modulator. | |
| − | * | + | *It is worth mentioning that $|q(t)| < q_{\rm max} = 1.45 \ \rm V$ . |
| − | * | + | *This maximum value is slightly smaller than the sum $A_1 + A_2$ of the individual amplitudes when a sine and a cosine function with the given amplitudes are added up. |
| − | + | In the following questionnaire, | |
| − | *$S_{\rm TP}(f)$ | + | *$S_{\rm TP}(f)$ denotes the spectral function of the equivalent low-pass signal, |
| − | *$S_+(f)$ | + | *$S_+(f)$ denotes the spectral functions of the analytic signal, |
| − | + | in both cases assuming that $q(t) = q_1(t) + q_2(t)$ holds and that the carrier frequency is $f_{\rm T} = 100 \ \rm kHz$ . | |
| Line 46: | Line 46: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Modulation_Methods/Phase_Modulation_(PM)|Phase Modulation]]. |
| − | * | + | *Particular reference is made to the page [[Modulation_Methods/Phase_Modulation_(PM)#Equivalent_low-pass_signal_in_phase_modulation|Equivalent low-pass signal in phase modulation]]. |
| − | * | + | *The values of the Bessel functions can be found in formula collections in table form. |
| − | * | + | *You can also use the interactive applet [[Applets:Bessel_functions_of_the_first_kind| Bessel functions of the first kind]] to solve this task. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Let $q(t) = q_1(t)+q_2(t)$. Which geometric figure describes the given locus curve $s_{\rm TP}(t)$? |
|type="()"} | |type="()"} | ||
| − | - | + | - The locus curve is an ellipse. |
| − | - | + | - The locus curve is a circle. |
| − | + | + | + The locus curve is approximately a semi-circle. |
| − | - | + | - The locus curve is an arc, with an approximate opening angle of $90^\circ$. |
| − | { | + | {Calculate the spectral function $S_{\rm TP}(f)$. Between what frequencies $f_{\rm min}$ and $f_{\rm max}$ do the spectral lines lie? |
|type="{}"} | |type="{}"} | ||
$f_{\rm min} \ = \ $ { -5.15--4.85 } $\ \rm kHz$ | $f_{\rm min} \ = \ $ { -5.15--4.85 } $\ \rm kHz$ | ||
$f_{\rm max} \ = \ ${ 5 3% } $\ \rm kHz$ | $f_{\rm max} \ = \ ${ 5 3% } $\ \rm kHz$ | ||
| − | { | + | {Calculate the weight of the Dirac function at $f = 0$. |
|type="{}"} | |type="{}"} | ||
${\rm Re}\big[S_{\rm TP}(f = 0)\big] \ = \ $ { 0.72 3% } | ${\rm Re}\big[S_{\rm TP}(f = 0)\big] \ = \ $ { 0.72 3% } | ||
${\rm Im}\big[S_{\rm TP}(f = 0)\big] \ = \ $ { 0. } | ${\rm Im}\big[S_{\rm TP}(f = 0)\big] \ = \ $ { 0. } | ||
| − | { | + | {Calculate the weight of the Dirac function at $f = 1\ \rm kHz$. |
|type="{}"} | |type="{}"} | ||
${\rm Re}\big[S_{\rm TP}(f = 1 \ \rm kHz)\big] \ = \ $ { 0.36 3% } | ${\rm Re}\big[S_{\rm TP}(f = 1 \ \rm kHz)\big] \ = \ $ { 0.36 3% } | ||
${\rm Im}\big[S_{\rm TP}(f = 1 \ \rm kHz)\big] \ = \ $ { 0.03 3% } | ${\rm Im}\big[S_{\rm TP}(f = 1 \ \rm kHz)\big] \ = \ $ { 0.03 3% } | ||
| − | { | + | {Calculate the weight of the $S_+(f)$–Dirac function at $f = 98 \ \rm kHz$. |
|type="{}"} | |type="{}"} | ||
${\rm Re}\big[S_{\rm +}(f = 98 \ \rm kHz)\big] \ = \ $ { 0.09 3% } | ${\rm Re}\big[S_{\rm +}(f = 98 \ \rm kHz)\big] \ = \ $ { 0.09 3% } | ||
| Line 85: | Line 85: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The <u>third answer</u> is correct: |
| − | * | + | *In angle modulation, the complex pointer $s_{\rm TP}(t)$ always moves on a circular arc with the following opening angle: |
:$$2 · K_{\rm PM} · q_{\rm max} = 2 \cdot {\rm 1/V} \cdot 1.45 \ \rm V = 2.9 \ \rm rad \approx 166^\circ.$$ | :$$2 · K_{\rm PM} · q_{\rm max} = 2 \cdot {\rm 1/V} \cdot 1.45 \ \rm V = 2.9 \ \rm rad \approx 166^\circ.$$ | ||
| − | * | + | *Using the (admittedly very rough) approximation $166^\circ \approx 180^\circ$ we indeed get a semicircle. |
| − | '''(2)''' | + | '''(2)''' In general, $S_{\rm TP}(f) = B_1(f) ∗ B_2(f)$ holds. |
| − | * | + | *Since $B_1(f)$ is limited to the frequencies $|f| ≤ 2 \ \rm kHz$ and $B_2(f)$ is limited to the range $±3 \ \rm kHz$ , the convolution product is limited to $|f| ≤ 5 \ \rm kHz$ . |
| − | * | + | *It follows that: |
:$$f_{\rm min} \hspace{0.15cm}\underline {= -5 \ \rm kHz},$$ | :$$f_{\rm min} \hspace{0.15cm}\underline {= -5 \ \rm kHz},$$ | ||
:$$f_{\rm max} \hspace{0.15cm}\underline {=+5 \ \rm kHz}.$$ | :$$f_{\rm max} \hspace{0.15cm}\underline {=+5 \ \rm kHz}.$$ | ||
| Line 102: | Line 102: | ||
| − | '''(3)''' | + | '''(3)''' The convolution product for frequency $f = 0$ results from multiplying $B_1(f)$ with $B_2(f)$ and summing. |
| − | * | + | *Only for $f = 0$ are both $B_1(f)$ and $B_2(f)$ non-zero. |
| − | * | + | *Thus, we get: |
:$$ S_{\rm TP}(f = 0) = B_{1}(f = 0) \cdot B_{2}(f = 0)= 0.8 \cdot 0.9 \hspace{0.15cm}\underline {= 0.72}\hspace{0.2cm}{\rm (rein \hspace{0.15cm} reell)} \hspace{0.05cm}.$$ | :$$ S_{\rm TP}(f = 0) = B_{1}(f = 0) \cdot B_{2}(f = 0)= 0.8 \cdot 0.9 \hspace{0.15cm}\underline {= 0.72}\hspace{0.2cm}{\rm (rein \hspace{0.15cm} reell)} \hspace{0.05cm}.$$ | ||
| − | '''(4)''' | + | '''(4)''' Now, before multiplication and summation there needs to be a frequency shift of $B_2(f)$ to the right – or of $B_1(f)$ to the left– by $1 \ \rm kHz$ . This gives: |
:$$S_{\rm TP}(f = 1\,{\rm kHz}) = B_{1}(f = -2\,{\rm kHz}) \cdot B_{2}(f = 3\,{\rm kHz}) | :$$S_{\rm TP}(f = 1\,{\rm kHz}) = B_{1}(f = -2\,{\rm kHz}) \cdot B_{2}(f = 3\,{\rm kHz}) | ||
+ B_{1}(f = 1\,{\rm kHz}) \cdot B_{2}(f = 0) | + B_{1}(f = 1\,{\rm kHz}) \cdot B_{2}(f = 0) | ||
| Line 117: | Line 117: | ||
| − | '''(5)''' | + | '''(5)''' The Dirac delta line $S_+(f = 98 \ \rm kHz)$ corresponds to the $S_{\rm TP}(f)$–line at $f = -2 \ \rm kHz$. This is |
:$$S_{\rm TP}(f \hspace{-0.05cm}=\hspace{-0.05cm} -2\,{\rm kHz}) \hspace{-0.03cm}=\hspace{-0.03cm} B_{1}(f = -2\,{\rm kHz}) \cdot B_{2}(f \hspace{-0.05cm}=\hspace{-0.05cm} 0) + | :$$S_{\rm TP}(f \hspace{-0.05cm}=\hspace{-0.05cm} -2\,{\rm kHz}) \hspace{-0.03cm}=\hspace{-0.03cm} B_{1}(f = -2\,{\rm kHz}) \cdot B_{2}(f \hspace{-0.05cm}=\hspace{-0.05cm} 0) + | ||
B_{1}(f \hspace{-0.05cm}=\hspace{-0.05cm} 1\,{\rm kHz}) \cdot B_{2}(f \hspace{-0.05cm}=\hspace{-0.05cm} -3\,{\rm kHz})= 0.1 \cdot 0.9 + 0.4 \cdot {\rm j} \cdot 0.3 \hspace{0.15cm}\hspace{-0.03cm}=\hspace{-0.03cm} 0.09 + {\rm j} \cdot 0.12$$ | B_{1}(f \hspace{-0.05cm}=\hspace{-0.05cm} 1\,{\rm kHz}) \cdot B_{2}(f \hspace{-0.05cm}=\hspace{-0.05cm} -3\,{\rm kHz})= 0.1 \cdot 0.9 + 0.4 \cdot {\rm j} \cdot 0.3 \hspace{0.15cm}\hspace{-0.03cm}=\hspace{-0.03cm} 0.09 + {\rm j} \cdot 0.12$$ | ||
| Line 126: | Line 126: | ||
| − | [[Category: | + | [[Category:Modulation Methods: Exercises|^3.1 Phase Modulation^]] |
Latest revision as of 15:21, 18 January 2023
The equivalent low-pass signal with phase modulation, when normalized to the carrier amplitude $(A_{\rm T} = 1)$ is:
- $$ s_{\rm TP}(t) = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}K_{\rm PM}\hspace{0.05cm}\cdot \hspace{0.05cm}q(t) }\hspace{0.05cm},$$
The modulator constant is assumed to be $K_{\rm PM} = \rm 1/V$ throughout the task.
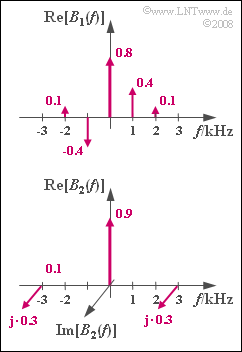
The upper graph shows the corresponding spectral function $B_1(f)$, when the source signal is
- $$q_1(t) = 0.9\,{\rm V} \cdot \sin(2 \pi \cdot 1\,{\rm kHz} \cdot t)$$
The weights of the Bessel-Dirac delta lines when $η_1 = 0.9$ are obtained as follows:
- $${\rm J}_0 (0.9) = 0.808 \approx 0.8,\hspace{1cm} {\rm J}_1 (0.9) = 0.406 \approx 0.4,$$
- $${\rm J}_2 (0.9) = 0.095 \approx 0.1,\hspace{1cm} {\rm J}_3 (0.9) \approx {\rm J}_4 (0.9) \approx\ \text{ ...} \ \approx 0 \hspace{0.05cm}.$$
Use the approximations given in the graph to simplify the calculations.
The Bessel function $B_2(f)$ is obtained for the source signal
- $$q_2(t) = 0.65\,{\rm V} \cdot \cos(2 \pi \cdot 3\,{\rm kHz} \cdot t)$$
The numerical values of the Dirac delta lines are obtained from the following:
- $${\rm J}_0 (0.65) = 0.897 \approx 0.9,\hspace{0.3cm}{\rm J}_1 (0.65) = 0.308 \approx 0.3, \hspace{0.3cm}{\rm J}_2 (0.65) = 0.051 \approx 0\hspace{0.05cm}.$$
From the graph, it can be seen that due to the cosine source signal $q_2(t)$ and the cosine carrier signal $z(t)$ , the spectral lines at $±3 \ \rm kHz$ are both positive and imaginary.
In the context of this task, we will now investigate the case where the source signal
- $$q(t) = q_1(t) + q_2(t)$$
is applied to the input of the phase modulator.
- It is worth mentioning that $|q(t)| < q_{\rm max} = 1.45 \ \rm V$ .
- This maximum value is slightly smaller than the sum $A_1 + A_2$ of the individual amplitudes when a sine and a cosine function with the given amplitudes are added up.
In the following questionnaire,
- $S_{\rm TP}(f)$ denotes the spectral function of the equivalent low-pass signal,
- $S_+(f)$ denotes the spectral functions of the analytic signal,
in both cases assuming that $q(t) = q_1(t) + q_2(t)$ holds and that the carrier frequency is $f_{\rm T} = 100 \ \rm kHz$ .
Hints:
- This exercise belongs to the chapter Phase Modulation.
- Particular reference is made to the page Equivalent low-pass signal in phase modulation.
- The values of the Bessel functions can be found in formula collections in table form.
- You can also use the interactive applet Bessel functions of the first kind to solve this task.
Questions
Solution
- In angle modulation, the complex pointer $s_{\rm TP}(t)$ always moves on a circular arc with the following opening angle:
- $$2 · K_{\rm PM} · q_{\rm max} = 2 \cdot {\rm 1/V} \cdot 1.45 \ \rm V = 2.9 \ \rm rad \approx 166^\circ.$$
- Using the (admittedly very rough) approximation $166^\circ \approx 180^\circ$ we indeed get a semicircle.
(2) In general, $S_{\rm TP}(f) = B_1(f) ∗ B_2(f)$ holds.
- Since $B_1(f)$ is limited to the frequencies $|f| ≤ 2 \ \rm kHz$ and $B_2(f)$ is limited to the range $±3 \ \rm kHz$ , the convolution product is limited to $|f| ≤ 5 \ \rm kHz$ .
- It follows that:
- $$f_{\rm min} \hspace{0.15cm}\underline {= -5 \ \rm kHz},$$
- $$f_{\rm max} \hspace{0.15cm}\underline {=+5 \ \rm kHz}.$$
(3) The convolution product for frequency $f = 0$ results from multiplying $B_1(f)$ with $B_2(f)$ and summing.
- Only for $f = 0$ are both $B_1(f)$ and $B_2(f)$ non-zero.
- Thus, we get:
- $$ S_{\rm TP}(f = 0) = B_{1}(f = 0) \cdot B_{2}(f = 0)= 0.8 \cdot 0.9 \hspace{0.15cm}\underline {= 0.72}\hspace{0.2cm}{\rm (rein \hspace{0.15cm} reell)} \hspace{0.05cm}.$$
(4) Now, before multiplication and summation there needs to be a frequency shift of $B_2(f)$ to the right – or of $B_1(f)$ to the left– by $1 \ \rm kHz$ . This gives:
- $$S_{\rm TP}(f = 1\,{\rm kHz}) = B_{1}(f = -2\,{\rm kHz}) \cdot B_{2}(f = 3\,{\rm kHz}) + B_{1}(f = 1\,{\rm kHz}) \cdot B_{2}(f = 0) = 0.1 \cdot {\rm j} \cdot 0.3 + 0.4 \cdot 0.9\hspace{0.15cm} = 0.36 + {\rm j} \cdot 0.03$$
- $$\Rightarrow \hspace{0.3cm} {\rm Re}[S_{\rm TP}(f = 1\,{\rm kHz})] \hspace{0.15cm}\underline {= 0.36} \hspace{0.05cm},\hspace{0.3cm} {\rm Im}[S_{\rm TP}(f = 1\,{\rm kHz})] \hspace{0.15cm}\underline {= 0.03} \hspace{0.05cm}.$$
(5) The Dirac delta line $S_+(f = 98 \ \rm kHz)$ corresponds to the $S_{\rm TP}(f)$–line at $f = -2 \ \rm kHz$. This is
- $$S_{\rm TP}(f \hspace{-0.05cm}=\hspace{-0.05cm} -2\,{\rm kHz}) \hspace{-0.03cm}=\hspace{-0.03cm} B_{1}(f = -2\,{\rm kHz}) \cdot B_{2}(f \hspace{-0.05cm}=\hspace{-0.05cm} 0) + B_{1}(f \hspace{-0.05cm}=\hspace{-0.05cm} 1\,{\rm kHz}) \cdot B_{2}(f \hspace{-0.05cm}=\hspace{-0.05cm} -3\,{\rm kHz})= 0.1 \cdot 0.9 + 0.4 \cdot {\rm j} \cdot 0.3 \hspace{0.15cm}\hspace{-0.03cm}=\hspace{-0.03cm} 0.09 + {\rm j} \cdot 0.12$$
- $$\Rightarrow \hspace{0.3cm} {\rm Re}[S_{\rm +}(f = 98\,{\rm kHz})] \hspace{0.15cm}\underline {= 0.09} \hspace{0.05cm}, \hspace{0.3cm} {\rm Im}[S_{\rm +}(f = 98\,{\rm kHz})] \hspace{0.15cm}\underline {= 0.12} \hspace{0.05cm}.$$