Difference between revisions of "Aufgaben:Exercise 3.8: Modulation Index and Bandwidth"
From LNTwww
m |
|||
| (7 intermediate revisions by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Frequency_Modulation_(FM) |
}} | }} | ||
| − | [[File:P_ID1105__Mod_A_3_7.png|right|frame| | + | [[File:P_ID1105__Mod_A_3_7.png|right|frame|Bessel function values]] |
| − | + | A harmonic oscillation of the form | |
:q(t)=AN⋅cos(2π⋅fN⋅t+ϕN) | :q(t)=AN⋅cos(2π⋅fN⋅t+ϕN) | ||
| − | + | is angle-modulated and then the one-sided magnitude spectrum |S+(f)| is obtained. | |
| − | * | + | *with a message frequency of fN=2 kHz the following spectral lines can be seen with the following weights: |
:|S+(98kHz)|=|S+(102kHz)|=1.560V, |S+(96kHz)|=|S+(104kHz)|=1.293V, | :|S+(98kHz)|=|S+(102kHz)|=1.560V, |S+(96kHz)|=|S+(104kHz)|=1.293V, | ||
:|S+(94kHz)|=|S+(106kHz)|=0.594V. | :|S+(94kHz)|=|S+(106kHz)|=0.594V. | ||
| − | : | + | :Further spectral lines follow each with frequency spacing fN=2 kHz, but are not given here and can be ignored. |
| − | * | + | *If one increases the message frequency to fN=4 kHz, there occur dominant lines |
:|S+(100kHz)|=2.013V, | :|S+(100kHz)|=2.013V, | ||
:|S+(96kHz)|=|S+(104kHz)|=1.494V, | :|S+(96kHz)|=|S+(104kHz)|=1.494V, | ||
:|S+(92kHz)|=|S+(108kHz)|=0.477V, | :|S+(92kHz)|=|S+(108kHz)|=0.477V, | ||
| − | : | + | :as well as further, negligible Dirac delta lines with spacing fN=4 kHz. |
| Line 37: | Line 37: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which modulation method is used here? |
|type="()"} | |type="()"} | ||
| − | - | + | - Phase modulation. |
| − | + | + | + Frequency modulation. |
| − | { | + | {What is the modulation index η_2 at message frequency f_{\rm N} = 2 \ \rm kHz? |
|type="{}"} | |type="{}"} | ||
η_2 \ = \ { 2.4 3% } | η_2 \ = \ { 2.4 3% } | ||
| − | { | + | {What is the carrier amplitude? |
|type="{}"} | |type="{}"} | ||
A_{\rm T} \ = \ { 3 3% } \ \rm V | A_{\rm T} \ = \ { 3 3% } \ \rm V | ||
| − | { | + | {Specify the bandwidth B_2 for f_{\rm N} = 2 \ \rm kHz if a distortion factor K < 1\% is desired. |
|type="{}"} | |type="{}"} | ||
B_2 \ = \ { 17.6 3% } \ \rm kHz | B_2 \ = \ { 17.6 3% } \ \rm kHz | ||
| − | { | + | {What is the modulation index η_4 at message frequency f_{\rm N} = 4 \ \rm kHz? |
|type="{}"} | |type="{}"} | ||
η_4\ = \ { 1.2 3% } | η_4\ = \ { 1.2 3% } | ||
| − | { | + | {What channel bandwidth B_4 is now required to ensure K < 1\% ? |
|type="{}"} | |type="{}"} | ||
B_4 \ = \ { 25.6 3% } \ \rm kHz | B_4 \ = \ { 25.6 3% } \ \rm kHz | ||
| Line 66: | Line 66: | ||
===Solution=== | ===Solution=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' We are dealing with a frequency modulation⇒ <u>Answer 2</u>. |
| − | * | + | *In phase modulation, the weights of the Dirac delta lines would not change when the frequency is doubled. |
| − | + | '''(2)''' The spectral function given suggests the carrier frequency f_{\rm T} = 100 \ \rm kHz due to the symmetry properties. | |
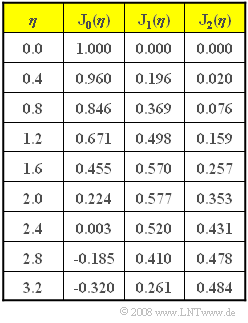
| − | '''(2)''' | + | *Since at f_{\rm N} = 2 \ \rm kHz the spectral line disappears at f_{\rm T} = 100 \ \rm kHz , we can assume η_2 \hspace{0.15cm}\underline { ≈ 2.4} . |
| − | * | + | *A check of the other pulse weights confirms this result: |
| − | * | ||
:\frac { |S_{\rm +}(f =102\,{\rm kHz})|}{ |S_{\rm +}(f =104\,{\rm kHz})|} = 1.206,\hspace{0.2cm} \frac { {\rm J}_1(2.4)}{ {\rm J}_2(2.4)}= 1.206 \hspace{0.05cm}. | :\frac { |S_{\rm +}(f =102\,{\rm kHz})|}{ |S_{\rm +}(f =104\,{\rm kHz})|} = 1.206,\hspace{0.2cm} \frac { {\rm J}_1(2.4)}{ {\rm J}_2(2.4)}= 1.206 \hspace{0.05cm}. | ||
| − | '''(3)''' | + | '''(3)''' The weights of the Dirac delta lines at f_{\rm T} + n · f_{\rm N} are generally: |
:D_n = A_{\rm T} \cdot { {\rm J}_n(\eta)} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} D_1 = A_{\rm T} \cdot { {\rm J}_1(\eta)}\hspace{0.3cm}\Rightarrow \hspace{0.3cm} A_{\rm T} = D_1/{\rm J}_1(η) = 1.560\ \rm V/0.520\hspace{0.15cm}\underline { = 3 \ V}. | :D_n = A_{\rm T} \cdot { {\rm J}_n(\eta)} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} D_1 = A_{\rm T} \cdot { {\rm J}_1(\eta)}\hspace{0.3cm}\Rightarrow \hspace{0.3cm} A_{\rm T} = D_1/{\rm J}_1(η) = 1.560\ \rm V/0.520\hspace{0.15cm}\underline { = 3 \ V}. | ||
| − | '''(4)''' | + | '''(4)''' Given the requirement K < 1\% , one can use the following rule of thumb (''Carson's rule''): |
:B_{\rm 2} = 2 \cdot f_{\rm N} \cdot (\eta +2) \hspace{0.15cm}\underline {= 17.6\,{\rm kHz}}\hspace{0.05cm}. | :B_{\rm 2} = 2 \cdot f_{\rm N} \cdot (\eta +2) \hspace{0.15cm}\underline {= 17.6\,{\rm kHz}}\hspace{0.05cm}. | ||
| − | * | + | *Thus, the Fourier coefficients D_{–4}, ... , D_4 are available. |
| − | '''(5)''' | + | '''(5)''' For frequency modulation, the general rule is: |
:\eta = \frac{K_{\rm FM} \cdot A_{\rm N}}{ \omega_{\rm N}} \hspace{0.05cm}. | :\eta = \frac{K_{\rm FM} \cdot A_{\rm N}}{ \omega_{\rm N}} \hspace{0.05cm}. | ||
| − | * | + | *Thus, by doubling the message frequency f_{\rm N}, the modulation index is halved: η_4 = η_2/2\hspace{0.15cm}\underline { = 1.2}. |
| − | '''(6)''' | + | '''(6)''' Using the same calculation as in question '''(4)''' , the channel bandwidth necessary for K < 1\% is obtained using |
:B_4 = 3.2 · 8\ \rm kHz \hspace{0.15cm}\underline {= 25.6 \ \rm kHz}. | :B_4 = 3.2 · 8\ \rm kHz \hspace{0.15cm}\underline {= 25.6 \ \rm kHz}. | ||
| − | * | + | *Because the modulation index is only half as large, transmitting the Fourier coefficients D_{–3}, ... , D_3 is sufficient for limiting the distortion factor to 1\%. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
Latest revision as of 16:22, 18 January 2023
A harmonic oscillation of the form
- q(t) = A_{\rm N} \cdot \cos(2 \pi \cdot f_{\rm N} \cdot t + \phi_{\rm N})
is angle-modulated and then the one-sided magnitude spectrum |S_+(f)| is obtained.
- with a message frequency of f_{\rm N} = 2 \ \rm kHz the following spectral lines can be seen with the following weights:
- |S_{\rm +}(98\,{\rm kHz})| = |S_{\rm +}(102\,{\rm kHz})| = 1.560\,{\rm V}\hspace{0.05cm}, |S_{\rm +}(96\,{\rm kHz})| = |S_{\rm +}(104\,{\rm kHz})| = 1.293\,{\rm V}\hspace{0.05cm},
- |S_{\rm +}(94\,{\rm kHz})| = |S_{\rm +}(106\,{\rm kHz})| = 0.594\,{\rm V}\hspace{0.05cm}.
- Further spectral lines follow each with frequency spacing f_{\rm N} = 2 \ \rm kHz, but are not given here and can be ignored.
- If one increases the message frequency to f_{\rm N} = 4 \ \rm kHz, there occur dominant lines
- |S_{\rm +}(100\,{\rm kHz})| = 2.013\,{\rm V}\hspace{0.05cm},
- |S_{\rm +}(96\,{\rm kHz})|\hspace{0.2cm} = |S_{\rm +}(104\,{\rm kHz})| = 1.494\,{\rm V}\hspace{0.05cm},
- |S_{\rm +}(92\,{\rm kHz})|\hspace{0.2cm} = |S_{\rm +}(108\,{\rm kHz})| = 0.477\,{\rm V},
- as well as further, negligible Dirac delta lines with spacing f_{\rm N} = 4 \ \rm kHz.
Hints:
- This exercise belongs to the chapter Frequency Modulation.
- Reference is also made to the chapter Phase Modulation and particularly to the section Signal characteristics with frequency modulation.
Questions
Solution
(1) We are dealing with a frequency modulation⇒ Answer 2.
- In phase modulation, the weights of the Dirac delta lines would not change when the frequency is doubled.
(2) The spectral function given suggests the carrier frequency f_{\rm T} = 100 \ \rm kHz due to the symmetry properties.
- Since at f_{\rm N} = 2 \ \rm kHz the spectral line disappears at f_{\rm T} = 100 \ \rm kHz , we can assume η_2 \hspace{0.15cm}\underline { ≈ 2.4} .
- A check of the other pulse weights confirms this result:
- \frac { |S_{\rm +}(f =102\,{\rm kHz})|}{ |S_{\rm +}(f =104\,{\rm kHz})|} = 1.206,\hspace{0.2cm} \frac { {\rm J}_1(2.4)}{ {\rm J}_2(2.4)}= 1.206 \hspace{0.05cm}.
(3) The weights of the Dirac delta lines at f_{\rm T} + n · f_{\rm N} are generally:
- D_n = A_{\rm T} \cdot { {\rm J}_n(\eta)} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} D_1 = A_{\rm T} \cdot { {\rm J}_1(\eta)}\hspace{0.3cm}\Rightarrow \hspace{0.3cm} A_{\rm T} = D_1/{\rm J}_1(η) = 1.560\ \rm V/0.520\hspace{0.15cm}\underline { = 3 \ V}.
(4) Given the requirement K < 1\% , one can use the following rule of thumb (Carson's rule):
- B_{\rm 2} = 2 \cdot f_{\rm N} \cdot (\eta +2) \hspace{0.15cm}\underline {= 17.6\,{\rm kHz}}\hspace{0.05cm}.
- Thus, the Fourier coefficients D_{–4}, ... , D_4 are available.
(5) For frequency modulation, the general rule is:
- \eta = \frac{K_{\rm FM} \cdot A_{\rm N}}{ \omega_{\rm N}} \hspace{0.05cm}.
- Thus, by doubling the message frequency f_{\rm N}, the modulation index is halved: η_4 = η_2/2\hspace{0.15cm}\underline { = 1.2}.
(6) Using the same calculation as in question (4) , the channel bandwidth necessary for K < 1\% is obtained using
- B_4 = 3.2 · 8\ \rm kHz \hspace{0.15cm}\underline {= 25.6 \ \rm kHz}.
- Because the modulation index is only half as large, transmitting the Fourier coefficients D_{–3}, ... , D_3 is sufficient for limiting the distortion factor to 1\%.