Difference between revisions of "Aufgaben:Exercise 1.08Z: Equivalent Codes"
From LNTwww
m (Guenter moved page Aufgabe 1.08Z: Äquivalente Codes to Exercise 1.08Z: Equivalent Codes) |
|||
| (6 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/General_Description_of_Linear_Block_Codes |
}} | }} | ||
| − | [[File:P_ID2394__KC_Z_1_8.png|right|frame| | + | [[File:P_ID2394__KC_Z_1_8.png|right|frame|Four $(6, 3)$ block codes]] |
| − | In | + | In the graph, the mappings $\underline{u} \rightarrow \underline{x}$ for different codes are given, each characterized below by the generator matrix $\boldsymbol{\rm G}$ and the parity-check matrix $\boldsymbol{\rm H}$, respectively: |
*${\boldsymbol{\rm Code \ A}}$: | *${\boldsymbol{\rm Code \ A}}$: | ||
| Line 19: | Line 19: | ||
:$${ \boldsymbol{\rm G}}_{\rm D} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0 \end{pmatrix} \hspace{0.05cm},\hspace{0.5cm}{ \boldsymbol{\rm H}}_{\rm D} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0\\ 1 &0 &0 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | :$${ \boldsymbol{\rm G}}_{\rm D} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0 \end{pmatrix} \hspace{0.05cm},\hspace{0.5cm}{ \boldsymbol{\rm H}}_{\rm D} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0\\ 1 &0 &0 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | This task is to investigate which of these codes or code pairs are | |
| − | * | + | *are systematic, |
| − | * | + | *are identical (that is: Different codes have same code words), |
| − | * | + | *are equivalent (that is: Different codes have same code parameters). |
| + | Hints : | ||
| + | *This exercise belongs to the chapter [[Channel_Coding/General_Description_of_Linear_Block_Codes|"General Description of Linear Block Codes"]]. | ||
| − | + | *Reference is made in particular to the sections [[Channel_Coding/General_Description_of_Linear_Block_Codes#Systematic_Codes|"Systematic Codes"]] and [[Channel_Coding/General_Description_of_Linear_Block_Codes#Identical_Codes|"Identical Codes"]]. | |
| − | * | + | *Note that the specification of a parity-check matrix $\boldsymbol{\rm H}$ is not unique. If one changes the order of the parity-check equations, this corresponds to a swapping of rows. |
| − | |||
| − | |||
| − | |||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the codes listed below are systematic? |
|type="[]"} | |type="[]"} | ||
+ Code $\rm A$, | + Code $\rm A$, | ||
| Line 49: | Line 48: | ||
+ Code $\rm D$. | + Code $\rm D$. | ||
| − | { | + | {Which of the given code pairs are identical? |
|type="[]"} | |type="[]"} | ||
| − | + Code $\rm A$ | + | + Code $\rm A$ and code $\rm B$, |
| − | - Code $\rm B$ | + | - Code $\rm B$ and code $\rm C$, |
| − | - Code $\rm C$ | + | - Code $\rm C$ and code $\rm D$. |
| − | { | + | {Which of the given code pairs are equivalent but not identical? |
|type="[]"} | |type="[]"} | ||
| − | - Code $\rm A$ | + | - Code $\rm A$ and code $\rm B$, |
| − | + Code $\rm B$ | + | + Code $\rm B$ and code $\rm C$, |
| − | - Code $\rm C$ | + | - Code $\rm C$ and code $\rm D$. |
| − | { | + | {How do the generator matrices $G_{\rm B}$ and $G_{\rm C}$ differ? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - By different linear combinations of different rows. |
| − | - | + | - By cyclic shifting of rows by $1$ down. |
| − | + | + | + By cyclic shifting of columns by $1$ to the right? |
| − | { | + | {For which codes applies ${ \boldsymbol{\rm H}} \cdot { \boldsymbol{\rm G}}^{\rm T} = \boldsymbol{0}$? |
|type="[]"} | |type="[]"} | ||
+ Code $\rm A$, | + Code $\rm A$, | ||
| Line 83: | Line 82: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct are the <u>answers 1, 3 and 4</u>: |
| − | * | + | *For a systematic $(6, 3)$ block code, the following must hold: |
:$$\underline{x} = ( x_1, x_2, x_3, x_4, x_5, x_6) = ( u_1, u_2, u_3, p_1, p_2, p_{3}) \hspace{0.05cm}.$$ | :$$\underline{x} = ( x_1, x_2, x_3, x_4, x_5, x_6) = ( u_1, u_2, u_3, p_1, p_2, p_{3}) \hspace{0.05cm}.$$ | ||
| − | + | *This condition is satisfied by code $\rm A$, code $\rm C$, and code $\rm D$, but not by code $\rm B$. | |
| − | '''(2)''' | + | '''(2)''' Correct is only <u>answer 1</u>: |
| − | * | + | *Only code $\rm A$ and code $\rm B$ are identical codes. They contain exactly the same code words and differ only by other assignments $\underline{u} \rightarrow \underline{x}$. |
| − | * | + | |
| − | :* | + | *As indicated in the solution to [[Aufgaben:Exercise_1.08:_Identical_Codes|"Exercise 1.8 (3)"]], one gets from the generator matrix ${ \boldsymbol{\rm G}}_{\rm B}$ to the generator matrix ${ \boldsymbol{\rm G}}_{\rm A}$ |
| − | :* | + | :*by swapping/permuting rows alone, or |
| + | :*by replacing a row with the linear combination between that row and another. | ||
| − | '''(3)''' | + | '''(3)''' Thus, the correct answer is <u>answer 2</u> alone: |
| − | *Code A | + | *Code $\rm A$ and code $\rm B$ are more than equivalent, namely identical. |
| − | *Code C | + | |
| − | + | *Code $\rm C$ and code $\rm D$ also differ, for example, by the minimum Hamming distance $d_{\rm min} = 3$ and $d_{\rm min} = 2$, respectively, and are thus also not equivalent. | |
| + | *Code $\rm B$ and code $\rm C$ show the same properties, for example $d_{\rm min} = 3$ holds for both. However, they contain different code words. | ||
| − | |||
| − | + | '''(4)''' Correct is <u>answer 3</u>: | |
| − | |||
| − | |||
| + | *The last column of ${ \boldsymbol{\rm G}}_{\rm B}$ gives the first column of ${ \boldsymbol{\rm G}}_{\rm C}$. | ||
| + | *The first column of ${ \boldsymbol{\rm G}}_{\rm B}$ gives the second column of ${ \boldsymbol{\rm G}}_{\rm C}$. | ||
| + | *The second column of ${ \boldsymbol{\rm G}}_{\rm B}$ gives the third column of ${ \boldsymbol{\rm G}}_{\rm C}$, etc. | ||
| − | '''(5)''' | + | |
| − | * | + | '''(5)''' All statements are true</u>: |
| + | *The condition ${ \boldsymbol{\rm H}} \cdot { \boldsymbol{\rm G}}^{\rm T} = \boldsymbol{0}$ holds for all linear codes. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 125: | Line 127: | ||
| − | [[Category:Channel Coding: Exercises|^1.4 | + | [[Category:Channel Coding: Exercises|^1.4 Linear Block Code Description |
^]] | ^]] | ||
Latest revision as of 17:00, 23 January 2023
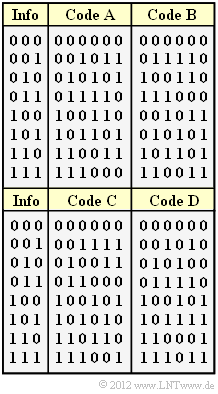
In the graph, the mappings $\underline{u} \rightarrow \underline{x}$ for different codes are given, each characterized below by the generator matrix $\boldsymbol{\rm G}$ and the parity-check matrix $\boldsymbol{\rm H}$, respectively:
- ${\boldsymbol{\rm Code \ A}}$:
- $${ \boldsymbol{\rm G}}_{\rm A} = \begin{pmatrix} 1 &0 &0 &1 &1 &0\\ 0 &1 &0 &1 &0 &1\\ 0 &0 &1 &0 &1 &1 \end{pmatrix} \hspace{0.05cm},\hspace{0.5cm}{ \boldsymbol{\rm H}}_{\rm A} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 1 &0 &1 &0 &1 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
- ${\boldsymbol{\rm Code \ B}}$:
- $${ \boldsymbol{\rm G}}_{\rm B} = \begin{pmatrix} 0 &0 &1 &0 &1 &1\\ 1 &0 &0 &1 &1 &0\\ 0 &1 &1 &1 &1 &0 \end{pmatrix} \hspace{0.05cm},\hspace{0.5cm} { \boldsymbol{\rm H}}_{\rm B} = \begin{pmatrix} 1 &0 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0\\ 0 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
- ${\boldsymbol{\rm Code \ C}}$:
- $${ \boldsymbol{\rm G}}_{\rm C} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &0 &1 &1\\ 0 &0 &1 &1 &1 &1 \end{pmatrix} \hspace{0.05cm},\hspace{0.5cm}{ \boldsymbol{\rm H}}_{\rm C} = \begin{pmatrix} 1 &0 &1 &1 &0 &0\\ 0 &1 &1 &0 &1 &0\\ 1 &1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm},$$
- ${\boldsymbol{\rm Code \ D}}$:
- $${ \boldsymbol{\rm G}}_{\rm D} = \begin{pmatrix} 1 &0 &0 &1 &0 &1\\ 0 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0 \end{pmatrix} \hspace{0.05cm},\hspace{0.5cm}{ \boldsymbol{\rm H}}_{\rm D} = \begin{pmatrix} 1 &1 &0 &1 &0 &0\\ 0 &0 &1 &0 &1 &0\\ 1 &0 &0 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
This task is to investigate which of these codes or code pairs are
- are systematic,
- are identical (that is: Different codes have same code words),
- are equivalent (that is: Different codes have same code parameters).
Hints :
- This exercise belongs to the chapter "General Description of Linear Block Codes".
- Reference is made in particular to the sections "Systematic Codes" and "Identical Codes".
- Note that the specification of a parity-check matrix $\boldsymbol{\rm H}$ is not unique. If one changes the order of the parity-check equations, this corresponds to a swapping of rows.
Questions
Solution
(1) Correct are the answers 1, 3 and 4:
- For a systematic $(6, 3)$ block code, the following must hold:
- $$\underline{x} = ( x_1, x_2, x_3, x_4, x_5, x_6) = ( u_1, u_2, u_3, p_1, p_2, p_{3}) \hspace{0.05cm}.$$
- This condition is satisfied by code $\rm A$, code $\rm C$, and code $\rm D$, but not by code $\rm B$.
(2) Correct is only answer 1:
- Only code $\rm A$ and code $\rm B$ are identical codes. They contain exactly the same code words and differ only by other assignments $\underline{u} \rightarrow \underline{x}$.
- As indicated in the solution to "Exercise 1.8 (3)", one gets from the generator matrix ${ \boldsymbol{\rm G}}_{\rm B}$ to the generator matrix ${ \boldsymbol{\rm G}}_{\rm A}$
- by swapping/permuting rows alone, or
- by replacing a row with the linear combination between that row and another.
(3) Thus, the correct answer is answer 2 alone:
- Code $\rm A$ and code $\rm B$ are more than equivalent, namely identical.
- Code $\rm C$ and code $\rm D$ also differ, for example, by the minimum Hamming distance $d_{\rm min} = 3$ and $d_{\rm min} = 2$, respectively, and are thus also not equivalent.
- Code $\rm B$ and code $\rm C$ show the same properties, for example $d_{\rm min} = 3$ holds for both. However, they contain different code words.
(4) Correct is answer 3:
- The last column of ${ \boldsymbol{\rm G}}_{\rm B}$ gives the first column of ${ \boldsymbol{\rm G}}_{\rm C}$.
- The first column of ${ \boldsymbol{\rm G}}_{\rm B}$ gives the second column of ${ \boldsymbol{\rm G}}_{\rm C}$.
- The second column of ${ \boldsymbol{\rm G}}_{\rm B}$ gives the third column of ${ \boldsymbol{\rm G}}_{\rm C}$, etc.
(5) All statements are true:
- The condition ${ \boldsymbol{\rm H}} \cdot { \boldsymbol{\rm G}}^{\rm T} = \boldsymbol{0}$ holds for all linear codes.