Difference between revisions of "Aufgaben:Exercise 1.13: Binary Erasure Channel Decoding"
m (Text replacement - "[File:" to "[File:") |
|||
| (12 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Decoding_of_Linear_Block_Codes}} |
| − | [[File: | + | [[File:EN_KC_A_1_13.png|right|frame|Decoding at the BEC]] |
| − | + | We assume here the model in section [[Channel_Coding/Decoding_of_Linear_Block_Codes#Decoding_at_the_Binary_Erasure_Channel|"Decoding at the Binary Erasure Channel"]] (BEC configuration highlighted in green): | |
| − | * | + | *Each information word $\underline{u}$ is encoded blockwise and yields the code word $\underline{x}$. Let the block code be linear and completely given by its parity-check matrix $\boldsymbol{\rm H}$. |
| − | * | + | *During transmission $n_{\rm E}$ bits of the code word are erased ⇒ [[Channel_Coding/Channel_Models_and_Decision_Structures#Binary_Erasure_Channel_.E2.80.93_BEC|"Binary Erasure Channel"]] $\rm (BEC)$. The code word $\underline{x}$ thus becomes the received word $\underline{y}$. |
| − | * | + | *If the number $n_{\rm E}$ of erasures is less than the [[Channel_Coding/Objective_of_Channel_Coding#Important_definitions_for_block_coding|"minimum distance"]] $d_{\rm min}$ of the code, it is possible to reconstruct from $\underline{y}$ the code word $\underline{z} = \underline{x}$ without error, and thus the correct information word $\underline{v} = \underline{u}$ is also obtained. |
| − | + | For exercise description, let us now consider the Hamming code word $\underline{x} = (0, 1, 0, 1, 1, 0, 0)$ and the received word $\underline{y} = (0, 1, {\rm E} , {\rm E}, 1, 0, 0).$ | |
| − | * | + | *The third and fourth bit were thus erased by the channel. The "code word finder" thus has the task to determine the vector $z_{\rm E} = (z_{3}, z_{4})$ with $z_{3}, \ z_{4} \in \{0, 1\}$ to be determined. This is done according to the equation |
| − | |||
:$${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.05cm}.$$ | :$${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.05cm}.$$ | ||
| − | * | + | *In the present example: |
:$$\underline{z}_{\rm K} = (0, 1, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &1 &0 &0\\ 0 &1 &0 &1 &0\\ 1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &1\\ 0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | :$$\underline{z}_{\rm K} = (0, 1, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &1 &0 &0\\ 0 &1 &0 &1 &0\\ 1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &1\\ 0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | * | + | *This equation gives two equations for the bits to be determined, whose solution leads to the result $z_{3} = 0$ and $z_{4} = 1$. |
| + | Hints: | ||
| + | * This exercise belongs to the chapter [[Channel_Coding/Decoding_of_Linear_Block_Codes|"Decoding of Linear Block Codes"]]. | ||
| − | + | * The algorithm for mapping the received word $\underline{y}$ to the correct code word $\underline{z} = \underline{x}$ is described in detail in the [[Channel_Coding/Decoding_of_Linear_Block_Codes#Decoding_at_the_Binary_Erasure_Channel|"theory section"]] . | |
| − | + | ||
| − | + | * We would like to remind again that in BEC decoding we use the first decoder block $\underline{y} → \underline{z}$ as "code word finder" since wrong decisions are excluded here. Each received word is decoded correctly, or it may not be decoded at all. | |
| − | + | ||
| − | * | + | *In the BSC model, on the other hand, decoding errors cannot be avoided. Accordingly, we refer to the corresponding block there as "code word estimator". |
| − | * | ||
| − | * | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | { $\underline{y} = (1, {\rm E}, 0, 1, 0, 0, {\rm E})$ was received. Which sequence does the code word finder decide to use? |
|type="()"} | |type="()"} | ||
- $\underline{z} = (1, 0, 0, 1, 0, 0, 0),$ | - $\underline{z} = (1, 0, 0, 1, 0, 0, 0),$ | ||
| Line 48: | Line 47: | ||
- $\underline{z} = (1, 0, 0, 1, 0, 0, 1).$ | - $\underline{z} = (1, 0, 0, 1, 0, 0, 1).$ | ||
| − | { | + | {What are the consequences of the red entries for $\boldsymbol{\rm H}_{\rm K}$ and $z_{\rm K}$ (see graphic in the information section)? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The erasure vector is $\underline{z}_{\rm E} = (z_{5}, z_{6}, z_{7}).$ |
| − | + | + | + The received word is $\underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E}).$ |
| − | - $\boldsymbol{\rm H}_{\rm E}$ | + | - $\boldsymbol{\rm H}_{\rm E}$ is a $2 \times 3$ matrix. |
| − | + $\boldsymbol{\rm H}_{\rm E}$ | + | + $\boldsymbol{\rm H}_{\rm E}$ is a $3 \times 3$ matrix. |
| − | { | + | {Now apply $\underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E}).$ Which code word is selected? |
|type="()"} | |type="()"} | ||
- $\underline{z} = (1, 1, 0, 1, 1, 1, 0),$ | - $\underline{z} = (1, 1, 0, 1, 1, 1, 0),$ | ||
+ $\underline{z} = (1, 1, 0, 1, 0, 0, 1),$ | + $\underline{z} = (1, 1, 0, 1, 0, 0, 1),$ | ||
- $\underline{z} = (1, 1, 0, 0, 0, 1, 0).$ | - $\underline{z} = (1, 1, 0, 0, 0, 1, 0).$ | ||
| − | - | + | - For the present $\underline{y}$ no unique decoding is possible. |
| − | { | + | {What are the consequences of the green entries for $\boldsymbol{\rm H}_{\rm K}$ and $z_{\rm K}$ (see graphic in the information section)? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The received word is $\underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E}).$ |
| − | - $\boldsymbol{\rm H}_{\rm K}$ | + | - $\boldsymbol{\rm H}_{\rm K}$ differs from subtask '''(2)''' in the last row. |
| − | + $\boldsymbol{\rm H}_{\rm K}$ | + | + $\boldsymbol{\rm H}_{\rm K}$ differs from subtask '''(2)''' in the last column. |
| − | { | + | {Now apply $\underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E}).$ Which code word is selected? |
|type="()"} | |type="()"} | ||
- $\underline{z} = (1, 1, 0, 1, 1, 1, 0),$ | - $\underline{z} = (1, 1, 0, 1, 1, 1, 0),$ | ||
- $\underline{z} = (1, 1, 0, 1, 0, 0, 1),$ | - $\underline{z} = (1, 1, 0, 1, 0, 0, 1),$ | ||
- $\underline{z} = (1, 1, 0, 0, 0, 1, 0).$ | - $\underline{z} = (1, 1, 0, 0, 0, 1, 0).$ | ||
| − | + | + | + For the present $\underline{y}$ no unique decoding is possible. |
| − | { | + | {Which statements result for the correction capability at the BEC? $n_{\rm E}$ indicates in the following the number of erasures. |
|type="[]"} | |type="[]"} | ||
| − | + | + | + For $n_{\rm E} < d_{\rm min}$ unique decoding is always possible. |
| − | - | + | - For $n_{\rm E} = d_{\rm min}$ unique decoding is always possible. |
| − | + | + | + For $n_{\rm E} = d_{\rm min}$ sometimes a unique decoding is possible. |
| − | + | + | + For $n_{\rm E} > d_{\rm min}$ unique decoding is never possible. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The received vector is $\underline{y} = (1, {\rm E}, 0, 1, 0, 0, {\rm E})$. Thus, the code symbols at positions 2 and 7 were erased. Based on the given parity-check matrix |
| − | |||
| − | |||
:$${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &0 &1 &0 &0\\ 0 &1 &1 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0 &1 \end{pmatrix}$$ | :$${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &0 &1 &0 &0\\ 0 &1 &1 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0 &1 \end{pmatrix}$$ | ||
| − | + | of the Hamming code is obtained for vector and matrix | |
| − | * | + | * with respect to all "correctly transmitted code symbols" $($index "$\rm K$" from German "korrekt"$)$ which are known to the code word finder: |
:$$\underline{z}_{\rm K} = (1, 0, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \hspace{0.05cm},$$ | :$$\underline{z}_{\rm K} = (1, 0, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \hspace{0.05cm},$$ | ||
| − | * | + | *with respect to the two "erased code symbols" $z_{2}$ and $z_{7}$ $($index $\rm E)$ to be determined: |
:$$\underline{z}_{\rm E} = (z_2, z_7)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \hspace{0.05cm}.$$ | :$$\underline{z}_{\rm E} = (z_2, z_7)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | The equation of determination is thus: | |
| − | |||
:$${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.3cm} | :$${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.3cm} | ||
\Rightarrow \hspace{0.3cm} \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \cdot \begin{pmatrix} z_2 \\ z_7 \end{pmatrix} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 0 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 1 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm}.$$ | \Rightarrow \hspace{0.3cm} \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \cdot \begin{pmatrix} z_2 \\ z_7 \end{pmatrix} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 0 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 1 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | This results in three equations for the two unknowns $z_{2}$ and $z_{7}$: | |
:$${\rm (a)}\ z_{2} = 1,$$ | :$${\rm (a)}\ z_{2} = 1,$$ | ||
| Line 112: | Line 108: | ||
:$${\rm (c)}\ z_{2} + z_{7} = 0 \ \Rightarrow \ z_{7}= 1.$$ | :$${\rm (c)}\ z_{2} + z_{7} = 0 \ \Rightarrow \ z_{7}= 1.$$ | ||
| + | Thus, the code word finder returns $\underline{z} = (1, 1, 0, 1, 0, 0, 1)$ ⇒ <u>Suggested solution 2</u>. | ||
| − | |||
| − | + | '''(2)''' Looking at the given matrix $\boldsymbol{\rm H}_{\rm K}$, we see that it coincides with the first four columns of the parity-check matrix $\boldsymbol{\rm H}$. | |
| − | + | ||
| − | '''(2)''' | + | *The erasures thus affect the last three bits of the received word ⇒ $\underline{z}_{\rm E} = (z_{5}, z_{6}, z_{7})$ ⇒ $\underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E})$. |
| − | * | + | *The erasure matrix reads: |
| − | * | ||
:$${ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | :$${ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | Correct are therefore the <u>statements 1, 2 and 4</u>. | |
| − | |||
| − | '''(3)''' | + | '''(3)''' One obtains after some matrix multiplications: |
:$${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &0\\ 0 &1 &1 &1\\ 1 &1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} | :$${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &0\\ 0 &1 &1 &1\\ 1 &1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} | ||
{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} \hspace{0.05cm}.$$ | { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | + | *By equating it follows $z_{5} = 0, \ z_{6} = 0, \ z_{7} = 1$ ⇒ <u>Suggested solution 2</u>. | |
| − | '''(4)''' | + | '''(4)''' The matrix comparison shows that the first three columns of $\boldsymbol{\rm H}$ and $\boldsymbol{\rm H}_{\rm K}$ are identical. |
| − | * | + | * The fourth column of $\boldsymbol{\rm H}_{\rm K}$ is equal to the fifth column of the parity-check matrix $\boldsymbol{\rm H}$. |
| − | * | + | |
| + | *From this follows for the vector $z_{\rm E} = (z_{4}, z_{6}, z_{7})$ and further for the received vector $\underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E})$ ⇒ <u>Suggested solutions 1 and 3</u>. | ||
| − | + | '''(5)''' Analogous to subtask '''(3)''', we obtain: | |
| − | '''(5)''' | ||
:$${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &1\\ 0 &1 &1 &0\\ 1 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 0 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} | :$${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &1\\ 0 &1 &1 &0\\ 1 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 0 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} | ||
{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 0 &0 &0\\ 1 &1 &0\\ 1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_4 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} 0 \\ z_4 + z_6 \\ z_4 + z_7 \end{pmatrix} \hspace{0.05cm}.$$ | { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 0 &0 &0\\ 1 &1 &0\\ 1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_4 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} 0 \\ z_4 + z_6 \\ z_4 + z_7 \end{pmatrix} \hspace{0.05cm}.$$ | ||
| − | * | + | *If we now set the two column vectors equal, we obtain only two equations for the three unknowns ⇒ <u>Suggested solution 4</u>. Or in other words: |
| − | + | ||
| + | *If the number of extinctions of the BEC channel is larger than the rank of the matrix $\boldsymbol{\rm H}_{\rm E}$, then no unique solution of the resulting system of equations results. | ||
| + | |||
| + | |||
| + | '''(6)''' To solve this exercise, we again refer to the systematic Hamming code $(7, 4, 3)$ according to the given parity-check equation and code table. <u>Note:</u> | ||
| + | [[File:P_ID2540__KC_A_1_13f.png|right|frame|Code table of the systematic $(7, 4, 3)$ Hamming code<br><br><br>]] | ||
| + | *The information bits are shown in black and the parity bits in red. The minimum distance of this code is $d_{\rm min} = 3$. | ||
| + | *Further we assume that always the code word $\underline{x} = (1, 1, 0, 1, 0, 0, 1)$ with yellow background was sent. Then holds: | ||
| − | + | *If the number $n_{\rm E}$ of erasures is smaller than $d_{\rm min} = 3$, decoding by the method described here is always possible ⇒ see e.g. subtask '''(1)''' with $n_{\rm E}= 2$. | |
| − | '''( | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | * | + | *For $n_{\rm E} = d_{\rm min} = 3$ decoding is sometimes possible, see subtask '''(3)'''. In the code table, there is only one code word that could match $\underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E})$ namely the code word highlighted in yellow $\underline{x} = (1, 1, 0, 1, 0, 0, 1)$ . |
| − | * | + | *In contrast $\underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E})$ according to subtask '''(4)''' could not be decoded. In the code table, besides $(1, 1, 0, 1, 0, 0, 1)$ with $(1, 1, 0, 0, 0, 1, 0)$ one can recognize another code word (highlighted in green), which becomes the received word $\underline{y}$ due to the $n_{\rm E} = 3$ erasures concerning bit 4, 6 and 7. |
| − | * | + | *This case, when the $n_{\rm E} = d_{\rm min}$ extinctions affect exactly the $d_{\rm min}$ different bits of two code words, leads to a matrix $\mathbf{H}_{\rm E}$ with rank smaller $d_{\rm min}$. |
| − | * | + | *If $n_{\rm E} > d_{\rm min}$, the number $n - n_{\rm E}$ of bits not erased is smaller than the number $k$ of information bits. In this case, of course, the code word cannot be decoded. |
| − | + | That is: Applicable are the <u>statements 1, 3 and 4</u>. | |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Channel Coding: Exercises|^1.5 Linear Block Code Decoding^]] |
Latest revision as of 17:02, 23 January 2023
We assume here the model in section "Decoding at the Binary Erasure Channel" (BEC configuration highlighted in green):
- Each information word $\underline{u}$ is encoded blockwise and yields the code word $\underline{x}$. Let the block code be linear and completely given by its parity-check matrix $\boldsymbol{\rm H}$.
- During transmission $n_{\rm E}$ bits of the code word are erased ⇒ "Binary Erasure Channel" $\rm (BEC)$. The code word $\underline{x}$ thus becomes the received word $\underline{y}$.
- If the number $n_{\rm E}$ of erasures is less than the "minimum distance" $d_{\rm min}$ of the code, it is possible to reconstruct from $\underline{y}$ the code word $\underline{z} = \underline{x}$ without error, and thus the correct information word $\underline{v} = \underline{u}$ is also obtained.
For exercise description, let us now consider the Hamming code word $\underline{x} = (0, 1, 0, 1, 1, 0, 0)$ and the received word $\underline{y} = (0, 1, {\rm E} , {\rm E}, 1, 0, 0).$
- The third and fourth bit were thus erased by the channel. The "code word finder" thus has the task to determine the vector $z_{\rm E} = (z_{3}, z_{4})$ with $z_{3}, \ z_{4} \in \{0, 1\}$ to be determined. This is done according to the equation
- $${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.05cm}.$$
- In the present example:
- $$\underline{z}_{\rm K} = (0, 1, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &1 &0 &0\\ 0 &1 &0 &1 &0\\ 1 &1 &0 &0 &1 \end{pmatrix} \hspace{0.05cm}, \hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &1\\ 0 &1 \end{pmatrix} \hspace{0.05cm}.$$
- This equation gives two equations for the bits to be determined, whose solution leads to the result $z_{3} = 0$ and $z_{4} = 1$.
Hints:
- This exercise belongs to the chapter "Decoding of Linear Block Codes".
- The algorithm for mapping the received word $\underline{y}$ to the correct code word $\underline{z} = \underline{x}$ is described in detail in the "theory section" .
- We would like to remind again that in BEC decoding we use the first decoder block $\underline{y} → \underline{z}$ as "code word finder" since wrong decisions are excluded here. Each received word is decoded correctly, or it may not be decoded at all.
- In the BSC model, on the other hand, decoding errors cannot be avoided. Accordingly, we refer to the corresponding block there as "code word estimator".
Questions
Solution
- $${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 &1 &1 &0 &1 &0 &0\\ 0 &1 &1 &1 &0 &1 &0\\ 1 &1 &0 &1 &0 &0 &1 \end{pmatrix}$$
of the Hamming code is obtained for vector and matrix
- with respect to all "correctly transmitted code symbols" $($index "$\rm K$" from German "korrekt"$)$ which are known to the code word finder:
- $$\underline{z}_{\rm K} = (1, 0, 1, 0, 0)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm K} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \hspace{0.05cm},$$
- with respect to the two "erased code symbols" $z_{2}$ and $z_{7}$ $($index $\rm E)$ to be determined:
- $$\underline{z}_{\rm E} = (z_2, z_7)\hspace{0.05cm},\hspace{0.3cm}{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \hspace{0.05cm}.$$
The equation of determination is thus:
- $${ \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T}= { \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} \begin{pmatrix} 1 &0\\ 1 &0\\ 1 &1 \end{pmatrix} \cdot \begin{pmatrix} z_2 \\ z_7 \end{pmatrix} = \begin{pmatrix} 1 &1 &0 &1 &0\\ 0 &1 &1 &0 &1\\ 1 &0 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 0 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 1 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm}.$$
This results in three equations for the two unknowns $z_{2}$ and $z_{7}$:
- $${\rm (a)}\ z_{2} = 1,$$
- $${\rm (b)}\ z_{2} = 1,$$
- $${\rm (c)}\ z_{2} + z_{7} = 0 \ \Rightarrow \ z_{7}= 1.$$
Thus, the code word finder returns $\underline{z} = (1, 1, 0, 1, 0, 0, 1)$ ⇒ Suggested solution 2.
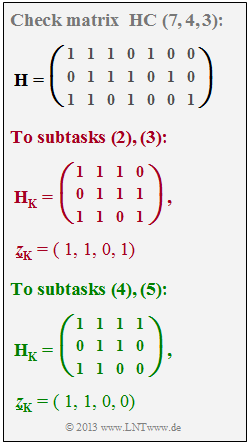
(2) Looking at the given matrix $\boldsymbol{\rm H}_{\rm K}$, we see that it coincides with the first four columns of the parity-check matrix $\boldsymbol{\rm H}$.
- The erasures thus affect the last three bits of the received word ⇒ $\underline{z}_{\rm E} = (z_{5}, z_{6}, z_{7})$ ⇒ $\underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E})$.
- The erasure matrix reads:
- $${ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \hspace{0.05cm}.$$
Correct are therefore the statements 1, 2 and 4.
(3) One obtains after some matrix multiplications:
- $${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &0\\ 0 &1 &1 &1\\ 1 &1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 1 &0 &0\\ 0 &1 &0\\ 0 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} z_5 \\ z_6 \\ z_7 \end{pmatrix} \hspace{0.05cm}.$$
- By equating it follows $z_{5} = 0, \ z_{6} = 0, \ z_{7} = 1$ ⇒ Suggested solution 2.
(4) The matrix comparison shows that the first three columns of $\boldsymbol{\rm H}$ and $\boldsymbol{\rm H}_{\rm K}$ are identical.
- The fourth column of $\boldsymbol{\rm H}_{\rm K}$ is equal to the fifth column of the parity-check matrix $\boldsymbol{\rm H}$.
- From this follows for the vector $z_{\rm E} = (z_{4}, z_{6}, z_{7})$ and further for the received vector $\underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E})$ ⇒ Suggested solutions 1 and 3.
(5) Analogous to subtask (3), we obtain:
- $${ \boldsymbol{\rm H}}_{\rm K} \cdot \underline{z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 &1 &1 &1\\ 0 &1 &1 &0\\ 1 &1 &0 &0 \end{pmatrix} \cdot \begin{pmatrix} 1 \\ 1 \\ 0 \\ 0 \end{pmatrix} = \begin{pmatrix} 0 \\ 1 \\ 0 \end{pmatrix} \hspace{0.05cm},\hspace{1cm} { \boldsymbol{\rm H}}_{\rm E} \cdot \underline{z}_{\rm E}^{\rm T} = \begin{pmatrix} 0 &0 &0\\ 1 &1 &0\\ 1 &0 &1 \end{pmatrix} \cdot \begin{pmatrix} z_4 \\ z_6 \\ z_7 \end{pmatrix} = \begin{pmatrix} 0 \\ z_4 + z_6 \\ z_4 + z_7 \end{pmatrix} \hspace{0.05cm}.$$
- If we now set the two column vectors equal, we obtain only two equations for the three unknowns ⇒ Suggested solution 4. Or in other words:
- If the number of extinctions of the BEC channel is larger than the rank of the matrix $\boldsymbol{\rm H}_{\rm E}$, then no unique solution of the resulting system of equations results.
(6) To solve this exercise, we again refer to the systematic Hamming code $(7, 4, 3)$ according to the given parity-check equation and code table. Note:
- The information bits are shown in black and the parity bits in red. The minimum distance of this code is $d_{\rm min} = 3$.
- Further we assume that always the code word $\underline{x} = (1, 1, 0, 1, 0, 0, 1)$ with yellow background was sent. Then holds:
- If the number $n_{\rm E}$ of erasures is smaller than $d_{\rm min} = 3$, decoding by the method described here is always possible ⇒ see e.g. subtask (1) with $n_{\rm E}= 2$.
- For $n_{\rm E} = d_{\rm min} = 3$ decoding is sometimes possible, see subtask (3). In the code table, there is only one code word that could match $\underline{y} = (1, 1, 0, 1, {\rm E}, {\rm E}, {\rm E})$ namely the code word highlighted in yellow $\underline{x} = (1, 1, 0, 1, 0, 0, 1)$ .
- In contrast $\underline{y} = (1, 1, 0, {\rm E}, 0, {\rm E}, {\rm E})$ according to subtask (4) could not be decoded. In the code table, besides $(1, 1, 0, 1, 0, 0, 1)$ with $(1, 1, 0, 0, 0, 1, 0)$ one can recognize another code word (highlighted in green), which becomes the received word $\underline{y}$ due to the $n_{\rm E} = 3$ erasures concerning bit 4, 6 and 7.
- This case, when the $n_{\rm E} = d_{\rm min}$ extinctions affect exactly the $d_{\rm min}$ different bits of two code words, leads to a matrix $\mathbf{H}_{\rm E}$ with rank smaller $d_{\rm min}$.
- If $n_{\rm E} > d_{\rm min}$, the number $n - n_{\rm E}$ of bits not erased is smaller than the number $k$ of information bits. In this case, of course, the code word cannot be decoded.
That is: Applicable are the statements 1, 3 and 4.