Difference between revisions of "Aufgaben:Exercise 5.5Z: About the Rake Receiver"
From LNTwww
(Die Seite wurde neu angelegt: „ {{quiz-Header|Buchseite=Modulationsverfahren/Fehlerwahrscheinlichkeit der PN–Modulation }} [[File:|right|]] ===Fragebogen=== <quiz display=simple> {Mul…“) |
|||
| (27 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation_Methods/Error_Probability_of_Direct-Sequence_Spread_Spectrum_Modulation |
}} | }} | ||
| − | [[File:|right|]] | + | [[File:EN_Mod_Z_5_5.png|right|frame|Two-way channel <br>& rake receiver]] |
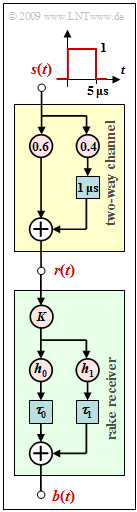
| + | The diagram shows a two-way channel (yellow background). The corresponding descriptive equation is: | ||
| + | :$$ r(t) =0.6 \cdot s(t) + 0.4 \cdot s (t - \tau) \hspace{0.05cm}.$$ | ||
| + | Let the delay on the secondary path be $τ = 1 \ \rm µ s$. | ||
| + | Drawn below is the structure of a rake receiver (green background) with general coefficients $K$, $h_0$, $h_1$, $τ_0$ and $τ_1$. | ||
| − | === | + | *The purpose of the rake receiver is to combine the energy of the two signal paths, making the decision more reliable. |
| + | |||
| + | *The combined impulse response of the channel (German: "Kanal" ⇒ subscript "K") and the rake receiver can be expressed in the form | ||
| + | :$$h_{\rm KR}(t) = A_0 \cdot \delta (t ) + A_1 \cdot \delta (t - \tau) + A_2 \cdot \delta (t - 2\tau)$$ | ||
| + | :but only if the rake coefficients $h_0$, $h_1$, $τ_0$ and $τ_1$ are appropriately chosen. | ||
| + | *The main part of $h_{\rm KR}(t)$ is supposed to be at $t = τ$. | ||
| + | *The constant $K$ is to be chosen so that the amplitude of the main path $A_1 = 1$ : | ||
| + | :$$K= \frac{1}{h_0^2 + h_1^2}.$$ | ||
| + | Apart from the rake parameters, the signals $r(t)$ and $b(t)$ are sought when $s(t)$ is a rectangle of height $s_0 = 1$ and width $T = \ \rm 5 µ s$. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | Notes: | ||
| + | *The exercise belongs to the chapter [[Modulation_Methods/Error_Probability_of_Direct-Sequence_Spread_Spectrum_Modulation|Error Probability of Direct-Sequence Spread Spectrum Modulation]]. | ||
| + | *Reference is made in particular to the section [[Modulation_Methods/Error_Probability_of_Direct-Sequence_Spread_Spectrum_Modulation#Principle_of_the_rake_receiver |Principle of the rake receiver]]. | ||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which statements are valid for the channel impulse response $h_{\rm K}(t)$? |
|type="[]"} | |type="[]"} | ||
| − | - | + | + $h_{\rm K}(t)$ consists of two Dirac delta functions. |
| − | + | - $h_{\rm K}(t)$ is complex-valued. | |
| + | - $h_{\rm K}(t)$ is a function periodic with delay time $\tau$. | ||
| + | {Which statements are true for the channel frequency response $H_{\rm K}(f)$? | ||
| + | |type="[]"} | ||
| + | - $H_{\rm K}(f = 0) = 2$ is true. | ||
| + | + $H_{\rm K}(f)$ is complex-valued. | ||
| + | + $|H_{\rm K}(f)|$ is a function periodic with frequency $1/τ$. | ||
| + | |||
| + | {Set $K = 1$, $h_0 = 0.6$ and $h_1 = 0.4$. Determine the delays $τ_0$ and $τ_1$ so that the $h_{\rm KR}(t)$ equation is satisfied with $A_0 = A_2$. | ||
| + | |type="{}"} | ||
| + | $τ_0 \ = \ $ { 1 3% } $\ \rm µ s$ | ||
| + | $τ_1 \ = \ $ { 0. } $\ \rm µ s$ | ||
| − | { | + | {What value should be chosen for the constant $K$? |
|type="{}"} | |type="{}"} | ||
| − | $\ | + | $K \ = \ $ { 1.923 3% } |
| + | |||
| + | {Which statements are valid for the signals $r(t)$ and $b(t)$? | ||
| + | |type="[]"} | ||
| + | + The maximum value of $r(t)$ is $1$. | ||
| + | - The width of $r(t)$ is $7 \ µ s$. | ||
| + | - The maximum value of $b(t)$ is $1$. | ||
| + | + The width of $b(t)$ is $7 \ µ s$. | ||
| + | |||
| Line 23: | Line 65: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' <u>Solution 1</u> is correct: |
| − | '''2 | + | *The impulse response $h_{\rm K}(t)$ is obtained as the received signal $r(t)$ when there is a Dirac delta pulse at the input ⇒ $s(t) = δ(t)$. It follows that: |
| − | '''3 | + | :$$ h_{\rm K}(t) = 0.6 \cdot \delta (t ) + 0.4 \cdot \delta (t - \tau) \hspace{0.05cm}.$$ |
| − | '''4 | + | |
| − | '''5 | + | |
| − | + | '''(2)''' <u>Solutions 2 and 3</u> are correct: | |
| − | + | *By definition, the channel frequency response $H_{\rm K}(f)$ is the Fourier transform of the impulse response $h_{\rm K}(t)$. With the shift theorem this results in: | |
| + | :$$H_{\rm K}(f) = 0.6 + 0.4 \cdot {\rm e}^{ \hspace{0.03cm}{\rm j} \hspace{0.03cm} \cdot \hspace{0.03cm}2 \pi f \tau}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} H_{\rm K}(f= 0) = 0.6 + 0.4 = 1 \hspace{0.05cm}.$$ | ||
| + | *Accordingly, the first proposed solution is incorrect in contrast to the other two: | ||
| + | # $H_{\rm K}(f)$ is complex-valued and | ||
| + | # the magnitude is periodic with $1/τ$, as the following calculation shows: | ||
| + | :$$|H_{\rm K}(f)|^2 = \left [0.6 + 0.4 \cdot \cos(2 \pi f \tau) \right ]^2 + \left [ 0.4 \cdot \sin(2 \pi f \tau) \right ]^2 = \left [0.6^2 + 0.4^2 \cdot \left ( \cos^2(2 \pi f \tau) + \sin^2(2 \pi f \tau)\right ) \right ] + 2 \cdot 0.6 \cdot 0.4 \cdot \cos(2 \pi f \tau).$$ | ||
| + | *For $f = 0$, $|H_{\rm K}(f)| = 1$. This value is repeated in the respective frequency spacing $1/τ$. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | '''(3)''' We first set $K = 1$ as agreed. | ||
| + | *Altogether we get from $s(t)$ to the output signal $b(t)$ via four paths. | ||
| + | *To satisfy the given $h_{\rm KR}(t)$ equation, either $τ_0 = 0$ must hold or $τ_1 = 0$. With $τ_0 = 0$ we obtain for the impulse response: | ||
| + | :$$h_{\rm KR}(t) = 0.6 \cdot h_0 \cdot \delta (t ) + 0.4 \cdot h_0 \cdot \delta (t - \tau) + 0.6 \cdot h_1 \cdot \delta (t -\tau_1) + 0.4 \cdot h_1 \cdot \delta (t - \tau-\tau_1) \hspace{0.05cm}.$$ | ||
| + | *To be able to focus the "main energy" at a certain time point, $τ_1 = τ$ would have to be chosen. | ||
| + | * With $h_0 = 0.6$ and $h_1 = 0.4$, we then obtain $A_0 ≠ A_2$: | ||
| + | :$$h_{\rm KR}(t) = 0.36 \cdot \delta (t ) +0.48 \cdot \delta (t - \tau) + 0.16 \cdot \delta (t - 2\tau)\hspace{0.05cm}.$$ | ||
| + | *In contrast, with $h_0 = 0.6$, $h_1 = 0.4$, $τ_0 = τ$ and $τ_1 = 0$: | ||
| + | :$$h_{\rm KR}(t) = 0.6 \cdot h_0 \cdot \delta (t - \tau ) + 0.4 \cdot h_0 \cdot \delta (t - 2\tau) + 0.6 \cdot h_1 \cdot \delta (t) + 0.4 \cdot h_1 \cdot \delta (t - \tau)= 0.24 \cdot \delta (t ) +0.52 \cdot \delta (t - \tau) + 0.24 \cdot \delta (t - 2\tau) \hspace{0.05cm}.$$ | ||
| + | *Here, the additional condition $A_0 = A_2$ is satisfied. Thus, the result we are looking for is: | ||
| + | :$$ \underline{\tau_0 = \tau = 1\,{\rm µ s} \hspace{0.05cm},\hspace{0.2cm}\tau_1 =0} \hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | |||
| + | '''(4)''' The following must apply to the normalization factor: | ||
| + | :$$K= \frac{1}{h_0^2 + h_1^2} = \frac{1}{0.6^2 + 0.4^2} = \frac{1}{0.52} \hspace{0.15cm}\underline {\approx 1.923} \hspace{0.05cm}.$$ | ||
| + | *This gives for the common impulse response $($it holds $0.24/0.52 = 6/13)$: | ||
| + | :$$ h_{\rm KR}(t) = \frac{6}{13} \cdot \delta (t ) + 1.00 \cdot \delta (t - \tau) + \frac{6}{13} \cdot \delta (t - 2\tau)\hspace{0.05cm}.$$ | ||
| + | |||
| + | |||
| + | |||
| + | [[File:P_ID1902__Mod_Z_5_5e.png|right|frame|Signals to illustrate the rake receiver]] | ||
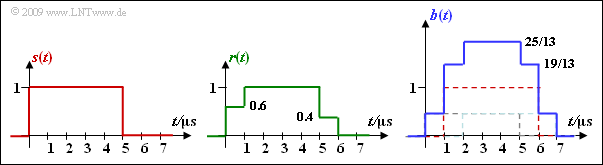
| + | '''(5)''' <u>Statements 1 and 4</u> are correct, as shown in the diagram: | ||
| + | *For the received signal $r(t)$ holds: | ||
| + | :$$r(t) = 0.6 \cdot s(t) + 0.4 \cdot s (t - 1\,{\rm µ s})\hspace{0.05cm},$$ | ||
| + | *and for the rake output signal $b(t)$: | ||
| + | :$$b(t) = \frac{6}{13} \cdot s(t) + 1 \cdot s (t - 1\,{\rm µ s}) + \frac{6}{13} \cdot s (t - 2\,{\rm µ s}) \hspace{0.05cm}.$$ | ||
| + | *The overshoot of the output signal ⇒ $b(t) > 1$ is due to the normalization factor $K = 25/13$. | ||
| + | *With $K = 1$, the maximum value of $b(t)$ would actually be $1$. | ||
| + | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Modulation Methods: Exercises|^5.4 BER of the PN Modulation^]] |
Latest revision as of 16:03, 7 February 2023
The diagram shows a two-way channel (yellow background). The corresponding descriptive equation is:
- $$ r(t) =0.6 \cdot s(t) + 0.4 \cdot s (t - \tau) \hspace{0.05cm}.$$
Let the delay on the secondary path be $τ = 1 \ \rm µ s$.
Drawn below is the structure of a rake receiver (green background) with general coefficients $K$, $h_0$, $h_1$, $τ_0$ and $τ_1$.
- The purpose of the rake receiver is to combine the energy of the two signal paths, making the decision more reliable.
- The combined impulse response of the channel (German: "Kanal" ⇒ subscript "K") and the rake receiver can be expressed in the form
- $$h_{\rm KR}(t) = A_0 \cdot \delta (t ) + A_1 \cdot \delta (t - \tau) + A_2 \cdot \delta (t - 2\tau)$$
- but only if the rake coefficients $h_0$, $h_1$, $τ_0$ and $τ_1$ are appropriately chosen.
- The main part of $h_{\rm KR}(t)$ is supposed to be at $t = τ$.
- The constant $K$ is to be chosen so that the amplitude of the main path $A_1 = 1$ :
- $$K= \frac{1}{h_0^2 + h_1^2}.$$
Apart from the rake parameters, the signals $r(t)$ and $b(t)$ are sought when $s(t)$ is a rectangle of height $s_0 = 1$ and width $T = \ \rm 5 µ s$.
Notes:
- The exercise belongs to the chapter Error Probability of Direct-Sequence Spread Spectrum Modulation.
- Reference is made in particular to the section Principle of the rake receiver.
Questions
Solution
(1) Solution 1 is correct:
- The impulse response $h_{\rm K}(t)$ is obtained as the received signal $r(t)$ when there is a Dirac delta pulse at the input ⇒ $s(t) = δ(t)$. It follows that:
- $$ h_{\rm K}(t) = 0.6 \cdot \delta (t ) + 0.4 \cdot \delta (t - \tau) \hspace{0.05cm}.$$
(2) Solutions 2 and 3 are correct:
- By definition, the channel frequency response $H_{\rm K}(f)$ is the Fourier transform of the impulse response $h_{\rm K}(t)$. With the shift theorem this results in:
- $$H_{\rm K}(f) = 0.6 + 0.4 \cdot {\rm e}^{ \hspace{0.03cm}{\rm j} \hspace{0.03cm} \cdot \hspace{0.03cm}2 \pi f \tau}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} H_{\rm K}(f= 0) = 0.6 + 0.4 = 1 \hspace{0.05cm}.$$
- Accordingly, the first proposed solution is incorrect in contrast to the other two:
- $H_{\rm K}(f)$ is complex-valued and
- the magnitude is periodic with $1/τ$, as the following calculation shows:
- $$|H_{\rm K}(f)|^2 = \left [0.6 + 0.4 \cdot \cos(2 \pi f \tau) \right ]^2 + \left [ 0.4 \cdot \sin(2 \pi f \tau) \right ]^2 = \left [0.6^2 + 0.4^2 \cdot \left ( \cos^2(2 \pi f \tau) + \sin^2(2 \pi f \tau)\right ) \right ] + 2 \cdot 0.6 \cdot 0.4 \cdot \cos(2 \pi f \tau).$$
- For $f = 0$, $|H_{\rm K}(f)| = 1$. This value is repeated in the respective frequency spacing $1/τ$.
(3) We first set $K = 1$ as agreed.
- Altogether we get from $s(t)$ to the output signal $b(t)$ via four paths.
- To satisfy the given $h_{\rm KR}(t)$ equation, either $τ_0 = 0$ must hold or $τ_1 = 0$. With $τ_0 = 0$ we obtain for the impulse response:
- $$h_{\rm KR}(t) = 0.6 \cdot h_0 \cdot \delta (t ) + 0.4 \cdot h_0 \cdot \delta (t - \tau) + 0.6 \cdot h_1 \cdot \delta (t -\tau_1) + 0.4 \cdot h_1 \cdot \delta (t - \tau-\tau_1) \hspace{0.05cm}.$$
- To be able to focus the "main energy" at a certain time point, $τ_1 = τ$ would have to be chosen.
- With $h_0 = 0.6$ and $h_1 = 0.4$, we then obtain $A_0 ≠ A_2$:
- $$h_{\rm KR}(t) = 0.36 \cdot \delta (t ) +0.48 \cdot \delta (t - \tau) + 0.16 \cdot \delta (t - 2\tau)\hspace{0.05cm}.$$
- In contrast, with $h_0 = 0.6$, $h_1 = 0.4$, $τ_0 = τ$ and $τ_1 = 0$:
- $$h_{\rm KR}(t) = 0.6 \cdot h_0 \cdot \delta (t - \tau ) + 0.4 \cdot h_0 \cdot \delta (t - 2\tau) + 0.6 \cdot h_1 \cdot \delta (t) + 0.4 \cdot h_1 \cdot \delta (t - \tau)= 0.24 \cdot \delta (t ) +0.52 \cdot \delta (t - \tau) + 0.24 \cdot \delta (t - 2\tau) \hspace{0.05cm}.$$
- Here, the additional condition $A_0 = A_2$ is satisfied. Thus, the result we are looking for is:
- $$ \underline{\tau_0 = \tau = 1\,{\rm µ s} \hspace{0.05cm},\hspace{0.2cm}\tau_1 =0} \hspace{0.05cm}.$$
(4) The following must apply to the normalization factor:
- $$K= \frac{1}{h_0^2 + h_1^2} = \frac{1}{0.6^2 + 0.4^2} = \frac{1}{0.52} \hspace{0.15cm}\underline {\approx 1.923} \hspace{0.05cm}.$$
- This gives for the common impulse response $($it holds $0.24/0.52 = 6/13)$:
- $$ h_{\rm KR}(t) = \frac{6}{13} \cdot \delta (t ) + 1.00 \cdot \delta (t - \tau) + \frac{6}{13} \cdot \delta (t - 2\tau)\hspace{0.05cm}.$$
(5) Statements 1 and 4 are correct, as shown in the diagram:
- For the received signal $r(t)$ holds:
- $$r(t) = 0.6 \cdot s(t) + 0.4 \cdot s (t - 1\,{\rm µ s})\hspace{0.05cm},$$
- and for the rake output signal $b(t)$:
- $$b(t) = \frac{6}{13} \cdot s(t) + 1 \cdot s (t - 1\,{\rm µ s}) + \frac{6}{13} \cdot s (t - 2\,{\rm µ s}) \hspace{0.05cm}.$$
- The overshoot of the output signal ⇒ $b(t) > 1$ is due to the normalization factor $K = 25/13$.
- With $K = 1$, the maximum value of $b(t)$ would actually be $1$.