Difference between revisions of "Aufgaben:Exercise 3.3Z: Rectangular Pulse and Dirac Delta"
From LNTwww
m (Guenter moved page Exercise 3.3Z: Rectangular Pulse and Dirac Impulse to Exercise 3.3Z: Rectangular Pulse and Dirac Delta) |
|||
| (36 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Signal_Representation/Special_Cases_of_Impulse_Signals |
}} | }} | ||
| − | [[File:P_ID507__Sig_Z_3_3.png|right| | + | [[File:P_ID507__Sig_Z_3_3.png|right|frame|Various rectangular pulses]] |
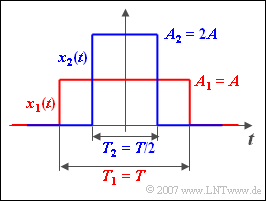
| − | + | We consider here a multitude of symmetrical rectangular functions $x_k(t)$. The individual rectangles differ in amplitudes (heights) | |
:$$A_k = k \cdot A$$ | :$$A_k = k \cdot A$$ | ||
| − | + | and different pulse durations (widths) | |
:$$T_k = T/k.$$ | :$$T_k = T/k.$$ | ||
| − | + | Let $k$ be any positive value. | |
| − | + | *The rectangular pulse $x_1(t)$ shown in red has the amplitude $A_1 = {A} = 2 \,\text{V}$ and the duration $T_1 = {T} = 500 \,µ\text{s}$. | |
| − | * | + | *The pulse $x_2(t)$ shown in blue is half as wide ⇒ $T_2 =250 \,µ\text{s}$, but twice as high ⇒ $A_2 = 4 \text{ V}$. |
| − | * | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | === | + | ''Hints:'' |
| + | *This task belongs to the chapter [[Signal_Representation/Special_Cases_of_Pulses|Special Cases of Pulses]]. | ||
| + | *Use one of the functions $\text{si}(x) = \sin(x)/x$ or $\text{sinc}(x) = \sin(\pi x)/(\pi x)$. | ||
| + | |||
| + | *You can check your results using the two interactive applets | ||
| + | :[[Applets:Pulses_and_Spectra|Pulses and Spectra]], | ||
| + | :[[Applets:Frequency_%26_Impulse_Responses|Frequency & Impulse Responses]]. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the following statements are true regarding the spectrum $X_1(f)$? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The spectral value $X_1(f = 0)$ is equal to $10^{–3} \,\text{V/Hz}$. |
| − | + $X_1(f)$ | + | + $X_1(f)$ has zeros at the interval of $2 \,\text{kHz}$. |
| − | - $X_1(f)$ | + | - $X_1(f)$ has zeros at the interval of $4 \,\text{kHz}$. |
| − | { | + | {Which of the following statements are true regarding the spectrum $X_2(f)$? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The spectral value is $X_2(f = 0)$ is equal to $10^{–3} \,\text{V/Hz}$. |
| − | - $X_2(f)$ | + | - $X_2(f)$ has zeros at the interval of $2\, \text{kHz}$. |
| − | + $X_2(f)$ | + | + $X_2(f)$ has zeros at the interval of $4 \,\text{kHz}$. |
| − | { | + | {Let $k = 10$. Calculate the frequency $f_{10}$ of the first zero and the spectral value at $f = 2 \,\text{kHz}$. |
|type="{}"} | |type="{}"} | ||
| − | $f_{10}$ | + | $f_{10} \ = \ ${ 20 3% } $\text{kHz}$ |
| − | $X_{10}(f = 2 \text{kHz})$ | + | $X_{10}(f = 2 \text{kHz})\ = \ $ { 0.984 3% } $\text{mV/Hz}$ |
| − | { | + | {What is the spectral value at $f = 2 \,\text{kHz}$ in the limiting case $k \rightarrow \infty$? Interpret the result. |
|type="{}"} | |type="{}"} | ||
| − | $X_{\infty}(f = 2 \text{kHz}$ | + | $X_{\infty}(f = 2 \,\text{kHz})\ = \ $ { 1 3% } $\text{mV/Hz}$ |
| Line 57: | Line 62: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solutions=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' The <u>proposed solutions 1 and 2</u> are correct: |
| + | *The spectral value at frequency $f = 0$ is always equal to the area under the time function according to [[Signal_Representation/Fourier_Transform_and_Its_Inverse#The_First_Fourier_Integral|the first Fourier integral]] : | ||
:$$X( f ) = \int_{ - \infty }^{ + \infty } {x( t )} \cdot {\rm{e}}^{ - {\rm{j2\pi }}ft} \hspace{0.1cm} {\rm d}t \hspace{0.5cm} \Rightarrow \hspace{0.5cm} \;X( {f = 0} ) = \int_{ - \infty }^{ + \infty } {x( t )}\hspace{0.1cm} {\rm d}t.$$ | :$$X( f ) = \int_{ - \infty }^{ + \infty } {x( t )} \cdot {\rm{e}}^{ - {\rm{j2\pi }}ft} \hspace{0.1cm} {\rm d}t \hspace{0.5cm} \Rightarrow \hspace{0.5cm} \;X( {f = 0} ) = \int_{ - \infty }^{ + \infty } {x( t )}\hspace{0.1cm} {\rm d}t.$$ | ||
| − | + | *In the present case, the pulse area is always $A \cdot T = 10^{–3} \,\text{Vs} = 1\, \text{mV/Hz}$. | |
| + | *Because of $T_1 = 500 \,µ\text{s}$ the spectrum $X_1(f)$ has zero crossings at the interval $f_1 = 1/T_1 = 2 \,\text{kHz}$ . | ||
| + | |||
| + | |||
| + | '''(2)''' The <u>proposed solutions 1 and 3</u> are correct: | ||
| + | *Due to equal pulse areas, the spectral value is not changed at the frequency $f = 0$ . | ||
| + | *The equidistant zero crossings now occur at the interval $f_2 = 1/T_2 = 4 \,\text{kHz}$. | ||
| − | |||
| − | |||
| − | '''3 | + | '''(3)''' Zero crossings occur at multiples of $f_{10} = 1/T_{10} = 20 \,\text{kHz}$, and the spectral function is: |
:$$X_{10} ( f ) = X_0 \cdot {\mathop{\rm si}\nolimits} ( {{\rm{\pi }}f/f_{10} } ).$$ | :$$X_{10} ( f ) = X_0 \cdot {\mathop{\rm si}\nolimits} ( {{\rm{\pi }}f/f_{10} } ).$$ | ||
| − | + | *At frequency $f = 2 \,\text{kHz}$ the argument of the $\rm si$-function is equal to $\pi/10$ $($or $18^{\circ})$: | |
| − | :$$X_{10} ( {f = 2\;{\rm{kHz}}}) = 10^{ - 3} \;{\rm{V/Hz}} \cdot \frac{{\sin ( {18^\circ } )}}{{{\rm{\pi /10}}}} \hspace{0.15 cm}\underline{= 0.984 | + | :$$X_{10} ( {f = 2\;{\rm{kHz}}}) = 10^{ - 3} \;{\rm{V/Hz}} \cdot \frac{{\sin ( {18^\circ } )}}{{{\rm{\pi /10}}}} \hspace{0.15 cm}\underline{= 0.984 \;{\rm{mV/Hz}}}{\rm{.}}$$ |
| − | '''4 | + | |
| + | '''(4)''' In the limiting case $k \rightarrow \infty$ the then infinitely high and infinitely narrow [[Signal_Representation/Special_Cases_of_Pulses#Rectangular_pulse|Rectangular pulse]] changes into the [[Signal_Representation/Special_Cases_of_Pulses#Dirac_.28delta.29_impulse|Dirac delta impulse]]. | ||
| + | *Its spectrum is constant for all frequencies. | ||
| + | *Thus the spectral value $X_{\infty}(f = 2 \,\text{kHz})\hspace{0.15 cm}\underline{=1 \text{ mV/Hz}}$ also applies at the frequency $f = 2 \,\text{kHz}$ . | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^3.2 Special Cases of Pulses^]] |
Latest revision as of 17:42, 25 May 2021
We consider here a multitude of symmetrical rectangular functions $x_k(t)$. The individual rectangles differ in amplitudes (heights)
- $$A_k = k \cdot A$$
and different pulse durations (widths)
- $$T_k = T/k.$$
Let $k$ be any positive value.
- The rectangular pulse $x_1(t)$ shown in red has the amplitude $A_1 = {A} = 2 \,\text{V}$ and the duration $T_1 = {T} = 500 \,µ\text{s}$.
- The pulse $x_2(t)$ shown in blue is half as wide ⇒ $T_2 =250 \,µ\text{s}$, but twice as high ⇒ $A_2 = 4 \text{ V}$.
Hints:
- This task belongs to the chapter Special Cases of Pulses.
- Use one of the functions $\text{si}(x) = \sin(x)/x$ or $\text{sinc}(x) = \sin(\pi x)/(\pi x)$.
- You can check your results using the two interactive applets
Questions
Solutions
(1) The proposed solutions 1 and 2 are correct:
- The spectral value at frequency $f = 0$ is always equal to the area under the time function according to the first Fourier integral :
- $$X( f ) = \int_{ - \infty }^{ + \infty } {x( t )} \cdot {\rm{e}}^{ - {\rm{j2\pi }}ft} \hspace{0.1cm} {\rm d}t \hspace{0.5cm} \Rightarrow \hspace{0.5cm} \;X( {f = 0} ) = \int_{ - \infty }^{ + \infty } {x( t )}\hspace{0.1cm} {\rm d}t.$$
- In the present case, the pulse area is always $A \cdot T = 10^{–3} \,\text{Vs} = 1\, \text{mV/Hz}$.
- Because of $T_1 = 500 \,µ\text{s}$ the spectrum $X_1(f)$ has zero crossings at the interval $f_1 = 1/T_1 = 2 \,\text{kHz}$ .
(2) The proposed solutions 1 and 3 are correct:
- Due to equal pulse areas, the spectral value is not changed at the frequency $f = 0$ .
- The equidistant zero crossings now occur at the interval $f_2 = 1/T_2 = 4 \,\text{kHz}$.
(3) Zero crossings occur at multiples of $f_{10} = 1/T_{10} = 20 \,\text{kHz}$, and the spectral function is:
- $$X_{10} ( f ) = X_0 \cdot {\mathop{\rm si}\nolimits} ( {{\rm{\pi }}f/f_{10} } ).$$
- At frequency $f = 2 \,\text{kHz}$ the argument of the $\rm si$-function is equal to $\pi/10$ $($or $18^{\circ})$:
- $$X_{10} ( {f = 2\;{\rm{kHz}}}) = 10^{ - 3} \;{\rm{V/Hz}} \cdot \frac{{\sin ( {18^\circ } )}}{{{\rm{\pi /10}}}} \hspace{0.15 cm}\underline{= 0.984 \;{\rm{mV/Hz}}}{\rm{.}}$$
(4) In the limiting case $k \rightarrow \infty$ the then infinitely high and infinitely narrow Rectangular pulse changes into the Dirac delta impulse.
- Its spectrum is constant for all frequencies.
- Thus the spectral value $X_{\infty}(f = 2 \,\text{kHz})\hspace{0.15 cm}\underline{=1 \text{ mV/Hz}}$ also applies at the frequency $f = 2 \,\text{kHz}$ .