Difference between revisions of "Aufgaben:Exercise 4.4Z: Pointer Diagram for SSB-AM"
| (31 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Signal_Representation/Analytical_Signal_and_Its_Spectral_Function |
}} | }} | ||
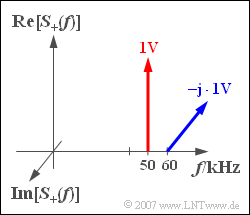
| − | [[File:P_ID732__Sig_Z_4_4_neu.png|right| | + | [[File:P_ID732__Sig_Z_4_4_neu.png|right|frame|Given analytical spectrum S+(f)]] |
| − | + | ||
| + | The analytical signal s+(t) with the line spectrum | ||
:$$S_{+}(f) = {\rm 1 \hspace{0.05cm} V} \cdot\delta (f - f_{\rm | :$$S_{+}(f) = {\rm 1 \hspace{0.05cm} V} \cdot\delta (f - f_{\rm | ||
50})- {\rm j} \cdot {\rm 1 \hspace{0.05cm} V} \cdot\delta (f - | 50})- {\rm j} \cdot {\rm 1 \hspace{0.05cm} V} \cdot\delta (f - | ||
| − | f_{\rm 60}) | + | f_{\rm 60})$$ |
| − | + | is to be considered. | |
| + | Here f50 and f60 are abbreviations for the frequencies 50 kHz and 60 kHz, respectively. | ||
| + | |||
| + | This analytical signal could occur, for example, with the [[Modulation_Methods/Einseitenbandmodulation|Single Sideband Amplitude Modulation]] (SSB–AM) of a sinusoidal message signal (Frequenz fN=10 kHz) with a cosinusoidal carrier signal $(f_{\rm T} = 50 \ \text{kHz}) , whereby only the upper sideband is transmitted ⇒ \text{Upper Sideband Modulation}$. | ||
| + | |||
| + | However, the analytical signal could also result from a Lower Sideband Modulation of the same sinusoidal signal if a sinusoidal carrier with frequency $f_{\rm T} = 60 \ \text{kHz}$ is used. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | ''Hints:'' | ||
| + | *This exercise belongs to the chapter [[Signal_Representation/Analytical_Signal_and_Its_Spectral_Function|Analytical Signal and its Spectral Function]]. | ||
| + | |||
| + | *You can check your solution with the interaction module [[Applets:Physical_Signal_%26_Analytic_Signal|Physical and Analytical Signal]]. | ||
| − | === | + | |
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Give the analytical signal s+(t) as a formula. What value results at the starting time t=0? |
|type="{}"} | |type="{}"} | ||
| − | Re[s+(t=0)] | + | $\text{Re}[s_+(t = 0)]\ = \ $ { 1 3% } V |
| − | Im[s+(t=0)] | + | $\text{Im}[s_+(t = 0)]\ = \ { -1.03--0.97 } \text{V}$ |
| − | { | + | {At what time t1 does the first zero crossing of the physical signal s(t) occur relative to the first zero crossing of the $50 \ \text{kHz-cosine signal}$ ? <br>''Note:'' The latter is at time $T_0/4 = 1/(4 \cdot f_{50}) = 5 \ µ \text{s}$. |
| − | |type=" | + | |type="()"} |
| − | - | + | - It is $t_1 < 5 \ {\rm µ} \text{s}$. |
| − | - | + | - It is $t_1 = 5 \ {\rm µ}\text{s}$. |
| − | + | + | + It is $t_1 > 5 \ {\rm µ} \text{s}$. |
| − | { | + | {What is the maximum value of |s+(t)|? At what time t2 is this maximum value reached for the first time? |
|type="{}"} | |type="{}"} | ||
| − | |s+(t)|max | + | $|s_+(t)|_{\rm max}\ = \ { 2 3% } \text{V}$ |
| − | t2 | + | $t_2\ = \ { 25 3% } {\rm µ s}$ |
| − | { | + | {At what time t3 is the pointer length |s+(t)| equal to zero for the first time? |
|type="{}"} | |type="{}"} | ||
| − | t3 | + | $t_3\ = \ { 75 3% } {\rm µ s}$ |
| Line 50: | Line 57: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
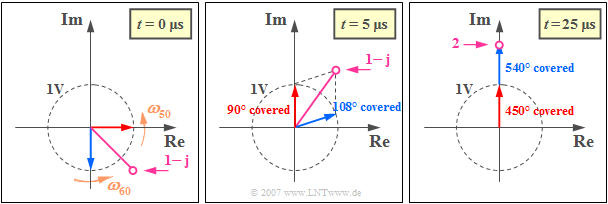
| − | '''1 | + | [[File:EN_Sig_Z_4_4_ML.png|right|frame|Three different analytical signals]] |
| + | '''(1)''' The analytical signal is generally: | ||
:$$s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | :$$s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | ||
j}\hspace{0.05cm} \omega_{\rm 50}\hspace{0.05cm} t } - {\rm | j}\hspace{0.05cm} \omega_{\rm 50}\hspace{0.05cm} t } - {\rm | ||
j}\cdot{\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | j}\cdot{\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm | ||
j}\hspace{0.05cm} \omega_{\rm 60} \hspace{0.05cm} t }.$$ | j}\hspace{0.05cm} \omega_{\rm 60} \hspace{0.05cm} t }.$$ | ||
| − | + | At time t=0 the complex exponential functions each take the value 1 and one obtains (see left graph): | |
| − | + | *$\text{Re}[s_+(t = 0)] \; \underline{= +1\ \text{V}}$, | |
| − | + | *$\text{Im}[s_+(t = 0)]\; \underline{ = \,-\hspace{-0.08cm}1\ \text{V}}$. | |
| − | + | <br clear=all> | |
| − | + | '''(2)''' For the analytical signal it can also be written: | |
| − | '''2 | ||
:$$s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm | :$$s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm | ||
50}\hspace{0.05cm} t }) + {\rm j} \cdot{\rm 1 \hspace{0.05cm} V} | 50}\hspace{0.05cm} t }) + {\rm j} \cdot{\rm 1 \hspace{0.05cm} V} | ||
| − | \cdot \sin({ \omega_{\rm 50}\hspace{0.05cm} t }) | + | \cdot \sin({ \omega_{\rm 50}\hspace{0.05cm} t }) - {\rm j} |
\cdot | \cdot | ||
{\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm | {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm | ||
60}\hspace{0.05cm} t }) + {\rm 1 \hspace{0.05cm} V} \cdot \sin({ | 60}\hspace{0.05cm} t }) + {\rm 1 \hspace{0.05cm} V} \cdot \sin({ | ||
\omega_{\rm 60}\hspace{0.05cm} t }).$$ | \omega_{\rm 60}\hspace{0.05cm} t }).$$ | ||
| − | + | The real part of this describes the actual physical signal: | |
:$$s(t) = {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm | :$$s(t) = {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm | ||
50}\hspace{0.05cm} t }) + {\rm 1 \hspace{0.05cm} V} \cdot \sin({ | 50}\hspace{0.05cm} t }) + {\rm 1 \hspace{0.05cm} V} \cdot \sin({ | ||
\omega_{\rm 60}\hspace{0.05cm} t }).$$ | \omega_{\rm 60}\hspace{0.05cm} t }).$$ | ||
| − | |||
| − | + | Correct is the <u>proposed solution 3</u>: | |
| + | *Considering the 50 kHz cosine signal alone, the first zero crossing would occur at t1=T0/4 , i.e. after 5 \ {\rm µ s}, where T_0 = 1/f_{50} = 20 \ {\rm µ s} denotes the period duration of this signal. | ||
| + | *The sinusoidal signal with the frequency 60 kHz is positive during the entire first half-wave (0 \, \text{...} \, 8.33\ {\rm µ s}) . | ||
| + | *Due to the plus sign, the first zero crossing of s(t) \ \Rightarrow \ t_1 > 5\ {\rm µ s} is delayed. | ||
| + | *The middle graph shows the analytical signal at time t=T0/4, when the red carrier would have its zero crossing. | ||
| + | *The zero crossing of the violet cumulative pointer only occurs when it points in the direction of the imaginary axis. Then s(t1)=Re[s+(t1)]=0. | ||
| + | |||
| − | '''3 | + | '''(3)''' The maximum value of |s+(t)| is reached when both pointers point in the same direction. The magnitude of the sum pointer is then equal to the sum of the two individual pointers; i.e. 2 V_. |
| − | + | This case is reached for the first time when the faster pointer with circular velocity ω60 has caught up its "lag" of 90∘(π/2) with the slower pointer (ω50) : | |
:$$\omega_{\rm 60} \cdot t_2 - \omega_{\rm | :$$\omega_{\rm 60} \cdot t_2 - \omega_{\rm | ||
50}\cdot t_2 = \frac{\pi}{2} \hspace{0.3cm} | 50}\cdot t_2 = \frac{\pi}{2} \hspace{0.3cm} | ||
\Rightarrow\hspace{0.3cm}t_2 = \frac{\pi/2}{2\pi (f_{\rm 60}- | \Rightarrow\hspace{0.3cm}t_2 = \frac{\pi/2}{2\pi (f_{\rm 60}- | ||
f_{\rm 50})} = \frac{1}{4 | f_{\rm 50})} = \frac{1}{4 | ||
| − | \cdot(f_{\rm 60}- f_{\rm 50})}\hspace{0.15 cm}\underline{= {\rm 25 \hspace{0.05cm} \ | + | \cdot(f_{\rm 60}- f_{\rm 50})}\hspace{0.15 cm}\underline{= {\rm 25 \hspace{0.05cm} {\rm µ s}}}.$$ |
| − | + | *At this point, the two pointers have made 5/4 and 6/4 rotations respectively and both point in the direction of the imaginary axis (see right graph). | |
| + | *The actual physical signal s(t) – i.e. the real part of s+(t) – is therefore zero at this moment. | ||
| + | |||
| + | |||
| + | |||
| + | '''(4)''' The condition for $|s_+(t_3)| = 0$ is that there is a phase offset of 180∘ between the two equally long pointers so that they cancel each other out. | ||
| + | *This further means that the faster pointer has rotated 3π/2 further than the 50 kHz component. | ||
| − | ''' | + | *Analogous to the sample solution of sub-task '''(3)''' , therefore the following applies: |
:$$t_3 = \frac{3\pi/2}{2\pi (f_{\rm 60}- f_{\rm 50})} \hspace{0.15 cm}\underline{= | :$$t_3 = \frac{3\pi/2}{2\pi (f_{\rm 60}- f_{\rm 50})} \hspace{0.15 cm}\underline{= | ||
| − | {\rm 75 \hspace{0.05cm} \ | + | {\rm 75 \hspace{0.05cm} {\rm µ s}}}.$$ |
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
| − | [[Category: | + | [[Category:Signal Representation: Exercises|^4.2 Analytical Signal and its Spectral Function^]] |
Latest revision as of 05:15, 18 September 2022
The analytical signal s+(t) with the line spectrum
- S+(f)=1V⋅δ(f−f50)−j⋅1V⋅δ(f−f60)
is to be considered. Here f50 and f60 are abbreviations for the frequencies 50 kHz and 60 kHz, respectively.
This analytical signal could occur, for example, with the Single Sideband Amplitude Modulation (SSB–AM) of a sinusoidal message signal (Frequenz fN=10 kHz) with a cosinusoidal carrier signal (fT=50 kHz) , whereby only the upper sideband is transmitted ⇒ Upper Sideband Modulation.
However, the analytical signal could also result from a Lower Sideband Modulation of the same sinusoidal signal if a sinusoidal carrier with frequency fT=60 kHz is used.
Hints:
- This exercise belongs to the chapter Analytical Signal and its Spectral Function.
- You can check your solution with the interaction module Physical and Analytical Signal.
Questions
Solution
(1) The analytical signal is generally:
- s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} \omega_{\rm 50}\hspace{0.05cm} t } - {\rm j}\cdot{\rm 1 \hspace{0.05cm} V} \cdot {\rm e}^{{\rm j}\hspace{0.05cm} \omega_{\rm 60} \hspace{0.05cm} t }.
At time t = 0 the complex exponential functions each take the value 1 and one obtains (see left graph):
- \text{Re}[s_+(t = 0)] \; \underline{= +1\ \text{V}},
- \text{Im}[s_+(t = 0)]\; \underline{ = \,-\hspace{-0.08cm}1\ \text{V}}.
(2) For the analytical signal it can also be written:
- s_{+}(t) = {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm 50}\hspace{0.05cm} t }) + {\rm j} \cdot{\rm 1 \hspace{0.05cm} V} \cdot \sin({ \omega_{\rm 50}\hspace{0.05cm} t }) - {\rm j} \cdot {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm 60}\hspace{0.05cm} t }) + {\rm 1 \hspace{0.05cm} V} \cdot \sin({ \omega_{\rm 60}\hspace{0.05cm} t }).
The real part of this describes the actual physical signal:
- s(t) = {\rm 1 \hspace{0.05cm} V} \cdot \cos({ \omega_{\rm 50}\hspace{0.05cm} t }) + {\rm 1 \hspace{0.05cm} V} \cdot \sin({ \omega_{\rm 60}\hspace{0.05cm} t }).
Correct is the proposed solution 3:
- Considering the 50 \ \text{kHz} cosine signal alone, the first zero crossing would occur at t_1 = T_0/4 , i.e. after 5 \ {\rm µ s}, where T_0 = 1/f_{50} = 20 \ {\rm µ s} denotes the period duration of this signal.
- The sinusoidal signal with the frequency 60 \ \text{kHz} is positive during the entire first half-wave (0 \, \text{...} \, 8.33\ {\rm µ s}) .
- Due to the plus sign, the first zero crossing of s(t) \ \Rightarrow \ t_1 > 5\ {\rm µ s} is delayed.
- The middle graph shows the analytical signal at time t = T_0/4, when the red carrier would have its zero crossing.
- The zero crossing of the violet cumulative pointer only occurs when it points in the direction of the imaginary axis. Then s(t_1) = \text{Re}[s_+(t_1)] = 0.
(3) The maximum value of |s_+(t)| is reached when both pointers point in the same direction. The magnitude of the sum pointer is then equal to the sum of the two individual pointers; i.e. \underline {2\ \text{V}}.
This case is reached for the first time when the faster pointer with circular velocity \omega_{60} has caught up its "lag" of 90^{\circ} \; (\pi /2) with the slower pointer (\omega_{50}) :
- \omega_{\rm 60} \cdot t_2 - \omega_{\rm 50}\cdot t_2 = \frac{\pi}{2} \hspace{0.3cm} \Rightarrow\hspace{0.3cm}t_2 = \frac{\pi/2}{2\pi (f_{\rm 60}- f_{\rm 50})} = \frac{1}{4 \cdot(f_{\rm 60}- f_{\rm 50})}\hspace{0.15 cm}\underline{= {\rm 25 \hspace{0.05cm} {\rm µ s}}}.
- At this point, the two pointers have made 5/4 and 6/4 rotations respectively and both point in the direction of the imaginary axis (see right graph).
- The actual physical signal s(t) – i.e. the real part of s_+(t) – is therefore zero at this moment.
(4) The condition for |s_+(t_3)| = 0 is that there is a phase offset of 180^\circ between the two equally long pointers so that they cancel each other out.
- This further means that the faster pointer has rotated 3\pi /2 further than the 50 \ \text{kHz} component.
- Analogous to the sample solution of sub-task (3) , therefore the following applies:
- t_3 = \frac{3\pi/2}{2\pi (f_{\rm 60}- f_{\rm 50})} \hspace{0.15 cm}\underline{= {\rm 75 \hspace{0.05cm} {\rm µ s}}}.